IB Docs (2) Team
Chi squared: Practice problems
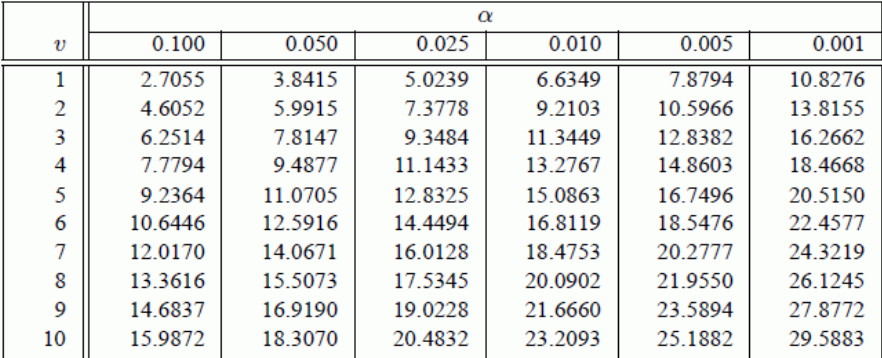
Below you will find three problems that are based on research that you may study in the IB psychology course. For each problem, decide whether the data is significant by applying the chi-squared of difference. You may either do the problems by hand or use the chi-square calculator. If you are calculating the chi-squared by hand, you will need to use the following critical values table. Remember, the value in the first column is the degrees of freedom.
Problem 1. Snacking habits in men and women.
In a study in health psychology, they wanted to see if there are gender differences in one’s choice of high fat, high protein or high sugar foods when choosing a snack. The sample was made up of 42 men and 36 women. The results are below:
| Type of snack | Men | Women |
| High fat | 8 | 12 |
| High protein | 21 | 8 |
| High sugar | 13 | 16 |
Please note that there are three different choices here, so the degrees of freedom will be different from the example above.
Problem 2. Training people to be more prosocial
A study by Beaman et al wanted to see if giving a lecture on helping behaviour would lead to a person helping someone a week later in a staged helping scenario. There were 80 participants. 40 of the participants listened to a lecture on helping research, including videos of the original studies. The other 40 participants were given a lecture on obesity and emotions - in other words, a study not focused on helping. In the helping lecture, 17 of the participants helped. In the control condition - the obesity lecture - 10 of the participants helped. Is this a significant difference?
Problem 3: Studies of attraction
In a replication of a study by Dion wanted to see if the attractiveness of an individual would lead to female participants automatically assigning positive or negative traits to an individual. The participants were shown three different photos: an attractive male, an average-looking male, and an unattractive male. They were then given a set of traits and asked to rate the male on those traits. This gave a composite score that was either “positive”, “negative” or “neutral.” There were 200 participants. The final scores are below. Is this a significant finding?
| Personality rating | Unattractive | Average attraction | Attractive |
| Positive personality | 61 | 65 | 94 |
| Neutral personality | 65 | 54 | 56 |
| Negative personality | 74 | 81 | 50 |
Twitter
Facebook
LinkedIn