Pause for thought

Hydrogen gas discharge tube

Each element has a unique atomic spectrum which consists of discrete lines and is therefore not continuous. The simplest element, hydrogen has lines in the visible region at wavelengths of 656 nm, 486 nm, 434 nm 410 nm etc. which converge at the higher energy end of the spectrum at 365 nm.

These lines are governed by a simple mathematical relationship: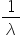

= R (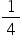 − 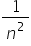) where n = 3 (to give 656 nm), 4 (to give 486 nm), 5,6….. etc. R is known as the Rydberg constant.

It can be seen that as 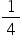is also 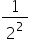this equation is a special case of a more general equation:
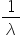= R ( 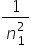− 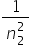) where n₁ = 1, 2, 3, 4….. and n₂ = (n₁+1), (n₁+2), (n₁+3), (n₁+4)……

If n₁ = 1 then the series of lines will be of higher energy (shorter wavelength) and appear in the ultraviolet region of the spectrum. n₁= 2 gives the lines in the visible region and n₁ =3 will give a series of lines in the infra-red region etc.

For emission spectra these series of lines represent electrons falling from higher to lower energy levels.

Evidence that they do relate to the energy levels that can be occupied by the electron can be obtained by considering promoting an electron from the lowest possible energy level to infinity, i.e. removing the electron completely, in other words ionizing the atom. In this case n₁= 1 and n₂ = infinity.

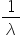= R (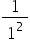 − 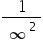) so that 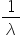= R. Since c = λv and E= hv then

E = hcR for one electron or hcRL for one mole of electrons.

Planck’s constant (h), the velocity of light (c), Rydberg’s constant (R) and Avogadro's constant (L) are four of the most accurately known constants in science. By substituting their values into this theoretically derived equation we can calculate the energy required to remove one electron from a mole of gaseous hydrogen atoms i.e. the ionization energy.

E = (6.6261 x 10⁻³⁴ Js) x (2.9980 x 10⁸ ms⁻¹) x (1.0974 x 10⁷ m⁻¹) x (6.0221 x 10²³ mol⁻¹)

= 1.313 x 10⁶ J mol⁻¹ = 1313 kJ mol⁻¹ which agrees completely with the experimentally determined value given in Section 8 of the IB data booklet (1312 kJ mol⁻¹).This provides strong evidence that atomic spectra are due to electron transitions between energy levels within the atom.

Although the above calculation shows that the emission spectrum of hydrogen does very much support the Bohr model of the atom, Higher Level students do not have to use the Rydberg equation in calculations. They can obtain the ionization energy of hydrogen directly from the fact that the convergence line in the ultraviolet appears at a wavelength of 9.12 x 10⁻⁸ m.

E = hv = 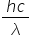= = 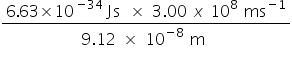2.18 x 10⁻¹⁸J (for one electron)

= 2.18 x 10⁻¹⁸ J x 6.02 x 10²³ mol⁻¹ = 1.31 x 10⁶ J mol⁻¹ = 1310 kJ mol⁻¹ (to 3 SF)

Some teachers have asked where they can find the relevant convergence limit for other elements. The NIST source given below is one place but in fact they are already there in Section 8 of the IB data booklet disguised as first ionization energies. For any element E = hcL/λ where E is the 1st ionization energy and λ is the wavelength of the relevant convergence limit. hcL is a constant which has the value 6.63 x 10⁻³⁴ x 3 x 10⁸ x 6.02 x 10²³ = 1.1974 x 10⁻¹ J m mol^-1 or 1.198 x 10⁻⁴ kJ m mol⁻¹. This means that for any element you can work out the convergence limit by simply dividing 1.198 x 10^-4 by the IE given in Section 8 of the data booklet to give the wavelength in metres. So for sodium it would be 1.198 x 10⁻⁴ kJ m mol⁻¹ divided by 496 kJ mol⁻¹ which gives 2.413 x 10⁻⁷ m or 241.3 nm which corresponds to the value given by NIST.

If you need a convergence limit to give to your students just do exactly the same sum for any element by dividing 1.198 x 10⁻⁴ by the first IE for that element. Then to fulfill the syllabus requirements you can get them to divide 1.198 x 10⁻⁴ kJ m mol^-1 by the value you have given them to get the IE. Surprise, surprise - it will be the same value as the one given in the data booklet! The whole thing seems a rather pointless exercise because the first ionization energy of any element is inversely proportional to the wavelength of its convergence limit so once you know one and you know the proportionality constant you can work out the other. It means that students are being asked to manipulate numbers using an equation without really having to explain exactly which electron is being promoted to the infinite level and in which region of the spectrum the relevant convergence limit is located. You can read more about this in Convergence limits which is a page in the section on Novel uses for the IB data booklet.