DP Mathematics: Applications and Interpretation Questionbank

Topic 5—Calculus
| Path: |
Description
[N/A]Directly related questions
-
21M.2.AHL.TZ1.6a:
Find the velocity vector at time t.
-
21M.2.AHL.TZ1.7a:
Find the population of rabbits 1 year after they were introduced.
-
21M.2.AHL.TZ1.6b:
Show that the magnitude of the velocity of the ice-skater at time t is given by
a ebt√(1+b2).
-
21M.2.AHL.TZ1.6d:
Find the magnitude of the velocity of the ice-skater when t=2.
-
21M.2.AHL.TZ1.6c:
Find the value of a and the value of b.
-
21M.2.AHL.TZ1.6e:
At a point P, the ice-skater is skating parallel to the y-axis for the first time.
Find OP.
-
21M.2.AHL.TZ1.7b:
(i) the population of rabbits 1 year after the foxes were introduced.
(ii) the population of foxes 1 year after the foxes were introduced.
-
21M.2.AHL.TZ1.7c.ii:
point B.
-
21M.2.AHL.TZ1.7c.i:
point A.
-
21M.2.AHL.TZ1.7d:
Find the non-zero equilibrium point for the populations of rabbits and foxes.
-
21M.2.SL.TZ2.5d:
Find an expression for dVdx.
-
21M.2.SL.TZ2.5e:
Find the value of x which maximizes the volume of the box.
-
21M.2.SL.TZ2.5f:
Hence, or otherwise, find the maximum possible volume of the box.
-
21M.2.AHL.TZ2.6c:
Find the greatest speed of P in the interval 0≤t≤6.
-
21M.2.AHL.TZ2.7c:
Determine, with justification, whether the equilibrium point (0, is stable or unstable.
-
21M.2.SL.TZ2.5g:
The box will contain spherical chocolates. The production manager assumes that they can calculate the exact number of chocolates in each box by dividing the volume of the box by the volume of a single chocolate and then rounding down to the nearest integer.
Explain why the production manager is incorrect.
-
21M.2.AHL.TZ2.6a:
Find the times when is at instantaneous rest.
-
21M.2.AHL.TZ2.6d:
The particle starts from the origin . Find an expression for the displacement of from at time seconds.
-
21M.2.AHL.TZ2.7b:
Hence, write down the general solution of the system.
-
21M.2.AHL.TZ2.6b:
Find the magnitude of the particle’s acceleration at seconds.
-
21M.2.AHL.TZ2.6e:
Find the total distance travelled by in the interval .
-
21M.2.AHL.TZ2.7a:
Find the eigenvalues and corresponding eigenvectors of the matrix .
-
21M.2.AHL.TZ2.7d:
(i) at .
(ii) at .
-
21M.2.AHL.TZ2.7e:
Sketch a phase portrait for the general solution to the system of coupled differential equations for , .
-
21M.3.AHL.TZ2.2f.i:
Use Euler’s method to sketch the trajectory of the phase portrait, for and , over the first nine years.
-
21M.3.AHL.TZ2.2a.i:
-
21M.3.AHL.TZ2.2c.i:
Toxic sewage is added to the Mediterranean Sea. Alessia claims this reduces the shark population growth rate and hence the value of is halved. No other parameter changes.
-
21M.3.AHL.TZ2.2d.iii:
Alessia estimates that the mackerel population density increases by a factor of three every two years. Show that to three significant figures.
-
21M.3.AHL.TZ2.2f.ii:
Using your phase portrait, or otherwise, determine whether the mackerel population density would be sufficient to support sustainable fishing during the first nine years.
-
21M.3.AHL.TZ2.2a.ii:
-
21M.3.AHL.TZ2.2b.i:
show that, at the equilibrium point, the value of the mackerel population density is ;
-
21M.3.AHL.TZ2.2d.ii:
Show that the expression for the mackerel population density after years is
-
21M.3.AHL.TZ2.2e.i:
Write down expressions for and in terms of and .
-
21M.3.AHL.TZ2.2f.iii:
State two reasons why Alessia’s conclusion, found in part (f)(ii), might not be valid.
-
20N.1.SL.TZ0.S_10a.i:
Find in terms of and .
-
20N.1.SL.TZ0.S_10a.ii:
Show that the equation of is .
-
20N.1.SL.TZ0.S_10b:
Find the area of triangle in terms of .
-
20N.1.SL.TZ0.S_10c:
The graph of is translated by to give the graph of .
In the following diagram:- point lies on the graph of
- points , and lie on the vertical asymptote of
- points and lie on the horizontal asymptote of
- point lies on the -axis such that is parallel to .
Line is the tangent to the graph of at , and passes through and .
Given that triangle and rectangle have equal areas, find the gradient of in terms of .
-
20N.1.SL.TZ0.S_3a:
Find the value of .
-
20N.1.SL.TZ0.S_3b:
Find the volume of the solid formed when the shaded region is revolved about the -axis.
-
20N.1.SL.TZ0.S_6:
The graph of a function passes through the point .
Given that , find .
-
20N.1.SL.TZ0.S_7a:
Find an expression for the velocity of at time .
-
20N.1.SL.TZ0.S_7b:
Particle also moves in a straight line. The position of is given by .
The speed of is greater than the speed of when .
Find the value of .
-
20N.2.SL.TZ0.S_10a:
Show that .
-
20N.2.SL.TZ0.S_10b:
Find the least value of .
-
20N.2.SL.TZ0.S_10c:
Find .
-
20N.2.SL.TZ0.S_10d:
Let be the region enclosed by the graph of , the -axis and the lines and . The area of is , correct to three significant figures.
Find .
-
20N.1.SL.TZ0.T_13a:
Write down .
-
20N.1.SL.TZ0.T_13b:
Write down the gradient of this tangent.
-
20N.1.SL.TZ0.T_13c:
Find the value of .
-
20N.2.SL.TZ0.T_4a:
Write down an equation in and that shows this information.
-
20N.2.SL.TZ0.T_4b:
Show that .
-
20N.2.SL.TZ0.T_4c:
Find .
-
20N.2.SL.TZ0.T_4d:
Using your answer to part (c), find the value of for which is a maximum.
-
20N.2.SL.TZ0.T_4e:
Find the maximum volume of the bird bath.
-
20N.2.SL.TZ0.T_4f:
To prevent leaks, a sealant is applied to the interior surface of the bird bath.
Find the surface area to be covered by the sealant, given that the bird bath has maximum volume.
-
21M.3.AHL.TZ2.2d.i:
Write down the differential equation for that models this situation.
-
21M.3.AHL.TZ2.2b.ii:
find the value of the shark population density at the equilibrium point.
-
21M.3.AHL.TZ2.2c.ii:
Global warming increases the temperature of the Mediterranean Sea. Alessia claims that this promotes the mackerel population growth rate and hence the value of is doubled. No other parameter changes.
-
21M.3.AHL.TZ2.2e.ii:
Use Euler’s method to find an estimate for the mackerel population density after one year.
-
16N.2.SL.TZ0.T_6g:
Find the value of this minimum area.
-
16N.2.SL.TZ0.T_6c:
Write down, in terms of and , an equation for the volume of this water container.
-
16N.2.SL.TZ0.T_6h:
Find the least number of cans of water-resistant material that will coat the area in part (g).
-
16N.2.SL.TZ0.T_6a:
Write down a formula for , the surface area to be coated.
-
16N.2.SL.TZ0.T_6d:
Show that .
-
16N.2.SL.TZ0.T_6b:
Express this volume in .
-
16N.2.SL.TZ0.T_6e:
Find .
-
16N.2.SL.TZ0.T_6f:
Using your answer to part (e), find the value of which minimizes .
-
EXN.1.SL.TZ0.7a:
Find an expression for .
-
EXN.1.SL.TZ0.7b:
Show that the normal to the curve at the point where is .
-
EXN.1.AHL.TZ0.10a.i:
Find .
-
EXN.1.AHL.TZ0.10a.ii:
State in context what this value represents.
-
EXN.1.AHL.TZ0.13a:
Write the differential equation as a system of coupled first order differential equations.
-
EXN.1.AHL.TZ0.15b:
Find in terms of the value of at which the maximum occurs.
-
EXN.1.AHL.TZ0.10c:
Determine and state what it represents.
-
EXN.1.AHL.TZ0.10b:
Find an expression for in terms of .
-
EXN.1.AHL.TZ0.13b:
When ,
Use Euler’s method with a step length of to find an estimate for the value of the displacement and velocity of the particle when .
-
EXN.1.AHL.TZ0.15c:
Hence find the value of for which has the smallest possible maximum value.
-
EXN.1.AHL.TZ0.15a:
Find .
-
EXN.2.SL.TZ0.2c:
Find
-
EXN.2.SL.TZ0.3b.i:
Find the value of at which the ball hits the ground.
-
EXN.2.SL.TZ0.2b:
Show that
-
EXN.2.SL.TZ0.3a:
Find an expression for the height of the ball at time .
-
EXN.2.SL.TZ0.3c:
Find the range of .
-
EXN.2.SL.TZ0.6b:
By dividing the area between the curve and the ‐axis into two trapezoids of unequal width show that , justifying the direction of the inequality.
-
EXN.2.SL.TZ0.6d:
Use differentiation to show that .
-
EXN.2.SL.TZ0.6g:
Use the expression found in (f) to calculate a value for .
-
EXN.2.AHL.TZ0.4b:
Show that
-
EXN.2.AHL.TZ0.4c:
Hence find three approximations for the value of .
-
EXN.2.AHL.TZ0.5a.ii:
the eigenvectors.
-
EXN.2.SL.TZ0.2a:
Find an expression for the total length of the ribbon in terms of and .
-
EXN.2.SL.TZ0.2e:
Hence or otherwise find the minimum length of ribbon required.
-
EXN.2.AHL.TZ0.4a:
Show that Jorge’s model satisfies the differential equation
-
EXN.2.SL.TZ0.2d:
Solve
-
EXN.2.SL.TZ0.3b.ii:
Hence write down the domain of .
-
EXN.2.AHL.TZ0.5e.ii:
Find the population of at this value of . Give your answer to the nearest herbivores.
-
EXN.2.AHL.TZ0.5c:
Sketch the phase portrait for this system, for .
On your sketch show
- the equation of the line defined by the eigenvector in the first quadrant
- at least two trajectories either side of this line using arrows on those trajectories to represent the change in populations as t increases
-
EXN.2.AHL.TZ0.5e.i:
Find the value of at which .
-
EXN.2.AHL.TZ0.5a.i:
the eigenvalues.
-
EXN.2.AHL.TZ0.5d:
Write down a condition on the size of the initial population of if it is to avoid its population reducing to zero.
-
EXN.2.AHL.TZ0.5b:
Hence write down the general solution of the system of equations.
-
EXN.2.AHL.TZ0.7c.i:
Find an expression for the acceleration of the ball at time .
-
EXN.2.AHL.TZ0.7a:
Show that the ball is moving in a circle with its centre at and state the radius of the circle.
-
EXN.2.AHL.TZ0.7b.ii:
Hence show that the velocity of the ball is always perpendicular to the position vector of the ball.
-
EXN.2.AHL.TZ0.7b.i:
Find an expression for the velocity of the ball at time .
-
EXN.2.AHL.TZ0.7c.ii:
Find the value of at the instant the string breaks.
-
EXN.2.AHL.TZ0.7c.iii:
How many complete revolutions has the ball completed from to the instant at which the string breaks?
-
21M.1.SL.TZ1.12a.i:
Find in terms of and .
-
21M.1.SL.TZ1.13a:
Determine whether the graph of against is increasing or decreasing at .
-
21M.1.SL.TZ1.12a.ii:
Show that .
-
21M.1.SL.TZ1.13b:
Sieun observes that when the angle is , the ball will travel a horizontal distance of .
Find an expression for the function .
-
21M.1.SL.TZ1.12b:
Find .
-
21M.1.SL.TZ1.12c.ii:
Hence, or otherwise, find this value of .
-
21M.1.SL.TZ1.12c.i:
Write down an equation Ellis could solve to find this value of .
-
21M.1.AHL.TZ1.15a:
Find the equation of , giving your answer in the form .
-
21M.1.AHL.TZ1.15b:
For the two solutions given, the local maximum points lie on the straight line .
Find the equation of .
-
21M.1.AHL.TZ1.12a:
Show that .
-
21M.1.AHL.TZ1.12b:
Find the time taken for the tank to empty.
-
21M.1.SL.TZ2.13b:
The company regularly increases the number of cars it produces.
Describe how their profit changes if they increase production to over cars per month and up to cars per month. Justify your answer.
-
21M.1.SL.TZ2.13a:
Find an expression for in terms of .
-
21M.2.SL.TZ1.5a.i:
Find .
-
21M.2.SL.TZ1.5b:
Use the trapezoidal rule, with three intervals, to estimate the cross-sectional area of the tunnel.
-
21M.2.SL.TZ1.5c.ii:
Hence find the cross-sectional area of the tunnel.
-
21M.2.SL.TZ1.5a.ii:
Hence find the maximum height of the tunnel.
-
21M.2.SL.TZ1.5c.i:
Write down the integral which can be used to find the cross-sectional area of the tunnel.
-
21M.2.AHL.TZ1.2a.i:
Find .
-
21M.2.AHL.TZ1.4d.ii:
Find the value of Charlotte’s estimate.
-
21M.2.AHL.TZ1.2a.ii:
Hence find the maximum height of the tunnel.
-
21M.2.AHL.TZ1.2c:
Use the trapezoidal rule, with three intervals, to estimate the cross-sectional area of the tunnel.
-
21M.2.AHL.TZ1.2d.i:
Write down the integral which can be used to find the cross-sectional area of the tunnel.
-
21M.2.AHL.TZ1.2d.ii:
Hence find the cross-sectional area of the tunnel.
-
21M.2.AHL.TZ1.4d.i:
Write down an expression for her estimate of the volume as a sum of two integrals.
-
21N.1.SL.TZ0.6a:
Use the trapezoidal rule with an interval width of to estimate the total amount of carbon dioxide emitted during these two hours.
-
21N.1.SL.TZ0.6b:
The real amount of carbon dioxide emitted during these two hours was tonnes.
Find the percentage error of the estimate found in part (a).
-
21N.1.SL.TZ0.12a:
Find .
-
21N.1.SL.TZ0.12b.i:
Solve .
-
21N.1.SL.TZ0.12b.ii:
Interpret your answer to (b)(i) in context.
-
21N.1.SL.TZ0.13a.i:
Write down the value of .
-
21N.1.SL.TZ0.13a.ii:
Hence form two equations in terms of and .
-
21N.1.SL.TZ0.13a.iii:
Hence find the equation of the quadratic curve.
-
21N.1.SL.TZ0.13b:
Find the area of the shaded region in Irina’s design.
-
21N.1.AHL.TZ0.8a.i:
Identify the value of the point where has its maximum value.
-
21N.1.AHL.TZ0.8a.ii:
Interpret this point in the given context.
-
21N.1.AHL.TZ0.13a:
Calculate the value of at the point .
-
21N.1.AHL.TZ0.13b:
Sketch, on the first graph, a curve that represents the points where .
-
21N.1.AHL.TZ0.15b.ii:
Find the expression .
-
21N.1.AHL.TZ0.13c:
(i) sketch the solution curve that passes through the point .
(ii) sketch the solution curve that passes through the point .
-
21N.1.AHL.TZ0.17b:
Hence find the maximum capacity of the bowl in .
-
21N.1.AHL.TZ0.17a:
Show that the volume of water, , in terms of is .
-
21N.1.AHL.TZ0.15b.iii:
Solve algebraically to find the value of that will maximize the volume, .
-
21N.1.AHL.TZ0.8b:
Juri starts at a height of metres and finishes at , where .
Sketch a possible diagram of the hill on the following pair of coordinate axes.
-
21N.1.AHL.TZ0.15a:
Show that .
-
21N.1.AHL.TZ0.15b.i:
Find an expression for in terms of .
-
21N.2.AHL.TZ0.6a:
Given that , show that .
-
21N.2.AHL.TZ0.6c.i:
Find the eigenvalues of matrix .
-
21N.2.AHL.TZ0.6b:
Write down the matrix .
-
21N.2.AHL.TZ0.6c.ii:
Find the eigenvectors of matrix .
-
21N.2.AHL.TZ0.6d:
Given that when the shock absorber is displaced and its velocity is zero, find an expression for in terms of .
-
21N.3.AHL.TZ0.2a.i:
Find the equation of the regression line of on .
-
21N.3.AHL.TZ0.2a.iii:
Suggest why Eva’s use of the linear regression equation in this way could be unreliable.
-
21N.3.AHL.TZ0.2b.i:
Find the equation of the least squares quadratic regression curve.
-
21N.3.AHL.TZ0.2b.ii:
Find the value of .
-
21N.3.AHL.TZ0.2b.iii:
Hence, write down a suitable domain for Eva’s function .
-
21N.3.AHL.TZ0.2a.ii:
Interpret the meaning of parameter in the context of the model.
-
21N.3.AHL.TZ0.2d:
By solving the differential equation , show that the general solution is given by , where .
-
21N.3.AHL.TZ0.2g.i:
Show that , where .
-
21N.3.AHL.TZ0.2g.ii:
Use Euler’s method with a step length of minutes to estimate the maximum value of .
-
21N.3.AHL.TZ0.2e:
Use the general solution from part (d) and the initial condition to predict the value of .
-
21N.3.AHL.TZ0.2f:
Find this new height.
-
21N.3.AHL.TZ0.2c:
Show that .
-
22M.1.SL.TZ1.6c:
Find the area of the unshaded region in the painting.
-
22M.1.SL.TZ1.9a:
Find .
-
22M.1.SL.TZ1.12a:
Determine whether the profit is increasing or decreasing when .
-
22M.1.SL.TZ1.12b:
One year after the company was founded, the profit was thousand dollars.
Find an expression for , when .
-
22M.1.SL.TZ1.9b:
Find the equation of the normal to the curve at in the form , where .
-
22M.1.SL.TZ1.6a:
Use the trapezoidal rule, with the values given in the following table, to approximate the area of the shaded region.
-
22M.1.SL.TZ1.6b:
Find the exact area of the shaded region in the painting.
-
22M.1.AHL.TZ1.7a.i:
Write down the equation of the curve on which all these maximum and minimum points lie.
-
22M.1.AHL.TZ1.7b:
The solution to the differential equation that passes through the point has both a local maximum point and a local minimum point.
On the slope field, sketch the solution to the differential equation that passes through .
-
22M.1.AHL.TZ1.8a.ii:
Find .
-
22M.1.AHL.TZ1.8b:
The curve has a point of inflexion at .
Find the value of .
-
22M.1.AHL.TZ1.7a.ii:
Sketch this curve on the slope field.
-
22M.1.AHL.TZ1.8a.i:
Find .
-
22M.1.AHL.TZ1.14a.i:
Expand .
-
22M.1.AHL.TZ1.14a.ii:
Find .
-
22M.1.AHL.TZ1.14b:
Find the volume of the solid formed. Give your answer in the form , where .
-
22M.1.AHL.TZ1.16a:
Find an expression for .
-
22M.1.AHL.TZ1.16b:
When Frieda arrives at the top of a hill, the speed of the wind is kilometres per hour and increasing at a rate of .
Find the rate of change of at this time.
-
22M.1.AHL.TZ1.17:
A function is of the form . Part of the graph of is shown.
The points and have coordinates and , and lie on .
The point is a local maximum and the point is a local minimum.
Find the value of , of and of .
-
22M.1.SL.TZ2.6a:
Calculate the area of the shaded region in diagram 1.
-
22M.1.SL.TZ2.6b.ii:
Calculate the area of this region.
-
22M.1.SL.TZ2.6b.i:
Write down an integral for the area of the shaded region in diagram 2.
-
22M.1.SL.TZ2.6c:
Hence, determine the area enclosed between and .
-
22M.1.SL.TZ2.11a:
Find .
-
22M.1.SL.TZ2.11b:
Use your answer to part (a) to find the gradient of .
-
22M.1.AHL.TZ2.14:
The shape of a vase is formed by rotating a curve about the -axis.
The vase is high. The internal radius of the vase is measured at intervals along the height:
Use the trapezoidal rule to estimate the volume of water that the vase can hold.
-
22M.1.AHL.TZ2.17b.ii:
Hence show that the snail reaches the point before the water does.
-
22M.1.AHL.TZ2.16a:
Find the velocity vector of .
-
22M.1.AHL.TZ2.17a:
When has an -coordinate equal to , find the horizontal component of the velocity of .
-
22M.1.AHL.TZ2.17b.i:
Find the time taken for the snail to reach the point .
-
22M.1.AHL.TZ2.16b:
Show that the acceleration vector of is never parallel to the position vector of .
-
EXM.3.AHL.TZ0.1c:
In the case when there is a pair of purely imaginary eigenvalues you are told that the solution will form an ellipse. You are also told that the initial conditions are such that the ellipse is large enough that it will cross both the positive and negative axes and the positive and negative axes.
By considering the differential equations at these four crossing point investigate if the trajectory is in a clockwise or anticlockwise direction round the ellipse. Give your decision in terms of and . Using part (b) (ii) show that your conclusions are consistent.
-
EXM.3.AHL.TZ0.1a:
Show that if the system has two distinct real eigenvalues then .
-
EXM.3.AHL.TZ0.1b.ii:
Explain why and must have opposite signs.
-
EXM.2.AHL.TZ0.1a:
Use the eigenvalue method to find the general solution to this system of equations.
-
EXM.3.AHL.TZ0.1b.i:
Find two conditions that must be satisfied by , , , .
-
22M.2.SL.TZ1.4c:
Find the value of at which the goat is eating grass at the greatest rate.
-
22M.2.AHL.TZ1.2c:
Find the value of at which the goat is eating grass at the greatest rate.
-
22M.2.AHL.TZ1.2d:
The goat is tied in the field for hours.
Find the total volume of grass eaten by the goat during this time.
-
22M.2.AHL.TZ1.4a.ii:
Find the eigenvalues for the matrix .
-
22M.2.AHL.TZ1.4a.iii:
Hence state the long-term velocity of the particle.
-
22M.2.AHL.TZ1.4b.i:
Use the substitution to write the differential equation as a system of coupled, first order differential equations.
-
22M.2.AHL.TZ1.4b.ii:
Use Euler’s method with a step length of to find the displacement of the particle when .
-
22M.2.AHL.TZ1.4b.iii:
Find the long-term velocity of the particle.
-
22M.2.AHL.TZ1.4a.i:
Use the substitution to show that this equation can be written as
.
-
22M.2.AHL.TZ1.6a.i:
Find .
-
22M.2.AHL.TZ1.6a.ii:
Hence show that the equation of the tangent to the curve at the point is .
-
EXM.2.AHL.TZ0.1c:
As , find an asymptote to the trajectory of the particular solution found in (b)(i) and state if this trajectory will be moving towards or away from the origin.
-
SPM.1.SL.TZ0.10c:
The three points A(0, 0) , B(3, 10) and C(, 0) define the vertices of a triangle.
Find the value of , the -coordinate of C, such that the area of the triangle is equal to the area of region R.
-
SPM.1.SL.TZ0.10a:
Write down an integral for the area of region R.
-
22M.2.AHL.TZ1.6d.i:
Find the area enclosed by , the -axis and the line .
-
22M.2.AHL.TZ1.6d.ii:
Find the area of the shaded region on the diagram.
-
EXM.2.AHL.TZ0.1b.ii:
Hence find the solution when .
-
EXM.2.AHL.TZ0.1b.i:
Given the initial conditions that when , , , find the particular solution.
-
SPM.1.SL.TZ0.10b:
Find the area of region R.
-
SPM.1.AHL.TZ0.7a:
Write down an expression for as a function of time.
-
SPM.1.AHL.TZ0.7b:
Hence find .
-
SPM.1.AHL.TZ0.7c:
Hence or otherwise find the first time at which the kinetic energy is changing at a rate of 5 J s−1.
-
SPM.1.AHL.TZ0.13a:
Find the value of when .
-
SPM.1.AHL.TZ0.13b:
On the following axes, sketch a possible trajectory for the growth of the two fungi, making clear any asymptotic behaviour.
-
SPM.1.AHL.TZ0.14a.i:
Write down the value of .
-
SPM.1.AHL.TZ0.14a.ii:
Find the value of .
-
SPM.1.AHL.TZ0.14b:
The outer dome of a large cathedral has the shape of a hemisphere of diameter 32 m, supported by vertical walls of height 17 m. It is also supported by an inner dome which can be modelled by rotating the curve through 360° about the -axis between = 0 and = 33, as indicated in the diagram.
Find the volume of the space between the two domes.
-
SPM.2.SL.TZ0.4a:
Calculate the surface area of the box in cm2.
-
SPM.2.SL.TZ0.4c:
Find the number of boxes that should be sold each week to maximize the profit.
-
SPM.2.SL.TZ0.4e:
Find the least number of boxes which must be sold each week in order to make a profit.
-
SPM.2.SL.TZ0.4d:
Find .
-
SPM.2.SL.TZ0.4b:
Calculate the length AG.
-
22M.3.AHL.TZ1.1e:
Determine the value of and of . Give your answers correct to one decimal place.
-
22M.2.SL.TZ2.5a:
Find an expression for in terms of and .
-
22M.2.AHL.TZ2.4a:
Show that is always positive.
-
22M.2.SL.TZ2.5b:
Hence find the maximum value of in terms of . Give your answer in the form , where is a constant.
-
EXM.2.AHL.TZ0.12b.i:
Use all the coordinates in the table to find the equation of the least squares cubic regression curve.
-
EXM.2.AHL.TZ0.12c.ii:
Find the value of this area.
-
EXM.2.AHL.TZ0.12a:
Use the trapezoidal rule to find an estimate for the area.
-
EXM.2.AHL.TZ0.12b.ii:
Write down the coefficient of determination.
-
EXM.2.AHL.TZ0.12c.i:
Write down an expression for the area enclosed by the cubic regression curve, the -axis, the -axis and the line .
-
22M.2.SL.TZ2.5c.ii:
Use the model to find how much coffee the cafe should make each morning to maximize its profit.
-
EXM.3.AHL.TZ0.2b:
Hence, verify that is a solution to the above system.
-
EXM.3.AHL.TZ0.2f.i:
Find the equation of this asymptote.
-
EXM.3.AHL.TZ0.3d:
Show how using the substitution transforms the system of differential equations into .
-
22M.2.AHL.TZ2.4b:
Given that and , sketch the graph of against .
-
EXM.3.AHL.TZ0.3i:
With the initial conditions as given in part (b) state if the equilibrium point is stable or unstable.
-
EXM.3.AHL.TZ0.3h:
Use part (f) to find limit of the ratio as .
-
EXM.3.AHL.TZ0.2f.ii:
State the direction of the trajectory, including the quadrant it is in as it approaches this asymptote.
-
EXM.3.AHL.TZ0.3a:
Find the equilibrium point for this system.
-
EXM.3.AHL.TZ0.3b:
If initially and use Euler’s method with an time increment of 0.1 to find an approximation for the values of and when .
-
EXM.3.AHL.TZ0.2d:
If initially at find the particular solution.
-
EXM.3.AHL.TZ0.2e:
Find the values of and when .
-
EXM.3.AHL.TZ0.3j:
If instead the initial conditions were given as and , find the particular solution for of the original system, in this case.
-
EXM.3.AHL.TZ0.3c:
Extend this method to conjecture the limit of the ratio as .
-
EXM.3.AHL.TZ0.2a:
Show that the matrix has (sadly) only one eigenvalue. Find this eigenvalue and an associated eigenvector.
-
EXM.3.AHL.TZ0.3f:
Find the particular solution to the original system, given the initial conditions of part (b).
-
EXM.3.AHL.TZ0.3e:
Solve this system of equations by the eigenvalue method and hence find the general solution for of the original system.
-
EXM.3.AHL.TZ0.3g:
Hence find the exact values of and when , giving the answers to 4 significant figures.
-
EXM.3.AHL.TZ0.3k:
With the initial conditions as given in part (j), determine if the equilibrium point is stable or unstable.
-
EXM.3.AHL.TZ0.2c:
Verify that is also a solution.
-
EXM.3.AHL.TZ0.7a.i:
Use the trapezoidal rule to find an estimate for the area.
-
EXM.3.AHL.TZ0.7c.ii:
Find the value of this area.
-
EXM.3.AHL.TZ0.7b.ii:
Write down the coefficient of determination.
-
EXM.3.AHL.TZ0.7a.ii:
With reference to the shape of the graph, explain whether your answer to part (a)(i) will be an over-estimate or an underestimate of the area.
-
EXM.3.AHL.TZ0.7d.i:
Show that .
-
EXM.3.AHL.TZ0.7d.iv:
Hence find the area enclosed by the exponential function, the -axis, the -axis and the line .
-
EXM.3.AHL.TZ0.7b.i:
Use all the coordinates in the table to find the equation of the least squares cubic regression curve.
-
EXM.3.AHL.TZ0.7d.iii:
By finding the equation of a suitable regression line, show that and .
-
EXM.3.AHL.TZ0.7c.i:
Write down an expression for the area enclosed by the cubic function, the -axis, the -axis and the line .
-
EXM.3.AHL.TZ0.7d.ii:
Hence explain how a straight line graph could be drawn using the coordinates in the table.
-
22M.2.AHL.TZ2.6a.i:
Find the initial speed of the ball.
-
22M.2.AHL.TZ2.7c:
Hence find the exact solution of the second order differential equation.
-
22M.2.AHL.TZ2.6a.ii:
Find the angle of elevation of the ball as it is launched.
-
22M.2.AHL.TZ2.7a:
Show that the system of coupled first order equations:
can be written as the given second order differential equation.
-
22M.2.AHL.TZ2.6b:
Find the maximum height reached by the ball.
-
22M.3.AHL.TZ1.1b.i:
Find the general solution of the differential equation .
-
18M.1.SL.TZ2.T_7c:
Two girls are selected at random.
Calculate the probability that one girl answered questions in Mandarin and the other answered questions in Hindi.
-
18M.2.SL.TZ2.T_1a.i:
Write down the value of a.
-
18M.2.SL.TZ2.T_1a.ii:
Write down the value of b.
-
18M.2.SL.TZ2.T_1b.i:
Use the tree diagram to find the probability that an employee encountered traffic and was late for work.
-
19M.2.SL.TZ2.T_1a:
Write down the null hypothesis, H0 , for this test.
-
19M.2.SL.TZ2.T_1b:
State the number of degrees of freedom.
-
19M.2.SL.TZ2.T_1c.i:
the expected frequency of female students who chose to take the Chinese class.
-
19M.2.SL.TZ2.T_1d:
State whether or not H0 should be rejected. Justify your statement.
-
19M.2.SL.TZ2.T_1e.i:
Find the probability that the student does not take the Spanish class.
-
19M.2.SL.TZ2.T_1e.ii:
Find the probability that neither of the two students take the Spanish class.
-
19M.2.SL.TZ2.T_1e.iii:
Find the probability that at least one of the two students is female.
-
17N.1.SL.TZ0.T_11c:
Find the value of and of .
-
17N.1.SL.TZ0.T_2c.ii:
Write down, in the form , the equation of .
-
17N.1.SL.TZ0.T_15a:
Write down how many kilograms of cheese Maria sells in one week if the price of a kilogram of cheese is 8 EUR.
-
17N.1.SL.TZ0.T_15b:
Find how much Maria earns in one week, from selling cheese, if the price of a kilogram of cheese is 8 EUR.
-
17N.1.SL.TZ0.T_15c:
Write down an expression for in terms of .
-
17N.1.SL.TZ0.T_15d:
Find the price, , that will give Maria the highest weekly profit.
-
17N.2.SL.TZ0.T_5a:
Find the exact value of each of the zeros of .
-
17N.2.SL.TZ0.T_5b.i:
Expand the expression for .
-
17N.2.SL.TZ0.T_5b.ii:
Find .
-
17N.2.SL.TZ0.T_5c:
Use your answer to part (b)(ii) to find the values of for which is increasing.
-
17N.2.SL.TZ0.T_5d:
Draw the graph of for and . Use a scale of 2 cm to represent 1 unit on the -axis and 1 cm to represent 5 units on the -axis.
-
17N.2.SL.TZ0.T_5e:
Write down the coordinates of the point of intersection.
-
19M.2.SL.TZ2.T_5d:
Find .
-
19M.2.SL.TZ2.T_5e:
Find the gradient of the graph of at .
-
19M.2.SL.TZ2.T_5f:
Find the equation of the tangent line to the graph of at . Give the equation in the form where, , , and .
-
18N.1.SL.TZ0.T_11a:
Find .
-
18N.1.SL.TZ0.T_11b:
Find the gradient of this tangent at point P.
-
18N.1.SL.TZ0.T_11c:
Find the equation of this tangent. Give your answer in the form y = mx + c.
-
18N.2.SL.TZ0.T_4a:
Sketch the graph of y = f (x), for −4 ≤ x ≤ 3 and −50 ≤ y ≤ 100.
-
18N.2.SL.TZ0.T_4b.i:
Use your graphic display calculator to find the zero of f (x).
-
18N.2.SL.TZ0.T_4b.ii:
Use your graphic display calculator to find the coordinates of the local minimum point.
-
18N.2.SL.TZ0.T_4b.iii:
Use your graphic display calculator to find the equation of the tangent to the graph of y = f (x) at the point (–2, 38.75).
Give your answer in the form y = mx + c.
-
16N.2.SL.TZ0.S_10a:
(i) Find the value of .
(ii) Show that .
(iii) Find the value of .
-
16N.2.SL.TZ0.S_10b:
(i) Write down the value of .
(ii) Find .
-
16N.2.SL.TZ0.S_10c:
(i) Find .
(ii) Hence or otherwise, find the maximum positive rate of change of .
-
19M.1.SL.TZ2.T_15a:
Find the value of if no vases are sold.
-
19M.1.SL.TZ2.T_15b:
Differentiate .
-
19M.1.SL.TZ1.T_12a:
Find the probability that both spins are yellow.
-
19M.1.SL.TZ1.T_12b:
Find the probability that at least one of the spins is yellow.
-
19M.1.SL.TZ1.T_12c:
Write down the probability that the second spin is yellow, given that the first spin is blue.
-
18M.1.SL.TZ2.T_13a:
Find the cost of producing 70 shirts.
-
18M.1.SL.TZ2.T_13b:
Find the value of s.
-
18M.1.SL.TZ2.T_13c:
Find the number of shirts produced when the cost of production is lowest.
-
18M.1.SL.TZ2.T_14a:
Find f'(x)
-
18M.1.SL.TZ2.T_14b:
Find the gradient of the graph of f at .
-
18M.1.SL.TZ2.T_14c:
Find the x-coordinate of the point at which the normal to the graph of f has gradient .
-
18M.2.SL.TZ1.T_4a:
Find the value of k.
-
18M.2.SL.TZ1.T_4b:
Using your value of k , find f ′(x).
-
18M.2.SL.TZ1.T_4c:
Use your answer to part (b) to show that the minimum value of f(x) is −22 .
-
19M.2.SL.TZ1.T_6b:
Find the coordinates of the local minimum.
-
19M.2.SL.TZ1.T_6c:
Write down the interval where the gradient of the graph of is negative.
-
19M.2.SL.TZ1.T_6d:
Determine the equation of the normal at in the form .
-
19M.1.SL.TZ1.T_15a:
Write down an equation for the area, , of the curved surface in terms of .
-
19M.1.SL.TZ1.T_15b:
Find .
-
19M.1.SL.TZ1.T_15c:
Find the value of when the area of the curved surface is maximized.
-
17N.1.SL.TZ0.T_14a:
Write down the derivative of .
-
17N.1.SL.TZ0.T_14b:
Find the point on the graph of at which the gradient of the tangent is equal to 6.
-
18M.2.SL.TZ2.T_6d:
Find .
-
18M.2.SL.TZ2.T_6f:
Given that y = 2x3 − 9x2 + 12x + 2 = k has three solutions, find the possible values of k.
-
19M.2.SL.TZ1.T_6a:
Show that .
-
16N.1.SL.TZ0.T_14a:
Find .
-
16N.1.SL.TZ0.T_14b:
Find the coordinates of P.
-
18N.1.SL.TZ0.S_10a:
Find the coordinates of P.
-
18N.1.SL.TZ0.S_10b.i:
Find .
-
18N.1.SL.TZ0.S_10b.ii:
Hence, find the equation of L in terms of .
-
18N.1.SL.TZ0.S_10c:
The graph of has a local minimum at the point Q. The line L passes through Q.
Find the value of .
-
17N.2.SL.TZ0.T_4a:
Find the probability that this person is not allergic to nuts.
-
17N.2.SL.TZ0.T_4b:
Find the probability that both people chosen are not allergic to nuts.
-
17N.2.SL.TZ0.T_4c:
Copy and complete the tree diagram.
-
17N.2.SL.TZ0.T_4d:
Find the probability that this adult is allergic to nuts and the liquid turns blue.
-
17N.2.SL.TZ0.T_4e:
Find the probability that the liquid turns blue.
-
17N.2.SL.TZ0.T_4f:
Find the probability that the tested adult is allergic to nuts given that the liquid turned blue.
-
17N.2.SL.TZ0.T_4g:
Estimate the number of employees, from this 38, who are allergic to nuts.
-
19M.2.AHL.TZ1.H_10a:
Write down the maximum and minimum value of .
-
19M.2.AHL.TZ1.H_10b:
Write down two transformations that will transform the graph of onto the graph of .
-
19M.2.AHL.TZ1.H_10c:
Sketch the graph of for 0 ≤ ≤ 0.02 , showing clearly the coordinates of the first maximum and the first minimum.
-
19M.2.AHL.TZ1.H_10d:
Find the total time in the interval 0 ≤ ≤ 0.02 for which ≥ 3.
-
19M.2.AHL.TZ1.H_10e:
Find (0.007).
-
19M.2.AHL.TZ1.H_10f:
With reference to your graph of explain why > 0 for all > 0.
-
19M.2.AHL.TZ1.H_10g:
Given that can be written as where , , , > 0, use your graph to find the values of , , and .
-
18M.1.AHL.TZ1.H_9a:
The graph of has a local maximum at A. Find the coordinates of A.
-
18M.1.AHL.TZ1.H_9b.i:
Show that there is exactly one point of inflexion, B, on the graph of .
-
18M.1.AHL.TZ1.H_9b.ii:
The coordinates of B can be expressed in the form B where a, b. Find the value of a and the value of b.
-
18M.1.AHL.TZ1.H_9c:
Sketch the graph of showing clearly the position of the points A and B.
-
17M.2.AHL.TZ1.H_2a:
Find in terms of and .
-
17M.2.AHL.TZ1.H_8a:
Find an expression for the volume of water in the trough in terms of .
-
19M.1.AHL.TZ2.H_8a:
Show that the volume of the cone may be expressed by .
-
18M.1.AHL.TZ1.H_2a:
Find
-
18M.1.AHL.TZ1.H_2b:
Hence find the values of θ for which .
-
16N.2.SL.TZ0.S_6a:
Use the model to find the volume of the barrel.
-
16N.2.SL.TZ0.S_6b:
The empty barrel is being filled with water. The volume of water in the barrel after minutes is given by . How long will it take for the barrel to be half-full?
-
17M.1.AHL.TZ2.H_10a.i:
Find the area of the window in terms of P and .
-
17M.1.AHL.TZ2.H_10a.ii:
Find the width of the window in terms of P when the area is a maximum, justifying that this is a maximum.
-
17M.1.AHL.TZ2.H_10b:
Show that in this case the height of the rectangle is equal to the radius of the semicircle.
-
17M.2.SL.TZ2.T_6a:
Write down the -intercept of the graph.
-
17M.2.SL.TZ2.T_6b:
Find .
-
17M.2.SL.TZ2.T_6c.i:
Show that .
-
17M.2.SL.TZ2.T_6c.ii:
Find .
-
17M.2.SL.TZ2.T_6d.i:
Write down the -coordinates of these two points;
-
17M.2.SL.TZ2.T_6d.ii:
Write down the intervals where the gradient of the graph of is positive.
-
17M.2.SL.TZ2.T_6e:
Write down the range of .
-
17M.2.SL.TZ2.T_6f:
Write down the number of possible solutions to the equation .
-
17M.2.SL.TZ2.T_6g:
The equation , where , has four solutions. Find the possible values of .
-
18M.1.SL.TZ1.T_5a:
Write down the coordinates of C, the midpoint of line segment AB.
-
19M.1.AHL.TZ1.H_8a:
Write down the -coordinate of the point of inflexion on the graph of .
-
19M.1.AHL.TZ1.H_8b:
find the value of .
-
19M.1.AHL.TZ1.H_8c:
find the value of .
-
19M.1.AHL.TZ1.H_8d:
Sketch the curve , 0 ≤ ≤ 5 indicating clearly the coordinates of the maximum and minimum points and any intercepts with the coordinate axes.
-
17N.1.SL.TZ0.S_5a:
Find .
-
17N.1.SL.TZ0.S_5b:
Given that , find the value of .
-
17M.1.AHL.TZ1.H_11a.i:
Express in the form .
-
17M.1.AHL.TZ1.H_11a.ii:
Factorize .
-
17M.1.AHL.TZ1.H_11b:
Sketch the graph of , indicating on it the equations of the asymptotes, the coordinates of the -intercept and the local maximum.
-
17M.1.AHL.TZ1.H_11c:
Show that .
-
17M.1.AHL.TZ1.H_11d:
Hence find the value of if .
-
17M.1.AHL.TZ1.H_11e:
Sketch the graph of .
-
17M.1.AHL.TZ1.H_11f:
Determine the area of the region enclosed between the graph of , the -axis and the lines with equations and .
-
19M.2.SL.TZ2.S_2a:
Find the -intercept of the graph of .
-
19M.2.SL.TZ2.S_2b:
The region enclosed by the graph of , the -axis and the -axis is rotated 360º about the -axis. Find the volume of the solid formed.
-
18M.2.SL.TZ2.S_3a:
Find the x-intercept of the graph of .
-
18M.2.SL.TZ2.S_3b:
The region enclosed by the graph of , the y-axis and the x-axis is rotated 360° about the x-axis.
Find the volume of the solid formed.
-
17M.2.AHL.TZ1.H_4a:
Write down a definite integral to represent the area of .
-
17M.2.AHL.TZ1.H_4b:
Calculate the area of .
-
17M.2.AHL.TZ1.H_8b:
Calculate when .
-
17M.1.SL.TZ1.S_5a:
Find .
-
17M.1.SL.TZ1.S_5b:
Find , given that and .
-
19M.1.AHL.TZ1.H_5:
A camera at point C is 3 m from the edge of a straight section of road as shown in the following diagram. The camera detects a car travelling along the road at = 0. It then rotates, always pointing at the car, until the car passes O, the point on the edge of the road closest to the camera.
A car travels along the road at a speed of 24 ms−1. Let the position of the car be X and let OĈX = θ.
Find , the rate of rotation of the camera, in radians per second, at the instant the car passes the point O .
-
19M.2.AHL.TZ2.H_6:
A particle moves along a horizontal line such that at time seconds, ≥ 0, its acceleration is given by = 2 − 1. When = 6 , its displacement from a fixed origin O is 18.25 m. When = 15, its displacement from O is 922.75 m. Find an expression for in terms of .
-
18M.3.AHL.TZ0.Hca_3a:
Find the value of .
-
18M.3.AHL.TZ0.Hca_3b:
Illustrate graphically the inequality .
-
18M.3.AHL.TZ0.Hca_3c:
Hence write down a lower bound for .
-
18M.3.AHL.TZ0.Hca_3d:
Find an upper bound for .
-
18M.2.AHL.TZ2.H_7a:
Determine the first time t1 at which P has zero velocity.
-
18M.2.AHL.TZ2.H_7b.i:
Find an expression for the acceleration of P at time t.
-
18M.2.AHL.TZ2.H_7b.ii:
Find the value of the acceleration of P at time t1.
-
17N.2.AHL.TZ0.H_11a.i:
Determine an expression for in terms of .
-
17N.2.AHL.TZ0.H_11a.ii:
Sketch a graph of for .
-
17N.2.AHL.TZ0.H_11a.iii:
Find the -coordinate(s) of the point(s) of inflexion of the graph of , labelling these clearly on the graph of .
-
17N.2.AHL.TZ0.H_11b.i:
Express in terms of .
-
17N.2.AHL.TZ0.H_11b.ii:
Express in terms of .
-
17N.2.AHL.TZ0.H_11b.iii:
Hence show that can be expressed as .
-
17N.2.AHL.TZ0.H_11c:
Solve the equation , giving your answers in the form where .
-
17N.2.AHL.TZ0.H_10a.i:
Show that the -coordinate of the minimum point on the curve satisfies the equation .
-
17N.2.AHL.TZ0.H_10a.ii:
Determine the values of for which is a decreasing function.
-
17N.2.AHL.TZ0.H_10b:
Sketch the graph of showing clearly the minimum point and any asymptotic behaviour.
-
17N.2.AHL.TZ0.H_10c:
Find the coordinates of the point on the graph of where the normal to the graph is parallel to the line .
-
17N.2.AHL.TZ0.H_10d:
This region is now rotated through radians about the -axis. Find the volume of revolution.
-
18M.1.AHL.TZ2.H_4:
Consider the curve .
Find the x-coordinates of the points on the curve where the gradient is zero.
-
17M.1.AHL.TZ2.H_4a:
Find and .
-
17M.1.AHL.TZ2.H_4b:
Find the displacement of the particle when
-
18M.1.AHL.TZ2.H_11a:
Show that where .
-
18M.1.AHL.TZ2.H_11b:
Express in terms of . Give your answer in the form , where p , q are constants.
-
18M.1.AHL.TZ2.H_11c:
The region R, is bounded by the graph of the function found in part (b), the x-axis, and the lines and where . The area of R is .
Find the value of .
-
19M.1.AHL.TZ1.H_7:
Find the coordinates of the points on the curve at which .
-
19M.1.AHL.TZ2.H_8b:
Given that there is one inscribed cone having a maximum volume, show that the volume of this cone is .
-
19M.2.AHL.TZ1.H_7:
The function is defined by , ≥ 1 and the function is defined by , ≥ 0.
The region is bounded by the curves , and the lines , and as shown on the following diagram.
The shape of a clay vase can be modelled by rotating the region through 360˚ about the -axis.
Find the volume of clay used to make the vase.
-
18N.2.AHL.TZ0.H_2:
A function satisfies the conditions , and its second derivative is , ≥ 0.
Find .
-
18M.2.AHL.TZ1.H_9a:
Show that there are exactly two points on the curve where the gradient is zero.
-
18M.2.AHL.TZ1.H_9b:
Find the equation of the normal to the curve at the point P.
-
18M.2.AHL.TZ1.H_9c:
The normal at P cuts the curve again at the point Q. Find the -coordinate of Q.
-
18M.2.AHL.TZ1.H_9d:
The shaded region is rotated by 2 about the -axis. Find the volume of the solid formed.
-
18M.2.AHL.TZ2.H_11a:
Show that .
-
18M.2.AHL.TZ2.H_11b.i:
Find the coordinates of P and Q.
-
18M.2.AHL.TZ2.H_11b.ii:
Given that the gradients of the tangents to C at P and Q are m1 and m2 respectively, show that m1 × m2 = 1.
-
18M.2.AHL.TZ2.H_11c:
Find the coordinates of the three points on C, nearest the origin, where the tangent is parallel to the line .
-
17N.1.AHL.TZ0.H_5:
A particle moves in a straight line such that at time seconds , its velocity , in , is given by . Find the exact distance travelled by the particle in the first half-second.
-
17N.1.AHL.TZ0.H_7:
The folium of Descartes is a curve defined by the equation , shown in the following diagram.
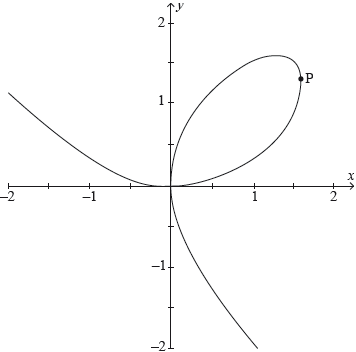
Determine the exact coordinates of the point P on the curve where the tangent line is parallel to the -axis.
-
17M.2.AHL.TZ1.H_11a:
Find his velocity when .
-
17M.2.AHL.TZ1.H_11b:
Calculate the vertical distance Xavier travelled in the first 10 seconds.
-
17M.2.AHL.TZ1.H_11c:
Determine the value of .
-
17M.2.AHL.TZ1.H_12a:
Find the largest possible domain for to be a function.
-
17M.2.AHL.TZ1.H_12b:
Sketch the graph of showing clearly the equations of asymptotes and the coordinates of any intercepts with the axes.
-
17M.2.AHL.TZ1.H_12c:
Explain why is an even function.
-
17M.2.AHL.TZ1.H_12d:
Explain why the inverse function does not exist.
-
17M.2.AHL.TZ1.H_12e:
Find the inverse function and state its domain.
-
17M.2.AHL.TZ1.H_12f:
Find .
-
17M.2.AHL.TZ1.H_12g.i:
Hence, show that there are no solutions to ;
-
17M.2.AHL.TZ1.H_12g.ii:
Hence, show that there are no solutions to .
-
17M.2.AHL.TZ1.H_2b:
Determine the equation of the tangent to at the point
-
16N.1.AHL.TZ0.H_9a:
Find an expression for in terms of and .
-
16N.1.AHL.TZ0.H_9b:
Find the equations of the tangents to this curve at the points where the curve intersects the line .
-
18N.2.SL.TZ0.S_4a:
Find when the particle is at rest.
-
18N.2.SL.TZ0.S_4b:
Find the acceleration of the particle when .
-
18N.2.SL.TZ0.S_4c:
Find the total distance travelled by the particle.
-
18N.2.SL.TZ0.S_10a:
Find the volume of the container.
-
18N.2.SL.TZ0.S_10b.i:
Find the value of and of .
-
18N.2.SL.TZ0.S_10b.ii:
During the interval < < , he volume of water in the container increases by m3. Find the value of .
-
18N.2.SL.TZ0.S_10c:
When = 0, the volume of water in the container is 2.3 m3. It is known that the container is never completely full of water during the 4 hour period.
Find the minimum volume of empty space in the container during the 4 hour period.
-
18N.1.SL.TZ0.S_6:
Let . The following diagram shows part of the graph of .
The region R is enclosed by the graph of , the -axis, and the -axis. Find the area of R.
-
17M.1.SL.TZ2.T_13a:
Write down the value of .
-
17M.1.SL.TZ2.T_13b:
Find the equation of . Give your answer in the form where , , .
-
17M.1.SL.TZ2.T_13c:
Draw the line on the diagram above.
-
19M.2.SL.TZ1.S_4a:
Sketch the graph of on the grid below:
-
19M.2.SL.TZ1.S_4b:
Find the -coordinates of the points of inflexion of the graph of .
-
18M.2.SL.TZ2.T_6b:
A teacher asks her students to make some observations about the curve.
Three students responded.
Nadia said “The x-intercept of the curve is between −1 and zero”.
Rick said “The curve is decreasing when x < 1 ”.
Paula said “The gradient of the curve is less than zero between x = 1 and x = 2 ”.State the name of the student who made an incorrect observation.
-
18M.1.SL.TZ2.S_9a:
Express h in terms of r.
-
18M.1.SL.TZ2.S_9b:
Show that .
-
18M.1.SL.TZ2.S_9c:
Given that there is a minimum value for C, find this minimum value in terms of .
-
18M.2.SL.TZ1.S_4a:
Write down the coordinates of the vertex of the graph of g.
-
18M.2.SL.TZ1.S_4b:
On the grid above, sketch the graph of g for −2 ≤ x ≤ 4.
-
18M.2.SL.TZ1.S_4c:
Find the area of the region enclosed by the graphs of f and g.
-
19M.2.SL.TZ1.S_4c:
Hence find the values of for which the graph of is concave-down.
-
19M.2.SL.TZ2.S_5a:
Find the population of fish at = 10.
-
19M.2.SL.TZ2.S_5b:
Find the rate at which the population of fish is increasing at = 10.
-
19M.2.SL.TZ2.S_5c:
Find the value of for which the population of fish is increasing most rapidly.
-
17M.2.SL.TZ1.T_6a:
Find .
-
17M.2.SL.TZ1.T_6b.i:
Show that .
-
17M.2.SL.TZ1.T_6b.ii:
Find the equation of the tangent to the graph of at . Give your answer in the form .
-
17M.2.SL.TZ1.T_6c:
Use your answer to part (a) and the value of , to find the -coordinates of the stationary points of the graph of .
-
17M.2.SL.TZ1.T_6d.i:
Find .
-
17M.2.SL.TZ1.T_6d.ii:
Hence justify that is decreasing at .
-
17M.2.SL.TZ1.T_6e:
Find the -coordinate of the local minimum.
-
18N.2.SL.TZ0.T_6a:
Calculate the area of cloth, in cm2, needed to make Haruka’s bag.
-
18N.2.SL.TZ0.T_6b:
Calculate the volume, in cm3, of the bag.
-
18N.2.SL.TZ0.T_6c:
Use this value to write down, and simplify, the equation in x and y for the volume of Nanako’s bag.
-
18N.2.SL.TZ0.T_6d:
Write down and simplify an expression in x and y for the area of cloth, A, used to make Nanako’s bag.
-
18N.2.SL.TZ0.T_6e:
Use your answers to parts (c) and (d) to show that
.
-
18N.2.SL.TZ0.T_6f:
Find .
-
18N.2.SL.TZ0.T_6g:
Use your answer to part (f) to show that the width of Nanako’s bag is 12 cm.
-
18N.2.SL.TZ0.T_6h:
The cloth used to make Nanako’s bag costs 4 Japanese Yen (JPY) per cm2.
Find the cost of the cloth used to make Nanako’s bag.
-
17M.1.SL.TZ2.S_5:
Let . Given that , find .
-
16N.1.SL.TZ0.S_6:
Let . Find , given that .
-
18M.2.SL.TZ1.S_1a:
Find f '(x).
-
18M.2.SL.TZ1.S_1b:
Find f "(x).
-
18M.2.SL.TZ1.S_1c:
Solve f '(x) = f "(x).
-
18M.1.SL.TZ1.S_5a:
Find .
-
18M.1.SL.TZ1.S_5b:
Part of the graph of f is shown in the following diagram.
The shaded region R is enclosed by the graph of f, the x-axis, and the lines x = 1 and x = 9 . Find the volume of the solid formed when R is revolved 360° about the x-axis.
-
18M.1.SL.TZ2.S_2a:
Find .
-
18M.1.SL.TZ2.S_2b:
Find the area of the region enclosed by the graph of , the x-axis and the lines x = 1 and x = 2 .
-
17M.1.SL.TZ1.S_6a.i:
Write down the gradient of the curve of at P.
-
17M.1.SL.TZ1.S_6a.ii:
Find the equation of the normal to the curve of at P.
-
17M.1.SL.TZ1.S_6b:
Determine the concavity of the graph of when and justify your answer.
-
17M.2.SL.TZ2.S_8a:
Find the value of .
-
17M.2.SL.TZ2.S_8b.i:
Write down the coordinates of A.
-
17M.2.SL.TZ2.S_8b.ii:
Write down the rate of change of at A.
-
17M.2.SL.TZ2.S_8c.i:
Find the coordinates of B.
-
17M.2.SL.TZ2.S_8c.ii:
Find the the rate of change of at B.
-
17M.2.SL.TZ2.S_8d:
Let be the region enclosed by the graph of , the -axis, the line and the line . The region is rotated 360° about the -axis. Find the volume of the solid formed.
-
17M.1.SL.TZ2.S_6a:
Find .
-
17M.1.SL.TZ2.S_6b:
Find .
-
17M.2.SL.TZ1.S_6:
Let . Find the term in in the expansion of the derivative, .
-
17N.1.SL.TZ0.S_7:
Consider , for , where .
The equation has exactly one solution. Find the value of .
-
18M.1.SL.TZ1.T_5b:
Find the gradient of the line DC.
-
18M.1.SL.TZ1.T_5c:
Find the equation of the line DC. Write your answer in the form ax + by + d = 0 where a , b and d are integers.
-
17N.2.SL.TZ0.S_5a:
Find the value of .
-
17N.2.SL.TZ0.S_5b:
The following diagram shows part of the graph of .

The region enclosed by the graph of , the -axis and the lines and is rotated 360° about the -axis. Find the volume of the solid formed.
-
16N.1.SL.TZ0.S_10a:
(i) Find the first four derivatives of .
(ii) Find .
-
16N.1.SL.TZ0.S_10b:
(i) Find the first three derivatives of .
(ii) Given that , find .
-
16N.1.SL.TZ0.S_10c:
(i) Find .
(ii) Hence, show that .
-
18M.1.SL.TZ1.S_7:
Consider f(x), g(x) and h(x), for x∈ where h(x) = (x).
Given that g(3) = 7 , g′ (3) = 4 and f ′ (7) = −5 , find the gradient of the normal to the curve of h at x = 3.
-
19N.1.SL.TZ0.T_14a:
Write down the value of .
-
19N.1.SL.TZ0.T_14b:
Find .
-
19N.1.SL.TZ0.T_14c:
At the point where , the gradient of the tangent to the curve is .
Find the value of .
-
19N.2.SL.TZ0.T_4a:
Find the value of .
-
19N.2.SL.TZ0.T_4b:
Write down the equation for the axis of symmetry of the graph.
-
19N.2.SL.TZ0.T_4c:
Use the symmetry of the graph to show that the second solution is .
-
19N.2.SL.TZ0.T_4d:
Write down the -intercepts of the graph.
-
19N.2.SL.TZ0.T_4e:
On graph paper, draw the graph of for and . Use a scale of to represent unit on the -axis and to represent units on the -axis.
-
19N.2.SL.TZ0.T_4f.i:
Write down the equation of .
-
19N.2.SL.TZ0.T_4f.ii:
Draw the tangent on your graph.
-
19N.2.SL.TZ0.T_4g:
Given and , state whether the function, , is increasing or decreasing at . Give a reason for your answer.
-
19N.2.SL.TZ0.T_6a:
Write down an expression for , the volume (cm3) of the speaker, in terms of , and .
-
19N.2.SL.TZ0.T_6b:
Write down an equation for the surface area of the speaker in terms of , and .
-
19N.2.SL.TZ0.T_6c:
Given the design constraint that , show that .
-
19N.2.SL.TZ0.T_6d:
Find .
-
19N.2.SL.TZ0.T_6e:
Using your answer to part (d), show that is a maximum when is equal to .
-
19N.2.SL.TZ0.T_6f:
Find the length of the cylinder for which is a maximum.
-
19N.2.SL.TZ0.T_6g:
Calculate the maximum value of .
-
19N.2.SL.TZ0.T_6h:
Use your answer to part (f) to identify the shape of the speaker with the best quality of sound.
-
19M.1.SL.TZ1.S_5:
The derivative of a function is given by . The graph of passes through .
Find .
-
17N.1.SL.TZ0.S_6:
Let , for . The following diagram shows part of the graph of and the rectangle OABC, where A is on the negative -axis, B is on the graph of , and C is on the -axis.
Find the -coordinate of A that gives the maximum area of OABC.
-
19M.1.SL.TZ1.S_8c:
Hence find the value of such that the area of PQRS is a maximum.
-
19M.2.SL.TZ1.S_9d.ii:
Hence, write down .
-
18M.1.AHL.TZ2.H_6b:
Hence, or otherwise, find .
-
17N.2.SL.TZ0.S_9d:
Find the total distance travelled by P when its velocity is increasing.
-
19M.1.SL.TZ2.S_9a:
Find the value of .
-
19M.2.SL.TZ2.S_8d:
Find the total distance travelled by particle A in the first 3 seconds.
-
17M.2.SL.TZ1.S_7b:
A second particle Q also moves along a straight line. Its velocity, after seconds is given by for . After seconds Q has travelled the same total distance as P.
Find .
-
16N.1.AHL.TZ0.H_11b:
Show that .
-
SPM.2.AHL.TZ0.7a:
By substituting into the equation, find an expression for the velocity of the particle at time . Give your answer in the form .
-
SPM.3.AHL.TZ0.2a.i:
Find the equilibrium population of brown squirrels suggested by this model.
-
18M.1.AHL.TZ1.H_4a:
.
-
17N.2.SL.TZ0.S_9a:
Write down the values of when .
-
16N.2.SL.TZ0.S_9a:
Find the initial velocity of .
-
16N.2.SL.TZ0.S_9c:
(i) Find the value of .
(ii) Hence, find the speed of P when .
-
19M.2.AHL.TZ1.H_1:
Let be the tangent to the curve at the point (1, ).
Find the coordinates of the point where meets the -axis.
-
19M.2.SL.TZ2.S_8b:
Find the value of when particle A first reaches point P.
-
17M.2.SL.TZ1.S_7a.i:
Write down the first value of at which P changes direction.
-
16N.1.AHL.TZ0.H_11c:
Show that the function has a local maximum value when .
-
SPM.3.AHL.TZ0.2d.i:
Write down the expressions for and that the conservationists will use.
-
SPM.3.AHL.TZ0.2e:
Use Euler’s method with step length 0.2 to sketch, on the same axes, the approximate trajectories for the populations with the following initial populations.
(i) ,
(ii) ,
-
18M.2.SL.TZ2.S_9c:
Write down the number of times that the acceleration of P is 0 m s−2 .
-
19M.1.SL.TZ1.S_8b:
Show that the area of PQRS is .
-
19M.1.SL.TZ1.S_7a:
Find the value of .
-
19M.1.SL.TZ2.S_10d:
Hence find the exact area of .
-
18M.2.SL.TZ2.S_9d:
Find the acceleration of P when it changes direction.
-
16N.1.AHL.TZ0.H_11a:
Find an expression for .
-
19M.1.SL.TZ1.S_8d:
Show that when the graphs of and intersect, .
-
19M.2.SL.TZ1.S_3b:
The graph of has a horizontal tangent line at and at . Find .
-
19M.1.SL.TZ2.S_9b:
Line passes through the origin and has a gradient of . Find the equation of .
-
SPM.3.AHL.TZ0.2c.i:
By using separation of variables, show that the general solution of is .
-
18M.1.AHL.TZ2.H_6a.ii:
Find .
-
18M.2.SL.TZ2.S_9e:
Find the total distance travelled by P.
-
19M.2.SL.TZ1.S_9a:
Find the gradient of .
-
SPM.2.AHL.TZ0.7g:
Use your answers to parts (d), (e) and (f) to comment on the accuracy of the Euler approximation to this model.
-
SPM.3.AHL.TZ0.2b.i:
Verify that , is an equilibrium point.
-
SPM.3.AHL.TZ0.2c.iii:
If both populations contain 10 squirrels at use the solutions to parts (c) (i) and (ii) to estimate the number of black and brown squirrels when . Give your answers to the nearest whole numbers.
-
SPM.3.AHL.TZ0.2d.iv:
Use the same method to find the long-term populations of squirrels when the initial populations are , .
-
19M.1.SL.TZ2.S_10b:
Hence find .
-
19M.2.SL.TZ1.S_9d.iii:
Hence or otherwise, find the obtuse angle formed by the tangent line to at and the tangent line to at .
-
SPM.2.AHL.TZ0.7e:
By repeated application of Euler’s method, find an approximation for the terminal velocity, to five significant figures.
-
SPM.3.AHL.TZ0.2d.iii:
Use further iterations of Euler’s method to find the long-term population for each species of squirrel from these initial values.
-
19M.1.SL.TZ2.S_10a:
Find .
-
18M.1.AHL.TZ1.H_4b:
.
-
19M.1.SL.TZ1.S_8e:
Given that the graphs of and intersect only once, find the value of .
-
19M.2.SL.TZ1.S_9c:
Find the acute angle between and .
-
19M.1.SL.TZ2.S_9d:
The following diagram shows the graph of for 0 ≤ ≤ 3. Line is a tangent to the graph of at point P.
Given that is parallel to , find the -coordinate of P.
-
SPM.2.AHL.TZ0.7c:
Write down the differential equation as a system of first order differential equations.
-
17N.2.SL.TZ0.S_9b:
Hence or otherwise, find all possible values of for which the velocity of P is decreasing.
-
17M.2.SL.TZ2.S_7:
Note: In this question, distance is in metres and time is in seconds.
A particle moves along a horizontal line starting at a fixed point A. The velocity of the particle, at time , is given by , for . The following diagram shows the graph of

There are -intercepts at and .
Find the maximum distance of the particle from A during the time and justify your answer.
-
19M.2.SL.TZ1.S_9b:
Find u.
-
19M.2.SL.TZ1.S_3a:
Find .
-
17M.2.SL.TZ1.S_7a.ii:
Find the total distance travelled by P, for .
-
16N.1.AHL.TZ0.H_11f:
Find the area of the region enclosed by the graph of and the -axis.
-
SPM.3.AHL.TZ0.2b.ii:
Find the other three equilibrium points.
-
SPM.3.AHL.TZ0.2d.ii:
Given that the initial populations are , , find the populations of each species of squirrel when .
-
19M.2.SL.TZ1.S_9d.i:
Find .
-
16N.2.AHL.TZ0.H_6:
An earth satellite moves in a path that can be described by the curve where and are in thousands of kilometres and is time in seconds.
Given that when , find the possible values of .
Give your answers in standard form.
-
SPM.2.AHL.TZ0.7d:
Use Euler’s method, with a step length of 0.2, to find the displacement and velocity of the object when .
-
SPM.3.AHL.TZ0.2a.ii:
Explain why the population of squirrels is increasing for values of less than this value.
-
SPM.3.AHL.TZ0.2f:
Given that the equilibrium point at (800, 600) is a saddle point, sketch the phase portrait for ≥ 0 , ≥ 0 on the same axes used in part (e).
-
19M.1.SL.TZ2.S_10c:
Write down an expression for the area of .
-
18M.1.AHL.TZ2.H_6a.i:
Find .
-
19M.2.SL.TZ2.S_8a:
Find the initial displacement of particle A from point P.
-
19M.2.SL.TZ2.S_8c:
Find the value of when particle A first changes direction.
-
19M.2.SL.TZ2.S_8e.ii:
Find the other value of when particles A and B meet.
-
16N.1.AHL.TZ0.H_11d:
Find the -coordinate of the point of inflexion of the graph of .
-
16N.1.AHL.TZ0.H_11h:
Find the value for and comment on its meaning with respect to the shape of the graph.
-
SPM.3.AHL.TZ0.2c.ii:
Write down the general solution of .
-
18M.2.SL.TZ2.S_9b:
Find the maximum speed of P.
-
19M.1.SL.TZ1.S_8a:
Find the -intercepts of the graph of .
-
19M.2.SL.TZ2.S_8e.i:
Given that particles A and B start at the same point, find the displacement function for particle B.
-
16N.1.AHL.TZ0.H_11g:
Find the value of the curvature of the graph of at the local maximum point.
-
SPM.2.AHL.TZ0.7f:
Use the differential equation to find the terminal velocity for the object.
-
19M.1.SL.TZ1.S_7b:
Find the total distance travelled in the first 5 seconds.
-
17N.2.SL.TZ0.S_9c:
Find an expression for the velocity of P at time .
-
16N.2.SL.TZ0.S_9b:
Find the value of .
-
19M.1.SL.TZ2.S_9c:
Find the derivative of .
-
16N.1.AHL.TZ0.H_11e:
Sketch the graph of , clearly indicating the position of the local maximum point, the point of inflexion and the axes intercepts.
-
SPM.2.AHL.TZ0.7b:
From your solution to part (a), or otherwise, find the terminal velocity of the object predicted by this model.
-
18M.2.SL.TZ2.S_9a:
Find the initial velocity of P.
-
16N.2.SL.TZ0.S_9d:
(i) Find the total distance travelled by P between and .
(ii) Hence or otherwise, find the displacement of P from A when .
-
18M.1.SL.TZ2.S_10c:
Let L2 be the tangent to the graph of g at P. L1 intersects L2 at the point Q.
Find the y-coordinate of Q.
-
18N.2.AHL.TZ0.H_9b:
Hence, or otherwise, find the coordinates of the point of inflexion on the graph of .
-
18N.2.AHL.TZ0.H_9c.ii:
sketch the graph of , showing clearly any axis intercepts and giving the equations of any asymptotes.
-
18M.1.SL.TZ2.S_10b:
Show that the graph of g has a gradient of 6 at P.
-
18M.1.SL.TZ2.S_10a.ii:
Find .
-
18M.1.SL.TZ2.S_10a.i:
Write down .
-
18N.2.AHL.TZ0.H_9c.i:
sketch the graph of , showing clearly any axis intercepts and giving the equations of any asymptotes.
-
18N.2.AHL.TZ0.H_9d:
Hence, or otherwise, solve the inequality .
-
18N.2.AHL.TZ0.H_9a:
Find .
Sub sections and their related questions
SL 5.1—Introduction of differential calculus
-
18M.2.AHL.TZ1.H_9a:
Show that there are exactly two points on the curve where the gradient is zero.
-
18M.2.AHL.TZ1.H_9b:
Find the equation of the normal to the curve at the point P.
-
18M.2.AHL.TZ1.H_9c:
The normal at P cuts the curve again at the point Q. Find the -coordinate of Q.
-
18M.2.AHL.TZ1.H_9d:
The shaded region is rotated by 2 about the -axis. Find the volume of the solid formed.
-
16N.2.SL.TZ0.T_6a:
Write down a formula for , the surface area to be coated.
-
16N.2.SL.TZ0.T_6b:
Express this volume in .
-
16N.2.SL.TZ0.T_6c:
Write down, in terms of and , an equation for the volume of this water container.
-
16N.2.SL.TZ0.T_6d:
Show that .
-
16N.2.SL.TZ0.T_6e:
Find .
-
16N.2.SL.TZ0.T_6f:
Using your answer to part (e), find the value of which minimizes .
-
16N.2.SL.TZ0.T_6g:
Find the value of this minimum area.
-
16N.2.SL.TZ0.T_6h:
Find the least number of cans of water-resistant material that will coat the area in part (g).
-
17N.2.AHL.TZ0.H_10a.i:
Show that the -coordinate of the minimum point on the curve satisfies the equation .
-
17N.2.AHL.TZ0.H_10a.ii:
Determine the values of for which is a decreasing function.
-
17N.2.AHL.TZ0.H_10b:
Sketch the graph of showing clearly the minimum point and any asymptotic behaviour.
-
17N.2.AHL.TZ0.H_10c:
Find the coordinates of the point on the graph of where the normal to the graph is parallel to the line .
-
17N.2.AHL.TZ0.H_10d:
This region is now rotated through radians about the -axis. Find the volume of revolution.
-
17N.2.AHL.TZ0.H_11a.i:
Determine an expression for in terms of .
-
17N.2.AHL.TZ0.H_11a.ii:
Sketch a graph of for .
-
17N.2.AHL.TZ0.H_11a.iii:
Find the -coordinate(s) of the point(s) of inflexion of the graph of , labelling these clearly on the graph of .
-
17N.2.AHL.TZ0.H_11b.i:
Express in terms of .
-
17N.2.AHL.TZ0.H_11b.ii:
Express in terms of .
-
17N.2.AHL.TZ0.H_11b.iii:
Hence show that can be expressed as .
-
17N.2.AHL.TZ0.H_11c:
Solve the equation , giving your answers in the form where .
-
18M.2.AHL.TZ2.H_11a:
Show that .
-
18M.2.AHL.TZ2.H_11b.i:
Find the coordinates of P and Q.
-
18M.2.AHL.TZ2.H_11b.ii:
Given that the gradients of the tangents to C at P and Q are m1 and m2 respectively, show that m1 × m2 = 1.
-
18M.2.AHL.TZ2.H_11c:
Find the coordinates of the three points on C, nearest the origin, where the tangent is parallel to the line .
-
17M.2.AHL.TZ1.H_2a:
Find in terms of and .
-
17M.2.AHL.TZ1.H_2b:
Determine the equation of the tangent to at the point
-
19M.1.AHL.TZ1.H_5:
A camera at point C is 3 m from the edge of a straight section of road as shown in the following diagram. The camera detects a car travelling along the road at = 0. It then rotates, always pointing at the car, until the car passes O, the point on the edge of the road closest to the camera.
A car travels along the road at a speed of 24 ms−1. Let the position of the car be X and let OĈX = θ.
Find , the rate of rotation of the camera, in radians per second, at the instant the car passes the point O .
-
16N.1.AHL.TZ0.H_9a:
Find an expression for in terms of and .
-
16N.1.AHL.TZ0.H_9b:
Find the equations of the tangents to this curve at the points where the curve intersects the line .
-
18M.2.SL.TZ2.T_6a:
Sketch the curve for −1 < x < 3 and −2 < y < 12.
-
18M.2.SL.TZ2.T_6b:
A teacher asks her students to make some observations about the curve.
Three students responded.
Nadia said “The x-intercept of the curve is between −1 and zero”.
Rick said “The curve is decreasing when x < 1 ”.
Paula said “The gradient of the curve is less than zero between x = 1 and x = 2 ”.State the name of the student who made an incorrect observation.
-
18M.2.SL.TZ2.T_6d:
Find .
-
18M.2.SL.TZ2.T_6f:
Given that y = 2x3 − 9x2 + 12x + 2 = k has three solutions, find the possible values of k.
-
19M.2.SL.TZ2.T_5d:
Find .
-
19M.2.SL.TZ2.T_5e:
Find the gradient of the graph of at .
-
19M.2.SL.TZ2.T_5f:
Find the equation of the tangent line to the graph of at . Give the equation in the form where, , , and .
-
17M.2.SL.TZ1.T_6a:
Find .
-
17M.2.SL.TZ1.T_6b.i:
Show that .
-
17M.2.SL.TZ1.T_6b.ii:
Find the equation of the tangent to the graph of at . Give your answer in the form .
-
17M.2.SL.TZ1.T_6c:
Use your answer to part (a) and the value of , to find the -coordinates of the stationary points of the graph of .
-
17M.2.SL.TZ1.T_6d.i:
Find .
-
17M.2.SL.TZ1.T_6d.ii:
Hence justify that is decreasing at .
-
17M.2.SL.TZ1.T_6e:
Find the -coordinate of the local minimum.
-
18M.1.SL.TZ2.T_14a:
Find f'(x)
-
18M.1.SL.TZ2.T_14b:
Find the gradient of the graph of f at .
-
18M.1.SL.TZ2.T_14c:
Find the x-coordinate of the point at which the normal to the graph of f has gradient .
-
17M.2.SL.TZ2.T_6a:
Write down the -intercept of the graph.
-
17M.2.SL.TZ2.T_6b:
Find .
-
17M.2.SL.TZ2.T_6c.i:
Show that .
-
17M.2.SL.TZ2.T_6c.ii:
Find .
-
17M.2.SL.TZ2.T_6d.i:
Write down the -coordinates of these two points;
-
17M.2.SL.TZ2.T_6d.ii:
Write down the intervals where the gradient of the graph of is positive.
-
17M.2.SL.TZ2.T_6e:
Write down the range of .
-
17M.2.SL.TZ2.T_6f:
Write down the number of possible solutions to the equation .
-
17M.2.SL.TZ2.T_6g:
The equation , where , has four solutions. Find the possible values of .
-
17N.1.SL.TZ0.T_14a:
Write down the derivative of .
-
17N.1.SL.TZ0.T_14b:
Find the point on the graph of at which the gradient of the tangent is equal to 6.
-
19M.2.SL.TZ1.T_6a:
Show that .
-
19M.2.SL.TZ1.T_6b:
Find the coordinates of the local minimum.
-
19M.2.SL.TZ1.T_6c:
Write down the interval where the gradient of the graph of is negative.
-
19M.2.SL.TZ1.T_6d:
Determine the equation of the normal at in the form .
-
18M.1.SL.TZ1.T_5a:
Write down the coordinates of C, the midpoint of line segment AB.
-
18M.1.SL.TZ1.T_5b:
Find the gradient of the line DC.
-
18M.1.SL.TZ1.T_5c:
Find the equation of the line DC. Write your answer in the form ax + by + d = 0 where a , b and d are integers.
-
18N.2.SL.TZ0.T_6a:
Calculate the area of cloth, in cm2, needed to make Haruka’s bag.
-
18N.2.SL.TZ0.T_6b:
Calculate the volume, in cm3, of the bag.
-
18N.2.SL.TZ0.T_6c:
Use this value to write down, and simplify, the equation in x and y for the volume of Nanako’s bag.
-
18N.2.SL.TZ0.T_6d:
Write down and simplify an expression in x and y for the area of cloth, A, used to make Nanako’s bag.
-
18N.2.SL.TZ0.T_6e:
Use your answers to parts (c) and (d) to show that
.
-
18N.2.SL.TZ0.T_6f:
Find .
-
18N.2.SL.TZ0.T_6g:
Use your answer to part (f) to show that the width of Nanako’s bag is 12 cm.
-
18N.2.SL.TZ0.T_6h:
The cloth used to make Nanako’s bag costs 4 Japanese Yen (JPY) per cm2.
Find the cost of the cloth used to make Nanako’s bag.
-
21N.1.AHL.TZ0.8a.i:
Identify the value of the point where has its maximum value.
-
21N.1.AHL.TZ0.8a.ii:
Interpret this point in the given context.
-
21N.1.AHL.TZ0.8b:
Juri starts at a height of metres and finishes at , where .
Sketch a possible diagram of the hill on the following pair of coordinate axes.
-
22M.3.AHL.TZ1.1e:
Determine the value of and of . Give your answers correct to one decimal place.
-
22M.2.AHL.TZ2.4b:
Given that and , sketch the graph of against .
SL 5.2—Increasing and decreasing functions
-
18M.2.SL.TZ2.T_6a:
Sketch the curve for −1 < x < 3 and −2 < y < 12.
-
18M.2.SL.TZ2.T_6b:
A teacher asks her students to make some observations about the curve.
Three students responded.
Nadia said “The x-intercept of the curve is between −1 and zero”.
Rick said “The curve is decreasing when x < 1 ”.
Paula said “The gradient of the curve is less than zero between x = 1 and x = 2 ”.State the name of the student who made an incorrect observation.
-
18M.2.SL.TZ2.T_6d:
Find .
-
18M.2.SL.TZ2.T_6f:
Given that y = 2x3 − 9x2 + 12x + 2 = k has three solutions, find the possible values of k.
-
17M.2.SL.TZ1.T_6a:
Find .
-
17M.2.SL.TZ1.T_6b.i:
Show that .
-
17M.2.SL.TZ1.T_6b.ii:
Find the equation of the tangent to the graph of at . Give your answer in the form .
-
17M.2.SL.TZ1.T_6c:
Use your answer to part (a) and the value of , to find the -coordinates of the stationary points of the graph of .
-
17M.2.SL.TZ1.T_6d.i:
Find .
-
17M.2.SL.TZ1.T_6d.ii:
Hence justify that is decreasing at .
-
17M.2.SL.TZ1.T_6e:
Find the -coordinate of the local minimum.
-
17N.2.SL.TZ0.T_5a:
Find the exact value of each of the zeros of .
-
17N.2.SL.TZ0.T_5b.i:
Expand the expression for .
-
17N.2.SL.TZ0.T_5b.ii:
Find .
-
17N.2.SL.TZ0.T_5c:
Use your answer to part (b)(ii) to find the values of for which is increasing.
-
17N.2.SL.TZ0.T_5d:
Draw the graph of for and . Use a scale of 2 cm to represent 1 unit on the -axis and 1 cm to represent 5 units on the -axis.
-
17N.2.SL.TZ0.T_5e:
Write down the coordinates of the point of intersection.
-
17M.2.SL.TZ2.T_6a:
Write down the -intercept of the graph.
-
17M.2.SL.TZ2.T_6b:
Find .
-
17M.2.SL.TZ2.T_6c.i:
Show that .
-
17M.2.SL.TZ2.T_6c.ii:
Find .
-
17M.2.SL.TZ2.T_6d.i:
Write down the -coordinates of these two points;
-
17M.2.SL.TZ2.T_6d.ii:
Write down the intervals where the gradient of the graph of is positive.
-
17M.2.SL.TZ2.T_6e:
Write down the range of .
-
17M.2.SL.TZ2.T_6f:
Write down the number of possible solutions to the equation .
-
17M.2.SL.TZ2.T_6g:
The equation , where , has four solutions. Find the possible values of .
-
19M.2.SL.TZ1.T_6a:
Show that .
-
19M.2.SL.TZ1.T_6b:
Find the coordinates of the local minimum.
-
19M.2.SL.TZ1.T_6c:
Write down the interval where the gradient of the graph of is negative.
-
19M.2.SL.TZ1.T_6d:
Determine the equation of the normal at in the form .
-
19N.2.SL.TZ0.T_4a:
Find the value of .
-
19N.2.SL.TZ0.T_4b:
Write down the equation for the axis of symmetry of the graph.
-
19N.2.SL.TZ0.T_4c:
Use the symmetry of the graph to show that the second solution is .
-
19N.2.SL.TZ0.T_4d:
Write down the -intercepts of the graph.
-
19N.2.SL.TZ0.T_4e:
On graph paper, draw the graph of for and . Use a scale of to represent unit on the -axis and to represent units on the -axis.
-
19N.2.SL.TZ0.T_4f.i:
Write down the equation of .
-
19N.2.SL.TZ0.T_4f.ii:
Draw the tangent on your graph.
-
19N.2.SL.TZ0.T_4g:
Given and , state whether the function, , is increasing or decreasing at . Give a reason for your answer.
-
21M.1.SL.TZ1.13a:
Determine whether the graph of against is increasing or decreasing at .
-
21M.1.SL.TZ1.13b:
Sieun observes that when the angle is , the ball will travel a horizontal distance of .
Find an expression for the function .
-
21N.1.AHL.TZ0.8a.i:
Identify the value of the point where has its maximum value.
-
21N.1.AHL.TZ0.8a.ii:
Interpret this point in the given context.
-
21N.1.AHL.TZ0.8b:
Juri starts at a height of metres and finishes at , where .
Sketch a possible diagram of the hill on the following pair of coordinate axes.
-
22M.1.SL.TZ1.12a:
Determine whether the profit is increasing or decreasing when .
-
22M.2.SL.TZ1.4c:
Find the value of at which the goat is eating grass at the greatest rate.
-
22M.2.AHL.TZ1.2c:
Find the value of at which the goat is eating grass at the greatest rate.
SL 5.3—Differentiating polynomials, n E Z
-
SPM.2.SL.TZ0.4a:
Calculate the surface area of the box in cm2.
-
SPM.2.SL.TZ0.4b:
Calculate the length AG.
-
SPM.2.SL.TZ0.4c:
Find the number of boxes that should be sold each week to maximize the profit.
-
SPM.2.SL.TZ0.4d:
Find .
-
SPM.2.SL.TZ0.4e:
Find the least number of boxes which must be sold each week in order to make a profit.
-
16N.2.SL.TZ0.T_6a:
Write down a formula for , the surface area to be coated.
-
16N.2.SL.TZ0.T_6b:
Express this volume in .
-
16N.2.SL.TZ0.T_6c:
Write down, in terms of and , an equation for the volume of this water container.
-
16N.2.SL.TZ0.T_6d:
Show that .
-
16N.2.SL.TZ0.T_6e:
Find .
-
16N.2.SL.TZ0.T_6f:
Using your answer to part (e), find the value of which minimizes .
-
16N.2.SL.TZ0.T_6g:
Find the value of this minimum area.
-
16N.2.SL.TZ0.T_6h:
Find the least number of cans of water-resistant material that will coat the area in part (g).
-
17M.2.AHL.TZ1.H_12a:
Find the largest possible domain for to be a function.
-
17M.2.AHL.TZ1.H_12b:
Sketch the graph of showing clearly the equations of asymptotes and the coordinates of any intercepts with the axes.
-
17M.2.AHL.TZ1.H_12c:
Explain why is an even function.
-
17M.2.AHL.TZ1.H_12d:
Explain why the inverse function does not exist.
-
17M.2.AHL.TZ1.H_12e:
Find the inverse function and state its domain.
-
17M.2.AHL.TZ1.H_12f:
Find .
-
17M.2.AHL.TZ1.H_12g.i:
Hence, show that there are no solutions to ;
-
17M.2.AHL.TZ1.H_12g.ii:
Hence, show that there are no solutions to .
-
17M.2.AHL.TZ1.H_8a:
Find an expression for the volume of water in the trough in terms of .
-
17M.2.AHL.TZ1.H_8b:
Calculate when .
-
18M.2.AHL.TZ2.H_11a:
Show that .
-
18M.2.AHL.TZ2.H_11b.i:
Find the coordinates of P and Q.
-
18M.2.AHL.TZ2.H_11b.ii:
Given that the gradients of the tangents to C at P and Q are m1 and m2 respectively, show that m1 × m2 = 1.
-
18M.2.AHL.TZ2.H_11c:
Find the coordinates of the three points on C, nearest the origin, where the tangent is parallel to the line .
-
17M.2.AHL.TZ1.H_2a:
Find in terms of and .
-
17M.2.AHL.TZ1.H_2b:
Determine the equation of the tangent to at the point
-
16N.1.AHL.TZ0.H_9a:
Find an expression for in terms of and .
-
16N.1.AHL.TZ0.H_9b:
Find the equations of the tangents to this curve at the points where the curve intersects the line .
-
18M.1.AHL.TZ1.H_2a:
Find
-
18M.1.AHL.TZ1.H_2b:
Hence find the values of θ for which .
-
19M.1.AHL.TZ1.H_7:
Find the coordinates of the points on the curve at which .
-
17N.1.AHL.TZ0.H_7:
The folium of Descartes is a curve defined by the equation , shown in the following diagram.

Determine the exact coordinates of the point P on the curve where the tangent line is parallel to the -axis.
-
19M.2.SL.TZ1.S_9a:
Find the gradient of .
-
19M.2.SL.TZ1.S_9b:
Find u.
-
19M.2.SL.TZ1.S_9c:
Find the acute angle between and .
-
19M.2.SL.TZ1.S_9d.i:
Find .
-
19M.2.SL.TZ1.S_9d.ii:
Hence, write down .
-
19M.2.SL.TZ1.S_9d.iii:
Hence or otherwise, find the obtuse angle formed by the tangent line to at and the tangent line to at .
-
18M.1.SL.TZ1.S_7:
Consider f(x), g(x) and h(x), for x∈ where h(x) = (x).
Given that g(3) = 7 , g′ (3) = 4 and f ′ (7) = −5 , find the gradient of the normal to the curve of h at x = 3.
-
18N.1.SL.TZ0.S_10a:
Find the coordinates of P.
-
18N.1.SL.TZ0.S_10b.i:
Find .
-
18N.1.SL.TZ0.S_10b.ii:
Hence, find the equation of L in terms of .
-
18N.1.SL.TZ0.S_10c:
The graph of has a local minimum at the point Q. The line L passes through Q.
Find the value of .
-
18M.2.SL.TZ1.S_1a:
Find f '(x).
-
18M.2.SL.TZ1.S_1b:
Find f "(x).
-
18M.2.SL.TZ1.S_1c:
Solve f '(x) = f "(x).
-
17M.2.SL.TZ1.S_6:
Let . Find the term in in the expansion of the derivative, .
-
18M.1.SL.TZ2.S_9a:
Express h in terms of r.
-
18M.1.SL.TZ2.S_9b:
Show that .
-
18M.1.SL.TZ2.S_9c:
Given that there is a minimum value for C, find this minimum value in terms of .
-
17M.1.SL.TZ2.S_6a:
Find .
-
17M.1.SL.TZ2.S_6b:
Find .
-
16N.1.SL.TZ0.S_10a:
(i) Find the first four derivatives of .
(ii) Find .
-
16N.1.SL.TZ0.S_10b:
(i) Find the first three derivatives of .
(ii) Given that , find .
-
16N.1.SL.TZ0.S_10c:
(i) Find .
(ii) Hence, show that .
-
19M.1.SL.TZ1.T_15a:
Write down an equation for the area, , of the curved surface in terms of .
-
19M.1.SL.TZ1.T_15b:
Find .
-
19M.1.SL.TZ1.T_15c:
Find the value of when the area of the curved surface is maximized.
-
18M.2.SL.TZ2.T_6a:
Sketch the curve for −1 < x < 3 and −2 < y < 12.
-
18M.2.SL.TZ2.T_6b:
A teacher asks her students to make some observations about the curve.
Three students responded.
Nadia said “The x-intercept of the curve is between −1 and zero”.
Rick said “The curve is decreasing when x < 1 ”.
Paula said “The gradient of the curve is less than zero between x = 1 and x = 2 ”.State the name of the student who made an incorrect observation.
-
18M.2.SL.TZ2.T_6d:
Find .
-
18M.2.SL.TZ2.T_6f:
Given that y = 2x3 − 9x2 + 12x + 2 = k has three solutions, find the possible values of k.
-
19M.2.SL.TZ2.T_5d:
Find .
-
19M.2.SL.TZ2.T_5e:
Find the gradient of the graph of at .
-
19M.2.SL.TZ2.T_5f:
Find the equation of the tangent line to the graph of at . Give the equation in the form where, , , and .
-
17M.2.SL.TZ1.T_6a:
Find .
-
17M.2.SL.TZ1.T_6b.i:
Show that .
-
17M.2.SL.TZ1.T_6b.ii:
Find the equation of the tangent to the graph of at . Give your answer in the form .
-
17M.2.SL.TZ1.T_6c:
Use your answer to part (a) and the value of , to find the -coordinates of the stationary points of the graph of .
-
17M.2.SL.TZ1.T_6d.i:
Find .
-
17M.2.SL.TZ1.T_6d.ii:
Hence justify that is decreasing at .
-
17M.2.SL.TZ1.T_6e:
Find the -coordinate of the local minimum.
-
18M.1.SL.TZ2.T_14a:
Find f'(x)
-
18M.1.SL.TZ2.T_14b:
Find the gradient of the graph of f at .
-
18M.1.SL.TZ2.T_14c:
Find the x-coordinate of the point at which the normal to the graph of f has gradient .
-
17N.2.SL.TZ0.T_5a:
Find the exact value of each of the zeros of .
-
17N.2.SL.TZ0.T_5b.i:
Expand the expression for .
-
17N.2.SL.TZ0.T_5b.ii:
Find .
-
17N.2.SL.TZ0.T_5c:
Use your answer to part (b)(ii) to find the values of for which is increasing.
-
17N.2.SL.TZ0.T_5d:
Draw the graph of for and . Use a scale of 2 cm to represent 1 unit on the -axis and 1 cm to represent 5 units on the -axis.
-
17N.2.SL.TZ0.T_5e:
Write down the coordinates of the point of intersection.
-
17M.2.SL.TZ2.T_6a:
Write down the -intercept of the graph.
-
17M.2.SL.TZ2.T_6b:
Find .
-
17M.2.SL.TZ2.T_6c.i:
Show that .
-
17M.2.SL.TZ2.T_6c.ii:
Find .
-
17M.2.SL.TZ2.T_6d.i:
Write down the -coordinates of these two points;
-
17M.2.SL.TZ2.T_6d.ii:
Write down the intervals where the gradient of the graph of is positive.
-
17M.2.SL.TZ2.T_6e:
Write down the range of .
-
17M.2.SL.TZ2.T_6f:
Write down the number of possible solutions to the equation .
-
17M.2.SL.TZ2.T_6g:
The equation , where , has four solutions. Find the possible values of .
-
17N.1.SL.TZ0.T_14a:
Write down the derivative of .
-
17N.1.SL.TZ0.T_14b:
Find the point on the graph of at which the gradient of the tangent is equal to 6.
-
19M.1.SL.TZ2.T_15a:
Find the value of if no vases are sold.
-
19M.1.SL.TZ2.T_15b:
Differentiate .
-
19M.2.SL.TZ1.T_6a:
Show that .
-
19M.2.SL.TZ1.T_6b:
Find the coordinates of the local minimum.
-
19M.2.SL.TZ1.T_6c:
Write down the interval where the gradient of the graph of is negative.
-
19M.2.SL.TZ1.T_6d:
Determine the equation of the normal at in the form .
-
18N.2.SL.TZ0.T_6a:
Calculate the area of cloth, in cm2, needed to make Haruka’s bag.
-
18N.2.SL.TZ0.T_6b:
Calculate the volume, in cm3, of the bag.
-
18N.2.SL.TZ0.T_6c:
Use this value to write down, and simplify, the equation in x and y for the volume of Nanako’s bag.
-
18N.2.SL.TZ0.T_6d:
Write down and simplify an expression in x and y for the area of cloth, A, used to make Nanako’s bag.
-
18N.2.SL.TZ0.T_6e:
Use your answers to parts (c) and (d) to show that
.
-
18N.2.SL.TZ0.T_6f:
Find .
-
18N.2.SL.TZ0.T_6g:
Use your answer to part (f) to show that the width of Nanako’s bag is 12 cm.
-
18N.2.SL.TZ0.T_6h:
The cloth used to make Nanako’s bag costs 4 Japanese Yen (JPY) per cm2.
Find the cost of the cloth used to make Nanako’s bag.
-
16N.1.SL.TZ0.T_14a:
Find .
-
16N.1.SL.TZ0.T_14b:
Find the coordinates of P.
-
19N.1.SL.TZ0.T_14a:
Write down the value of .
-
19N.1.SL.TZ0.T_14b:
Find .
-
19N.1.SL.TZ0.T_14c:
At the point where , the gradient of the tangent to the curve is .
Find the value of .
-
19N.2.SL.TZ0.T_6a:
Write down an expression for , the volume (cm3) of the speaker, in terms of , and .
-
19N.2.SL.TZ0.T_6b:
Write down an equation for the surface area of the speaker in terms of , and .
-
19N.2.SL.TZ0.T_6c:
Given the design constraint that , show that .
-
19N.2.SL.TZ0.T_6d:
Find .
-
19N.2.SL.TZ0.T_6e:
Using your answer to part (d), show that is a maximum when is equal to .
-
19N.2.SL.TZ0.T_6f:
Find the length of the cylinder for which is a maximum.
-
19N.2.SL.TZ0.T_6g:
Calculate the maximum value of .
-
19N.2.SL.TZ0.T_6h:
Use your answer to part (f) to identify the shape of the speaker with the best quality of sound.
-
20N.1.SL.TZ0.S_10a.i:
Find in terms of and .
-
20N.1.SL.TZ0.S_10a.ii:
Show that the equation of is .
-
20N.1.SL.TZ0.S_10b:
Find the area of triangle in terms of .
-
20N.1.SL.TZ0.S_10c:
The graph of is translated by to give the graph of .
In the following diagram:- point lies on the graph of
- points , and lie on the vertical asymptote of
- points and lie on the horizontal asymptote of
- point lies on the -axis such that is parallel to .
Line is the tangent to the graph of at , and passes through and .
Given that triangle and rectangle have equal areas, find the gradient of in terms of .
-
20N.1.SL.TZ0.T_13a:
Write down .
-
20N.1.SL.TZ0.T_13b:
Write down the gradient of this tangent.
-
20N.1.SL.TZ0.T_13c:
Find the value of .
-
EXN.1.SL.TZ0.7a:
Find an expression for .
-
EXN.1.SL.TZ0.7b:
Show that the normal to the curve at the point where is .
-
EXN.2.SL.TZ0.2a:
Find an expression for the total length of the ribbon in terms of and .
-
EXN.2.SL.TZ0.2b:
Show that
-
EXN.2.SL.TZ0.2c:
Find
-
EXN.2.SL.TZ0.2d:
Solve
-
EXN.2.SL.TZ0.2e:
Hence or otherwise find the minimum length of ribbon required.
-
EXN.2.SL.TZ0.6d:
Use differentiation to show that .
-
21M.2.AHL.TZ1.2a.i:
Find .
-
21M.2.SL.TZ2.5d:
Find an expression for .
-
21M.2.SL.TZ2.5e:
Find the value of which maximizes the volume of the box.
-
21N.1.SL.TZ0.12a:
Find .
-
21N.1.SL.TZ0.12b.i:
Solve .
-
21N.1.SL.TZ0.12b.ii:
Interpret your answer to (b)(i) in context.
-
22M.1.SL.TZ1.9a:
Find .
-
22M.1.SL.TZ2.11a:
Find .
-
22M.1.SL.TZ2.11b:
Use your answer to part (a) to find the gradient of .
-
22M.2.SL.TZ2.5a:
Find an expression for in terms of and .
SL 5.4—Tangents and normal
-
18M.2.AHL.TZ1.H_9a:
Show that there are exactly two points on the curve where the gradient is zero.
-
18M.2.AHL.TZ1.H_9b:
Find the equation of the normal to the curve at the point P.
-
18M.2.AHL.TZ1.H_9c:
The normal at P cuts the curve again at the point Q. Find the -coordinate of Q.
-
18M.2.AHL.TZ1.H_9d:
The shaded region is rotated by 2 about the -axis. Find the volume of the solid formed.
-
17N.2.AHL.TZ0.H_10a.i:
Show that the -coordinate of the minimum point on the curve satisfies the equation .
-
17N.2.AHL.TZ0.H_10a.ii:
Determine the values of for which is a decreasing function.
-
17N.2.AHL.TZ0.H_10b:
Sketch the graph of showing clearly the minimum point and any asymptotic behaviour.
-
17N.2.AHL.TZ0.H_10c:
Find the coordinates of the point on the graph of where the normal to the graph is parallel to the line .
-
17N.2.AHL.TZ0.H_10d:
This region is now rotated through radians about the -axis. Find the volume of revolution.
-
18M.2.AHL.TZ2.H_11a:
Show that .
-
18M.2.AHL.TZ2.H_11b.i:
Find the coordinates of P and Q.
-
18M.2.AHL.TZ2.H_11b.ii:
Given that the gradients of the tangents to C at P and Q are m1 and m2 respectively, show that m1 × m2 = 1.
-
18M.2.AHL.TZ2.H_11c:
Find the coordinates of the three points on C, nearest the origin, where the tangent is parallel to the line .
-
17M.2.AHL.TZ1.H_2a:
Find in terms of and .
-
17M.2.AHL.TZ1.H_2b:
Determine the equation of the tangent to at the point
-
19M.1.AHL.TZ1.H_5:
A camera at point C is 3 m from the edge of a straight section of road as shown in the following diagram. The camera detects a car travelling along the road at = 0. It then rotates, always pointing at the car, until the car passes O, the point on the edge of the road closest to the camera.
A car travels along the road at a speed of 24 ms−1. Let the position of the car be X and let OĈX = θ.
Find , the rate of rotation of the camera, in radians per second, at the instant the car passes the point O .
-
16N.1.AHL.TZ0.H_9a:
Find an expression for in terms of and .
-
16N.1.AHL.TZ0.H_9b:
Find the equations of the tangents to this curve at the points where the curve intersects the line .
-
17N.1.SL.TZ0.S_5a:
Find .
-
17N.1.SL.TZ0.S_5b:
Given that , find the value of .
-
18M.1.SL.TZ2.S_10a.i:
Write down .
-
18M.1.SL.TZ2.S_10a.ii:
Find .
-
18M.1.SL.TZ2.S_10b:
Show that the graph of g has a gradient of 6 at P.
-
18M.1.SL.TZ2.S_10c:
Let L2 be the tangent to the graph of g at P. L1 intersects L2 at the point Q.
Find the y-coordinate of Q.
-
16N.2.SL.TZ0.S_10a:
(i) Find the value of .
(ii) Show that .
(iii) Find the value of .
-
16N.2.SL.TZ0.S_10b:
(i) Write down the value of .
(ii) Find .
-
16N.2.SL.TZ0.S_10c:
(i) Find .
(ii) Hence or otherwise, find the maximum positive rate of change of .
-
17M.2.SL.TZ2.S_8a:
Find the value of .
-
17M.2.SL.TZ2.S_8b.i:
Write down the coordinates of A.
-
17M.2.SL.TZ2.S_8b.ii:
Write down the rate of change of at A.
-
17M.2.SL.TZ2.S_8c.i:
Find the coordinates of B.
-
17M.2.SL.TZ2.S_8c.ii:
Find the the rate of change of at B.
-
17M.2.SL.TZ2.S_8d:
Let be the region enclosed by the graph of , the -axis, the line and the line . The region is rotated 360° about the -axis. Find the volume of the solid formed.
-
18N.2.SL.TZ0.S_10a:
Find the volume of the container.
-
18N.2.SL.TZ0.S_10b.i:
Find the value of and of .
-
18N.2.SL.TZ0.S_10b.ii:
During the interval < < , he volume of water in the container increases by m3. Find the value of .
-
18N.2.SL.TZ0.S_10c:
When = 0, the volume of water in the container is 2.3 m3. It is known that the container is never completely full of water during the 4 hour period.
Find the minimum volume of empty space in the container during the 4 hour period.
-
18N.2.SL.TZ0.T_4a:
Sketch the graph of y = f (x), for −4 ≤ x ≤ 3 and −50 ≤ y ≤ 100.
-
18N.2.SL.TZ0.T_4b.i:
Use your graphic display calculator to find the zero of f (x).
-
18N.2.SL.TZ0.T_4b.ii:
Use your graphic display calculator to find the coordinates of the local minimum point.
-
18N.2.SL.TZ0.T_4b.iii:
Use your graphic display calculator to find the equation of the tangent to the graph of y = f (x) at the point (–2, 38.75).
Give your answer in the form y = mx + c.
-
17N.1.SL.TZ0.T_2c.ii:
Write down, in the form , the equation of .
-
18M.2.SL.TZ1.T_4a:
Find the value of k.
-
18M.2.SL.TZ1.T_4b:
Using your value of k , find f ′(x).
-
18M.2.SL.TZ1.T_4c:
Use your answer to part (b) to show that the minimum value of f(x) is −22 .
-
18M.2.SL.TZ1.T_4e:
Sketch the graph of y = f (x) for 0 < x ≤ 6 and −30 ≤ y ≤ 60.
Clearly indicate the minimum point P and the x-intercepts on your graph. -
18M.2.SL.TZ2.T_6a:
Sketch the curve for −1 < x < 3 and −2 < y < 12.
-
18M.2.SL.TZ2.T_6b:
A teacher asks her students to make some observations about the curve.
Three students responded.
Nadia said “The x-intercept of the curve is between −1 and zero”.
Rick said “The curve is decreasing when x < 1 ”.
Paula said “The gradient of the curve is less than zero between x = 1 and x = 2 ”.State the name of the student who made an incorrect observation.
-
18M.2.SL.TZ2.T_6d:
Find .
-
18M.2.SL.TZ2.T_6f:
Given that y = 2x3 − 9x2 + 12x + 2 = k has three solutions, find the possible values of k.
-
19M.2.SL.TZ2.T_5d:
Find .
-
19M.2.SL.TZ2.T_5e:
Find the gradient of the graph of at .
-
19M.2.SL.TZ2.T_5f:
Find the equation of the tangent line to the graph of at . Give the equation in the form where, , , and .
-
17M.2.SL.TZ1.T_6a:
Find .
-
17M.2.SL.TZ1.T_6b.i:
Show that .
-
17M.2.SL.TZ1.T_6b.ii:
Find the equation of the tangent to the graph of at . Give your answer in the form .
-
17M.2.SL.TZ1.T_6c:
Use your answer to part (a) and the value of , to find the -coordinates of the stationary points of the graph of .
-
17M.2.SL.TZ1.T_6d.i:
Find .
-
17M.2.SL.TZ1.T_6d.ii:
Hence justify that is decreasing at .
-
17M.2.SL.TZ1.T_6e:
Find the -coordinate of the local minimum.
-
18M.1.SL.TZ2.T_14a:
Find f'(x)
-
18M.1.SL.TZ2.T_14b:
Find the gradient of the graph of f at .
-
18M.1.SL.TZ2.T_14c:
Find the x-coordinate of the point at which the normal to the graph of f has gradient .
-
17M.2.SL.TZ2.T_6a:
Write down the -intercept of the graph.
-
17M.2.SL.TZ2.T_6b:
Find .
-
17M.2.SL.TZ2.T_6c.i:
Show that .
-
17M.2.SL.TZ2.T_6c.ii:
Find .
-
17M.2.SL.TZ2.T_6d.i:
Write down the -coordinates of these two points;
-
17M.2.SL.TZ2.T_6d.ii:
Write down the intervals where the gradient of the graph of is positive.
-
17M.2.SL.TZ2.T_6e:
Write down the range of .
-
17M.2.SL.TZ2.T_6f:
Write down the number of possible solutions to the equation .
-
17M.2.SL.TZ2.T_6g:
The equation , where , has four solutions. Find the possible values of .
-
17N.1.SL.TZ0.T_14a:
Write down the derivative of .
-
17N.1.SL.TZ0.T_14b:
Find the point on the graph of at which the gradient of the tangent is equal to 6.
-
18N.1.SL.TZ0.T_11a:
Find .
-
18N.1.SL.TZ0.T_11b:
Find the gradient of this tangent at point P.
-
18N.1.SL.TZ0.T_11c:
Find the equation of this tangent. Give your answer in the form y = mx + c.
-
17M.1.SL.TZ2.T_13a:
Write down the value of .
-
17M.1.SL.TZ2.T_13b:
Find the equation of . Give your answer in the form where , , .
-
17M.1.SL.TZ2.T_13c:
Draw the line on the diagram above.
-
19M.2.SL.TZ1.T_6a:
Show that .
-
19M.2.SL.TZ1.T_6b:
Find the coordinates of the local minimum.
-
19M.2.SL.TZ1.T_6c:
Write down the interval where the gradient of the graph of is negative.
-
19M.2.SL.TZ1.T_6d:
Determine the equation of the normal at in the form .
-
18M.1.SL.TZ1.T_5a:
Write down the coordinates of C, the midpoint of line segment AB.
-
18M.1.SL.TZ1.T_5b:
Find the gradient of the line DC.
-
18M.1.SL.TZ1.T_5c:
Find the equation of the line DC. Write your answer in the form ax + by + d = 0 where a , b and d are integers.
-
16N.1.SL.TZ0.T_14a:
Find .
-
16N.1.SL.TZ0.T_14b:
Find the coordinates of P.
-
19N.1.SL.TZ0.T_14a:
Write down the value of .
-
19N.1.SL.TZ0.T_14b:
Find .
-
19N.1.SL.TZ0.T_14c:
At the point where , the gradient of the tangent to the curve is .
Find the value of .
-
19N.2.SL.TZ0.T_4a:
Find the value of .
-
19N.2.SL.TZ0.T_4b:
Write down the equation for the axis of symmetry of the graph.
-
19N.2.SL.TZ0.T_4c:
Use the symmetry of the graph to show that the second solution is .
-
19N.2.SL.TZ0.T_4d:
Write down the -intercepts of the graph.
-
19N.2.SL.TZ0.T_4e:
On graph paper, draw the graph of for and . Use a scale of to represent unit on the -axis and to represent units on the -axis.
-
19N.2.SL.TZ0.T_4f.i:
Write down the equation of .
-
19N.2.SL.TZ0.T_4f.ii:
Draw the tangent on your graph.
-
19N.2.SL.TZ0.T_4g:
Given and , state whether the function, , is increasing or decreasing at . Give a reason for your answer.
-
20N.1.SL.TZ0.S_10a.i:
Find in terms of and .
-
20N.1.SL.TZ0.S_10a.ii:
Show that the equation of is .
-
20N.1.SL.TZ0.S_10b:
Find the area of triangle in terms of .
-
20N.1.SL.TZ0.S_10c:
The graph of is translated by to give the graph of .
In the following diagram:- point lies on the graph of
- points , and lie on the vertical asymptote of
- points and lie on the horizontal asymptote of
- point lies on the -axis such that is parallel to .
Line is the tangent to the graph of at , and passes through and .
Given that triangle and rectangle have equal areas, find the gradient of in terms of .
-
20N.1.SL.TZ0.T_13a:
Write down .
-
20N.1.SL.TZ0.T_13b:
Write down the gradient of this tangent.
-
20N.1.SL.TZ0.T_13c:
Find the value of .
-
EXN.1.SL.TZ0.7a:
Find an expression for .
-
EXN.1.SL.TZ0.7b:
Show that the normal to the curve at the point where is .
-
22M.1.SL.TZ1.9b:
Find the equation of the normal to the curve at in the form , where .
-
22M.1.SL.TZ2.11a:
Find .
-
22M.1.SL.TZ2.11b:
Use your answer to part (a) to find the gradient of .
-
22M.2.AHL.TZ1.6a.ii:
Hence show that the equation of the tangent to the curve at the point is .
SL 5.5—Integration introduction, areas between curve and x axis
-
SPM.1.SL.TZ0.10a:
Write down an integral for the area of region R.
-
SPM.1.SL.TZ0.10b:
Find the area of region R.
-
SPM.1.SL.TZ0.10c:
The three points A(0, 0) , B(3, 10) and C(, 0) define the vertices of a triangle.
Find the value of , the -coordinate of C, such that the area of the triangle is equal to the area of region R.
-
16N.2.SL.TZ0.T_6b:
Express this volume in .
-
18M.3.AHL.TZ0.Hca_3a:
Find the value of .
-
18M.3.AHL.TZ0.Hca_3b:
Illustrate graphically the inequality .
-
18M.3.AHL.TZ0.Hca_3c:
Hence write down a lower bound for .
-
18M.3.AHL.TZ0.Hca_3d:
Find an upper bound for .
-
16N.2.SL.TZ0.S_6a:
Use the model to find the volume of the barrel.
-
16N.2.SL.TZ0.S_6b:
The empty barrel is being filled with water. The volume of water in the barrel after minutes is given by . How long will it take for the barrel to be half-full?
-
17M.2.SL.TZ2.S_8a:
Find the value of .
-
17M.2.SL.TZ2.S_8b.i:
Write down the coordinates of A.
-
17M.2.SL.TZ2.S_8b.ii:
Write down the rate of change of at A.
-
17M.2.SL.TZ2.S_8c.i:
Find the coordinates of B.
-
17M.2.SL.TZ2.S_8c.ii:
Find the the rate of change of at B.
-
17M.2.SL.TZ2.S_8d:
Let be the region enclosed by the graph of , the -axis, the line and the line . The region is rotated 360° about the -axis. Find the volume of the solid formed.
-
18N.2.SL.TZ0.S_10a:
Find the volume of the container.
-
18N.2.SL.TZ0.S_10b.i:
Find the value of and of .
-
18N.2.SL.TZ0.S_10b.ii:
During the interval < < , he volume of water in the container increases by m3. Find the value of .
-
18N.2.SL.TZ0.S_10c:
When = 0, the volume of water in the container is 2.3 m3. It is known that the container is never completely full of water during the 4 hour period.
Find the minimum volume of empty space in the container during the 4 hour period.
-
18M.1.SL.TZ2.S_2a:
Find .
-
18M.1.SL.TZ2.S_2b:
Find the area of the region enclosed by the graph of , the x-axis and the lines x = 1 and x = 2 .
-
18M.1.SL.TZ1.S_5a:
Find .
-
18M.1.SL.TZ1.S_5b:
Part of the graph of f is shown in the following diagram.
The shaded region R is enclosed by the graph of f, the x-axis, and the lines x = 1 and x = 9 . Find the volume of the solid formed when R is revolved 360° about the x-axis.
-
18M.2.SL.TZ1.S_4a:
Write down the coordinates of the vertex of the graph of g.
-
18M.2.SL.TZ1.S_4b:
On the grid above, sketch the graph of g for −2 ≤ x ≤ 4.
-
18M.2.SL.TZ1.S_4c:
Find the area of the region enclosed by the graphs of f and g.
-
18M.2.SL.TZ2.S_3a:
Find the x-intercept of the graph of .
-
18M.2.SL.TZ2.S_3b:
The region enclosed by the graph of , the y-axis and the x-axis is rotated 360° about the x-axis.
Find the volume of the solid formed.
-
17N.2.SL.TZ0.S_5a:
Find the value of .
-
17N.2.SL.TZ0.S_5b:
The following diagram shows part of the graph of .

The region enclosed by the graph of , the -axis and the lines and is rotated 360° about the -axis. Find the volume of the solid formed.
-
19M.2.SL.TZ2.S_2a:
Find the -intercept of the graph of .
-
19M.2.SL.TZ2.S_2b:
The region enclosed by the graph of , the -axis and the -axis is rotated 360º about the -axis. Find the volume of the solid formed.
-
18M.1.SL.TZ2.T_7c:
Two girls are selected at random.
Calculate the probability that one girl answered questions in Mandarin and the other answered questions in Hindi.
-
18M.2.SL.TZ2.T_1a.i:
Write down the value of a.
-
18M.2.SL.TZ2.T_1a.ii:
Write down the value of b.
-
18M.2.SL.TZ2.T_1b.i:
Use the tree diagram to find the probability that an employee encountered traffic and was late for work.
-
17N.2.SL.TZ0.T_4a:
Find the probability that this person is not allergic to nuts.
-
17N.2.SL.TZ0.T_4b:
Find the probability that both people chosen are not allergic to nuts.
-
17N.2.SL.TZ0.T_4c:
Copy and complete the tree diagram.
-
17N.2.SL.TZ0.T_4d:
Find the probability that this adult is allergic to nuts and the liquid turns blue.
-
17N.2.SL.TZ0.T_4e:
Find the probability that the liquid turns blue.
-
17N.2.SL.TZ0.T_4f:
Find the probability that the tested adult is allergic to nuts given that the liquid turned blue.
-
17N.2.SL.TZ0.T_4g:
Estimate the number of employees, from this 38, who are allergic to nuts.
-
19M.2.SL.TZ2.T_1a:
Write down the null hypothesis, H0 , for this test.
-
19M.2.SL.TZ2.T_1b:
State the number of degrees of freedom.
-
19M.2.SL.TZ2.T_1c.i:
the expected frequency of female students who chose to take the Chinese class.
-
19M.2.SL.TZ2.T_1d:
State whether or not H0 should be rejected. Justify your statement.
-
19M.2.SL.TZ2.T_1e.i:
Find the probability that the student does not take the Spanish class.
-
19M.2.SL.TZ2.T_1e.ii:
Find the probability that neither of the two students take the Spanish class.
-
19M.2.SL.TZ2.T_1e.iii:
Find the probability that at least one of the two students is female.
-
19M.1.SL.TZ1.T_12a:
Find the probability that both spins are yellow.
-
19M.1.SL.TZ1.T_12b:
Find the probability that at least one of the spins is yellow.
-
19M.1.SL.TZ1.T_12c:
Write down the probability that the second spin is yellow, given that the first spin is blue.
-
EXN.1.AHL.TZ0.10a.i:
Find .
-
EXN.1.AHL.TZ0.10a.ii:
State in context what this value represents.
-
EXN.1.AHL.TZ0.10b:
Find an expression for in terms of .
-
EXN.1.AHL.TZ0.10c:
Determine and state what it represents.
-
EXN.2.SL.TZ0.3a:
Find an expression for the height of the ball at time .
-
EXN.2.SL.TZ0.3b.i:
Find the value of at which the ball hits the ground.
-
EXN.2.SL.TZ0.3b.ii:
Hence write down the domain of .
-
EXN.2.SL.TZ0.3c:
Find the range of .
-
EXN.2.SL.TZ0.6g:
Use the expression found in (f) to calculate a value for .
-
21M.1.SL.TZ1.13a:
Determine whether the graph of against is increasing or decreasing at .
-
21M.1.SL.TZ1.13b:
Sieun observes that when the angle is , the ball will travel a horizontal distance of .
Find an expression for the function .
-
21M.2.SL.TZ1.5a.i:
Find .
-
21M.2.SL.TZ1.5c.i:
Write down the integral which can be used to find the cross-sectional area of the tunnel.
-
21M.2.SL.TZ1.5c.ii:
Hence find the cross-sectional area of the tunnel.
-
21M.2.AHL.TZ1.2d.i:
Write down the integral which can be used to find the cross-sectional area of the tunnel.
-
21M.2.AHL.TZ1.2d.ii:
Hence find the cross-sectional area of the tunnel.
-
21M.1.SL.TZ2.13a:
Find an expression for in terms of .
-
21M.1.SL.TZ2.13b:
The company regularly increases the number of cars it produces.
Describe how their profit changes if they increase production to over cars per month and up to cars per month. Justify your answer.
-
21N.1.SL.TZ0.13a.i:
Write down the value of .
-
21N.1.SL.TZ0.13a.ii:
Hence form two equations in terms of and .
-
21N.1.SL.TZ0.13a.iii:
Hence find the equation of the quadratic curve.
-
21N.1.SL.TZ0.13b:
Find the area of the shaded region in Irina’s design.
-
22M.1.SL.TZ1.6b:
Find the exact area of the shaded region in the painting.
-
22M.1.SL.TZ1.6c:
Find the area of the unshaded region in the painting.
-
22M.1.SL.TZ1.12b:
One year after the company was founded, the profit was thousand dollars.
Find an expression for , when .
-
22M.1.SL.TZ2.6a:
Calculate the area of the shaded region in diagram 1.
-
22M.1.SL.TZ2.6b.i:
Write down an integral for the area of the shaded region in diagram 2.
-
22M.1.SL.TZ2.6b.ii:
Calculate the area of this region.
-
22M.1.SL.TZ2.6c:
Hence, determine the area enclosed between and .
-
22M.2.AHL.TZ1.2d:
The goat is tied in the field for hours.
Find the total volume of grass eaten by the goat during this time.
-
22M.2.AHL.TZ1.6d.i:
Find the area enclosed by , the -axis and the line .
-
22M.2.AHL.TZ1.6d.ii:
Find the area of the shaded region on the diagram.
SL 5.6—Stationary points, local max and min
-
SPM.2.SL.TZ0.4a:
Calculate the surface area of the box in cm2.
-
SPM.2.SL.TZ0.4b:
Calculate the length AG.
-
SPM.2.SL.TZ0.4c:
Find the number of boxes that should be sold each week to maximize the profit.
-
SPM.2.SL.TZ0.4d:
Find .
-
SPM.2.SL.TZ0.4e:
Find the least number of boxes which must be sold each week in order to make a profit.
-
19M.1.AHL.TZ2.H_8a:
Show that the volume of the cone may be expressed by .
-
19M.1.AHL.TZ2.H_8b:
Given that there is one inscribed cone having a maximum volume, show that the volume of this cone is .
-
18M.1.AHL.TZ1.H_9a:
The graph of has a local maximum at A. Find the coordinates of A.
-
18M.1.AHL.TZ1.H_9b.i:
Show that there is exactly one point of inflexion, B, on the graph of .
-
18M.1.AHL.TZ1.H_9b.ii:
The coordinates of B can be expressed in the form B where a, b. Find the value of a and the value of b.
-
18M.1.AHL.TZ1.H_9c:
Sketch the graph of showing clearly the position of the points A and B.
-
17M.1.AHL.TZ2.H_10a.i:
Find the area of the window in terms of P and .
-
17M.1.AHL.TZ2.H_10a.ii:
Find the width of the window in terms of P when the area is a maximum, justifying that this is a maximum.
-
17M.1.AHL.TZ2.H_10b:
Show that in this case the height of the rectangle is equal to the radius of the semicircle.
-
18M.1.AHL.TZ2.H_4:
Consider the curve .
Find the x-coordinates of the points on the curve where the gradient is zero.
-
18M.2.AHL.TZ1.H_9a:
Show that there are exactly two points on the curve where the gradient is zero.
-
18M.2.AHL.TZ1.H_9b:
Find the equation of the normal to the curve at the point P.
-
18M.2.AHL.TZ1.H_9c:
The normal at P cuts the curve again at the point Q. Find the -coordinate of Q.
-
18M.2.AHL.TZ1.H_9d:
The shaded region is rotated by 2 about the -axis. Find the volume of the solid formed.
-
19M.1.AHL.TZ1.H_8a:
Write down the -coordinate of the point of inflexion on the graph of .
-
19M.1.AHL.TZ1.H_8b:
find the value of .
-
19M.1.AHL.TZ1.H_8c:
find the value of .
-
19M.1.AHL.TZ1.H_8d:
Sketch the curve , 0 ≤ ≤ 5 indicating clearly the coordinates of the maximum and minimum points and any intercepts with the coordinate axes.
-
16N.2.SL.TZ0.T_6a:
Write down a formula for , the surface area to be coated.
-
16N.2.SL.TZ0.T_6c:
Write down, in terms of and , an equation for the volume of this water container.
-
16N.2.SL.TZ0.T_6d:
Show that .
-
16N.2.SL.TZ0.T_6e:
Find .
-
16N.2.SL.TZ0.T_6f:
Using your answer to part (e), find the value of which minimizes .
-
16N.2.SL.TZ0.T_6g:
Find the value of this minimum area.
-
16N.2.SL.TZ0.T_6h:
Find the least number of cans of water-resistant material that will coat the area in part (g).
-
16N.2.SL.TZ0.S_10a:
(i) Find the value of .
(ii) Show that .
(iii) Find the value of .
-
16N.2.SL.TZ0.S_10b:
(i) Write down the value of .
(ii) Find .
-
16N.2.SL.TZ0.S_10c:
(i) Find .
(ii) Hence or otherwise, find the maximum positive rate of change of .
-
17M.1.SL.TZ1.S_6a.i:
Write down the gradient of the curve of at P.
-
17M.1.SL.TZ1.S_6a.ii:
Find the equation of the normal to the curve of at P.
-
17M.1.SL.TZ1.S_6b:
Determine the concavity of the graph of when and justify your answer.
-
17M.2.SL.TZ2.S_8a:
Find the value of .
-
17M.2.SL.TZ2.S_8b.i:
Write down the coordinates of A.
-
17M.2.SL.TZ2.S_8b.ii:
Write down the rate of change of at A.
-
17M.2.SL.TZ2.S_8c.i:
Find the coordinates of B.
-
17M.2.SL.TZ2.S_8c.ii:
Find the the rate of change of at B.
-
17M.2.SL.TZ2.S_8d:
Let be the region enclosed by the graph of , the -axis, the line and the line . The region is rotated 360° about the -axis. Find the volume of the solid formed.
-
18N.1.SL.TZ0.S_10a:
Find the coordinates of P.
-
18N.1.SL.TZ0.S_10b.i:
Find .
-
18N.1.SL.TZ0.S_10b.ii:
Hence, find the equation of L in terms of .
-
18N.1.SL.TZ0.S_10c:
The graph of has a local minimum at the point Q. The line L passes through Q.
Find the value of .
-
18N.2.SL.TZ0.S_10a:
Find the volume of the container.
-
18N.2.SL.TZ0.S_10b.i:
Find the value of and of .
-
18N.2.SL.TZ0.S_10b.ii:
During the interval < < , he volume of water in the container increases by m3. Find the value of .
-
18N.2.SL.TZ0.S_10c:
When = 0, the volume of water in the container is 2.3 m3. It is known that the container is never completely full of water during the 4 hour period.
Find the minimum volume of empty space in the container during the 4 hour period.
-
18M.1.SL.TZ2.S_9a:
Express h in terms of r.
-
18M.1.SL.TZ2.S_9b:
Show that .
-
18M.1.SL.TZ2.S_9c:
Given that there is a minimum value for C, find this minimum value in terms of .
-
17N.1.SL.TZ0.S_7:
Consider , for , where .
The equation has exactly one solution. Find the value of .
-
19M.2.SL.TZ1.S_4a:
Sketch the graph of on the grid below:
-
19M.2.SL.TZ1.S_4b:
Find the -coordinates of the points of inflexion of the graph of .
-
19M.2.SL.TZ1.S_4c:
Hence find the values of for which the graph of is concave-down.
-
19M.2.SL.TZ2.S_5a:
Find the population of fish at = 10.
-
19M.2.SL.TZ2.S_5b:
Find the rate at which the population of fish is increasing at = 10.
-
19M.2.SL.TZ2.S_5c:
Find the value of for which the population of fish is increasing most rapidly.
-
18M.2.SL.TZ1.T_4a:
Find the value of k.
-
18M.2.SL.TZ1.T_4b:
Using your value of k , find f ′(x).
-
18M.2.SL.TZ1.T_4c:
Use your answer to part (b) to show that the minimum value of f(x) is −22 .
-
18M.2.SL.TZ1.T_4e:
Sketch the graph of y = f (x) for 0 < x ≤ 6 and −30 ≤ y ≤ 60.
Clearly indicate the minimum point P and the x-intercepts on your graph. -
18M.2.SL.TZ2.T_6a:
Sketch the curve for −1 < x < 3 and −2 < y < 12.
-
18M.2.SL.TZ2.T_6b:
A teacher asks her students to make some observations about the curve.
Three students responded.
Nadia said “The x-intercept of the curve is between −1 and zero”.
Rick said “The curve is decreasing when x < 1 ”.
Paula said “The gradient of the curve is less than zero between x = 1 and x = 2 ”.State the name of the student who made an incorrect observation.
-
18M.2.SL.TZ2.T_6d:
Find .
-
18M.2.SL.TZ2.T_6f:
Given that y = 2x3 − 9x2 + 12x + 2 = k has three solutions, find the possible values of k.
-
19M.2.SL.TZ2.T_5d:
Find .
-
19M.2.SL.TZ2.T_5e:
Find the gradient of the graph of at .
-
19M.2.SL.TZ2.T_5f:
Find the equation of the tangent line to the graph of at . Give the equation in the form where, , , and .
-
17N.1.SL.TZ0.T_11c:
Find the value of and of .
-
17M.2.SL.TZ1.T_6a:
Find .
-
17M.2.SL.TZ1.T_6b.i:
Show that .
-
17M.2.SL.TZ1.T_6b.ii:
Find the equation of the tangent to the graph of at . Give your answer in the form .
-
17M.2.SL.TZ1.T_6c:
Use your answer to part (a) and the value of , to find the -coordinates of the stationary points of the graph of .
-
17M.2.SL.TZ1.T_6d.i:
Find .
-
17M.2.SL.TZ1.T_6d.ii:
Hence justify that is decreasing at .
-
17M.2.SL.TZ1.T_6e:
Find the -coordinate of the local minimum.
-
18M.1.SL.TZ2.T_13a:
Find the cost of producing 70 shirts.
-
18M.1.SL.TZ2.T_13b:
Find the value of s.
-
18M.1.SL.TZ2.T_13c:
Find the number of shirts produced when the cost of production is lowest.
-
17M.2.SL.TZ2.T_6a:
Write down the -intercept of the graph.
-
17M.2.SL.TZ2.T_6b:
Find .
-
17M.2.SL.TZ2.T_6c.i:
Show that .
-
17M.2.SL.TZ2.T_6c.ii:
Find .
-
17M.2.SL.TZ2.T_6d.i:
Write down the -coordinates of these two points;
-
17M.2.SL.TZ2.T_6d.ii:
Write down the intervals where the gradient of the graph of is positive.
-
17M.2.SL.TZ2.T_6e:
Write down the range of .
-
17M.2.SL.TZ2.T_6f:
Write down the number of possible solutions to the equation .
-
17M.2.SL.TZ2.T_6g:
The equation , where , has four solutions. Find the possible values of .
-
19M.1.SL.TZ2.T_15a:
Find the value of if no vases are sold.
-
19M.1.SL.TZ2.T_15b:
Differentiate .
-
19M.2.SL.TZ1.T_6a:
Show that .
-
19M.2.SL.TZ1.T_6b:
Find the coordinates of the local minimum.
-
19M.2.SL.TZ1.T_6c:
Write down the interval where the gradient of the graph of is negative.
-
19M.2.SL.TZ1.T_6d:
Determine the equation of the normal at in the form .
-
18N.2.SL.TZ0.T_6a:
Calculate the area of cloth, in cm2, needed to make Haruka’s bag.
-
18N.2.SL.TZ0.T_6b:
Calculate the volume, in cm3, of the bag.
-
18N.2.SL.TZ0.T_6c:
Use this value to write down, and simplify, the equation in x and y for the volume of Nanako’s bag.
-
18N.2.SL.TZ0.T_6d:
Write down and simplify an expression in x and y for the area of cloth, A, used to make Nanako’s bag.
-
18N.2.SL.TZ0.T_6e:
Use your answers to parts (c) and (d) to show that
.
-
18N.2.SL.TZ0.T_6f:
Find .
-
18N.2.SL.TZ0.T_6g:
Use your answer to part (f) to show that the width of Nanako’s bag is 12 cm.
-
18N.2.SL.TZ0.T_6h:
The cloth used to make Nanako’s bag costs 4 Japanese Yen (JPY) per cm2.
Find the cost of the cloth used to make Nanako’s bag.
-
19N.2.SL.TZ0.T_6a:
Write down an expression for , the volume (cm3) of the speaker, in terms of , and .
-
19N.2.SL.TZ0.T_6b:
Write down an equation for the surface area of the speaker in terms of , and .
-
19N.2.SL.TZ0.T_6c:
Given the design constraint that , show that .
-
19N.2.SL.TZ0.T_6d:
Find .
-
19N.2.SL.TZ0.T_6e:
Using your answer to part (d), show that is a maximum when is equal to .
-
19N.2.SL.TZ0.T_6f:
Find the length of the cylinder for which is a maximum.
-
19N.2.SL.TZ0.T_6g:
Calculate the maximum value of .
-
19N.2.SL.TZ0.T_6h:
Use your answer to part (f) to identify the shape of the speaker with the best quality of sound.
-
EXN.1.AHL.TZ0.15a:
Find .
-
EXN.1.AHL.TZ0.15b:
Find in terms of the value of at which the maximum occurs.
-
EXN.1.AHL.TZ0.15c:
Hence find the value of for which has the smallest possible maximum value.
-
EXN.2.SL.TZ0.2a:
Find an expression for the total length of the ribbon in terms of and .
-
EXN.2.SL.TZ0.2b:
Show that
-
EXN.2.SL.TZ0.2c:
Find
-
EXN.2.SL.TZ0.2d:
Solve
-
EXN.2.SL.TZ0.2e:
Hence or otherwise find the minimum length of ribbon required.
-
EXN.2.SL.TZ0.6d:
Use differentiation to show that .
-
21M.2.SL.TZ1.5a.ii:
Hence find the maximum height of the tunnel.
-
21M.2.AHL.TZ1.2a.ii:
Hence find the maximum height of the tunnel.
-
21M.2.SL.TZ2.5f:
Hence, or otherwise, find the maximum possible volume of the box.
-
21M.2.SL.TZ2.5g:
The box will contain spherical chocolates. The production manager assumes that they can calculate the exact number of chocolates in each box by dividing the volume of the box by the volume of a single chocolate and then rounding down to the nearest integer.
Explain why the production manager is incorrect.
-
21N.1.SL.TZ0.12a:
Find .
-
21N.1.SL.TZ0.12b.i:
Solve .
-
21N.1.SL.TZ0.12b.ii:
Interpret your answer to (b)(i) in context.
-
21N.1.AHL.TZ0.8a.i:
Identify the value of the point where has its maximum value.
-
21N.1.AHL.TZ0.8a.ii:
Interpret this point in the given context.
-
21N.1.AHL.TZ0.8b:
Juri starts at a height of metres and finishes at , where .
Sketch a possible diagram of the hill on the following pair of coordinate axes.
-
21N.1.AHL.TZ0.15a:
Show that .
-
21N.1.AHL.TZ0.15b.i:
Find an expression for in terms of .
-
21N.1.AHL.TZ0.15b.ii:
Find the expression .
-
21N.1.AHL.TZ0.15b.iii:
Solve algebraically to find the value of that will maximize the volume, .
-
22M.1.AHL.TZ1.17:
A function is of the form . Part of the graph of is shown.
The points and have coordinates and , and lie on .
The point is a local maximum and the point is a local minimum.
Find the value of , of and of .
-
22M.2.SL.TZ1.4c:
Find the value of at which the goat is eating grass at the greatest rate.
-
22M.2.AHL.TZ1.2c:
Find the value of at which the goat is eating grass at the greatest rate.
-
22M.2.SL.TZ2.5b:
Hence find the maximum value of in terms of . Give your answer in the form , where is a constant.
-
22M.2.AHL.TZ2.6b:
Find the maximum height reached by the ball.
SL 5.7—Optimisation
-
SPM.2.SL.TZ0.4a:
Calculate the surface area of the box in cm2.
-
SPM.2.SL.TZ0.4b:
Calculate the length AG.
-
SPM.2.SL.TZ0.4c:
Find the number of boxes that should be sold each week to maximize the profit.
-
SPM.2.SL.TZ0.4d:
Find .
-
SPM.2.SL.TZ0.4e:
Find the least number of boxes which must be sold each week in order to make a profit.
-
19M.1.AHL.TZ2.H_8a:
Show that the volume of the cone may be expressed by .
-
19M.1.AHL.TZ2.H_8b:
Given that there is one inscribed cone having a maximum volume, show that the volume of this cone is .
-
18M.1.AHL.TZ1.H_9a:
The graph of has a local maximum at A. Find the coordinates of A.
-
18M.1.AHL.TZ1.H_9b.i:
Show that there is exactly one point of inflexion, B, on the graph of .
-
18M.1.AHL.TZ1.H_9b.ii:
The coordinates of B can be expressed in the form B where a, b. Find the value of a and the value of b.
-
18M.1.AHL.TZ1.H_9c:
Sketch the graph of showing clearly the position of the points A and B.
-
17M.1.AHL.TZ2.H_10a.i:
Find the area of the window in terms of P and .
-
17M.1.AHL.TZ2.H_10a.ii:
Find the width of the window in terms of P when the area is a maximum, justifying that this is a maximum.
-
17M.1.AHL.TZ2.H_10b:
Show that in this case the height of the rectangle is equal to the radius of the semicircle.
-
18M.1.AHL.TZ2.H_4:
Consider the curve .
Find the x-coordinates of the points on the curve where the gradient is zero.
-
18M.2.AHL.TZ1.H_9a:
Show that there are exactly two points on the curve where the gradient is zero.
-
18M.2.AHL.TZ1.H_9b:
Find the equation of the normal to the curve at the point P.
-
18M.2.AHL.TZ1.H_9c:
The normal at P cuts the curve again at the point Q. Find the -coordinate of Q.
-
18M.2.AHL.TZ1.H_9d:
The shaded region is rotated by 2 about the -axis. Find the volume of the solid formed.
-
19M.1.AHL.TZ1.H_8a:
Write down the -coordinate of the point of inflexion on the graph of .
-
19M.1.AHL.TZ1.H_8b:
find the value of .
-
19M.1.AHL.TZ1.H_8c:
find the value of .
-
19M.1.AHL.TZ1.H_8d:
Sketch the curve , 0 ≤ ≤ 5 indicating clearly the coordinates of the maximum and minimum points and any intercepts with the coordinate axes.
-
16N.2.SL.TZ0.T_6a:
Write down a formula for , the surface area to be coated.
-
16N.2.SL.TZ0.T_6b:
Express this volume in .
-
16N.2.SL.TZ0.T_6c:
Write down, in terms of and , an equation for the volume of this water container.
-
16N.2.SL.TZ0.T_6d:
Show that .
-
16N.2.SL.TZ0.T_6e:
Find .
-
16N.2.SL.TZ0.T_6f:
Using your answer to part (e), find the value of which minimizes .
-
16N.2.SL.TZ0.T_6g:
Find the value of this minimum area.
-
16N.2.SL.TZ0.T_6h:
Find the least number of cans of water-resistant material that will coat the area in part (g).
-
17N.1.SL.TZ0.S_6:
Let , for . The following diagram shows part of the graph of and the rectangle OABC, where A is on the negative -axis, B is on the graph of , and C is on the -axis.

Find the -coordinate of A that gives the maximum area of OABC.
-
16N.2.SL.TZ0.S_10a:
(i) Find the value of .
(ii) Show that .
(iii) Find the value of .
-
16N.2.SL.TZ0.S_10b:
(i) Write down the value of .
(ii) Find .
-
16N.2.SL.TZ0.S_10c:
(i) Find .
(ii) Hence or otherwise, find the maximum positive rate of change of .
-
17M.1.SL.TZ1.S_6a.i:
Write down the gradient of the curve of at P.
-
17M.1.SL.TZ1.S_6a.ii:
Find the equation of the normal to the curve of at P.
-
17M.1.SL.TZ1.S_6b:
Determine the concavity of the graph of when and justify your answer.
-
17M.2.SL.TZ2.S_8a:
Find the value of .
-
17M.2.SL.TZ2.S_8b.i:
Write down the coordinates of A.
-
17M.2.SL.TZ2.S_8b.ii:
Write down the rate of change of at A.
-
17M.2.SL.TZ2.S_8c.i:
Find the coordinates of B.
-
17M.2.SL.TZ2.S_8c.ii:
Find the the rate of change of at B.
-
17M.2.SL.TZ2.S_8d:
Let be the region enclosed by the graph of , the -axis, the line and the line . The region is rotated 360° about the -axis. Find the volume of the solid formed.
-
18N.1.SL.TZ0.S_10a:
Find the coordinates of P.
-
18N.1.SL.TZ0.S_10b.i:
Find .
-
18N.1.SL.TZ0.S_10b.ii:
Hence, find the equation of L in terms of .
-
18N.1.SL.TZ0.S_10c:
The graph of has a local minimum at the point Q. The line L passes through Q.
Find the value of .
-
18N.2.SL.TZ0.S_10a:
Find the volume of the container.
-
18N.2.SL.TZ0.S_10b.i:
Find the value of and of .
-
18N.2.SL.TZ0.S_10b.ii:
During the interval < < , he volume of water in the container increases by m3. Find the value of .
-
18N.2.SL.TZ0.S_10c:
When = 0, the volume of water in the container is 2.3 m3. It is known that the container is never completely full of water during the 4 hour period.
Find the minimum volume of empty space in the container during the 4 hour period.
-
19M.1.SL.TZ1.S_8a:
Find the -intercepts of the graph of .
-
19M.1.SL.TZ1.S_8b:
Show that the area of PQRS is .
-
19M.1.SL.TZ1.S_8c:
Hence find the value of such that the area of PQRS is a maximum.
-
19M.1.SL.TZ1.S_8d:
Show that when the graphs of and intersect, .
-
19M.1.SL.TZ1.S_8e:
Given that the graphs of and intersect only once, find the value of .
-
18M.1.SL.TZ2.S_9a:
Express h in terms of r.
-
18M.1.SL.TZ2.S_9b:
Show that .
-
18M.1.SL.TZ2.S_9c:
Given that there is a minimum value for C, find this minimum value in terms of .
-
17N.1.SL.TZ0.S_7:
Consider , for , where .
The equation has exactly one solution. Find the value of .
-
19M.2.SL.TZ1.S_4a:
Sketch the graph of on the grid below:
-
19M.2.SL.TZ1.S_4b:
Find the -coordinates of the points of inflexion of the graph of .
-
19M.2.SL.TZ1.S_4c:
Hence find the values of for which the graph of is concave-down.
-
19M.2.SL.TZ2.S_5a:
Find the population of fish at = 10.
-
19M.2.SL.TZ2.S_5b:
Find the rate at which the population of fish is increasing at = 10.
-
19M.2.SL.TZ2.S_5c:
Find the value of for which the population of fish is increasing most rapidly.
-
19M.1.SL.TZ1.T_15a:
Write down an equation for the area, , of the curved surface in terms of .
-
19M.1.SL.TZ1.T_15b:
Find .
-
19M.1.SL.TZ1.T_15c:
Find the value of when the area of the curved surface is maximized.
-
18M.1.SL.TZ2.T_13a:
Find the cost of producing 70 shirts.
-
18M.1.SL.TZ2.T_13b:
Find the value of s.
-
18M.1.SL.TZ2.T_13c:
Find the number of shirts produced when the cost of production is lowest.
-
17N.1.SL.TZ0.T_15a:
Write down how many kilograms of cheese Maria sells in one week if the price of a kilogram of cheese is 8 EUR.
-
17N.1.SL.TZ0.T_15b:
Find how much Maria earns in one week, from selling cheese, if the price of a kilogram of cheese is 8 EUR.
-
17N.1.SL.TZ0.T_15c:
Write down an expression for in terms of .
-
17N.1.SL.TZ0.T_15d:
Find the price, , that will give Maria the highest weekly profit.
-
19M.1.SL.TZ2.T_15a:
Find the value of if no vases are sold.
-
19M.1.SL.TZ2.T_15b:
Differentiate .
-
18N.2.SL.TZ0.T_6a:
Calculate the area of cloth, in cm2, needed to make Haruka’s bag.
-
18N.2.SL.TZ0.T_6b:
Calculate the volume, in cm3, of the bag.
-
18N.2.SL.TZ0.T_6c:
Use this value to write down, and simplify, the equation in x and y for the volume of Nanako’s bag.
-
18N.2.SL.TZ0.T_6d:
Write down and simplify an expression in x and y for the area of cloth, A, used to make Nanako’s bag.
-
18N.2.SL.TZ0.T_6e:
Use your answers to parts (c) and (d) to show that
.
-
18N.2.SL.TZ0.T_6f:
Find .
-
18N.2.SL.TZ0.T_6g:
Use your answer to part (f) to show that the width of Nanako’s bag is 12 cm.
-
18N.2.SL.TZ0.T_6h:
The cloth used to make Nanako’s bag costs 4 Japanese Yen (JPY) per cm2.
Find the cost of the cloth used to make Nanako’s bag.
-
19N.2.SL.TZ0.T_6a:
Write down an expression for , the volume (cm3) of the speaker, in terms of , and .
-
19N.2.SL.TZ0.T_6b:
Write down an equation for the surface area of the speaker in terms of , and .
-
19N.2.SL.TZ0.T_6c:
Given the design constraint that , show that .
-
19N.2.SL.TZ0.T_6d:
Find .
-
19N.2.SL.TZ0.T_6e:
Using your answer to part (d), show that is a maximum when is equal to .
-
19N.2.SL.TZ0.T_6f:
Find the length of the cylinder for which is a maximum.
-
19N.2.SL.TZ0.T_6g:
Calculate the maximum value of .
-
19N.2.SL.TZ0.T_6h:
Use your answer to part (f) to identify the shape of the speaker with the best quality of sound.
-
20N.2.SL.TZ0.T_4a:
Write down an equation in and that shows this information.
-
20N.2.SL.TZ0.T_4b:
Show that .
-
20N.2.SL.TZ0.T_4c:
Find .
-
20N.2.SL.TZ0.T_4d:
Using your answer to part (c), find the value of for which is a maximum.
-
20N.2.SL.TZ0.T_4e:
Find the maximum volume of the bird bath.
-
20N.2.SL.TZ0.T_4f:
To prevent leaks, a sealant is applied to the interior surface of the bird bath.
Find the surface area to be covered by the sealant, given that the bird bath has maximum volume.
-
EXN.2.SL.TZ0.2a:
Find an expression for the total length of the ribbon in terms of and .
-
EXN.2.SL.TZ0.2b:
Show that
-
EXN.2.SL.TZ0.2c:
Find
-
EXN.2.SL.TZ0.2d:
Solve
-
EXN.2.SL.TZ0.2e:
Hence or otherwise find the minimum length of ribbon required.
-
21M.2.SL.TZ1.5a.ii:
Hence find the maximum height of the tunnel.
-
21M.2.AHL.TZ1.2a.ii:
Hence find the maximum height of the tunnel.
-
21M.1.SL.TZ2.13a:
Find an expression for in terms of .
-
21M.1.SL.TZ2.13b:
The company regularly increases the number of cars it produces.
Describe how their profit changes if they increase production to over cars per month and up to cars per month. Justify your answer.
-
21N.1.SL.TZ0.12a:
Find .
-
21N.1.SL.TZ0.12b.i:
Solve .
-
21N.1.SL.TZ0.12b.ii:
Interpret your answer to (b)(i) in context.
-
21N.1.AHL.TZ0.15a:
Show that .
-
21N.1.AHL.TZ0.15b.i:
Find an expression for in terms of .
-
21N.1.AHL.TZ0.15b.ii:
Find the expression .
-
21N.1.AHL.TZ0.15b.iii:
Solve algebraically to find the value of that will maximize the volume, .
-
22M.2.SL.TZ1.4c:
Find the value of at which the goat is eating grass at the greatest rate.
-
22M.2.AHL.TZ1.2c:
Find the value of at which the goat is eating grass at the greatest rate.
-
22M.2.SL.TZ2.5c.ii:
Use the model to find how much coffee the cafe should make each morning to maximize its profit.
SL 5.8—Trapezoid rule
-
EXM.2.AHL.TZ0.12a:
Use the trapezoidal rule to find an estimate for the area.
-
EXM.2.AHL.TZ0.12b.i:
Use all the coordinates in the table to find the equation of the least squares cubic regression curve.
-
EXM.2.AHL.TZ0.12b.ii:
Write down the coefficient of determination.
-
EXM.2.AHL.TZ0.12c.i:
Write down an expression for the area enclosed by the cubic regression curve, the -axis, the -axis and the line .
-
EXM.2.AHL.TZ0.12c.ii:
Find the value of this area.
-
EXM.3.AHL.TZ0.7a.i:
Use the trapezoidal rule to find an estimate for the area.
-
EXM.3.AHL.TZ0.7a.ii:
With reference to the shape of the graph, explain whether your answer to part (a)(i) will be an over-estimate or an underestimate of the area.
-
EXM.3.AHL.TZ0.7b.i:
Use all the coordinates in the table to find the equation of the least squares cubic regression curve.
-
EXM.3.AHL.TZ0.7b.ii:
Write down the coefficient of determination.
-
EXM.3.AHL.TZ0.7c.i:
Write down an expression for the area enclosed by the cubic function, the -axis, the -axis and the line .
-
EXM.3.AHL.TZ0.7c.ii:
Find the value of this area.
-
EXM.3.AHL.TZ0.7d.i:
Show that .
-
EXM.3.AHL.TZ0.7d.ii:
Hence explain how a straight line graph could be drawn using the coordinates in the table.
-
EXM.3.AHL.TZ0.7d.iii:
By finding the equation of a suitable regression line, show that and .
-
EXM.3.AHL.TZ0.7d.iv:
Hence find the area enclosed by the exponential function, the -axis, the -axis and the line .
-
EXN.2.SL.TZ0.6b:
By dividing the area between the curve and the ‐axis into two trapezoids of unequal width show that , justifying the direction of the inequality.
-
21M.2.SL.TZ1.5b:
Use the trapezoidal rule, with three intervals, to estimate the cross-sectional area of the tunnel.
-
21M.2.AHL.TZ1.2c:
Use the trapezoidal rule, with three intervals, to estimate the cross-sectional area of the tunnel.
-
21N.1.SL.TZ0.6a:
Use the trapezoidal rule with an interval width of to estimate the total amount of carbon dioxide emitted during these two hours.
-
21N.1.SL.TZ0.6b:
The real amount of carbon dioxide emitted during these two hours was tonnes.
Find the percentage error of the estimate found in part (a).
-
22M.1.SL.TZ1.6a:
Use the trapezoidal rule, with the values given in the following table, to approximate the area of the shaded region.
-
22M.1.AHL.TZ2.14:
The shape of a vase is formed by rotating a curve about the -axis.
The vase is high. The internal radius of the vase is measured at intervals along the height:
Use the trapezoidal rule to estimate the volume of water that the vase can hold.
AHL 5.9—Differentiating standard functions and derivative rules
-
SPM.1.AHL.TZ0.7a:
Write down an expression for as a function of time.
-
SPM.1.AHL.TZ0.7b:
Hence find .
-
SPM.1.AHL.TZ0.7c:
Hence or otherwise find the first time at which the kinetic energy is changing at a rate of 5 J s−1.
-
18N.2.AHL.TZ0.H_9a:
Find .
-
18N.2.AHL.TZ0.H_9b:
Hence, or otherwise, find the coordinates of the point of inflexion on the graph of .
-
18N.2.AHL.TZ0.H_9c.i:
sketch the graph of , showing clearly any axis intercepts and giving the equations of any asymptotes.
-
18N.2.AHL.TZ0.H_9c.ii:
sketch the graph of , showing clearly any axis intercepts and giving the equations of any asymptotes.
-
18N.2.AHL.TZ0.H_9d:
Hence, or otherwise, solve the inequality .
-
17M.2.AHL.TZ1.H_12a:
Find the largest possible domain for to be a function.
-
17M.2.AHL.TZ1.H_12b:
Sketch the graph of showing clearly the equations of asymptotes and the coordinates of any intercepts with the axes.
-
17M.2.AHL.TZ1.H_12c:
Explain why is an even function.
-
17M.2.AHL.TZ1.H_12d:
Explain why the inverse function does not exist.
-
17M.2.AHL.TZ1.H_12e:
Find the inverse function and state its domain.
-
17M.2.AHL.TZ1.H_12f:
Find .
-
17M.2.AHL.TZ1.H_12g.i:
Hence, show that there are no solutions to ;
-
17M.2.AHL.TZ1.H_12g.ii:
Hence, show that there are no solutions to .
-
17M.2.AHL.TZ1.H_8a:
Find an expression for the volume of water in the trough in terms of .
-
17M.2.AHL.TZ1.H_8b:
Calculate when .
-
19M.2.AHL.TZ1.H_1:
Let be the tangent to the curve at the point (1, ).
Find the coordinates of the point where meets the -axis.
-
18M.1.AHL.TZ1.H_2a:
Find
-
18M.1.AHL.TZ1.H_2b:
Hence find the values of θ for which .
-
19M.1.SL.TZ2.S_9a:
Find the value of .
-
19M.1.SL.TZ2.S_9b:
Line passes through the origin and has a gradient of . Find the equation of .
-
19M.1.SL.TZ2.S_9c:
Find the derivative of .
-
19M.1.SL.TZ2.S_9d:
The following diagram shows the graph of for 0 ≤ ≤ 3. Line is a tangent to the graph of at point P.
Given that is parallel to , find the -coordinate of P.
-
19M.2.SL.TZ1.S_3a:
Find .
-
19M.2.SL.TZ1.S_3b:
The graph of has a horizontal tangent line at and at . Find .
-
17M.1.SL.TZ2.S_6a:
Find .
-
17M.1.SL.TZ2.S_6b:
Find .
-
16N.1.SL.TZ0.S_10a:
(i) Find the first four derivatives of .
(ii) Find .
-
16N.1.SL.TZ0.S_10b:
(i) Find the first three derivatives of .
(ii) Given that , find .
-
16N.1.SL.TZ0.S_10c:
(i) Find .
(ii) Hence, show that .
-
20N.2.SL.TZ0.S_10a:
Show that .
-
20N.2.SL.TZ0.S_10b:
Find the least value of .
-
20N.2.SL.TZ0.S_10c:
Find .
-
20N.2.SL.TZ0.S_10d:
Let be the region enclosed by the graph of , the -axis and the lines and . The area of is , correct to three significant figures.
Find .
-
EXN.1.AHL.TZ0.15a:
Find .
-
EXN.1.AHL.TZ0.15b:
Find in terms of the value of at which the maximum occurs.
-
EXN.1.AHL.TZ0.15c:
Hence find the value of for which has the smallest possible maximum value.
-
EXN.2.AHL.TZ0.4a:
Show that Jorge’s model satisfies the differential equation
-
21N.1.AHL.TZ0.15a:
Show that .
-
21N.1.AHL.TZ0.15b.i:
Find an expression for in terms of .
-
21N.1.AHL.TZ0.15b.ii:
Find the expression .
-
21N.1.AHL.TZ0.15b.iii:
Solve algebraically to find the value of that will maximize the volume, .
-
21N.3.AHL.TZ0.2a.i:
Find the equation of the regression line of on .
-
21N.3.AHL.TZ0.2a.ii:
Interpret the meaning of parameter in the context of the model.
-
21N.3.AHL.TZ0.2a.iii:
Suggest why Eva’s use of the linear regression equation in this way could be unreliable.
-
21N.3.AHL.TZ0.2b.i:
Find the equation of the least squares quadratic regression curve.
-
21N.3.AHL.TZ0.2b.ii:
Find the value of .
-
21N.3.AHL.TZ0.2b.iii:
Hence, write down a suitable domain for Eva’s function .
-
21N.3.AHL.TZ0.2c:
Show that .
-
21N.3.AHL.TZ0.2d:
By solving the differential equation , show that the general solution is given by , where .
-
21N.3.AHL.TZ0.2e:
Use the general solution from part (d) and the initial condition to predict the value of .
-
21N.3.AHL.TZ0.2f:
Find this new height.
-
21N.3.AHL.TZ0.2g.i:
Show that , where .
-
21N.3.AHL.TZ0.2g.ii:
Use Euler’s method with a step length of minutes to estimate the maximum value of .
-
22M.1.AHL.TZ1.8a.i:
Find .
-
22M.1.AHL.TZ1.16a:
Find an expression for .
-
22M.1.AHL.TZ1.16b:
When Frieda arrives at the top of a hill, the speed of the wind is kilometres per hour and increasing at a rate of .
Find the rate of change of at this time.
-
22M.1.AHL.TZ1.17:
A function is of the form . Part of the graph of is shown.
The points and have coordinates and , and lie on .
The point is a local maximum and the point is a local minimum.
Find the value of , of and of .
-
22M.1.AHL.TZ2.17a:
When has an -coordinate equal to , find the horizontal component of the velocity of .
-
22M.1.AHL.TZ2.17b.i:
Find the time taken for the snail to reach the point .
-
22M.1.AHL.TZ2.17b.ii:
Hence show that the snail reaches the point before the water does.
-
22M.2.AHL.TZ1.6a.i:
Find .
-
22M.2.AHL.TZ2.4a:
Show that is always positive.
AHL 5.10—Second derivatives, testing for max and min
-
19M.1.AHL.TZ1.H_5:
A camera at point C is 3 m from the edge of a straight section of road as shown in the following diagram. The camera detects a car travelling along the road at = 0. It then rotates, always pointing at the car, until the car passes O, the point on the edge of the road closest to the camera.
A car travels along the road at a speed of 24 ms−1. Let the position of the car be X and let OĈX = θ.
Find , the rate of rotation of the camera, in radians per second, at the instant the car passes the point O .
-
16N.1.SL.TZ0.S_10a:
(i) Find the first four derivatives of .
(ii) Find .
-
16N.1.SL.TZ0.S_10b:
(i) Find the first three derivatives of .
(ii) Given that , find .
-
16N.1.SL.TZ0.S_10c:
(i) Find .
(ii) Hence, show that .
-
20N.1.SL.TZ0.S_7a:
Find an expression for the velocity of at time .
-
20N.1.SL.TZ0.S_7b:
Particle also moves in a straight line. The position of is given by .
The speed of is greater than the speed of when .
Find the value of .
-
20N.2.SL.TZ0.S_10a:
Show that .
-
20N.2.SL.TZ0.S_10b:
Find the least value of .
-
20N.2.SL.TZ0.S_10c:
Find .
-
20N.2.SL.TZ0.S_10d:
Let be the region enclosed by the graph of , the -axis and the lines and . The area of is , correct to three significant figures.
Find .
-
22M.1.AHL.TZ1.8a.ii:
Find .
-
22M.1.AHL.TZ1.8b:
The curve has a point of inflexion at .
Find the value of .
AHL 5.11—Indefinite integration, reverse chain, by substitution
-
19M.1.AHL.TZ1.H_8a:
Write down the -coordinate of the point of inflexion on the graph of .
-
19M.1.AHL.TZ1.H_8b:
find the value of .
-
19M.1.AHL.TZ1.H_8c:
find the value of .
-
19M.1.AHL.TZ1.H_8d:
Sketch the curve , 0 ≤ ≤ 5 indicating clearly the coordinates of the maximum and minimum points and any intercepts with the coordinate axes.
-
18M.1.AHL.TZ2.H_6a.i:
Find .
-
18M.1.AHL.TZ2.H_6a.ii:
Find .
-
18M.1.AHL.TZ2.H_6b:
Hence, or otherwise, find .
-
18N.2.AHL.TZ0.H_2:
A function satisfies the conditions , and its second derivative is , ≥ 0.
Find .
-
18M.1.AHL.TZ1.H_4a:
.
-
18M.1.AHL.TZ1.H_4b:
.
-
19M.1.SL.TZ2.S_10a:
Find .
-
19M.1.SL.TZ2.S_10b:
Hence find .
-
19M.1.SL.TZ2.S_10c:
Write down an expression for the area of .
-
19M.1.SL.TZ2.S_10d:
Hence find the exact area of .
-
18M.1.SL.TZ2.S_2a:
Find .
-
18M.1.SL.TZ2.S_2b:
Find the area of the region enclosed by the graph of , the x-axis and the lines x = 1 and x = 2 .
-
17M.1.SL.TZ1.S_5a:
Find .
-
17M.1.SL.TZ1.S_5b:
Find , given that and .
-
17M.1.SL.TZ2.S_5:
Let . Given that , find .
-
18M.1.SL.TZ1.S_5a:
Find .
-
18M.1.SL.TZ1.S_5b:
Part of the graph of f is shown in the following diagram.
The shaded region R is enclosed by the graph of f, the x-axis, and the lines x = 1 and x = 9 . Find the volume of the solid formed when R is revolved 360° about the x-axis.
-
18N.1.SL.TZ0.S_6:
Let . The following diagram shows part of the graph of .
The region R is enclosed by the graph of , the -axis, and the -axis. Find the area of R.
-
16N.1.SL.TZ0.S_6:
Let . Find , given that .
-
19M.1.SL.TZ1.S_5:
The derivative of a function is given by . The graph of passes through .
Find .
-
20N.1.SL.TZ0.S_6:
The graph of a function passes through the point .
Given that , find .
-
20N.2.SL.TZ0.S_10a:
Show that .
-
20N.2.SL.TZ0.S_10b:
Find the least value of .
-
20N.2.SL.TZ0.S_10c:
Find .
-
20N.2.SL.TZ0.S_10d:
Let be the region enclosed by the graph of , the -axis and the lines and . The area of is , correct to three significant figures.
Find .
-
EXN.1.AHL.TZ0.10a.i:
Find .
-
EXN.1.AHL.TZ0.10a.ii:
State in context what this value represents.
-
EXN.1.AHL.TZ0.10b:
Find an expression for in terms of .
-
EXN.1.AHL.TZ0.10c:
Determine and state what it represents.
-
22M.1.AHL.TZ1.14a.i:
Expand .
-
22M.1.AHL.TZ1.14a.ii:
Find .
AHL 5.12—Areas under a curve onto x or y axis. Volumes of revolution about x and y
-
SPM.1.AHL.TZ0.14a.i:
Write down the value of .
-
SPM.1.AHL.TZ0.14a.ii:
Find the value of .
-
SPM.1.AHL.TZ0.14b:
The outer dome of a large cathedral has the shape of a hemisphere of diameter 32 m, supported by vertical walls of height 17 m. It is also supported by an inner dome which can be modelled by rotating the curve through 360° about the -axis between = 0 and = 33, as indicated in the diagram.
Find the volume of the space between the two domes.
-
18M.2.AHL.TZ1.H_9a:
Show that there are exactly two points on the curve where the gradient is zero.
-
18M.2.AHL.TZ1.H_9b:
Find the equation of the normal to the curve at the point P.
-
18M.2.AHL.TZ1.H_9c:
The normal at P cuts the curve again at the point Q. Find the -coordinate of Q.
-
18M.2.AHL.TZ1.H_9d:
The shaded region is rotated by 2 about the -axis. Find the volume of the solid formed.
-
18M.1.AHL.TZ2.H_11a:
Show that where .
-
18M.1.AHL.TZ2.H_11b:
Express in terms of . Give your answer in the form , where p , q are constants.
-
18M.1.AHL.TZ2.H_11c:
The region R, is bounded by the graph of the function found in part (b), the x-axis, and the lines and where . The area of R is .
Find the value of .
-
17N.2.AHL.TZ0.H_10a.i:
Show that the -coordinate of the minimum point on the curve satisfies the equation .
-
17N.2.AHL.TZ0.H_10a.ii:
Determine the values of for which is a decreasing function.
-
17N.2.AHL.TZ0.H_10b:
Sketch the graph of showing clearly the minimum point and any asymptotic behaviour.
-
17N.2.AHL.TZ0.H_10c:
Find the coordinates of the point on the graph of where the normal to the graph is parallel to the line .
-
17N.2.AHL.TZ0.H_10d:
This region is now rotated through radians about the -axis. Find the volume of revolution.
-
17M.1.AHL.TZ1.H_11a.i:
Express in the form .
-
17M.1.AHL.TZ1.H_11a.ii:
Factorize .
-
17M.1.AHL.TZ1.H_11b:
Sketch the graph of , indicating on it the equations of the asymptotes, the coordinates of the -intercept and the local maximum.
-
17M.1.AHL.TZ1.H_11c:
Show that .
-
17M.1.AHL.TZ1.H_11d:
Hence find the value of if .
-
17M.1.AHL.TZ1.H_11e:
Sketch the graph of .
-
17M.1.AHL.TZ1.H_11f:
Determine the area of the region enclosed between the graph of , the -axis and the lines with equations and .
-
19M.2.AHL.TZ1.H_10a:
Write down the maximum and minimum value of .
-
19M.2.AHL.TZ1.H_10b:
Write down two transformations that will transform the graph of onto the graph of .
-
19M.2.AHL.TZ1.H_10c:
Sketch the graph of for 0 ≤ ≤ 0.02 , showing clearly the coordinates of the first maximum and the first minimum.
-
19M.2.AHL.TZ1.H_10d:
Find the total time in the interval 0 ≤ ≤ 0.02 for which ≥ 3.
-
19M.2.AHL.TZ1.H_10e:
Find (0.007).
-
19M.2.AHL.TZ1.H_10f:
With reference to your graph of explain why > 0 for all > 0.
-
19M.2.AHL.TZ1.H_10g:
Given that can be written as where , , , > 0, use your graph to find the values of , , and .
-
17M.2.AHL.TZ1.H_4a:
Write down a definite integral to represent the area of .
-
17M.2.AHL.TZ1.H_4b:
Calculate the area of .
-
19M.2.AHL.TZ1.H_7:
The function is defined by , ≥ 1 and the function is defined by , ≥ 0.
The region is bounded by the curves , and the lines , and as shown on the following diagram.
The shape of a clay vase can be modelled by rotating the region through 360˚ about the -axis.
Find the volume of clay used to make the vase.
-
16N.2.SL.TZ0.S_6a:
Use the model to find the volume of the barrel.
-
16N.2.SL.TZ0.S_6b:
The empty barrel is being filled with water. The volume of water in the barrel after minutes is given by . How long will it take for the barrel to be half-full?
-
17M.2.SL.TZ2.S_8a:
Find the value of .
-
17M.2.SL.TZ2.S_8b.i:
Write down the coordinates of A.
-
17M.2.SL.TZ2.S_8b.ii:
Write down the rate of change of at A.
-
17M.2.SL.TZ2.S_8c.i:
Find the coordinates of B.
-
17M.2.SL.TZ2.S_8c.ii:
Find the the rate of change of at B.
-
17M.2.SL.TZ2.S_8d:
Let be the region enclosed by the graph of , the -axis, the line and the line . The region is rotated 360° about the -axis. Find the volume of the solid formed.
-
18N.2.SL.TZ0.S_10a:
Find the volume of the container.
-
18N.2.SL.TZ0.S_10b.i:
Find the value of and of .
-
18N.2.SL.TZ0.S_10b.ii:
During the interval < < , he volume of water in the container increases by m3. Find the value of .
-
18N.2.SL.TZ0.S_10c:
When = 0, the volume of water in the container is 2.3 m3. It is known that the container is never completely full of water during the 4 hour period.
Find the minimum volume of empty space in the container during the 4 hour period.
-
18M.1.SL.TZ2.S_2a:
Find .
-
18M.1.SL.TZ2.S_2b:
Find the area of the region enclosed by the graph of , the x-axis and the lines x = 1 and x = 2 .
-
18M.1.SL.TZ1.S_5a:
Find .
-
18M.1.SL.TZ1.S_5b:
Part of the graph of f is shown in the following diagram.
The shaded region R is enclosed by the graph of f, the x-axis, and the lines x = 1 and x = 9 . Find the volume of the solid formed when R is revolved 360° about the x-axis.
-
18M.2.SL.TZ1.S_4a:
Write down the coordinates of the vertex of the graph of g.
-
18M.2.SL.TZ1.S_4b:
On the grid above, sketch the graph of g for −2 ≤ x ≤ 4.
-
18M.2.SL.TZ1.S_4c:
Find the area of the region enclosed by the graphs of f and g.
-
18M.2.SL.TZ2.S_3a:
Find the x-intercept of the graph of .
-
18M.2.SL.TZ2.S_3b:
The region enclosed by the graph of , the y-axis and the x-axis is rotated 360° about the x-axis.
Find the volume of the solid formed.
-
17N.2.SL.TZ0.S_5a:
Find the value of .
-
17N.2.SL.TZ0.S_5b:
The following diagram shows part of the graph of .

The region enclosed by the graph of , the -axis and the lines and is rotated 360° about the -axis. Find the volume of the solid formed.
-
19M.2.SL.TZ2.S_2a:
Find the -intercept of the graph of .
-
19M.2.SL.TZ2.S_2b:
The region enclosed by the graph of , the -axis and the -axis is rotated 360º about the -axis. Find the volume of the solid formed.
-
20N.1.SL.TZ0.S_3a:
Find the value of .
-
20N.1.SL.TZ0.S_3b:
Find the volume of the solid formed when the shaded region is revolved about the -axis.
-
21M.2.AHL.TZ1.4d.i:
Write down an expression for her estimate of the volume as a sum of two integrals.
-
21M.2.AHL.TZ1.4d.ii:
Find the value of Charlotte’s estimate.
-
21N.1.AHL.TZ0.17a:
Show that the volume of water, , in terms of is .
-
21N.1.AHL.TZ0.17b:
Hence find the maximum capacity of the bowl in .
-
22M.1.AHL.TZ1.14b:
Find the volume of the solid formed. Give your answer in the form , where .
-
22M.1.AHL.TZ2.14:
The shape of a vase is formed by rotating a curve about the -axis.
The vase is high. The internal radius of the vase is measured at intervals along the height:
Use the trapezoidal rule to estimate the volume of water that the vase can hold.
-
22M.2.AHL.TZ1.6d.i:
Find the area enclosed by , the -axis and the line .
-
22M.2.AHL.TZ1.6d.ii:
Find the area of the shaded region on the diagram.
AHL 5.13—Kinematic problems
-
17N.1.AHL.TZ0.H_5:
A particle moves in a straight line such that at time seconds , its velocity , in , is given by . Find the exact distance travelled by the particle in the first half-second.
-
18M.2.AHL.TZ2.H_7a:
Determine the first time t1 at which P has zero velocity.
-
18M.2.AHL.TZ2.H_7b.i:
Find an expression for the acceleration of P at time t.
-
18M.2.AHL.TZ2.H_7b.ii:
Find the value of the acceleration of P at time t1.
-
17M.1.AHL.TZ2.H_4a:
Find and .
-
17M.1.AHL.TZ2.H_4b:
Find the displacement of the particle when
-
17M.2.AHL.TZ1.H_11a:
Find his velocity when .
-
17M.2.AHL.TZ1.H_11b:
Calculate the vertical distance Xavier travelled in the first 10 seconds.
-
17M.2.AHL.TZ1.H_11c:
Determine the value of .
-
19M.2.AHL.TZ2.H_6:
A particle moves along a horizontal line such that at time seconds, ≥ 0, its acceleration is given by = 2 − 1. When = 6 , its displacement from a fixed origin O is 18.25 m. When = 15, its displacement from O is 922.75 m. Find an expression for in terms of .
-
18M.2.SL.TZ2.S_9a:
Find the initial velocity of P.
-
18M.2.SL.TZ2.S_9b:
Find the maximum speed of P.
-
18M.2.SL.TZ2.S_9c:
Write down the number of times that the acceleration of P is 0 m s−2 .
-
18M.2.SL.TZ2.S_9d:
Find the acceleration of P when it changes direction.
-
18M.2.SL.TZ2.S_9e:
Find the total distance travelled by P.
-
17M.2.SL.TZ1.S_7a.i:
Write down the first value of at which P changes direction.
-
17M.2.SL.TZ1.S_7a.ii:
Find the total distance travelled by P, for .
-
17M.2.SL.TZ1.S_7b:
A second particle Q also moves along a straight line. Its velocity, after seconds is given by for . After seconds Q has travelled the same total distance as P.
Find .
-
19M.2.SL.TZ2.S_8a:
Find the initial displacement of particle A from point P.
-
19M.2.SL.TZ2.S_8b:
Find the value of when particle A first reaches point P.
-
19M.2.SL.TZ2.S_8c:
Find the value of when particle A first changes direction.
-
19M.2.SL.TZ2.S_8d:
Find the total distance travelled by particle A in the first 3 seconds.
-
19M.2.SL.TZ2.S_8e.i:
Given that particles A and B start at the same point, find the displacement function for particle B.
-
19M.2.SL.TZ2.S_8e.ii:
Find the other value of when particles A and B meet.
-
17N.2.SL.TZ0.S_9a:
Write down the values of when .
-
17N.2.SL.TZ0.S_9b:
Hence or otherwise, find all possible values of for which the velocity of P is decreasing.
-
17N.2.SL.TZ0.S_9c:
Find an expression for the velocity of P at time .
-
17N.2.SL.TZ0.S_9d:
Find the total distance travelled by P when its velocity is increasing.
-
17M.2.SL.TZ2.S_7:
Note: In this question, distance is in metres and time is in seconds.
A particle moves along a horizontal line starting at a fixed point A. The velocity of the particle, at time , is given by , for . The following diagram shows the graph of

There are -intercepts at and .
Find the maximum distance of the particle from A during the time and justify your answer.
-
16N.2.SL.TZ0.S_9a:
Find the initial velocity of .
-
16N.2.SL.TZ0.S_9b:
Find the value of .
-
16N.2.SL.TZ0.S_9c:
(i) Find the value of .
(ii) Hence, find the speed of P when .
-
16N.2.SL.TZ0.S_9d:
(i) Find the total distance travelled by P between and .
(ii) Hence or otherwise, find the displacement of P from A when .
-
19M.1.SL.TZ1.S_7a:
Find the value of .
-
19M.1.SL.TZ1.S_7b:
Find the total distance travelled in the first 5 seconds.
-
18N.2.SL.TZ0.S_4a:
Find when the particle is at rest.
-
18N.2.SL.TZ0.S_4b:
Find the acceleration of the particle when .
-
18N.2.SL.TZ0.S_4c:
Find the total distance travelled by the particle.
-
EXN.2.AHL.TZ0.7a:
Show that the ball is moving in a circle with its centre at and state the radius of the circle.
-
EXN.2.AHL.TZ0.7b.i:
Find an expression for the velocity of the ball at time .
-
EXN.2.AHL.TZ0.7b.ii:
Hence show that the velocity of the ball is always perpendicular to the position vector of the ball.
-
EXN.2.AHL.TZ0.7c.i:
Find an expression for the acceleration of the ball at time .
-
EXN.2.AHL.TZ0.7c.ii:
Find the value of at the instant the string breaks.
-
EXN.2.AHL.TZ0.7c.iii:
How many complete revolutions has the ball completed from to the instant at which the string breaks?
-
21M.2.AHL.TZ1.6a:
Find the velocity vector at time .
-
21M.2.AHL.TZ1.6b:
Show that the magnitude of the velocity of the ice-skater at time is given by
.
-
21M.2.AHL.TZ1.6c:
Find the value of and the value of .
-
21M.2.AHL.TZ1.6d:
Find the magnitude of the velocity of the ice-skater when .
-
21M.2.AHL.TZ1.6e:
At a point , the ice-skater is skating parallel to the -axis for the first time.
Find .
-
21M.2.AHL.TZ2.6a:
Find the times when is at instantaneous rest.
-
21M.2.AHL.TZ2.6b:
Find the magnitude of the particle’s acceleration at seconds.
-
21M.2.AHL.TZ2.6c:
Find the greatest speed of in the interval .
-
21M.2.AHL.TZ2.6d:
The particle starts from the origin . Find an expression for the displacement of from at time seconds.
-
21M.2.AHL.TZ2.6e:
Find the total distance travelled by in the interval .
-
21N.1.AHL.TZ0.8a.i:
Identify the value of the point where has its maximum value.
-
21N.1.AHL.TZ0.8a.ii:
Interpret this point in the given context.
-
21N.1.AHL.TZ0.8b:
Juri starts at a height of metres and finishes at , where .
Sketch a possible diagram of the hill on the following pair of coordinate axes.
-
22M.1.AHL.TZ2.16a:
Find the velocity vector of .
-
22M.1.AHL.TZ2.16b:
Show that the acceleration vector of is never parallel to the position vector of .
-
22M.1.AHL.TZ2.17a:
When has an -coordinate equal to , find the horizontal component of the velocity of .
-
22M.1.AHL.TZ2.17b.i:
Find the time taken for the snail to reach the point .
-
22M.1.AHL.TZ2.17b.ii:
Hence show that the snail reaches the point before the water does.
-
22M.2.AHL.TZ2.6a.i:
Find the initial speed of the ball.
-
22M.2.AHL.TZ2.6a.ii:
Find the angle of elevation of the ball as it is launched.
AHL 5.14—Setting up a DE, solve by separating variables
-
SPM.2.AHL.TZ0.7a:
By substituting into the equation, find an expression for the velocity of the particle at time . Give your answer in the form .
-
SPM.2.AHL.TZ0.7b:
From your solution to part (a), or otherwise, find the terminal velocity of the object predicted by this model.
-
SPM.2.AHL.TZ0.7c:
Write down the differential equation as a system of first order differential equations.
-
SPM.2.AHL.TZ0.7d:
Use Euler’s method, with a step length of 0.2, to find the displacement and velocity of the object when .
-
SPM.2.AHL.TZ0.7e:
By repeated application of Euler’s method, find an approximation for the terminal velocity, to five significant figures.
-
SPM.2.AHL.TZ0.7f:
Use the differential equation to find the terminal velocity for the object.
-
SPM.2.AHL.TZ0.7g:
Use your answers to parts (d), (e) and (f) to comment on the accuracy of the Euler approximation to this model.
-
16N.2.AHL.TZ0.H_6:
An earth satellite moves in a path that can be described by the curve where and are in thousands of kilometres and is time in seconds.
Given that when , find the possible values of .
Give your answers in standard form.
-
21M.1.AHL.TZ1.12a:
Show that .
-
21M.1.AHL.TZ1.12b:
Find the time taken for the tank to empty.
-
21M.2.AHL.TZ1.7a:
Find the population of rabbits year after they were introduced.
-
21M.3.AHL.TZ2.2d.i:
Write down the differential equation for that models this situation.
-
21M.3.AHL.TZ2.2d.ii:
Show that the expression for the mackerel population density after years is
-
21M.3.AHL.TZ2.2d.iii:
Alessia estimates that the mackerel population density increases by a factor of three every two years. Show that to three significant figures.
-
21N.3.AHL.TZ0.2a.i:
Find the equation of the regression line of on .
-
21N.3.AHL.TZ0.2a.ii:
Interpret the meaning of parameter in the context of the model.
-
21N.3.AHL.TZ0.2a.iii:
Suggest why Eva’s use of the linear regression equation in this way could be unreliable.
-
21N.3.AHL.TZ0.2b.i:
Find the equation of the least squares quadratic regression curve.
-
21N.3.AHL.TZ0.2b.ii:
Find the value of .
-
21N.3.AHL.TZ0.2b.iii:
Hence, write down a suitable domain for Eva’s function .
-
21N.3.AHL.TZ0.2c:
Show that .
-
21N.3.AHL.TZ0.2d:
By solving the differential equation , show that the general solution is given by , where .
-
21N.3.AHL.TZ0.2e:
Use the general solution from part (d) and the initial condition to predict the value of .
-
21N.3.AHL.TZ0.2f:
Find this new height.
-
21N.3.AHL.TZ0.2g.i:
Show that , where .
-
21N.3.AHL.TZ0.2g.ii:
Use Euler’s method with a step length of minutes to estimate the maximum value of .
-
22M.3.AHL.TZ1.1b.i:
Find the general solution of the differential equation .
AHL 5.15—Slope fields
-
21M.1.AHL.TZ1.15a:
Find the equation of , giving your answer in the form .
-
21M.1.AHL.TZ1.15b:
For the two solutions given, the local maximum points lie on the straight line .
Find the equation of .
-
21N.1.AHL.TZ0.13a:
Calculate the value of at the point .
-
21N.1.AHL.TZ0.13b:
Sketch, on the first graph, a curve that represents the points where .
-
21N.1.AHL.TZ0.13c:
(i) sketch the solution curve that passes through the point .
(ii) sketch the solution curve that passes through the point .
-
22M.1.AHL.TZ1.7a.i:
Write down the equation of the curve on which all these maximum and minimum points lie.
-
22M.1.AHL.TZ1.7a.ii:
Sketch this curve on the slope field.
-
22M.1.AHL.TZ1.7b:
The solution to the differential equation that passes through the point has both a local maximum point and a local minimum point.
On the slope field, sketch the solution to the differential equation that passes through .
AHL 5.16—Eulers method for 1st order DEs
-
SPM.3.AHL.TZ0.2a.i:
Find the equilibrium population of brown squirrels suggested by this model.
-
SPM.3.AHL.TZ0.2a.ii:
Explain why the population of squirrels is increasing for values of less than this value.
-
SPM.3.AHL.TZ0.2b.i:
Verify that , is an equilibrium point.
-
SPM.3.AHL.TZ0.2b.ii:
Find the other three equilibrium points.
-
SPM.3.AHL.TZ0.2c.i:
By using separation of variables, show that the general solution of is .
-
SPM.3.AHL.TZ0.2c.ii:
Write down the general solution of .
-
SPM.3.AHL.TZ0.2c.iii:
If both populations contain 10 squirrels at use the solutions to parts (c) (i) and (ii) to estimate the number of black and brown squirrels when . Give your answers to the nearest whole numbers.
-
SPM.3.AHL.TZ0.2d.i:
Write down the expressions for and that the conservationists will use.
-
SPM.3.AHL.TZ0.2d.ii:
Given that the initial populations are , , find the populations of each species of squirrel when .
-
SPM.3.AHL.TZ0.2d.iii:
Use further iterations of Euler’s method to find the long-term population for each species of squirrel from these initial values.
-
SPM.3.AHL.TZ0.2d.iv:
Use the same method to find the long-term populations of squirrels when the initial populations are , .
-
SPM.3.AHL.TZ0.2e:
Use Euler’s method with step length 0.2 to sketch, on the same axes, the approximate trajectories for the populations with the following initial populations.
(i) ,
(ii) ,
-
SPM.3.AHL.TZ0.2f:
Given that the equilibrium point at (800, 600) is a saddle point, sketch the phase portrait for ≥ 0 , ≥ 0 on the same axes used in part (e).
-
16N.1.AHL.TZ0.H_11a:
Find an expression for .
-
16N.1.AHL.TZ0.H_11b:
Show that .
-
16N.1.AHL.TZ0.H_11c:
Show that the function has a local maximum value when .
-
16N.1.AHL.TZ0.H_11d:
Find the -coordinate of the point of inflexion of the graph of .
-
16N.1.AHL.TZ0.H_11e:
Sketch the graph of , clearly indicating the position of the local maximum point, the point of inflexion and the axes intercepts.
-
16N.1.AHL.TZ0.H_11f:
Find the area of the region enclosed by the graph of and the -axis.
-
16N.1.AHL.TZ0.H_11g:
Find the value of the curvature of the graph of at the local maximum point.
-
16N.1.AHL.TZ0.H_11h:
Find the value for and comment on its meaning with respect to the shape of the graph.
-
EXN.2.AHL.TZ0.4b:
Show that
-
EXN.2.AHL.TZ0.4c:
Hence find three approximations for the value of .
-
21M.2.AHL.TZ1.7b:
(i) the population of rabbits 1 year after the foxes were introduced.
(ii) the population of foxes 1 year after the foxes were introduced.
-
21M.2.AHL.TZ1.7c.i:
point .
-
21M.2.AHL.TZ1.7c.ii:
point .
-
21M.2.AHL.TZ1.7d:
Find the non-zero equilibrium point for the populations of rabbits and foxes.
-
21M.3.AHL.TZ2.2e.i:
Write down expressions for and in terms of and .
-
21M.3.AHL.TZ2.2e.ii:
Use Euler’s method to find an estimate for the mackerel population density after one year.
-
21N.3.AHL.TZ0.2a.i:
Find the equation of the regression line of on .
-
21N.3.AHL.TZ0.2a.ii:
Interpret the meaning of parameter in the context of the model.
-
21N.3.AHL.TZ0.2a.iii:
Suggest why Eva’s use of the linear regression equation in this way could be unreliable.
-
21N.3.AHL.TZ0.2b.i:
Find the equation of the least squares quadratic regression curve.
-
21N.3.AHL.TZ0.2b.ii:
Find the value of .
-
21N.3.AHL.TZ0.2b.iii:
Hence, write down a suitable domain for Eva’s function .
-
21N.3.AHL.TZ0.2c:
Show that .
-
21N.3.AHL.TZ0.2d:
By solving the differential equation , show that the general solution is given by , where .
-
21N.3.AHL.TZ0.2e:
Use the general solution from part (d) and the initial condition to predict the value of .
-
21N.3.AHL.TZ0.2f:
Find this new height.
-
21N.3.AHL.TZ0.2g.i:
Show that , where .
-
21N.3.AHL.TZ0.2g.ii:
Use Euler’s method with a step length of minutes to estimate the maximum value of .
AHL 5.17—Phase portrait
-
EXM.3.AHL.TZ0.1a:
Show that if the system has two distinct real eigenvalues then .
-
EXM.3.AHL.TZ0.1b.i:
Find two conditions that must be satisfied by , , , .
-
EXM.3.AHL.TZ0.1b.ii:
Explain why and must have opposite signs.
-
EXM.3.AHL.TZ0.1c:
In the case when there is a pair of purely imaginary eigenvalues you are told that the solution will form an ellipse. You are also told that the initial conditions are such that the ellipse is large enough that it will cross both the positive and negative axes and the positive and negative axes.
By considering the differential equations at these four crossing point investigate if the trajectory is in a clockwise or anticlockwise direction round the ellipse. Give your decision in terms of and . Using part (b) (ii) show that your conclusions are consistent.
-
EXM.2.AHL.TZ0.1a:
Use the eigenvalue method to find the general solution to this system of equations.
-
EXM.2.AHL.TZ0.1b.i:
Given the initial conditions that when , , , find the particular solution.
-
EXM.2.AHL.TZ0.1b.ii:
Hence find the solution when .
-
EXM.2.AHL.TZ0.1c:
As , find an asymptote to the trajectory of the particular solution found in (b)(i) and state if this trajectory will be moving towards or away from the origin.
-
SPM.1.AHL.TZ0.13a:
Find the value of when .
-
SPM.1.AHL.TZ0.13b:
On the following axes, sketch a possible trajectory for the growth of the two fungi, making clear any asymptotic behaviour.
-
EXM.3.AHL.TZ0.2a:
Show that the matrix has (sadly) only one eigenvalue. Find this eigenvalue and an associated eigenvector.
-
EXM.3.AHL.TZ0.2b:
Hence, verify that is a solution to the above system.
-
EXM.3.AHL.TZ0.2c:
Verify that is also a solution.
-
EXM.3.AHL.TZ0.2d:
If initially at find the particular solution.
-
EXM.3.AHL.TZ0.2e:
Find the values of and when .
-
EXM.3.AHL.TZ0.2f.i:
Find the equation of this asymptote.
-
EXM.3.AHL.TZ0.2f.ii:
State the direction of the trajectory, including the quadrant it is in as it approaches this asymptote.
-
EXM.3.AHL.TZ0.3a:
Find the equilibrium point for this system.
-
EXM.3.AHL.TZ0.3b:
If initially and use Euler’s method with an time increment of 0.1 to find an approximation for the values of and when .
-
EXM.3.AHL.TZ0.3c:
Extend this method to conjecture the limit of the ratio as .
-
EXM.3.AHL.TZ0.3d:
Show how using the substitution transforms the system of differential equations into .
-
EXM.3.AHL.TZ0.3e:
Solve this system of equations by the eigenvalue method and hence find the general solution for of the original system.
-
EXM.3.AHL.TZ0.3f:
Find the particular solution to the original system, given the initial conditions of part (b).
-
EXM.3.AHL.TZ0.3g:
Hence find the exact values of and when , giving the answers to 4 significant figures.
-
EXM.3.AHL.TZ0.3h:
Use part (f) to find limit of the ratio as .
-
EXM.3.AHL.TZ0.3i:
With the initial conditions as given in part (b) state if the equilibrium point is stable or unstable.
-
EXM.3.AHL.TZ0.3j:
If instead the initial conditions were given as and , find the particular solution for of the original system, in this case.
-
EXM.3.AHL.TZ0.3k:
With the initial conditions as given in part (j), determine if the equilibrium point is stable or unstable.
-
EXN.2.AHL.TZ0.5a.i:
the eigenvalues.
-
EXN.2.AHL.TZ0.5a.ii:
the eigenvectors.
-
EXN.2.AHL.TZ0.5b:
Hence write down the general solution of the system of equations.
-
EXN.2.AHL.TZ0.5c:
Sketch the phase portrait for this system, for .
On your sketch show
- the equation of the line defined by the eigenvector in the first quadrant
- at least two trajectories either side of this line using arrows on those trajectories to represent the change in populations as t increases
-
EXN.2.AHL.TZ0.5d:
Write down a condition on the size of the initial population of if it is to avoid its population reducing to zero.
-
EXN.2.AHL.TZ0.5e.i:
Find the value of at which .
-
EXN.2.AHL.TZ0.5e.ii:
Find the population of at this value of . Give your answer to the nearest herbivores.
-
21M.1.SL.TZ1.12a.i:
Find in terms of and .
-
21M.1.SL.TZ1.12a.ii:
Show that .
-
21M.1.SL.TZ1.12b:
Find .
-
21M.1.SL.TZ1.12c.i:
Write down an equation Ellis could solve to find this value of .
-
21M.1.SL.TZ1.12c.ii:
Hence, or otherwise, find this value of .
-
21M.2.AHL.TZ2.7a:
Find the eigenvalues and corresponding eigenvectors of the matrix .
-
21M.2.AHL.TZ2.7b:
Hence, write down the general solution of the system.
-
21M.2.AHL.TZ2.7c:
Determine, with justification, whether the equilibrium point is stable or unstable.
-
21M.2.AHL.TZ2.7d:
(i) at .
(ii) at .
-
21M.2.AHL.TZ2.7e:
Sketch a phase portrait for the general solution to the system of coupled differential equations for , .
-
21M.3.AHL.TZ2.2a.i:
-
21M.3.AHL.TZ2.2a.ii:
-
21M.3.AHL.TZ2.2b.i:
show that, at the equilibrium point, the value of the mackerel population density is ;
-
21M.3.AHL.TZ2.2b.ii:
find the value of the shark population density at the equilibrium point.
-
21M.3.AHL.TZ2.2c.i:
Toxic sewage is added to the Mediterranean Sea. Alessia claims this reduces the shark population growth rate and hence the value of is halved. No other parameter changes.
-
21M.3.AHL.TZ2.2c.ii:
Global warming increases the temperature of the Mediterranean Sea. Alessia claims that this promotes the mackerel population growth rate and hence the value of is doubled. No other parameter changes.
-
21M.3.AHL.TZ2.2f.i:
Use Euler’s method to sketch the trajectory of the phase portrait, for and , over the first nine years.
-
21M.3.AHL.TZ2.2f.ii:
Using your phase portrait, or otherwise, determine whether the mackerel population density would be sufficient to support sustainable fishing during the first nine years.
-
21M.3.AHL.TZ2.2f.iii:
State two reasons why Alessia’s conclusion, found in part (f)(ii), might not be valid.
-
21N.2.AHL.TZ0.6a:
Given that , show that .
-
21N.2.AHL.TZ0.6b:
Write down the matrix .
-
21N.2.AHL.TZ0.6c.i:
Find the eigenvalues of matrix .
-
21N.2.AHL.TZ0.6c.ii:
Find the eigenvectors of matrix .
-
21N.2.AHL.TZ0.6d:
Given that when the shock absorber is displaced and its velocity is zero, find an expression for in terms of .
-
22M.2.AHL.TZ1.4a.i:
Use the substitution to show that this equation can be written as
.
-
22M.2.AHL.TZ1.4a.ii:
Find the eigenvalues for the matrix .
-
22M.2.AHL.TZ1.4a.iii:
Hence state the long-term velocity of the particle.
-
22M.2.AHL.TZ2.7a:
Show that the system of coupled first order equations:
can be written as the given second order differential equation.
-
22M.2.AHL.TZ2.7c:
Hence find the exact solution of the second order differential equation.
AHL 5.18—Eulers method for 2nd order DEs
-
SPM.2.AHL.TZ0.7a:
By substituting into the equation, find an expression for the velocity of the particle at time . Give your answer in the form .
-
SPM.2.AHL.TZ0.7b:
From your solution to part (a), or otherwise, find the terminal velocity of the object predicted by this model.
-
SPM.2.AHL.TZ0.7c:
Write down the differential equation as a system of first order differential equations.
-
SPM.2.AHL.TZ0.7d:
Use Euler’s method, with a step length of 0.2, to find the displacement and velocity of the object when .
-
SPM.2.AHL.TZ0.7e:
By repeated application of Euler’s method, find an approximation for the terminal velocity, to five significant figures.
-
SPM.2.AHL.TZ0.7f:
Use the differential equation to find the terminal velocity for the object.
-
SPM.2.AHL.TZ0.7g:
Use your answers to parts (d), (e) and (f) to comment on the accuracy of the Euler approximation to this model.
-
EXN.1.AHL.TZ0.13a:
Write the differential equation as a system of coupled first order differential equations.
-
EXN.1.AHL.TZ0.13b:
When ,
Use Euler’s method with a step length of to find an estimate for the value of the displacement and velocity of the particle when .
-
22M.2.AHL.TZ1.4b.i:
Use the substitution to write the differential equation as a system of coupled, first order differential equations.
-
22M.2.AHL.TZ1.4b.ii:
Use Euler’s method with a step length of to find the displacement of the particle when .
-
22M.2.AHL.TZ1.4b.iii:
Find the long-term velocity of the particle.
