| Date | November 2017 | Marks available | 7 | Reference code | 17N.1.SL.TZ0.S_6 |
| Level | Standard Level | Paper | Paper 1 | Time zone | Time zone 0 |
| Command term | Find | Question number | S_6 | Adapted from | N/A |
Question
Let f(x)=15−x2, for x∈R. The following diagram shows part of the graph of f and the rectangle OABC, where A is on the negative x-axis, B is on the graph of f, and C is on the y-axis.
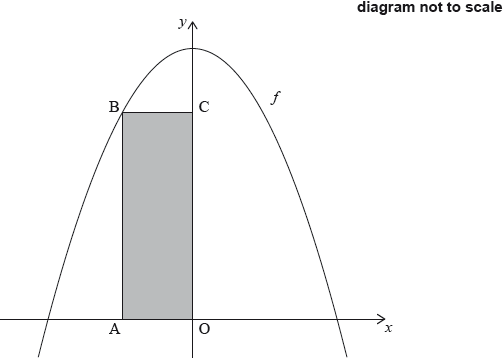
Find the x-coordinate of A that gives the maximum area of OABC.
Markscheme
* This question is from an exam for a previous syllabus, and may contain minor differences in marking or structure.
attempt to find the area of OABC (M1)
egOA×OC, x×f(x), f(x)×(−x)
correct expression for area in one variable (A1)
egarea=x(15−x2), 15x−x3, x3−15x
valid approach to find maximum area (seen anywhere) (M1)
egA′(x)=0
correct derivative A1
eg15−3x2, (15−x2)+x(−2x)=0, −15+3x2
correct working (A1)
eg15=3x2, x2=5, x=√5
x=−√5 (accept A(−√5, 0)) A2 N3
[7 marks]
Examiners report
Syllabus sections
-
18M.1.AHL.TZ1.H_9c:
Sketch the graph of y=f(x) showing clearly the position of the points A and B.
-
22M.2.SL.TZ2.5c.ii:
Use the model to find how much coffee the cafe should make each morning to maximize its profit.
-
17M.1.AHL.TZ2.H_10a.i:
Find the area of the window in terms of P and r.
-
18M.1.SL.TZ2.T_13b:
Find the value of s.
-
18N.2.SL.TZ0.T_6a:
Calculate the area of cloth, in cm2, needed to make Haruka’s bag.
-
18N.2.SL.TZ0.T_6e:
Use your answers to parts (c) and (d) to show that
A=3x2+10368x.
-
19M.1.SL.TZ1.S_8d:
Show that when the graphs of f and g intersect, 2x2−6x+k=0.
-
22M.2.SL.TZ1.4c:
Find the value of t at which the goat is eating grass at the greatest rate.
-
22M.2.AHL.TZ1.2c:
Find the value of t at which the goat is eating grass at the greatest rate.
-
21N.1.SL.TZ0.12b.i:
Solve S'(x)=0.
-
21N.1.AHL.TZ0.15b.i:
Find an expression for V in terms of θ.
-
21N.1.AHL.TZ0.15b.ii:
Find the expression dVdθ.
-
21N.1.AHL.TZ0.15b.iii:
Solve algebraically dVdθ=0 to find the value of θ that will maximize the volume, V.
-
16N.2.SL.TZ0.T_6b:
Express this volume in cm3.
-
SPM.2.SL.TZ0.4d:
Find P(x).
-
17M.1.SL.TZ1.S_6a.i:
Write down the gradient of the curve of f at P.
-
17M.2.SL.TZ2.S_8b.i:
Write down the coordinates of A.
-
19M.2.SL.TZ1.S_4a:
Sketch the graph of f″ on the grid below:
-
19M.2.SL.TZ2.S_5a:
Find the population of fish at t = 10.
-
18M.1.SL.TZ2.T_13c:
Find the number of shirts produced when the cost of production is lowest.
-
18N.2.SL.TZ0.T_6b:
Calculate the volume, in cm3, of the bag.
-
17M.1.AHL.TZ2.H_10a.ii:
Find the width of the window in terms of P when the area is a maximum, justifying that this is a maximum.
-
17M.1.AHL.TZ2.H_10b:
Show that in this case the height of the rectangle is equal to the radius of the semicircle.
-
18M.1.SL.TZ2.S_9c:
Given that there is a minimum value for C, find this minimum value in terms of π.
-
18M.1.SL.TZ2.T_13a:
Find the cost of producing 70 shirts.
-
17M.2.SL.TZ2.S_8b.ii:
Write down the rate of change of f at A.
-
18N.2.SL.TZ0.T_6h:
The cloth used to make Nanako’s bag costs 4 Japanese Yen (JPY) per cm2.
Find the cost of the cloth used to make Nanako’s bag.
-
19M.1.AHL.TZ1.H_8d:
Sketch the curve y=f(x), 0 ≤ x ≤ 5 indicating clearly the coordinates of the maximum and minimum points and any intercepts with the coordinate axes.
-
SPM.2.SL.TZ0.4a:
Calculate the surface area of the box in cm2.
-
19N.2.SL.TZ0.T_6c:
Given the design constraint that l=150-2πr2πr, show that V=150r-2πr33.
-
19M.2.SL.TZ1.S_4b:
Find the x-coordinates of the points of inflexion of the graph of f.
-
19M.1.SL.TZ1.T_15c:
Find the value of r when the area of the curved surface is maximized.
-
19M.2.SL.TZ2.S_5b:
Find the rate at which the population of fish is increasing at t = 10.
-
19N.2.SL.TZ0.T_6e:
Using your answer to part (d), show that V is a maximum when r is equal to √75π cm.
-
19N.2.SL.TZ0.T_6h:
Use your answer to part (f) to identify the shape of the speaker with the best quality of sound.
-
18N.2.SL.TZ0.T_6f:
Find dAdx.
-
17M.2.SL.TZ2.S_8c.i:
Find the coordinates of B.
-
19M.1.SL.TZ1.S_8e:
Given that the graphs of f and g intersect only once, find the value of k.
-
17N.1.SL.TZ0.T_15b:
Find how much Maria earns in one week, from selling cheese, if the price of a kilogram of cheese is 8 EUR.
-
19M.2.SL.TZ1.S_4c:
Hence find the values of x for which the graph of f is concave-down.
-
19N.2.SL.TZ0.T_6g:
Calculate the maximum value of V.
-
18N.1.SL.TZ0.S_10b.ii:
Hence, find the equation of L in terms of a.
-
18N.1.SL.TZ0.S_10a:
Find the coordinates of P.
-
17N.1.SL.TZ0.S_7:
Consider f(x)=logk(6x−3x2), for 0<x<2, where k>0.
The equation f(x)=2 has exactly one solution. Find the value of k.
-
16N.2.SL.TZ0.S_10a:
(i) Find the value of c.
(ii) Show that b=π6.
(iii) Find the value of a.
-
18N.2.SL.TZ0.T_6d:
Write down and simplify an expression in x and y for the area of cloth, A, used to make Nanako’s bag.
-
17M.1.SL.TZ1.S_6b:
Determine the concavity of the graph of f when 4<x<5 and justify your answer.
-
19M.1.SL.TZ2.T_15a:
Find the value of P if no vases are sold.
-
19N.2.SL.TZ0.T_6f:
Find the length of the cylinder for which V is a maximum.
-
19M.1.AHL.TZ2.H_8b:
Given that there is one inscribed cone having a maximum volume, show that the volume of this cone is 32πR381.
-
18N.2.SL.TZ0.T_6g:
Use your answer to part (f) to show that the width of Nanako’s bag is 12 cm.
-
19N.2.SL.TZ0.T_6d:
Find dVdr.
-
18N.1.SL.TZ0.S_10b.i:
Find f′(x).
-
17M.1.SL.TZ1.S_6a.ii:
Find the equation of the normal to the curve of f at P.
-
18N.1.SL.TZ0.S_10c:
The graph of f has a local minimum at the point Q. The line L passes through Q.
Find the value of a.
-
19N.2.SL.TZ0.T_6a:
Write down an expression for V, the volume (cm3) of the speaker, in terms of r, l and π.
-
19M.1.AHL.TZ1.H_8a:
Write down the x-coordinate of the point of inflexion on the graph of y=f(x).
-
19M.1.SL.TZ2.T_15b:
Differentiate P(x).
-
18M.1.AHL.TZ1.H_9b.i:
Show that there is exactly one point of inflexion, B, on the graph of y=f(x).
-
18M.1.AHL.TZ1.H_9a:
The graph of y=f(x) has a local maximum at A. Find the coordinates of A.
-
18N.2.SL.TZ0.S_10c:
When t = 0, the volume of water in the container is 2.3 m3. It is known that the container is never completely full of water during the 4 hour period.
Find the minimum volume of empty space in the container during the 4 hour period.
-
18M.1.AHL.TZ2.H_4:
Consider the curve y=11−x+4x−4.
Find the x-coordinates of the points on the curve where the gradient is zero.
-
16N.2.SL.TZ0.T_6f:
Using your answer to part (e), find the value of r which minimizes A.
-
18N.2.SL.TZ0.T_6c:
Use this value to write down, and simplify, the equation in x and y for the volume of Nanako’s bag.
-
18M.2.AHL.TZ1.H_9a:
Show that there are exactly two points on the curve where the gradient is zero.
-
20N.2.SL.TZ0.T_4a:
Write down an equation in r and h that shows this information.
-
20N.2.SL.TZ0.T_4d:
Using your answer to part (c), find the value of h for which V is a maximum.
-
19M.1.SL.TZ1.S_8b:
Show that the area of PQRS is 18b−2b3.
-
16N.2.SL.TZ0.S_10b:
(i) Write down the value of k.
(ii) Find g(x).
-
17M.2.SL.TZ2.S_8d:
Let R be the region enclosed by the graph of f , the x-axis, the line x=b and the line x=a. The region R is rotated 360° about the x-axis. Find the volume of the solid formed.
-
16N.2.SL.TZ0.T_6e:
Find dAdr.
-
17M.2.SL.TZ2.S_8a:
Find the value of p.
-
18M.1.SL.TZ2.S_9b:
Show that C=20πr2+320πr.
-
19M.2.SL.TZ2.S_5c:
Find the value of t for which the population of fish is increasing most rapidly.
-
18N.2.SL.TZ0.S_10a:
Find the volume of the container.
-
SPM.2.SL.TZ0.4e:
Find the least number of boxes which must be sold each week in order to make a profit.
-
18M.1.AHL.TZ1.H_9b.ii:
The coordinates of B can be expressed in the form B(2a,b×2−3a) where a, b∈Q. Find the value of a and the value of b.
-
17N.1.SL.TZ0.T_15a:
Write down how many kilograms of cheese Maria sells in one week if the price of a kilogram of cheese is 8 EUR.
-
18M.2.AHL.TZ1.H_9d:
The shaded region is rotated by 2π about the y-axis. Find the volume of the solid formed.
-
18N.2.SL.TZ0.S_10b.ii:
During the interval p < t < q, he volume of water in the container increases by k m3. Find the value of k.
-
20N.2.SL.TZ0.T_4e:
Find the maximum volume of the bird bath.
-
17N.1.SL.TZ0.T_15d:
Find the price, p, that will give Maria the highest weekly profit.
-
SPM.2.SL.TZ0.4b:
Calculate the length AG.
-
17N.1.SL.TZ0.T_15c:
Write down an expression for W in terms of p.
-
19M.1.AHL.TZ1.H_8b:
find the value of f(1).
-
20N.2.SL.TZ0.T_4c:
Find dVdh.
-
21M.1.SL.TZ2.13a:
Find an expression for P in terms of x.
-
20N.2.SL.TZ0.T_4b:
Show that V=2500πh3-πh33.
-
16N.2.SL.TZ0.T_6c:
Write down, in terms of r and h, an equation for the volume of this water container.
-
16N.2.SL.TZ0.T_6a:
Write down a formula for A, the surface area to be coated.
-
16N.2.SL.TZ0.T_6h:
Find the least number of cans of water-resistant material that will coat the area in part (g).
-
21M.2.SL.TZ1.5a.ii:
Hence find the maximum height of the tunnel.
-
21M.2.AHL.TZ1.2a.ii:
Hence find the maximum height of the tunnel.
-
18N.2.SL.TZ0.S_10b.i:
Find the value of p and of q.
-
16N.2.SL.TZ0.T_6d:
Show that A=πr2+1000000r.
-
SPM.2.SL.TZ0.4c:
Find the number of boxes that should be sold each week to maximize the profit.
-
16N.2.SL.TZ0.S_10c:
(i) Find w.
(ii) Hence or otherwise, find the maximum positive rate of change of g.
-
18M.2.AHL.TZ1.H_9c:
The normal at P cuts the curve again at the point Q. Find the x-coordinate of Q.
-
19M.1.SL.TZ1.S_8a:
Find the x-intercepts of the graph of f.
-
19N.2.SL.TZ0.T_6b:
Write down an equation for the surface area of the speaker in terms of r, l and π.
-
19M.1.SL.TZ1.S_8c:
Hence find the value of b such that the area of PQRS is a maximum.
-
21M.1.SL.TZ2.13b:
The company regularly increases the number of cars it produces.
Describe how their profit changes if they increase production to over 30 cars per month and up to 50 cars per month. Justify your answer.
-
19M.1.SL.TZ1.T_15a:
Write down an equation for the area, A, of the curved surface in terms of r.
-
16N.2.SL.TZ0.T_6g:
Find the value of this minimum area.
-
18M.2.AHL.TZ1.H_9b:
Find the equation of the normal to the curve at the point P.
-
19M.1.AHL.TZ1.H_8c:
find the value of f(4).
-
18M.1.SL.TZ2.S_9a:
Express h in terms of r.
-
17M.2.SL.TZ2.S_8c.ii:
Find the the rate of change of f at B.
-
19M.1.SL.TZ1.T_15b:
Find dAdr.
-
19M.1.AHL.TZ2.H_8a:
Show that the volume of the cone may be expressed by V=π3(2Rh2−h3).
-
20N.2.SL.TZ0.T_4f:
To prevent leaks, a sealant is applied to the interior surface of the bird bath.
Find the surface area to be covered by the sealant, given that the bird bath has maximum volume.
-
EXN.2.SL.TZ0.2e:
Hence or otherwise find the minimum length of ribbon required.
-
21N.1.AHL.TZ0.15a:
Show that r=62+θ.
-
EXN.2.SL.TZ0.2a:
Find an expression for the total length of the ribbon L in terms of x and y.
-
EXN.2.SL.TZ0.2b:
Show that L=2x+300x+22
-
EXN.2.SL.TZ0.2c:
Find dLdx
-
EXN.2.SL.TZ0.2d:
Solve dLdx=0
-
21N.1.SL.TZ0.12a:
Find S′(x).
-
21N.1.SL.TZ0.12b.ii:
Interpret your answer to (b)(i) in context.

