| Date | May 2017 | Marks available | 1 | Reference code | 17M.2.SL.TZ2.T_6 |
| Level | Standard Level | Paper | Paper 2 | Time zone | Time zone 2 |
| Command term | Write down | Question number | T_6 | Adapted from | N/A |
Question
Consider the function f(x)=−x4+ax2+5, where a is a constant. Part of the graph of y=f(x) is shown below.
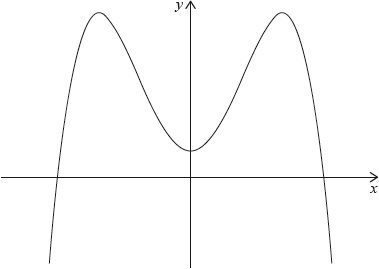
It is known that at the point where x=2 the tangent to the graph of y=f(x) is horizontal.
There are two other points on the graph of y=f(x) at which the tangent is horizontal.
Write down the y-intercept of the graph.
Find f′(x).
Show that a=8.
Find f(2).
Write down the x-coordinates of these two points;
Write down the intervals where the gradient of the graph of y=f(x) is positive.
Write down the range of f(x).
Write down the number of possible solutions to the equation f(x)=5.
The equation f(x)=m, where m∈R, has four solutions. Find the possible values of m.
Markscheme
* This question is from an exam for a previous syllabus, and may contain minor differences in marking or structure.
5 (A1)
Note: Accept an answer of (0, 5).
[1 mark]
(f′(x)=)−4x3+2ax (A1)(A1)
Note: Award (A1) for −4x3 and (A1) for +2ax. Award at most (A1)(A0) if extra terms are seen.
[2 marks]
−4×23+2a×2=0 (M1)(M1)
Note: Award (M1) for substitution of x=2 into their derivative, (M1) for equating their derivative, written in terms of a, to 0 leading to a correct answer (note, the 8 does not need to be seen).
a=8 (AG)
[2 marks]
(f(2)=)−24+8×22+5 (M1)
Note: Award (M1) for correct substitution of x=2 and a=8 into the formula of the function.
21 (A1)(G2)
[2 marks]
(x=) −2, (x=) 0 (A1)(A1)
Note: Award (A1) for each correct solution. Award at most (A0)(A1)(ft) if answers are given as (−2 ,21) and (0, 5) or (−2, 0) and (0, 0).
[2 marks]
x<−2, 0<x<2 (A1)(ft)(A1)(ft)
Note: Award (A1)(ft) for x<−2, follow through from part (d)(i) provided their value is negative.
Award (A1)(ft) for 0<x<2, follow through only from their 0 from part (d)(i); 2 must be the upper limit.
Accept interval notation.
[2 marks]
y⩽21 (A1)(ft)(A1)
Notes: Award (A1)(ft) for 21 seen in an interval or an inequality, (A1) for “y⩽”.
Accept interval notation.
Accept −∞<y⩽21 or f(x)⩽21.
Follow through from their answer to part (c)(ii). Award at most (A1)(ft)(A0) if x is seen instead of y. Do not award the second (A1) if a (finite) lower limit is seen.
[2 marks]
3 (solutions) (A1)
[1 mark]
5<m<21 or equivalent (A1)(ft)(A1)
Note: Award (A1)(ft) for 5 and 21 seen in an interval or an inequality, (A1) for correct strict inequalities. Follow through from their answers to parts (a) and (c)(ii).
Accept interval notation.
[2 marks]

