IB Questionbank

Updates to Questionbank

User interface language: English | Español

Date	November 2016	Marks available	2	Reference code	16N.2.SL.TZ0.T_6
Level	Standard Level	Paper	Paper 2	Time zone	Time zone 0
Command term	Show that	Question number	T_6	Adapted from	N/A

Question

A water container is made in the shape of a cylinder with internal height $h$ cm and internal base radius $r$ cm.

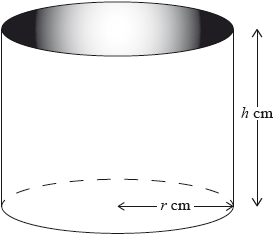
N16/5/MATSD/SP2/ENG/TZ0/06

The water container has no top. The inner surfaces of the container are to be coated with a water-resistant material.

The volume of the water container is $0.5{\text{ }}{{\text{m}}^3}$ .

The water container is designed so that the area to be coated is minimized.

One can of water-resistant material coats a surface area of $2000{\text{ c}}{{\text{m}}^2}$ .

Write down a formula for $A$ , the surface area to be coated.

[2]

a.

Express this volume in ${\text{c}}{{\text{m}}^3}$ .

[1]

b.

Write down, in terms of $r$ and $h$ , an equation for the volume of this water container.

[1]

c.

Show that $A = \pi {r^2} + \frac{{1\,000\,000}}{r}$ .

[2]

d.

Find $\frac{{{\text{d}}A}}{{{\text{d}}r}}$ .

[3]

e.

Using your answer to part (e), find the value of $r$ which minimizes $A$ .

[3]

f.

Find the value of this minimum area.

[2]

g.

Find the least number of cans of water-resistant material that will coat the area in part (g).

[3]

h.

Markscheme

* This question is from an exam for a previous syllabus, and may contain minor differences in marking or structure.

$(A = ){\text{ }}\pi {r^2} + 2\pi rh$ (A1)(A1)

Note: Award (A1) for either $\pi {r^2}$ OR $2\pi rh$ seen. Award (A1) for two correct terms added together.

[2 marks]

a.

$500\,000$ (A1)

Notes: Units not required.

[1 mark]

b.

$500\,000 = \pi {r^2}h$ (A1)(ft)

Notes: Award (A1)(ft) for $\pi {r^2}h$ equating to their part (b).

Do not accept unless $V = \pi {r^2}h$ is explicitly defined as their part (b).

[1 mark]

c.

$A = \pi {r^2} + 2\pi r\left( {\frac{{500\,000}}{{\pi {r^2}}}} \right)$ (A1)(ft)(M1)

Note: Award (A1)(ft) for their ${\frac{{500\,000}}{{\pi {r^2}}}}$ seen.

Award (M1) for correctly substituting only ${\frac{{500\,000}}{{\pi {r^2}}}}$ into a correct part (a).

Award (A1)(ft)(M1) for rearranging part (c) to $\pi rh = \frac{{500\,000}}{r}$ and substituting for $\pi rh$ in expression for $A$ .

$A = \pi {r^2} + \frac{{1\,000\,000}}{r}$ (AG)

Notes: The conclusion, $A = \pi {r^2} + \frac{{1\,000\,000}}{r}$ , must be consistent with their working seen for the (A1) to be awarded.

Accept ${10^6}$ as equivalent to ${1\,000\,000}$ .

[2 marks]

d.

$2\pi r - \frac{{{\text{1}}\,{\text{000}}\,{\text{000}}}}{{{r^2}}}$ (A1)(A1)(A1)

Note: Award (A1) for $2\pi r$ , (A1) for $\frac{1}{{{r^2}}}$ or ${r^{ - 2}}$ , (A1) for $- {\text{1}}\,{\text{000}}\,{\text{000}}$ .

[3 marks]

e.

$2\pi r - \frac{{1\,000\,000}}{{{r^2}}} = 0$ (M1)

Note: Award (M1) for equating their part (e) to zero.

${r^3} = \frac{{1\,000\,000}}{{2\pi }}$ OR $r = \sqrt[3]{{\frac{{1\,000\,000}}{{2\pi }}}}$ (M1)

Note: Award (M1) for isolating $r$ .

OR

sketch of derivative function (M1)

with its zero indicated (M1)

$(r = ){\text{ }}54.2{\text{ }}({\text{cm}}){\text{ }}(54.1926 \ldots )$ (A1)(ft)(G2)

[3 marks]

f.

$\pi {(54.1926 \ldots )^2} + \frac{{1\,000\,000}}{{(54.1926 \ldots )}}$ (M1)

Note: Award (M1) for correct substitution of their part (f) into the given equation.

$= 27\,700{\text{ }}({\text{c}}{{\text{m}}^2}){\text{ }}(27\,679.0 \ldots )$ (A1)(ft)(G2)

[2 marks]

g.

$\frac{{27\,679.0 \ldots }}{{2000}}$ (M1)

Note: Award (M1) for dividing their part (g) by 2000.

$= 13.8395 \ldots$ (A1)(ft)

Notes: Follow through from part (g).

14 (cans) (A1)(ft)(G3)

Notes: Final (A1) awarded for rounding up their $13.8395 \ldots$ to the next integer.

[3 marks]

h.

Examiners report

[N/A]

a.

[N/A]

b.

[N/A]

c.

[N/A]

d.

[N/A]

e.

[N/A]

f.

[N/A]

g.

[N/A]

h.

Syllabus sections

Topic 3—Geometry and trigonometry » SL 3.1—3d space, volume, angles, midpoints

Show 90 related questions

17M.2.SL.TZ1.T_4f:
In the context of this model, state what the value of 19 represents.
22M.1.SL.TZ1.1:
The front view of a doghouse is made up of a square with an isosceles triangle on top.

The doghouse is $1.35 m$ high and $0.9 m$ wide, and sits on a square base.

The top of the rectangular surfaces of the roof of the doghouse are to be painted.

Find the area to be painted.
18M.2.SL.TZ1.T_6c:
Find the height of the cylinder, h , of the new trash can, in terms of r.
18M.2.SL.TZ1.T_6a:
Write down the height of the cylinder.
22M.3.AHL.TZ1.2d:
A car departs from a point due north of Hamilton. It travels due east at constant speed to a destination point due North of Gaussville. It passes through the Edison, Isaacopolis and Fermitown districts. The car spends $30 %$ of the travel time in the Isaacopolis district.

Find the distance between Gaussville and the car’s destination point.
22M.1.AHL.TZ1.6a:
Find $\vec{AF}$ .
22M.1.AHL.TZ1.6b:
Find the length of the rope.
22M.1.AHL.TZ1.6c:
Find $FÂO$ , the angle the rope makes with the platform.
22M.2.SL.TZ2.3b:
Find the equation of $(XZ)$ .
22M.2.SL.TZ2.3d:
Determine the exact length of $[YZ]$ .
22M.2.SL.TZ2.3e:
Given that the exact length of $[XY]$ is $\sqrt{32}$ , find the size of $XŶZ$ in degrees.
16N.1.SL.TZ0.T_7a:
Calculate the volume of the balloon.
21N.1.SL.TZ0.8b:
Find the surface area of the outside of the hat.
21N.2.SL.TZ0.4b.ii:
Hence or otherwise, show that the volume of the reservoir is $29 600 m^{3}$ .
22M.1.SL.TZ1.2a:
Find the length of the rope connecting $A$ to $F$ .
22M.1.SL.TZ1.2b:
Find $FÂO$ , the angle the rope makes with the ground.
21N.1.AHL.TZ0.15b.i:
Find an expression for $V$ in terms of $θ$ .
21N.1.AHL.TZ0.15b.ii:
Find the expression $\frac{d V}{d θ}$ .
21N.1.AHL.TZ0.15b.iii:
Solve algebraically $\frac{d V}{d θ} = 0$ to find the value of $θ$ that will maximize the volume, $V$ .
18N.1.SL.TZ0.T_9a.i:
Write down the value of x.
16N.2.SL.TZ0.T_6b:
Express this volume in ${\text{c}}{{\text{m}}^3}$ .
17M.2.SL.TZ1.T_4e:
Calculate, to the nearest second, the time since the pizza was taken out of the oven until it can be eaten.
18M.2.SL.TZ1.T_1b:
Calculate the total volume of the barn.
18N.1.SL.TZ0.T_9a.ii:
Calculate the volume of the paperweight.
18N.1.SL.TZ0.T_9b:
1 cm³ of glass has a mass of 2.56 grams.

Calculate the mass, in grams, of the paperweight.
16N.1.SL.TZ0.T_7b:
Calculate the radius of the balloon following this increase.
18M.2.SL.TZ1.T_6e:
Using your graphic display calculator, find the value of r which maximizes the value of V.
18M.2.SL.TZ1.T_1d:
Calculate the length of AE.
19N.2.SL.TZ0.T_6c:
Given the design constraint that $l = \frac{150 - 2 π r^{2}}{π r}$ , show that $V = 150 r - \frac{2 π r^{3}}{3}$ .
18M.2.SL.TZ1.T_1e:
Show that Farmer Brown is incorrect.
19M.1.SL.TZ1.T_15c:
Find the value of $r$ when the area of the curved surface is maximized.
19N.2.SL.TZ0.T_6e:
Using your answer to part (d), show that $V$ is a maximum when $r$ is equal to $\sqrt{\frac{75}{π}} cm$ .
19N.2.SL.TZ0.T_6h:
Use your answer to part (f) to identify the shape of the speaker with the best quality of sound.
19N.2.SL.TZ0.T_6g:
Calculate the maximum value of $V$ .
19M.2.SL.TZ2.T_3e:
The factory director wants to increase the volume of coconut water sold per container.

State whether or not they should replace the cone-shaped containers with cylinder‑shaped containers. Justify your conclusion.
21M.1.SL.TZ1.3b:
The total surface of the candy is coated in chocolate. It is known that $1$ gram of the chocolate covers an area of $240 {mm}^{2}$ .

Calculate the weight of chocolate required to coat one piece of candy.
19N.1.SL.TZ0.T_5a:
Calculate the volume of oil drained from Yao’s motorbike.
17M.2.SL.TZ1.T_4b:
Find the radius of the sphere in cm, correct to one decimal place.
19N.2.SL.TZ0.T_6f:
Find the length of the cylinder for which $V$ is a maximum.
19N.2.SL.TZ0.T_6d:
Find $\frac{d V}{d r}$ .
SPM.1.SL.TZ0.11:
Helen is building a cabin using cylindrical logs of length 2.4 m and radius 8.4 cm. A wedge is cut from one log and the cross-section of this log is illustrated in the following diagram.

Find the volume of this log.
18M.2.SL.TZ1.T_1a:
Calculate the area of triangle EAD.
19N.2.SL.TZ0.T_6a:
Write down an expression for $V$ , the volume (cm³) of the speaker, in terms of $r$ , $l$ and $π$ .
17M.2.SL.TZ1.T_4a:
Calculate the volume of this pan.
17M.2.SL.TZ1.T_4d:
Find the temperature that the pizza will be 5 minutes after it is taken out of the oven.
17N.2.SL.TZ0.T_6e:
Find the total cost of the ingredients of one special dessert.
16N.2.SL.TZ0.T_6f:
Using your answer to part (e), find the value of $r$ which minimizes $A$ .
18M.2.SL.TZ1.T_1f:
Calculate the total length of metal required for one support.
16N.2.SL.TZ0.T_6e:
Find $\frac{{{\text{d}}A}}{{{\text{d}}r}}$ .
18M.2.SL.TZ1.T_6b:
Find the total volume of the trash can.
19M.2.SL.TZ2.T_3a:
Find the slant height of the cone-shaped container.
17N.2.SL.TZ0.T_6f:
Find the value of $x$ .
19M.2.SL.TZ2.T_3b:
Find the slant height of the cone-shaped container.
17N.2.SL.TZ0.T_6b:
Calculate the radius, $r$ , of a hemisphere shaped glass.
19M.1.SL.TZ1.T_6a:
Find the volume of the money box.
20N.1.SL.TZ0.T_1c:
The actual volume of the asteroid is found to be $6.074 \times 10^{6} {km}^{3}$ .

Find the percentage error in James’s estimate of the volume.
20N.1.SL.TZ0.T_1b:
Calculate James’s estimate of its volume, in ${km}^{3}$ .
18M.1.SL.TZ1.T_14a:
Calculate the radius of the base of the cone which has been removed.
18M.2.SL.TZ1.T_6f:
The designer claims that the new trash can has a capacity that is at least 40% greater than the capacity of the original trash can.

State whether the designer’s claim is correct. Justify your answer.
18M.2.SL.TZ1.T_6d:
Show that the volume, V cm³ , of the new trash can is given by

$V = 110\pi {r^3}$ .
16N.2.SL.TZ0.T_6c:
Write down, in terms of $r$ and $h$ , an equation for the volume of this water container.
16N.2.SL.TZ0.T_6a:
Write down a formula for $A$ , the surface area to be coated.
16N.2.SL.TZ0.T_6h:
Find the least number of cans of water-resistant material that will coat the area in part (g).
17M.2.SL.TZ1.T_4c:
Find the value of $a$ .
21M.1.SL.TZ2.3:
A storage container consists of a box of length $90 cm$ , width $42 cm$ and height $34 cm$ , and a lid in the shape of a half-cylinder, as shown in the diagram. The lid fits the top of the box exactly. The total exterior surface of the storage container is to be painted.

Find the area to be painted.
18M.2.SL.TZ1.T_1c:
Calculate the length of MN.
20N.1.SL.TZ0.T_1a:
Write down the value of the iron in the form $a \times 10^{k}$ where $1 \leq a < 10, k \in ℤ$ .
21M.1.SL.TZ2.2b:
Find the coordinates of station $M$ .
21M.1.SL.TZ2.2c:
Write down the height of station $M$ , in metres, above the ground.
21M.1.SL.TZ2.2a:
Find the distance between stations $A$ and $B$ .
19N.2.SL.TZ0.T_6b:
Write down an equation for the surface area of the speaker in terms of $r$ , $l$ and $π$ .
17N.2.SL.TZ0.T_6c:
Find the cost of $100{\text{ c}}{{\text{m}}^3}$ of chocolate mousse.
19M.1.SL.TZ1.T_6b:
A second money box is in the shape of a sphere and has the same volume as the cylindrical money box.

Find the diameter of the second money box.
21M.2.SL.TZ2.5b:
Given that the total external surface area of the box is $1200 {cm}^{2}$ , show that the volume of the box may be expressed as $V = 300 \sqrt{3} x - \frac{9}{4} x^{3}$ .
17N.2.SL.TZ0.T_6a:
Show that the volume of a cone shaped glass is $160{\text{ c}}{{\text{m}}^3}$ , correct to 3 significant figures.
19M.1.SL.TZ1.T_15a:
Write down an equation for the area, $A$ , of the curved surface in terms of $r$ .
16N.2.SL.TZ0.T_6g:
Find the value of this minimum area.
19N.1.SL.TZ0.T_5b:
Yao then pours all the oil from the cuboids into an empty cylindrical container. The height of the oil in the container is $30$ cm.

Find the internal radius, $r$ , of the container.
19M.2.SL.TZ2.T_3d:
Find the height, $h$ , of this cylinder-shaped container.
19M.2.SL.TZ2.T_3c:
Show that the total surface area of the cone-shaped container is 314 cm², correct to three significant figures.
17N.2.SL.TZ0.T_6d:
Show that there is $20{\text{ c}}{{\text{m}}^3}$ of orange paste in each special dessert.
19M.1.SL.TZ1.T_15b:
Find $\frac{{{\text{d}}A}}{{{\text{d}}r}}$ .
21M.1.SL.TZ1.3a:
Calculate the total surface area of one piece of candy.
21N.1.AHL.TZ0.15a:
Show that $r = \frac{6}{2 + θ}$ .
21N.1.SL.TZ0.8a.i:
Write down the perimeter of the base of the hat in terms of $π$ .
21N.1.SL.TZ0.8a.ii:
Find the value of $θ$ .
21N.2.SL.TZ0.4a:
Find the angle of depression from $M$ to $N$ .
21N.2.SL.TZ0.4b.i:
Find $CV$ .
21N.2.SL.TZ0.4c:
By finding an appropriate value, determine whether Joshua is correct.
21N.2.SL.TZ0.4d:
To avoid water leaking into the ground, the five interior sides of the reservoir have been painted with a watertight material.

Find the area that was painted.

Hide related questions

Topic 5—Calculus » SL 5.1—Introduction of differential calculus

Topic 5—Calculus » SL 5.3—Differentiating polynomials, n E Z

Topic 2—Functions » SL 2.4—Key features of graphs, intersections using technology

Topic 4—Statistics and probability » SL 4.5—Probability concepts, expected numbers

Topic 2—Functions » SL 2.5—Modelling functions

Topic 4—Statistics and probability » SL 4.6—Combined, mutually exclusive, conditional, independence, prob diagrams

Topic 5—Calculus » SL 5.6—Stationary points, local max and min

Topic 5—Calculus » SL 5.7—Optimisation

Topic 1—Number and algebra » SL 1.8—Use of technology to solve systems of linear equations and polynomial equations

Topic 1—Number and algebra

Topic 2—Functions

Topic 3—Geometry and trigonometry

Topic 4—Statistics and probability

Topic 5—Calculus