 In this topic, we will look at
In this topic, we will look at
- Trigonometric Functions
- The Unit Circle
- Trigonometric Identities
- Solving Trigonometric Equations
- Triangle Geometry
- Vectors and Angles
- Equation of Lines
- Kinematics
- Intersections
- Vector Product
- Equation of Planes

3-Dimensional Solids
In this page, we will look at finding the volume and surface area of three-dimensional solids. The formulae for these solids can all be found in the formula booklet. You just need to know where to find them, as some are in the prior learning...

Radians, Arcs and Sectors
In this page, we will look at another measure of angles, that is, radians. This is a far more useful measure than degrees. Formula for calculating arcs and sectors become simpler and most importantly, we need to use radian measure whenever...
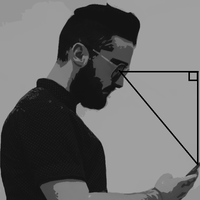
Right-angled Trigonometry
In this page, we will learn about right-angled trigonometry. For many, this will not be a new topic, nor will it be a difficult one. However, it deserves some time, as it forms the important step to understanding most of the other work in the...

Sine and Cosine Rule
In this page, we will look at the Sine Rule, the Cosine Rule and the formula for the Area of a Triangle. The formula for these three are fairly straight forward to use, but don't take this topic too lightly, sometimes the exam questions can be quite challe
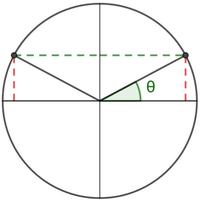
Unit Circle HL
The Unit Circle is probably the most important topic to understand from the whole of trigonometry. Lots of the properties of the trigonometric functions can be found from the unit circle. All the work on this page will help us understand all...
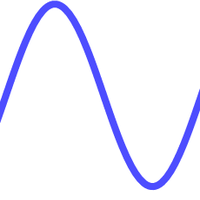
Trigonometric Graphs
In this page, we will learn about the graphs of the circular functions sinx, cosx and tanx. We will link this to work that we have done on Transforming Functions to see how translating and stretching the graphs affect the tigonometric functions....

Pythagorean Identities HL
In this page, we will will learn about the Pythagorean Identities. It is actually quite rare that exam questions are solely about these identities, but it is essential that you can use and manipulate them confidently because they are used in...
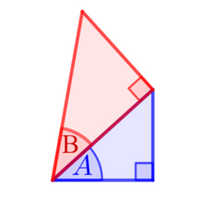
Compound Angle Formulae
In this page, we will will learn about the Compound Angle Formulae used in Trigonometry. These are really important because they open up so many other formulae in trigonometry. In particular, we can derive the double angle formula. There are...
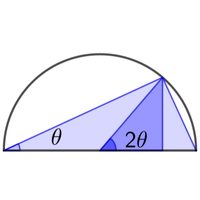
Double Angle Formulae HL
In this page, we will will learn about the Double Angle Formulae used in Trigonometry. It is actually quite rare that exam questions are solely about these identities, but it is essential that you can use and manipulate them confidently because...

Solving Trigonometric Equations
Solving trigonometric equations is a common topic on the examination. The key to solving them is a good knowledge of the trigonometric functions. Whether you prefer to use the Unit Circle or the graphs of the functions, you need to a method that works for

Scalar Product and Angles
In this topic, we will look at finding the angle between vectors in different circumstances. The whole topic revolves around the scalar (or dot) product. Often we are concerned with perpendicular vectors, and the fact that the scalar product equals zero in

Equation of a Line
You should already be familiar with the equation of a straight line in Cartesian form in 2 dimensions, y = ax + b. When we move into 3 dimensions, the Cartesian form becomes a little more awkward. Don't worry, vectors are here to help us out! Once you unde

Intersection of Lines
This page looks at the intersections of lines in 2 and 3 dimensions. In 2D, lines intersect or are parallel. In 3D, it is slightly more complicated, they can intersect or be parallel or be skew (not parallel and not intersect!). We will also look at applic

Kinematics
This page is all about kinematics for vectors. You can describe the position of a moving object using vectors. This is very much like the equation of a straight line except that the parameter is t for time. The equation below is an example:...

Vector Product
The vector product is a way of combining two vectors to find a third vector that is perpendicular to the first two. The vector product is a vector! Don't mix it up with the scalar product which is a scalar! The vector product is useful for...
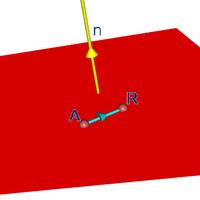
Equations of Planes
This is a hugely important topic in the HL course. We can describe planes in 3D in a number of different ways. It is not enough to learn the different equations, but it is vital that you have a strong conceptual understanding of the different forms of the

Intersection of Planes
This page is all about how planes meet (or not). Two planes will either be parallel or meet at a line. Three planes will either 1) not meet - zero solutions, 2) meet at a point - unique solution, or 3) meet at a line (or plane) - infinite solutions. We wil

Intersection of Line and Plane
For questions involving lines and planes, we are usually asked to find the point of intersection. However, when we consider a line and a plane, there are three possible situations. In this page, we will consider all the possible outcomes. 1) the line in


 Twitter
Twitter  Facebook
Facebook  LinkedIn
LinkedIn