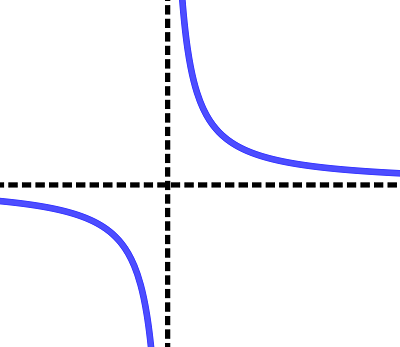
On this page, we will look at the properties of the reciprocal function and rational functions. You may be required to draw a sketch of these functions. In these cases, it is important to know how to find the vertical asymptote, the horizontal asymptote any oblique asymptote and the x and y intercepts.
On this page, you should learn about
- the reciprocal function \(f(x)=\frac{1}{x}\)
- rational functions of the form \(f(x)=\frac{ax+b}{cx+d}\)
- equations of vertical and horizontal asymptotes
- rational functions of the form \(f(x)=\frac{ax+b}{cx^2+dx+e}\)
- rational functions of the form \(f(x)=\frac{ax^2+bx+c}{dx+e}\)
- oblique asymptotes
Print from here
Here is a quiz about rational functions in the form \(f(x)=\frac{ax+b}{cx+d}\)
START QUIZ!
Here is a second quiz about rational functions in the form \(f(x)=\frac{ax+b}{cx^2+dx+e}\) and \(f(x)=\frac{ax^2+bx+c}{dx+e}\)
START QUIZ!
How much of Rational Functions HL have you understood?









 Twitter
Twitter  Facebook
Facebook  LinkedIn
LinkedIn