 In this page, we will learn about right-angled trigonometry. For many, this will not be a new topic, nor will it be a difficult one. However, it deserves some time, as it forms the important step to understanding most of the other work in the geometry and trigonometry topic. The challenging part is when we look at trigonometry in 3 dimensions, as it can be difficult to visualise the triangles needed. The emphasis of this page is looking at these 3-D problems.
In this page, we will learn about right-angled trigonometry. For many, this will not be a new topic, nor will it be a difficult one. However, it deserves some time, as it forms the important step to understanding most of the other work in the geometry and trigonometry topic. The challenging part is when we look at trigonometry in 3 dimensions, as it can be difficult to visualise the triangles needed. The emphasis of this page is looking at these 3-D problems.
On this page, you should learn about
- Using sine, cosine and tangent ratios to find the sides and angles of right-angled triangles.
- Angles of elevation and depression..
- The size of an angle between between a line and a plane.
Print from here
Here is a quiz that practises using the three trigonometric ratios
START QUIZ!
Here is a quiz that gets you to think about trigonometry in 3 dimensions
START QUIZ!


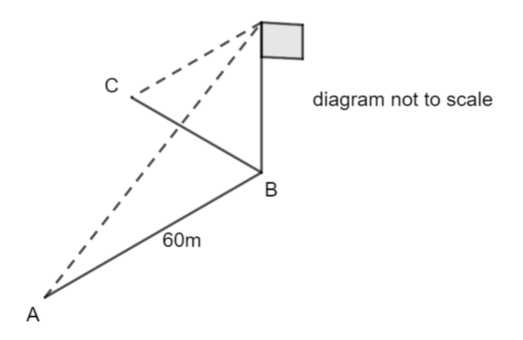
A, B and C are points on horizontal ground.
C is due West of B. A is due South of B. AB = 60m
A flagpole stands vertically at B.
From A, the angle of elevation of the top of the flagpole is 11°.
From B, the angle of elevation of the top of the flagpole is 15°.
Calculate the distance AC giving your answer to 3 significant figures.
Hint
Full Solution
How much of Right-angled Trigonometry have you understood?


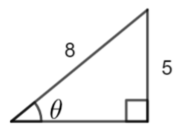
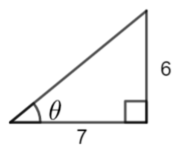

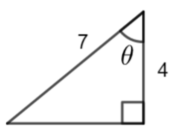
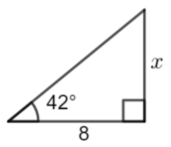
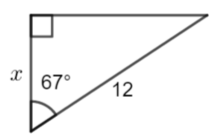
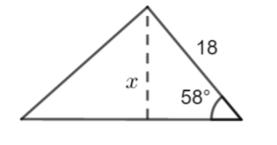

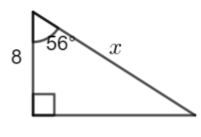

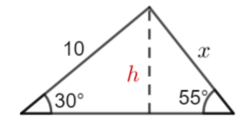 Calculate the height of the triangle first.
Calculate the height of the triangle first.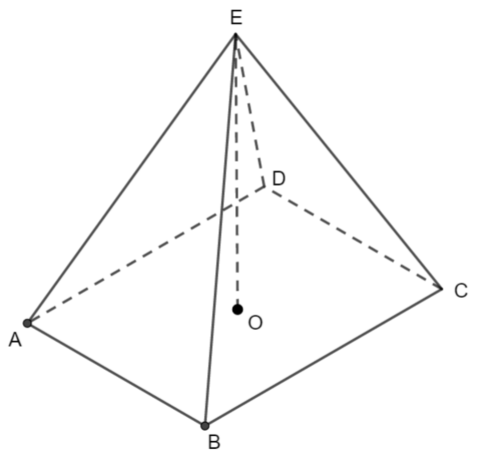
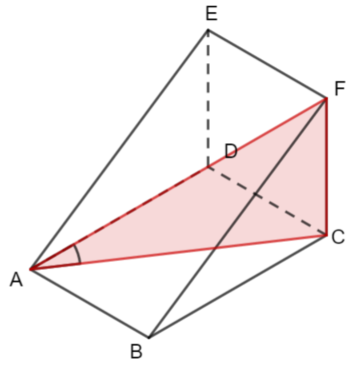



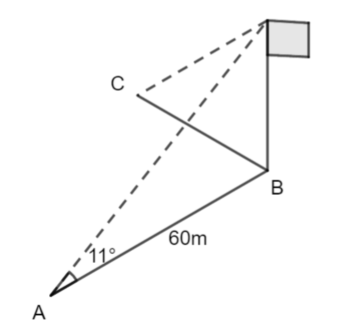 We can find the height of the flagpole
We can find the height of the flagpole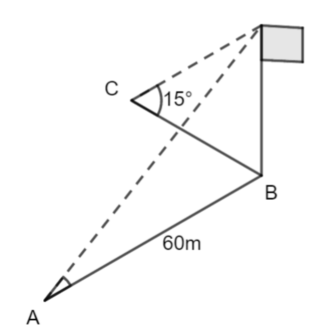 Now we can find the length BC
Now we can find the length BC


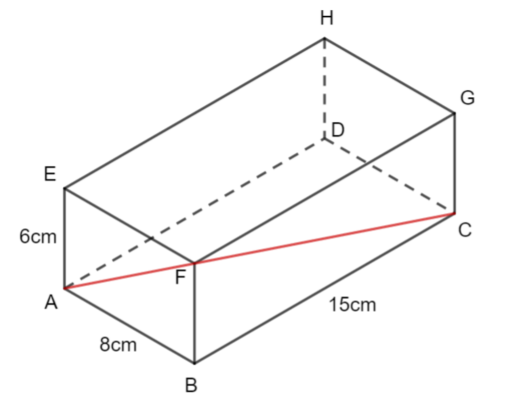
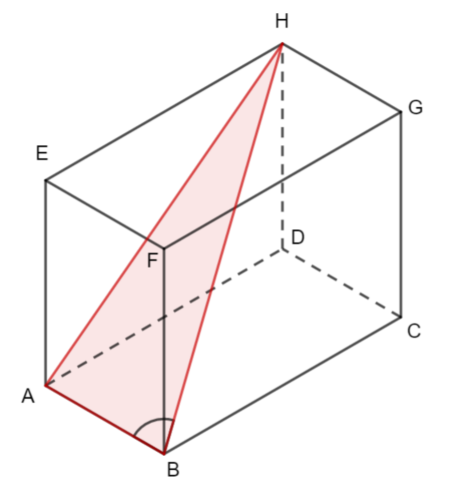
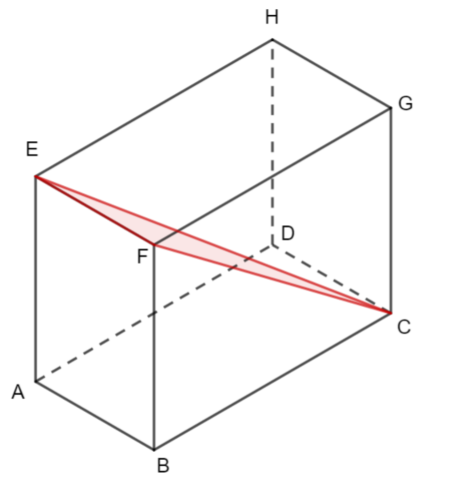
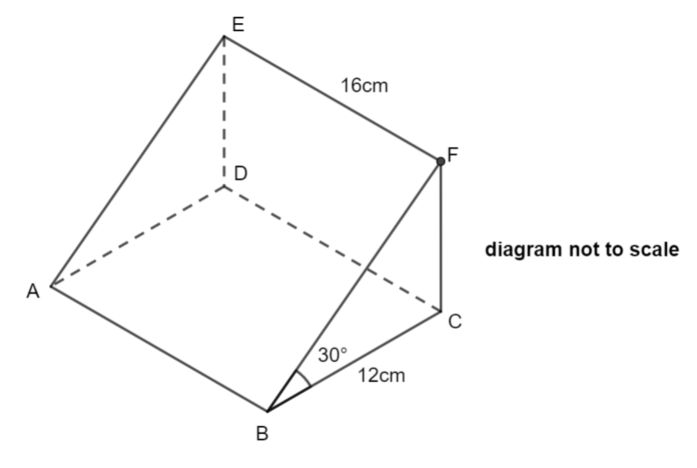
 The angle between the line EC and the plane ABCD can be visualised in the diagram.
The angle between the line EC and the plane ABCD can be visualised in the diagram.


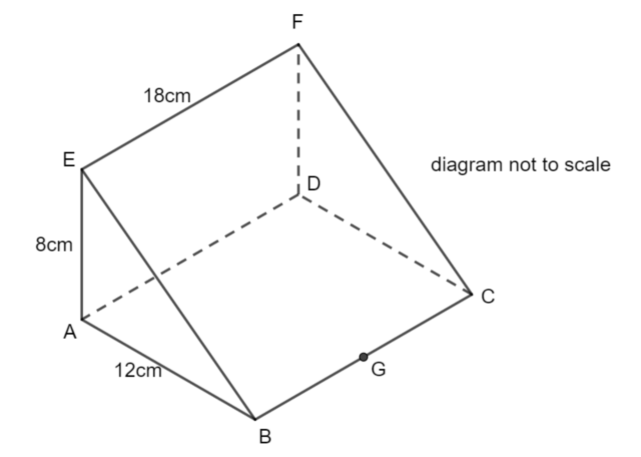
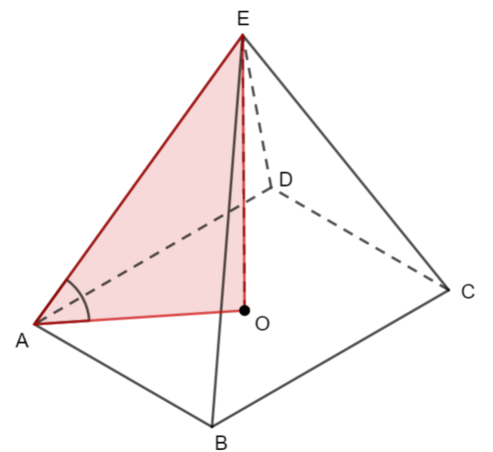
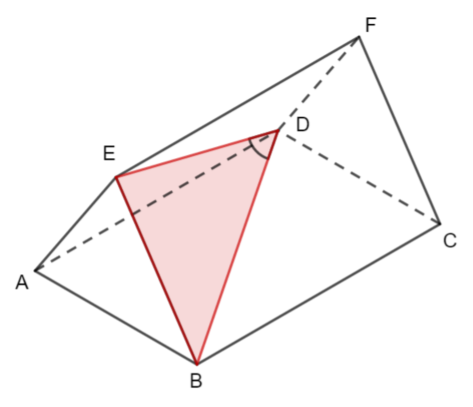
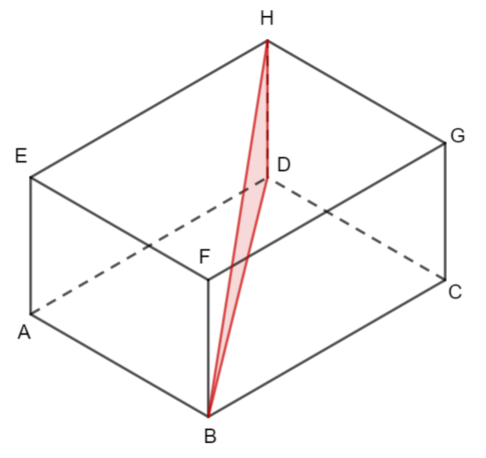
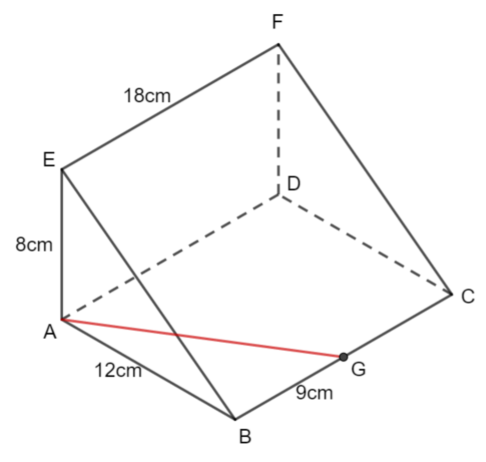 We shoud calculate the length AG using Pythagoras' Theorem.
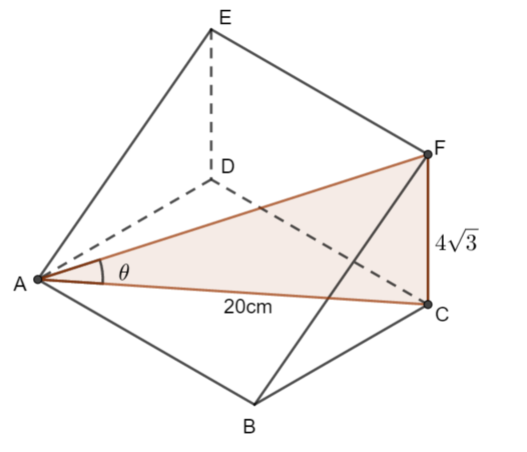
We shoud calculate the length AG using Pythagoras' Theorem. Here is the angle that we are required to find.
Here is the angle that we are required to find.

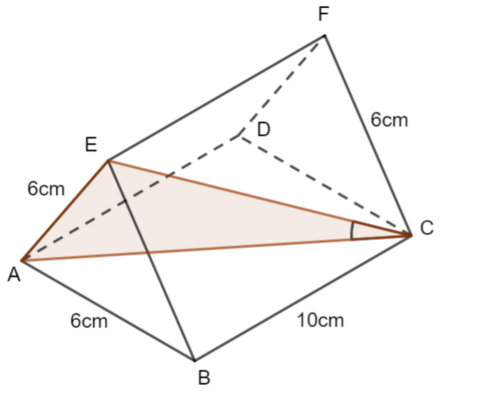
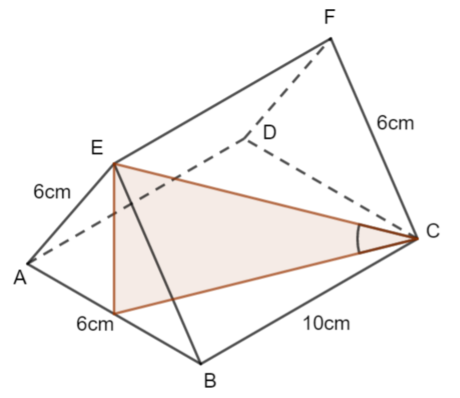
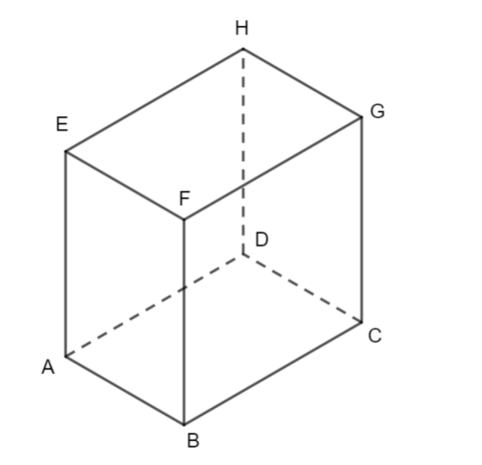
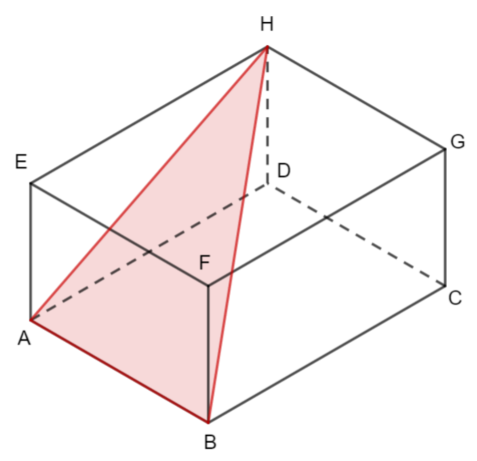
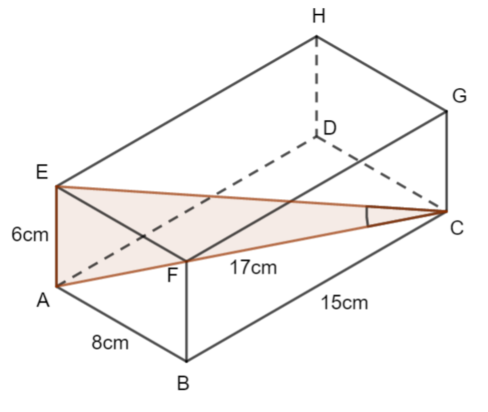
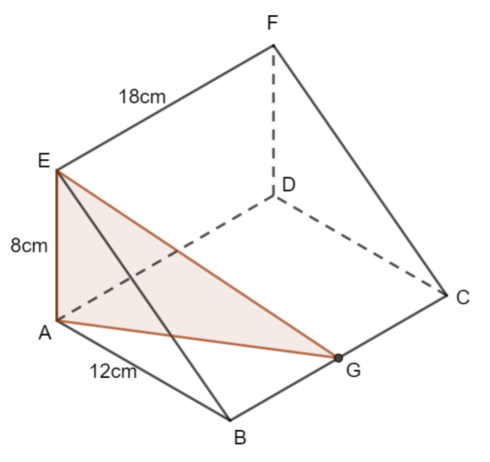
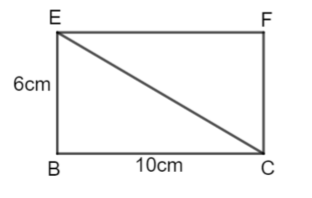 a) WE can find the length EC using Pythagoras' Theorem
a) WE can find the length EC using Pythagoras' Theorem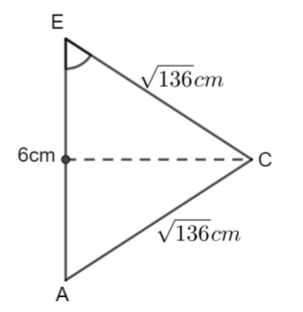 b) The length AC is the same as EC
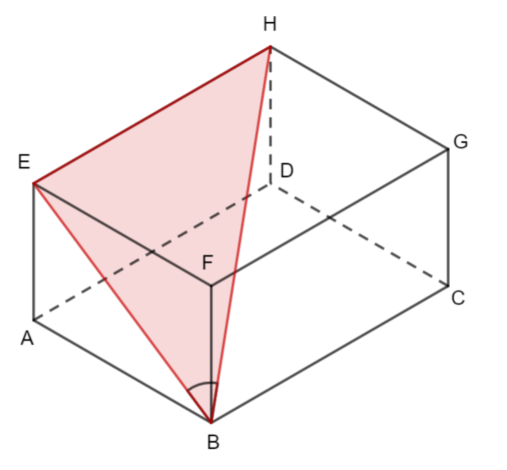
b) The length AC is the same as EC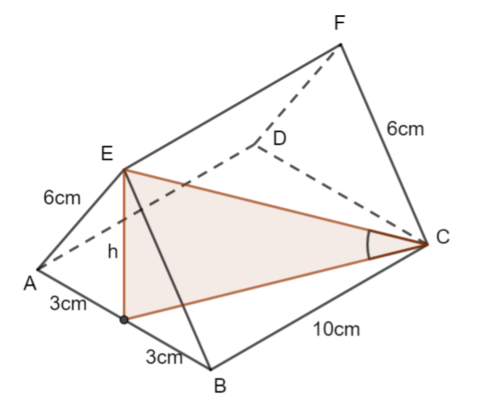 c) The angle between EC and the plane ABCD can be visualised in this diagram.
c) The angle between EC and the plane ABCD can be visualised in this diagram. Twitter
Twitter  Facebook
Facebook  LinkedIn
LinkedIn