 This page is all about how planes meet (or not). Two planes will either be parallel or meet at a line. Three planes will either 1) not meet - zero solutions, 2) meet at a point - unique solution, or 3) meet at a line (or plane) - infinite solutions. We will look at how to recognise each situation and how to find the solutions. This work overlaps with finding solutions to systems of linear equations.
This page is all about how planes meet (or not). Two planes will either be parallel or meet at a line. Three planes will either 1) not meet - zero solutions, 2) meet at a point - unique solution, or 3) meet at a line (or plane) - infinite solutions. We will look at how to recognise each situation and how to find the solutions. This work overlaps with finding solutions to systems of linear equations.
On this page, you should learn about
- the intersection of two planes
- the intersection of three planes (unique, infinite and zero solutions)
The following videos will help you understand all the concepts from this page
Print from here
How much of Intersection of Planes have you understood?


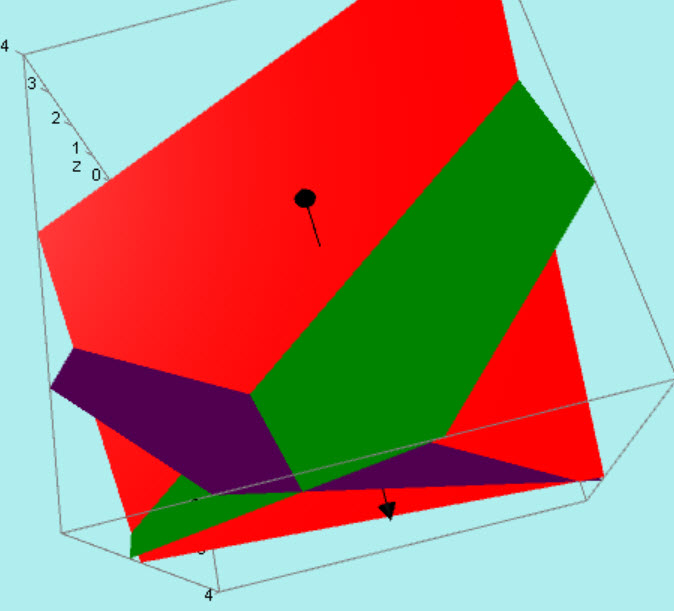
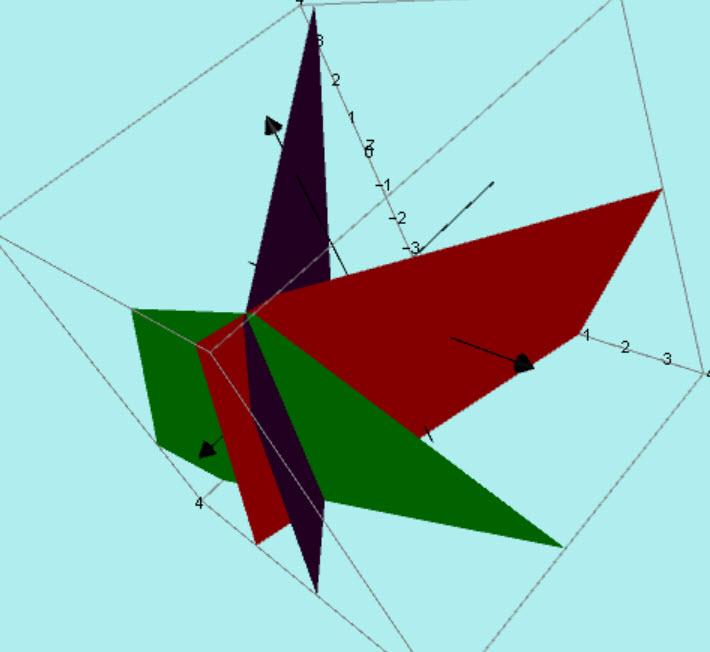
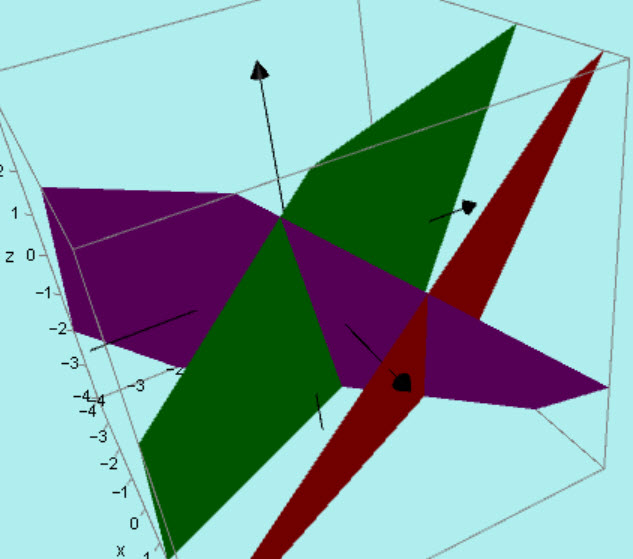
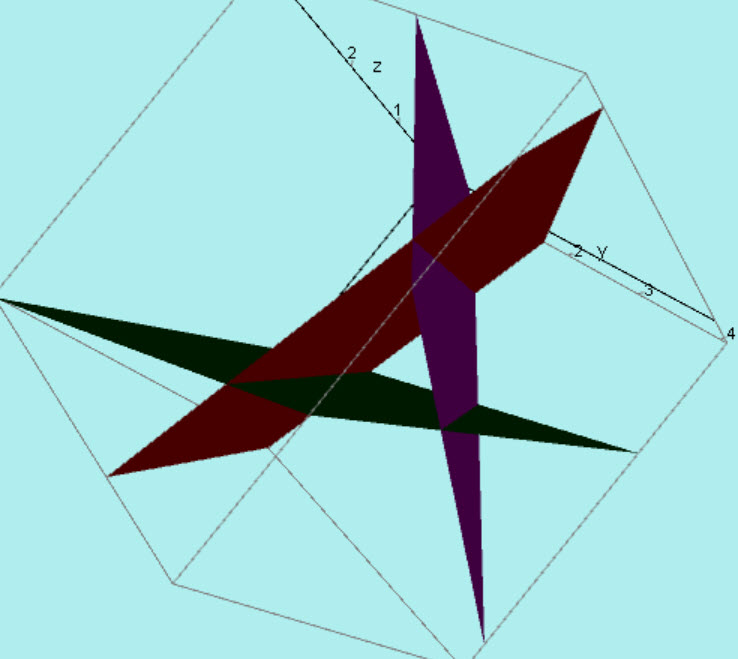

 Find the intersection of the planes
Find the intersection of the planes 
 Twitter
Twitter  Facebook
Facebook  LinkedIn
LinkedIn