 In this page, we will learn about how to find the volume generated by rotating a region around the x axis and the y axis. The formule for these are not difficult to use. The difficulty often comes with applying the integration techniques. It is therefore recommended that you revise these techniques before you go through this topic. In particular, you should be confident with Integration by Substitution and Integration by Parts.
In this page, we will learn about how to find the volume generated by rotating a region around the x axis and the y axis. The formule for these are not difficult to use. The difficulty often comes with applying the integration techniques. It is therefore recommended that you revise these techniques before you go through this topic. In particular, you should be confident with Integration by Substitution and Integration by Parts.
On this page, you should learn about
- finding the volume generated by rotating a region under a curve about the x axis/y axis
- finding the volume generated by rotating a region bounded by two graphs about the x axis/y axis
Here is a quiz that practises the skills from this page
START QUIZ!
Volume of Revolution 1/10
The following graph shows the curve y = f(x)
The region bounded by the curve and the lines y = 0 and x = a and x = b is rotated \(\large 2\pi\) around the x axis.
Which of the following represents the volume of this solid?
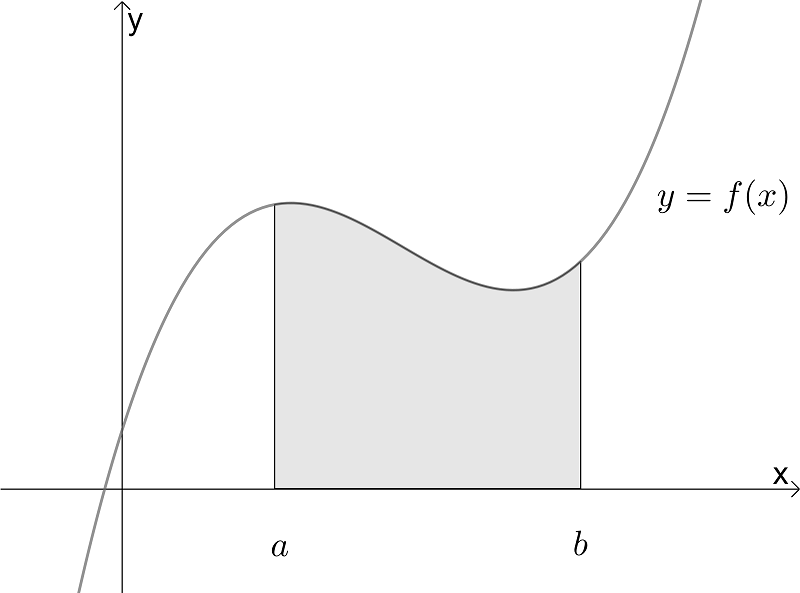
The volume of a solid rotated around the x axis is \(\large V=\pi\int_{a}^{b}[f(x)]^2 \,dx \)
The following graph shows the curve \(\large y = x^2\)
The region bounded by the curve and the lines x = 0 and y = 0 and y = a is rotated \(\large 2\pi\) around the y axis.
Which of the following represents the volume of this solid?

The volume of a solid rotated around the y axis is \(\large V=\pi\int_{a}^{b}x^2 \,dy \)
Since y = x², then \(\large V=\pi\int_{0}^{a}y \,dy \)
The following graph shows the curve \(\large f(x) = 2x(1-x)\) and \(\large g(x) = x(1-x)\)
The shaded region is rotated \(\large 2\pi\) around the x axis.
Which of the following represents the volume of this solid?

To find the volume of a solid generated from the region between two curves, you find the volume generated from the "upper" curve and subtract the volume generated from the "lower" curve.
In this case, the volume generated from the "upper" curve is \(\large V_{upper}=\pi\int_{0}^{1}[2x(1-x)]^2 \,dx\)
and the volume generated from the "lower" curve is \(\large V_{lower}=\pi\int_{0}^{1}[x(1-x)]^2 \,dx\)
The region bounded by the line y= x and the lines y = 0 and x = 1 and x = 2 is rotated \(\large 2\pi\) around the x axis.
Which of the following is the volume of this solid?

\(\large V=\pi\int_{1}^{2}x^2 \,dx \\ \large V=\pi[\frac{x^3}{3}]_{1}^{2} \\ \large V=\pi([\frac{2^3}{3}]-[\frac{1^3}{3}]) \\ \large V=\pi([\frac{8}{3}]-[\frac{1^3}{3}]) \\ \large V=\frac{7}{3}\pi\)
The following graph shows the curve \(\large f(x)=\sqrt{x}\)
The region bounded by the curve and the lines y = 0 and x = 0 and x = 2 is rotated \(\large 2\pi\) around the x axis.
If the volume is \(\large a\pi\) , find the value of a
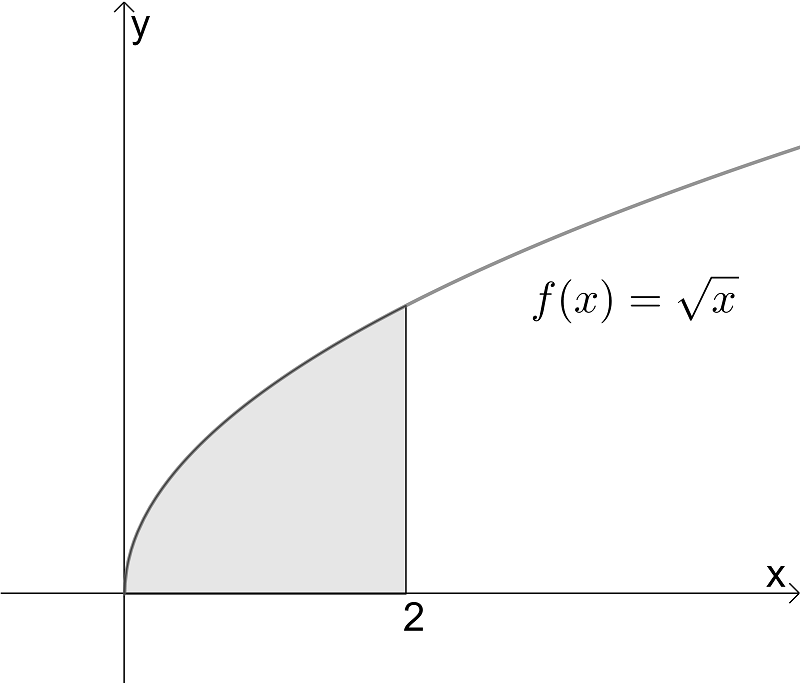
a =
\(\large V=\pi\int_{0}^{2}(\sqrt{x})^2 \,dx \\ \large V=\pi\int_{0}^{2}x \,dx \\ \large V=\pi[\frac{x^2}{2}]_{0}^{2} \\ \large V=\pi([\frac{2^2}{2}]-[\frac{0^2}{2}]) \\ \large V=2\pi\)
The following graph shows the curve \(\large f(x)=\frac{1}{x}\)
The region bounded by the curve and the lines y = 0 and x = 1 and x = 4 is rotated \(\large 2\pi\) around the x axis.
If the volume is \(\large a\pi\) , find the value of a
Give your answer as a decimal
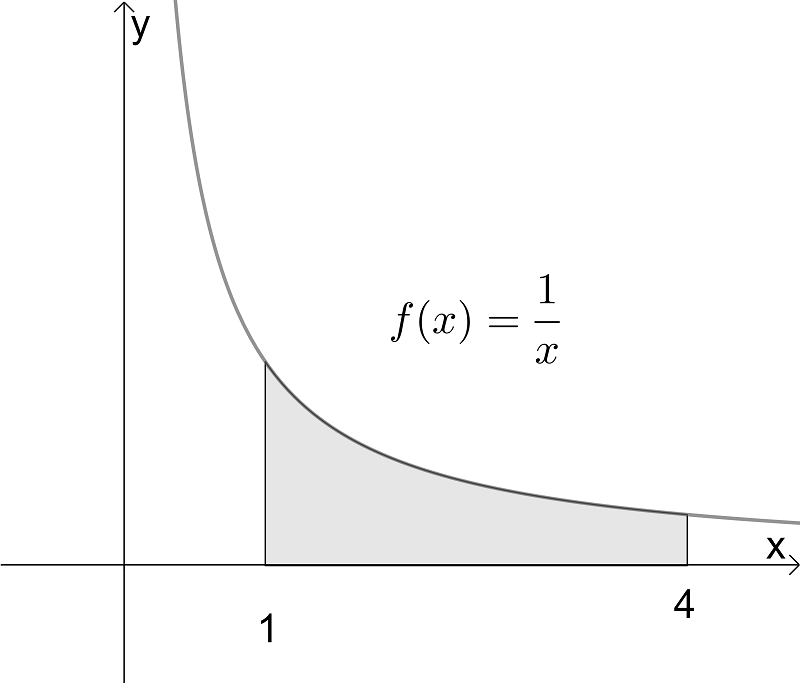
a =
\(\large V=\pi\int_{1}^{4}(\frac{1}{x})^2 \,dx \\ \large V=\pi\int_{1}^{4}x^{-2} \,dx \\ \large V=\pi[\frac{x^{-1}}{-1}]_{1}^{4} \\ \large V=\pi[\frac{-1}{x}]_{1}^{4} \\ \large V=\pi([\frac{-1}{4}]-[\frac{-1}{1}]) \\ \large V=\frac{3}{4}\pi\\ \large V=0.75\pi\\\)
The following graph shows the curve \(\large y = \frac{1}{x^2}\)
The region bounded by the curve and the lines x = 0 and y = 1 and y = 2 is rotated \(\large 2\pi\) around the y axis.
Which of the following represents the volume of this solid?

The volume of a solid rotated around the y axis is \(\large V=\pi\int_{a}^{b}x^2 \,dy \)
Since \(\large y = \frac{1}{x^2}\), then \(\large V=\pi\int_{1}^{2}\frac{1}{y} \,dy \)
\(\large V=\pi\int_{1}^{2}\frac{1}{y} \,dy \\ \large V=\pi[\ln |x|]_{1}^{2} \\ \large V=\pi(\ln 2-\ln 1)\\ \large V=(\ln 2)\pi\\\)
The following graph shows the curve \(\large f(x)=\frac{1}{\sqrt{x}}\)
The region bounded by the curve and the lines y = 0 and x = 1 and x = \(\large e^3\) is rotated \(\large 2\pi\) around the x axis.
If the volume is \(\large a\pi\) , find the value of a
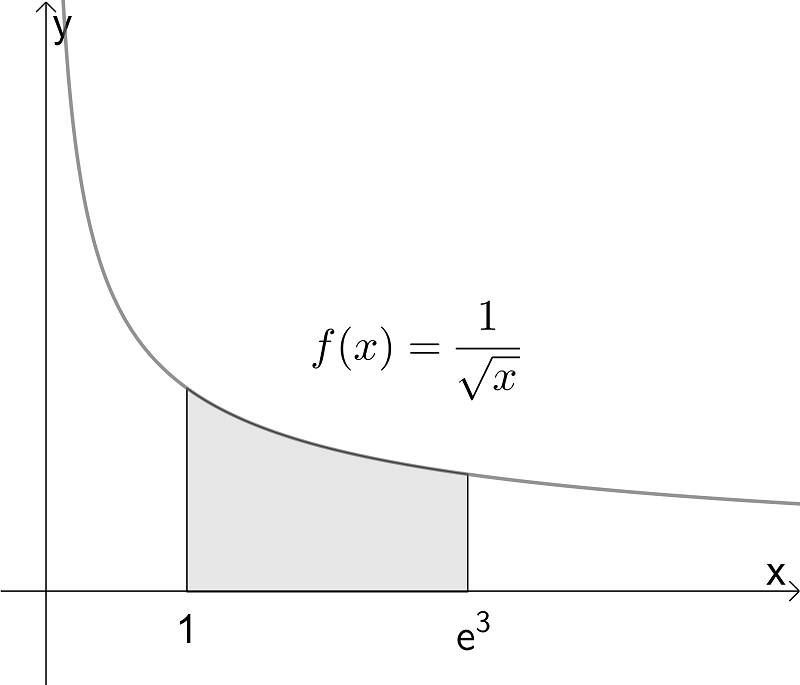
a =
\(\large V=\pi\int_{1}^{e^3}(\frac{1}{\sqrt{x}})^2 \,dx \\ \large V=\pi\int_{1}^{e^3}\frac{1}{x} \,dx \\ \large V=\pi[\ln| x|]_{1}^{e^3} \\ \large V=\pi([\ln e^3-\ln 1]) \\ \large V=\pi(\ln e^3)\\ \large V=3\pi\)
The following graph shows the curve \(\large y = 2\ln x\)
The region bounded by the curve and the lines x = 0 and y = 0 and y = 1 is rotated \(\large 2\pi\) around the y axis.
Which of the following represents the volume of this solid?
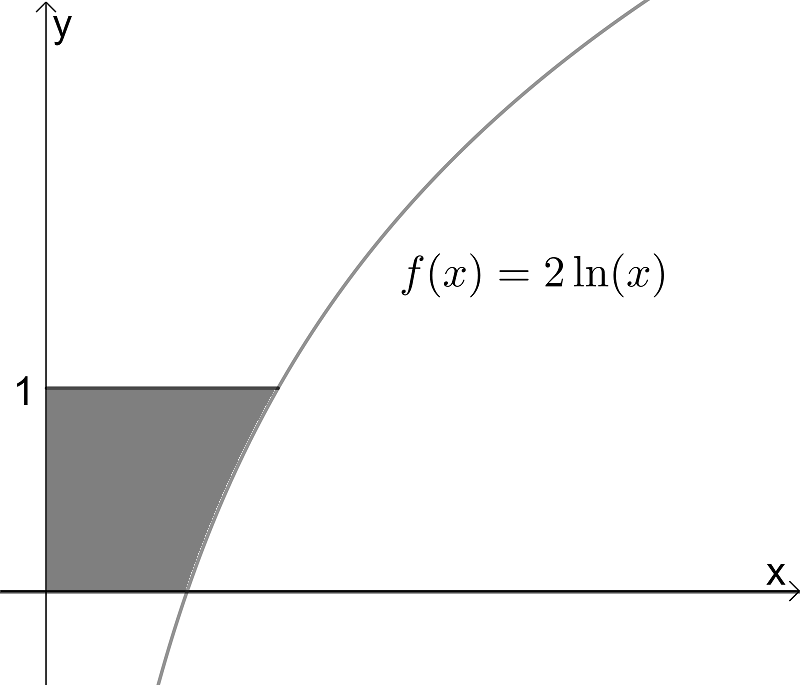
The volume of a solid rotated around the y axis is \(\large V=\pi\int_{a}^{b}x^2 \,dy \)
Since
\(\large y = 2\ln x\\ \large \frac{y}{2}=\ln x\\ \large e^{\frac{y}{2}}=x\\ \large (e^{\frac{y}{2}})^2=x^2\\ \large e^y=x^2\),
then
\(\large V=\pi\int_{0}^{1}e^y \,dy \)
\(\large V=\pi[e^y]_{0}^{1} \\ \large V=\pi(e^1-e^0)\\ \large V=(e-1)\pi\)
The following graph shows the curve \(\large y=\sin x\)
The region bounded by the curve and the lines y = 0 and x = 0 and x = \(\frac{\pi}{2}\) is rotated \(\large 2\pi\) around the x axis.
If the volume is \(\large \frac{\pi^2}{a}\) , find the value of a

a =
\(\large V=\pi\int_{0}^{\frac{\pi}{2}}(\sin x)^2 \,dx\)
To integrate sin²x, we need to use the double angle formula for cos2x
\(\large \cos2x\equiv1-2\sin^2x\\ \large 2\sin^2x\equiv 1-\cos2x\\ \large \sin^2x\equiv \frac{1}{2}-\frac{\cos2x}{2}\)
\(\large V=\pi\int_{0}^{\frac{\pi}{2}}(\sin x)^2 \,dx\\ \large V=\pi\int_{0}^{\frac{\pi}{2}}(\frac{1}{2}-\frac{\cos2x}{2}) \,dx\\ \large V=\pi[\frac{x}{2}-\frac{\sin2x}{4}]_{0}^{\frac{\pi}{2}} \\ \large V=\pi([\frac{\frac{\pi}{2}}{2}-\frac{\sin\pi}{4}]-[\frac{0}{2}-\frac{\sin0}{4}])\\ \large V=\pi(\frac{\pi}{4})\\ \large V=\frac{\pi^2}{4}\)
Exam-style Questions

a) Show that \(\large \int {x^2e^{2x}\,dx}=\frac{1}{4}e^{2x}(2x^2-2x+1)+c\)
The following graph shows the function \(\large f(x)=xe^x\)

b) Show that the equation of the tangent to the graph at x = 1 has the equation \(\large y=(2e)x-e\)
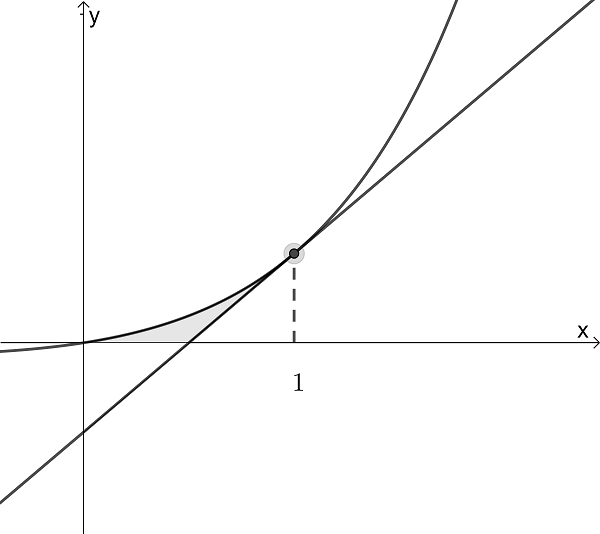
The region bounded by \(\large f\), the tangent \(\large y=(2e)x-e\) and y = 0 is rotated \(\large 2\pi\) around the x axis
c) Find the volume of this solid in terms of \(\large \pi\)
Hint
a) this is an integration by parts question
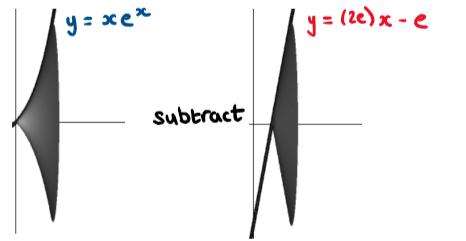
a) this is an integration by parts question

Full Solution
a)
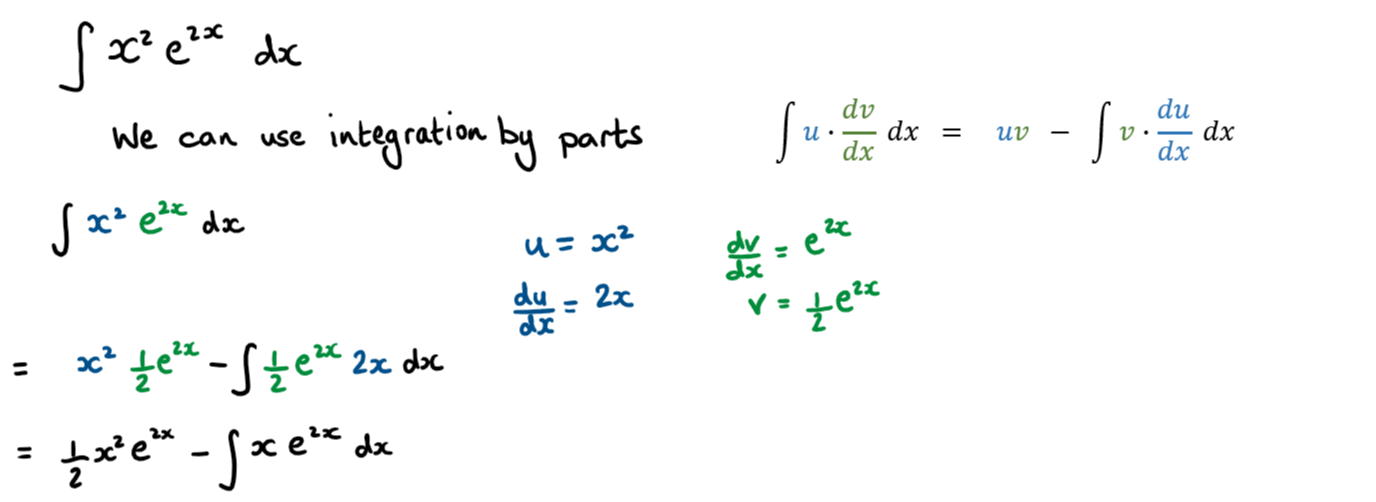
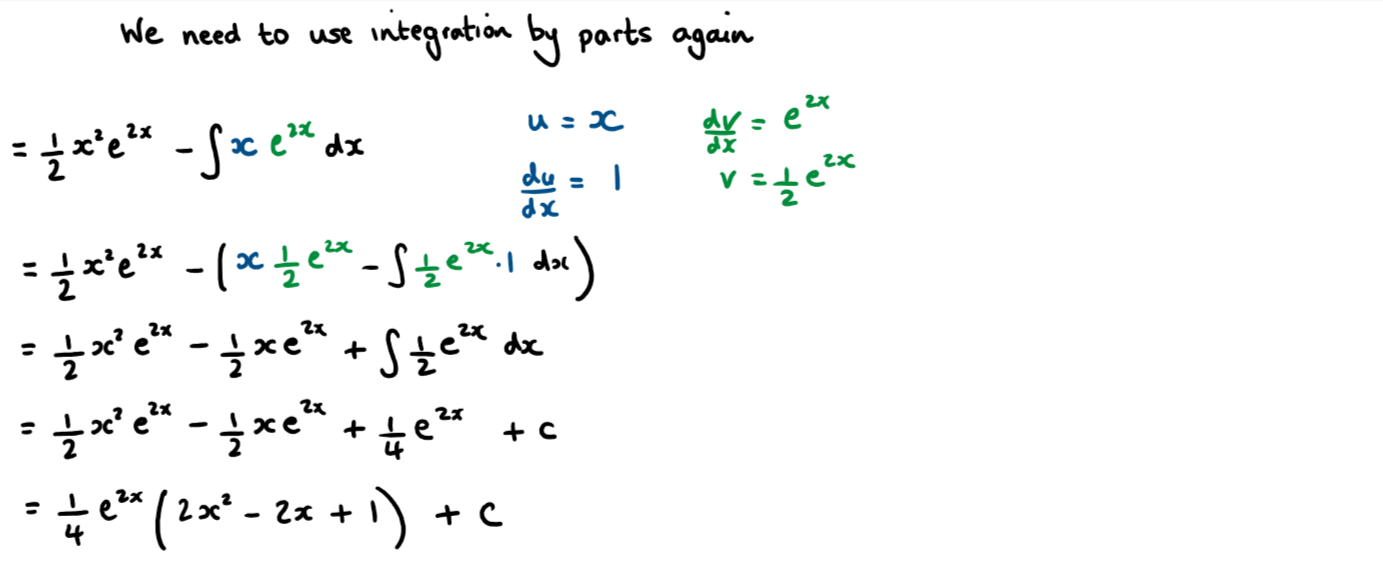
b)

c)
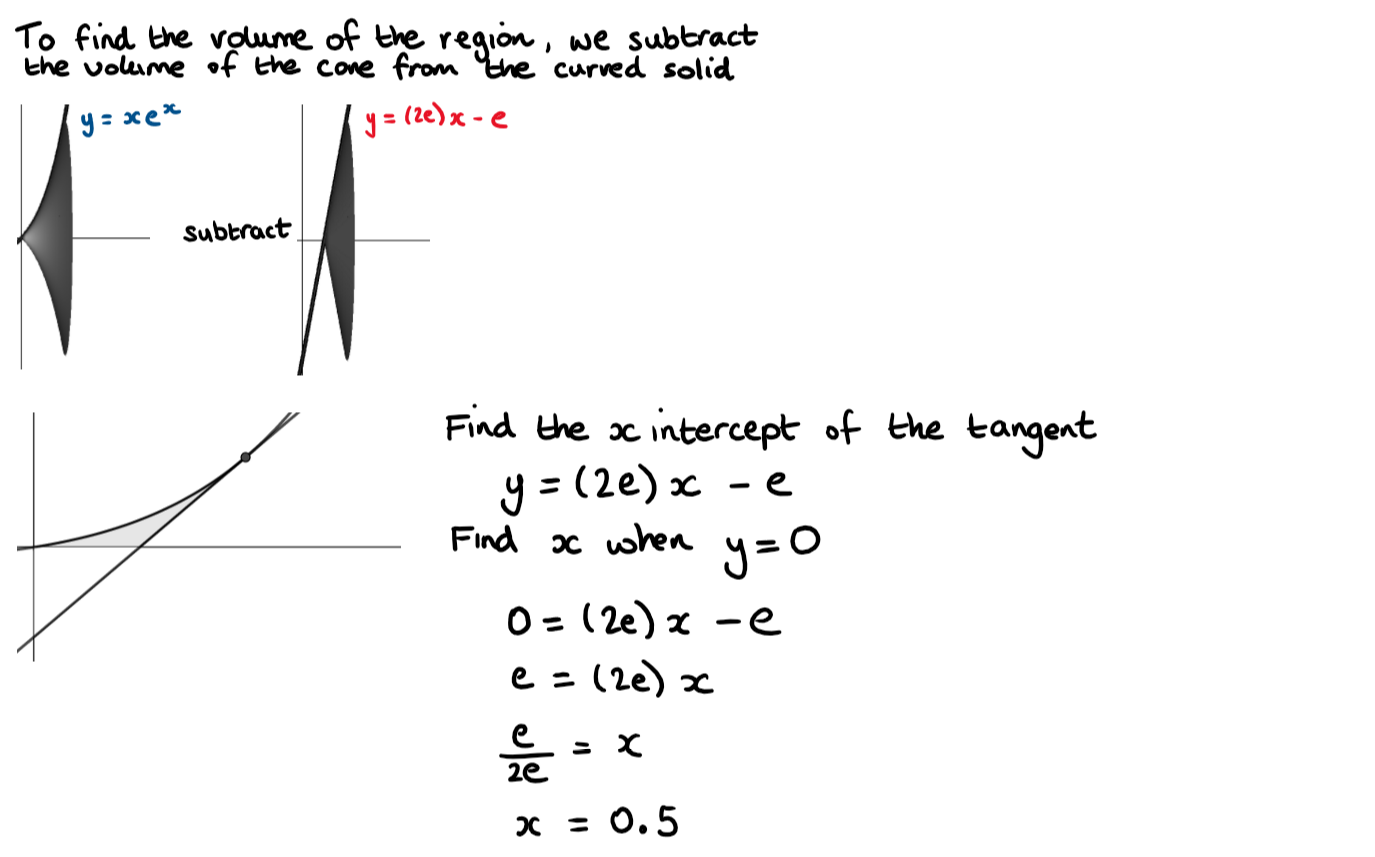

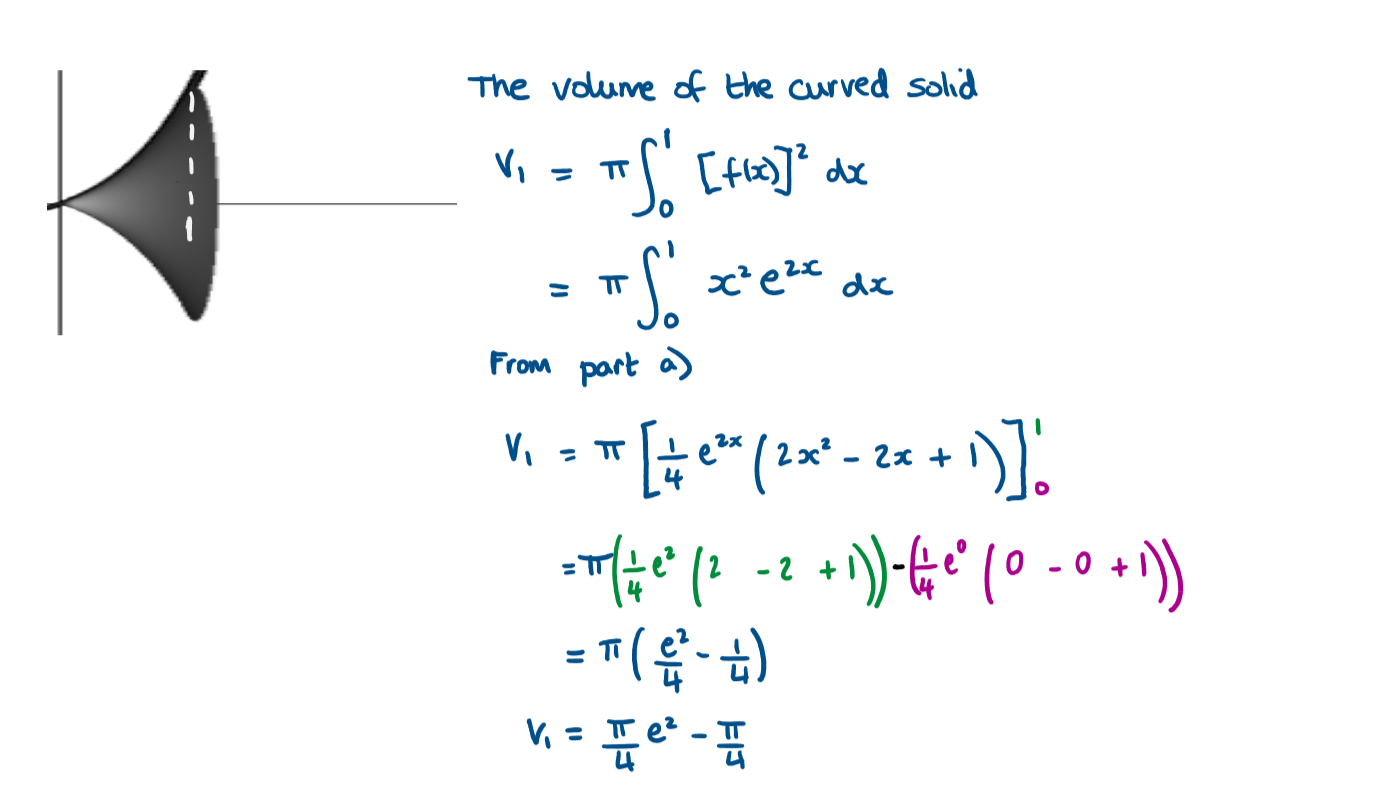

a)
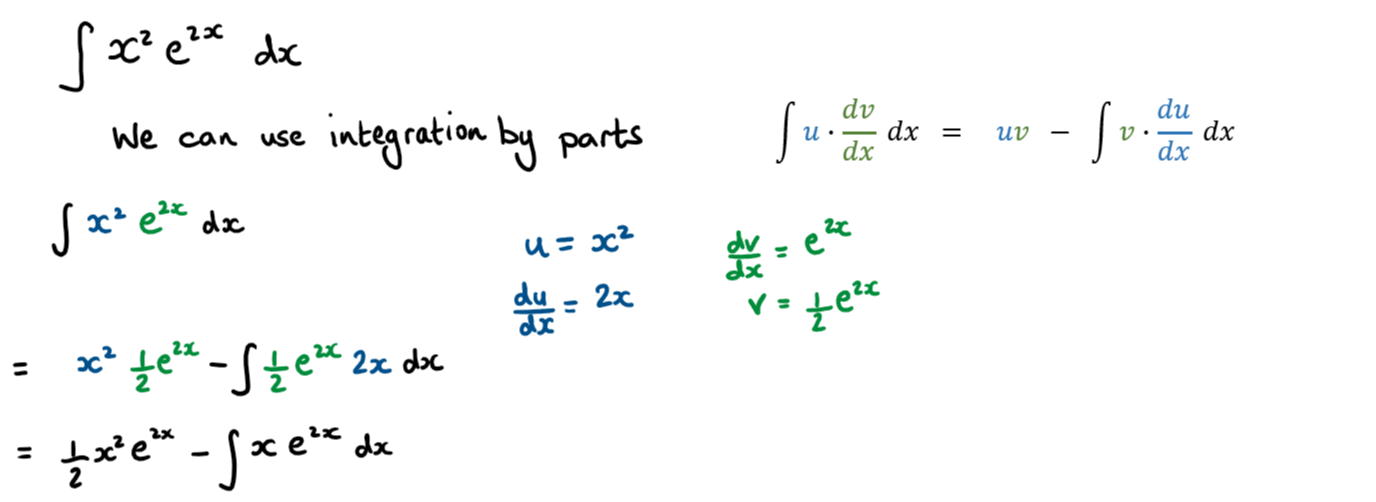

b)

c)


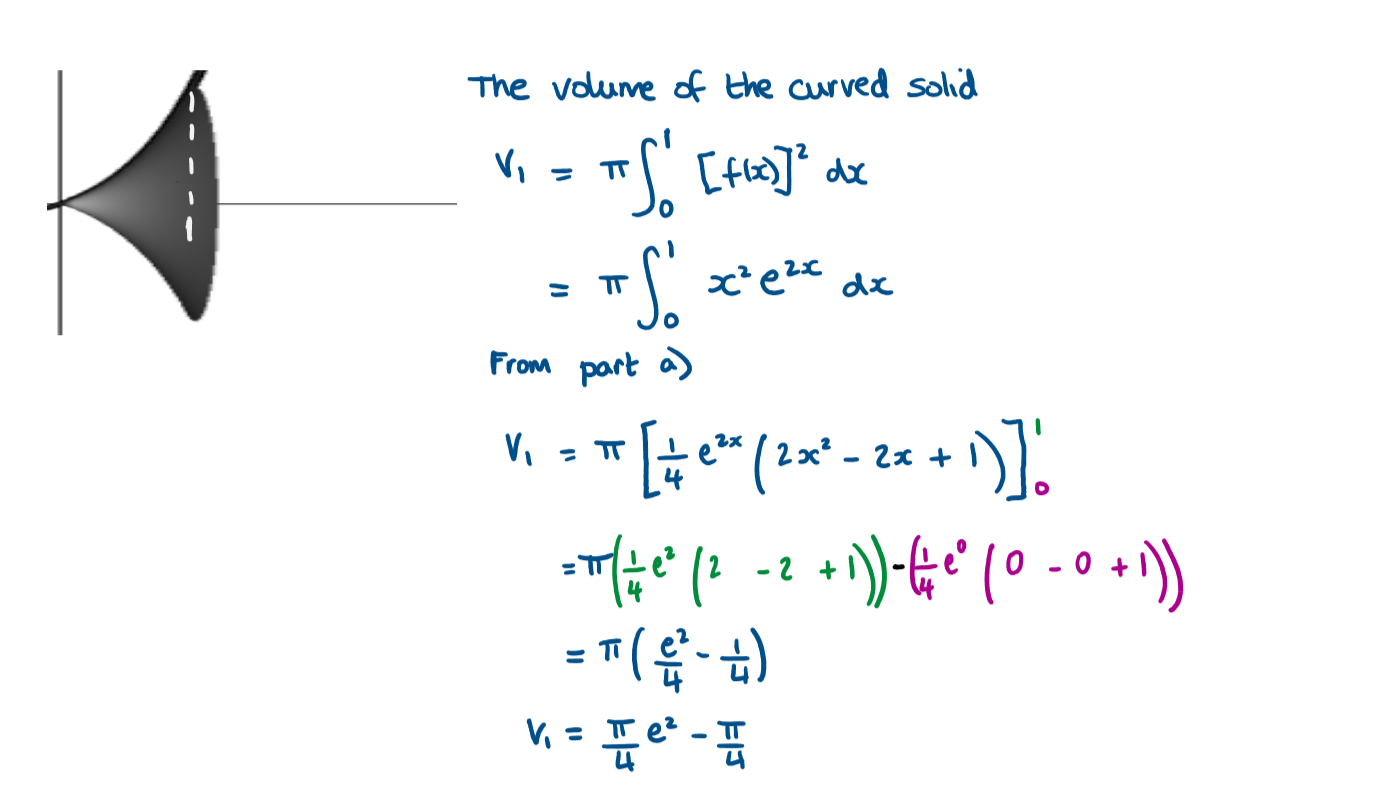


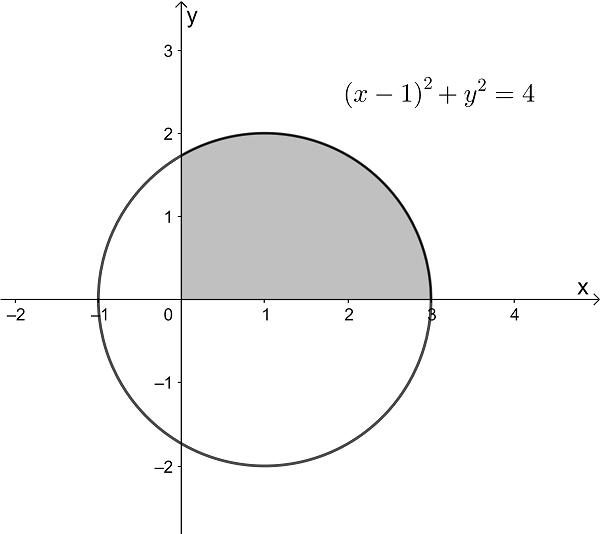
The following graph shows the curve defined by the equation \(\large (x-1)^2+y^2=4\).
The region bounded by the curve and the lines y = 0 and x = 0 is rotated \(\large 2\pi\) around the x axis.
Find the volume of this solid in terms of \(\large \pi\)
Hint
The volume of the solid is found using the formula
The volume of the solid is found using the formula
Full Solution
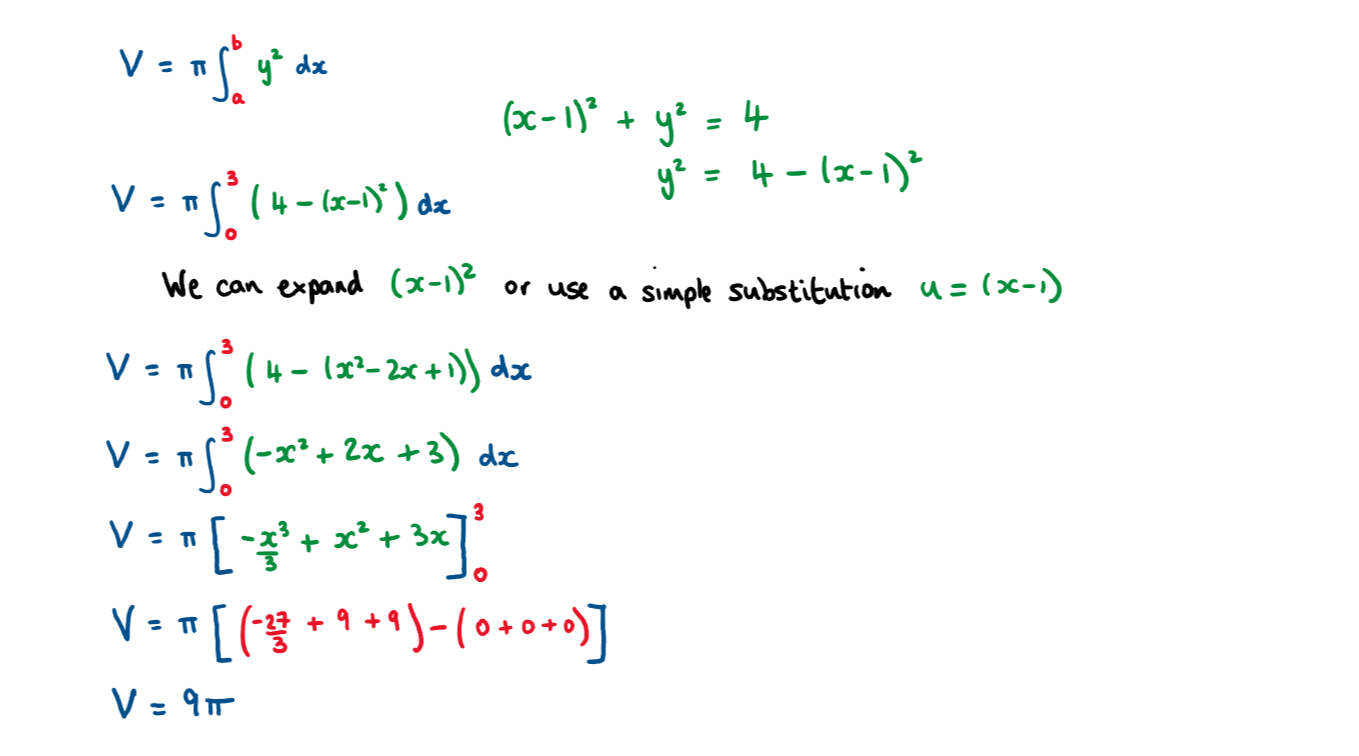
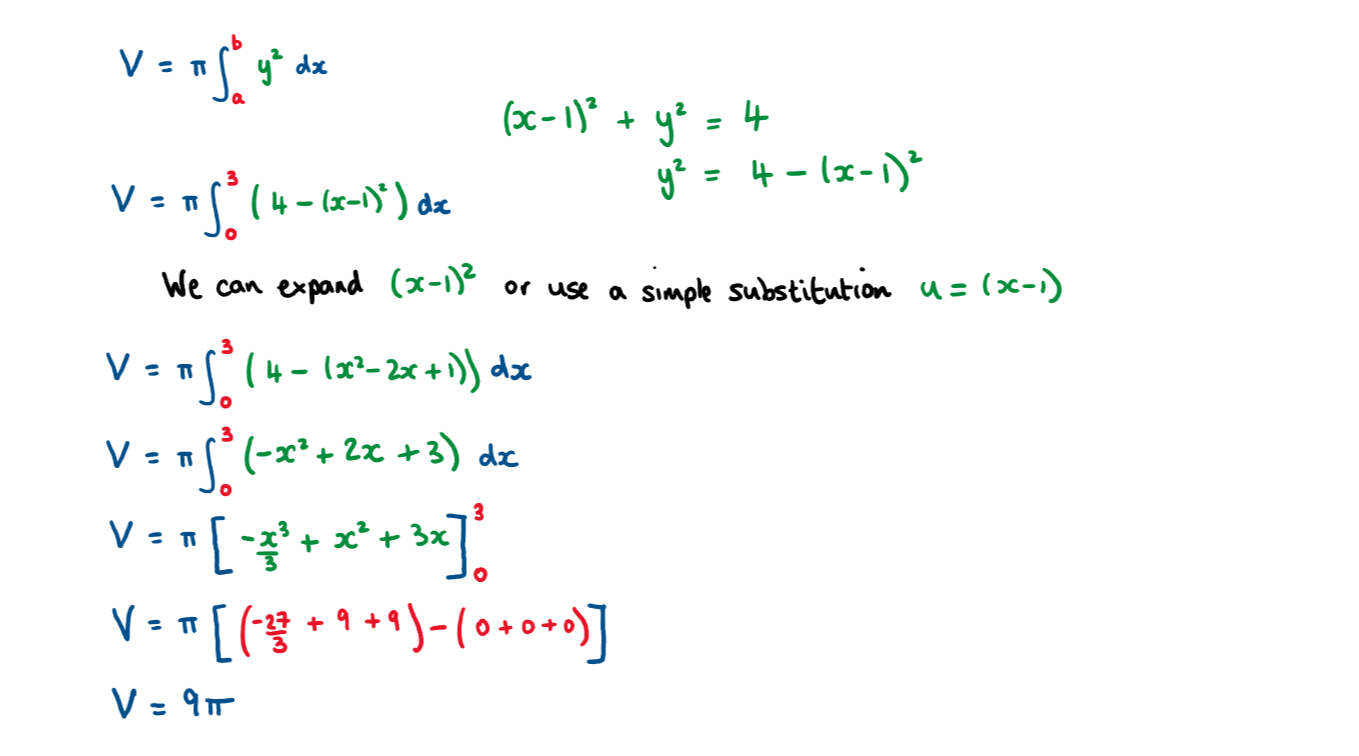

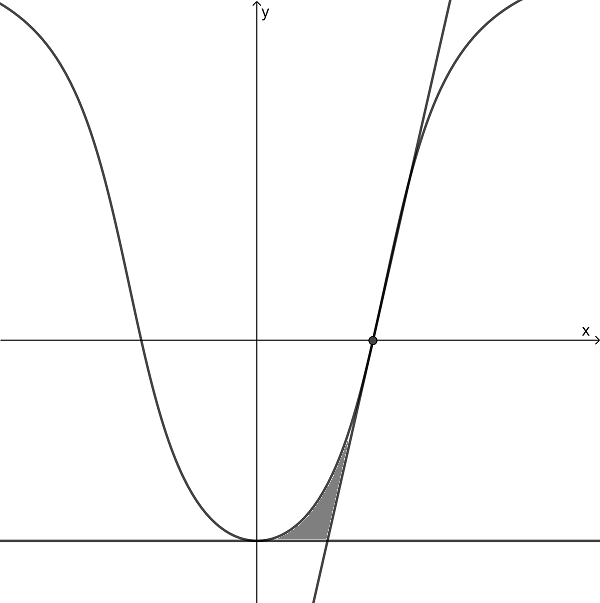
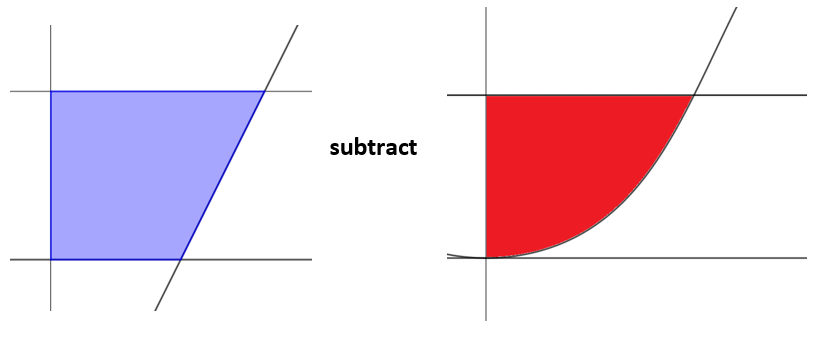
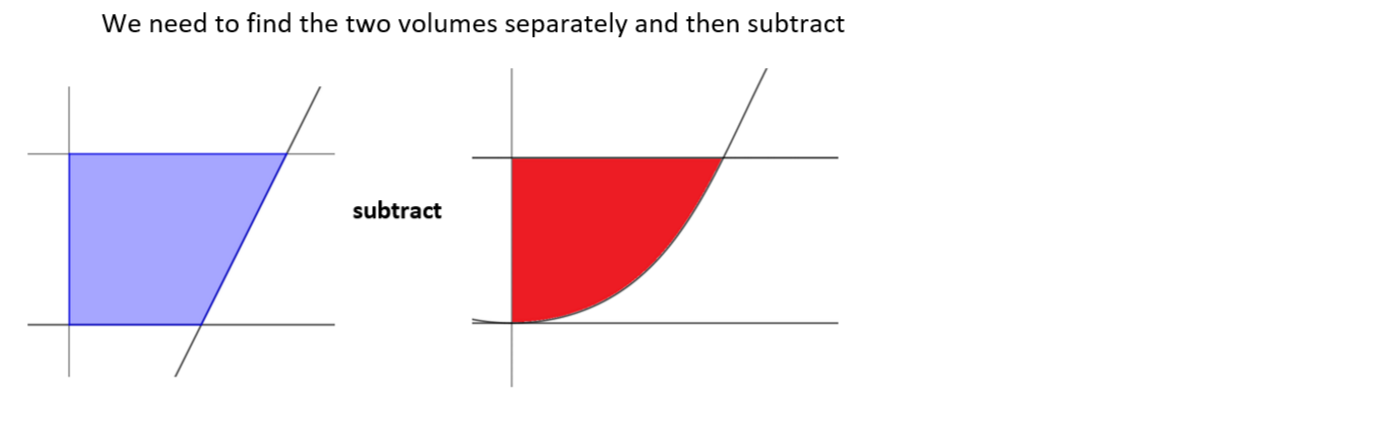
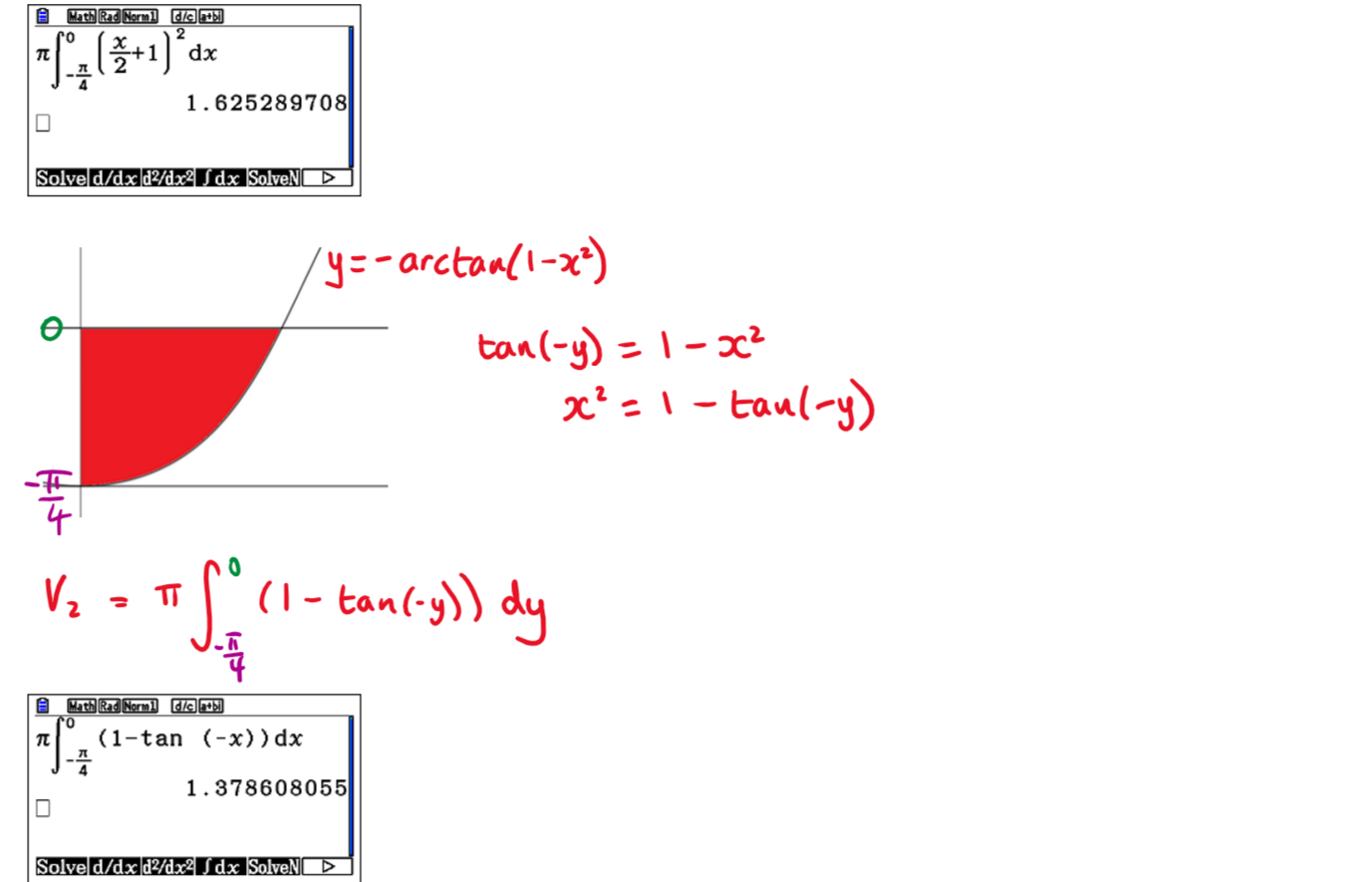
The graph shows \(\large f(x) = -\arctan(1-x^2)\), the tangent to the curve at (1 , 0) and the tangent to the curve at the point \(\large(-\frac{\pi}{4},0)\).
The shaded region is bounded by the curve and the two tangents. This region is rotated \(\large 2\pi\) around the y axis to forma solid.
Find the volume of this solid correct to 3 significant figures.
Hint
You can use your calculator for this question.
Ensure that your calculator is in radian mode.
You can use your calculator for this question.
Ensure that your calculator is in radian mode.

Full Solution







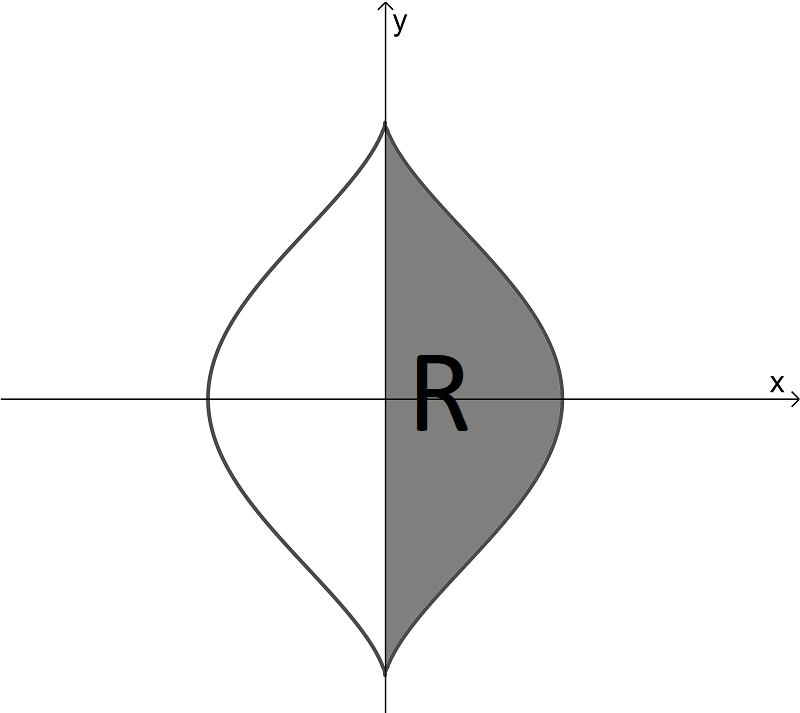
The following diagram shows the graph of \(\large x^2=\cos^3y\) for \(\large -\frac{\pi}{2}\le y\le\frac{\pi}{2}\)
The shaded region R is the area bounded by the curve, the y axis and the lines \(\large y=-\frac{\pi}{2}\) and \(\large y=\frac{\pi}{2}\).
The rgion is rotated about the y axis through \(\large 2\pi\) to form a solid.
Show that the volume of the solid is \(\large \frac{4\pi}{3}\)
Hint
To perfom the integration, write
\(\large \cos^3y=\cos y\cdot\cos^2y=\cos y\ (1-\sin^2y)\)
To perfom the integration, write
\(\large \cos^3y=\cos y\cdot\cos^2y=\cos y\ (1-\sin^2y)\)
Full Solution





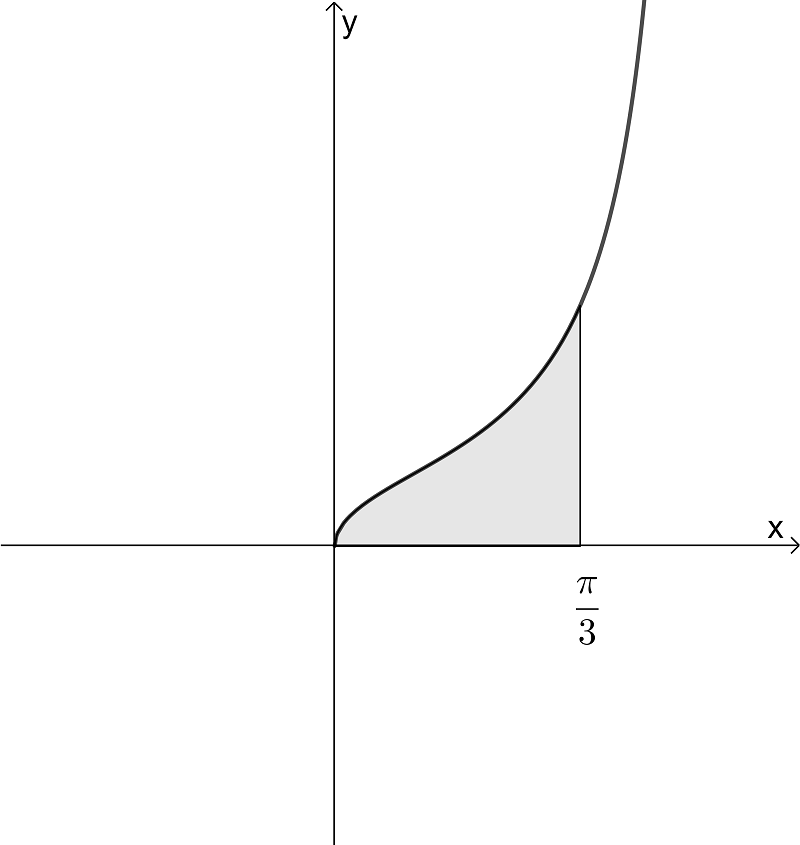
The following diagram shows the graph of the function \(\large f(x)=\frac{\sqrt{x}}{\cos x}\) for \(\large 0\le x \le\frac{\pi}{2}\)
The shaded region is the area bounded by f, the x axis, x= 0 and x = \(\large \frac{\pi}{3}\)
The region is rotated about the x axis through \(\large 2\pi\) to form a solid.
Find the volume of the solid.
Hint
It is the integration that makes this question difficult.
For the integration by substitution, let
\(\large u=x \qquad \frac{dv}{dx}=\sec^2x\)
It is the integration that makes this question difficult.
For the integration by substitution, let
\(\large u=x \qquad \frac{dv}{dx}=\sec^2x\)
Full Solution
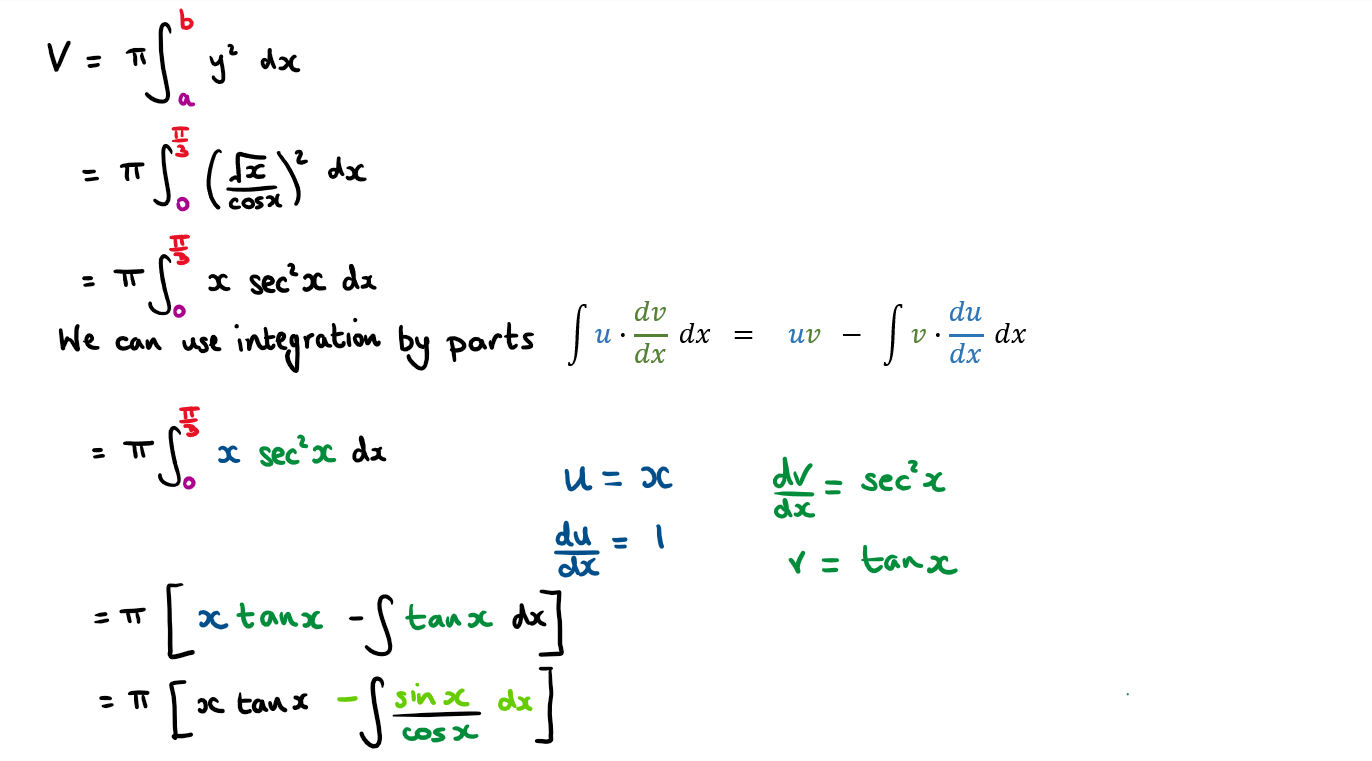

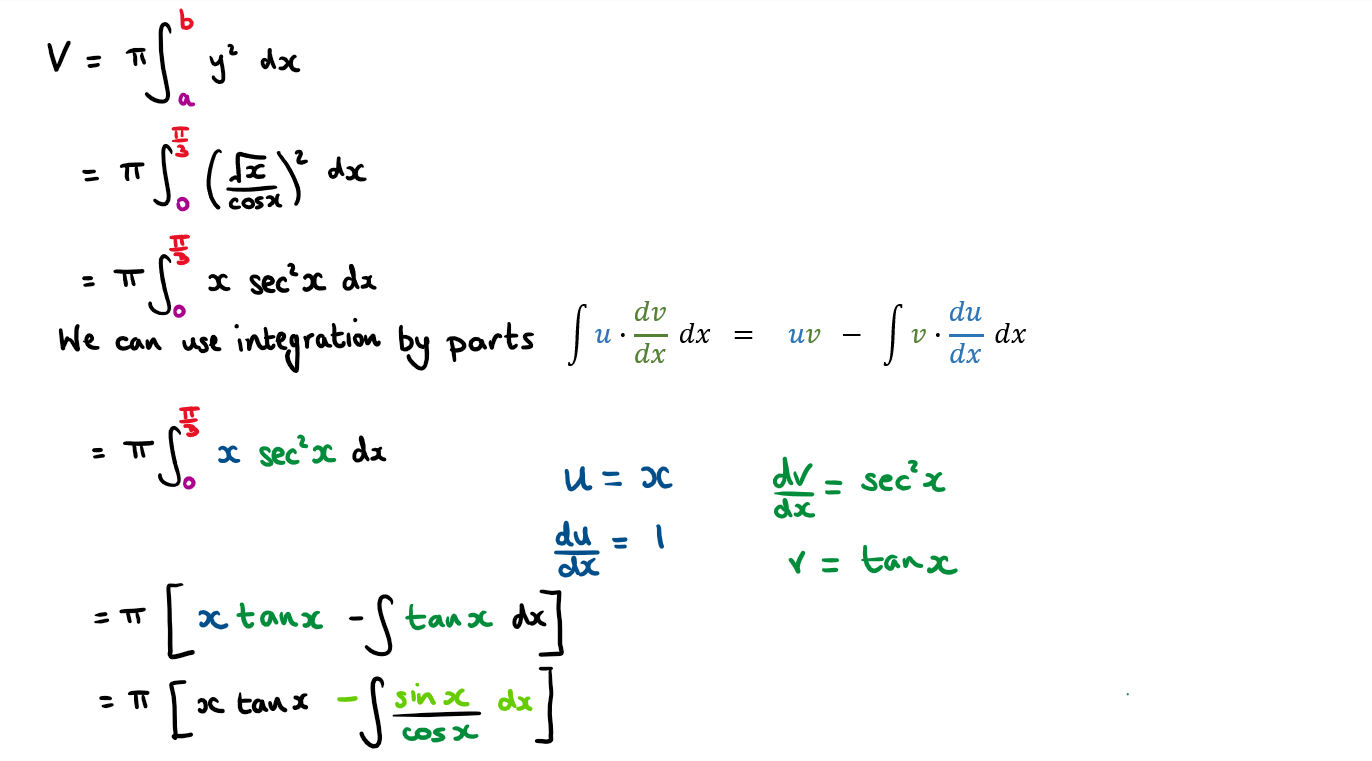

Feedback
Which of the following best describes your feedback?




 Twitter
Twitter  Facebook
Facebook  LinkedIn
LinkedIn