On this page you can find examination questions from the topic of geometry and trigonometry



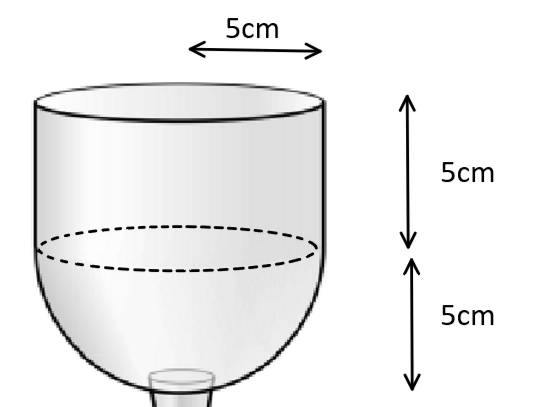
A glass is made up of a hemisphere and a cone.
Find the volume of the glass.
Give your answer to 3 significant figures
Hint
The radius of the cone and the hemisphere are both 5cm
Full Solution
 The radius of the cone and the hemisphere are both 5cm
The radius of the cone and the hemisphere are both 5cm
Volume of cone,
\(\large V_{cone}=\pi r^2h\\ \large V_{cone}=\pi \times5^2\times 5\\ \large V_{cone}=125\pi \)
Volume of hemisphere,
\(\large V_{hemisphere}=2\pi r^2\\ \large V_{hemisphere}=2\times \pi \times 5^2\\ \large V_{hemisphere}=50\pi\)
Total Volume\(\large=125\pi+50\pi\\ \large=175\pi\\ \large\approx549.779...cm^3\\ \large\approx550cm^3\)




The total surface area of a hemisphere is 1360 cm²
Find the radius.
Give your answer to 3 significant figures.
Hint
The surface of a hemisphere is made of two parts
- the curved surface
- the circular base
Full Solution
The surface area of a hemisphere,
\(\large A=\pi r^2+\pi r^2\\ \large A=3\pi r^2\\ \large 1360=3\pi r^2\\ \large r^2=\frac{1360}{3\pi}\\ \large r=\sqrt{\frac{1360}{3\pi}}\\ \large r\approx12.0125...\\ \large r\approx12.0cm \)


a) A sphere has a radius of 10cm. Find the volume, giving your answer in terms of \(\large \pi\).
b) A cone has the same volume and the same radius as the sphere. Find the height of the cone.
c) Another sphere and cone have the same volume and the same radius, r. Find an equation for the height of the cone, h in terms of r.

Hint
c) Solve \(\large \frac{4}{3}\pi r^3=\frac{1}{3}\pi r^2h\\\)
Full Solution
a)
\(\large V=\frac{4}{3}\pi r^3\\ \large V=\frac{4}{3}\pi \times10^3\\ \large V=\frac{4000}{3}\pi \ cm^3\)
b)
\(\large V=\frac{1}{3}\pi r^2h\\ \large \frac{4000}{3}\pi=\frac{1}{3}\pi \times 10^2\times h\\ \large h=40\ cm\)
c)
\(\large \frac{4}{3}\pi r^3=\frac{1}{3}\pi r^2h\\ \large \frac{4}{\rlap{/}3}\rlap{/}\pi r^3=\frac{1}{\rlap{/}3}\rlap{/}\pi r^2h\\ \large 4r^3=r^2h\\ \large 4r=h\\ \large h=4r\)


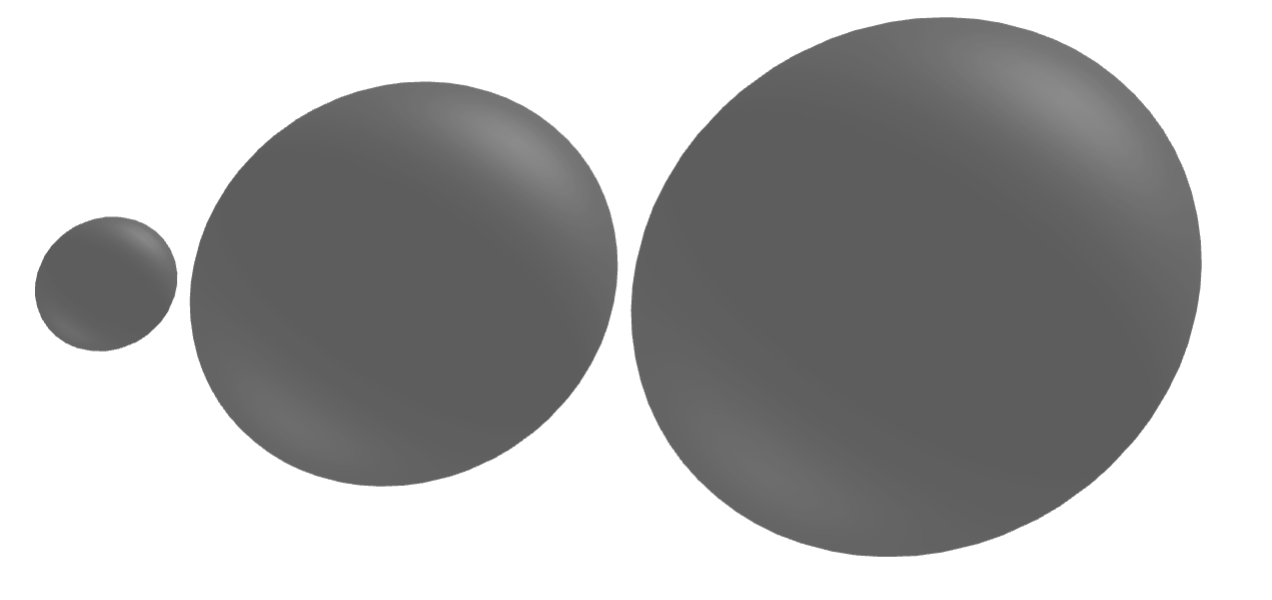
Three metal spheres have radii 1cm, 6cm and 8cm.
The spheres are melted down and made into one bigger sphere.
What is the radius of the single sphere?
Hint
Full Solution
The volume of a sphere is given by \(\large V=\frac{4}{3}\pi r^3\)
The Volume of the three spheres is
\(\large V=\frac{4}{3}\pi \times1^3+\frac{4}{3}\pi \times 6^3+\frac{4}{3}\pi \times 8^3\\ \large V=\frac{4}{3}\pi \times(1^3+6^3+8^3)\\ \large V=\frac{4}{3}\pi \times(729)\\ \)
The large sphere has the same volume as these three spheres
\(\large \frac{4}{3}\pi \times R^3=\frac{4}{3}\pi \times(729)\\ \large R^3=729\\ \large R=9\ cm \)


A cylindrical metal bar with height 12cm and diameter 12cm is melted down and made into spheres of diameter 3cm.
How many spheres will it make?
Hint
Full Solution
The volume of the cylindrical bar is
\(\large V=\frac{1}{3}\pi\times 6^2\times12\\ \large V=144\pi \)
The volume of one of the spheres is
\(\large V=\frac{4}{3}\pi\times 1.5^3\\ \large V=4.5\pi \)
Therefore, the number of spheres is \(\large \frac{144\pi}{4.5\pi}\)
\(\large 32\) spheres
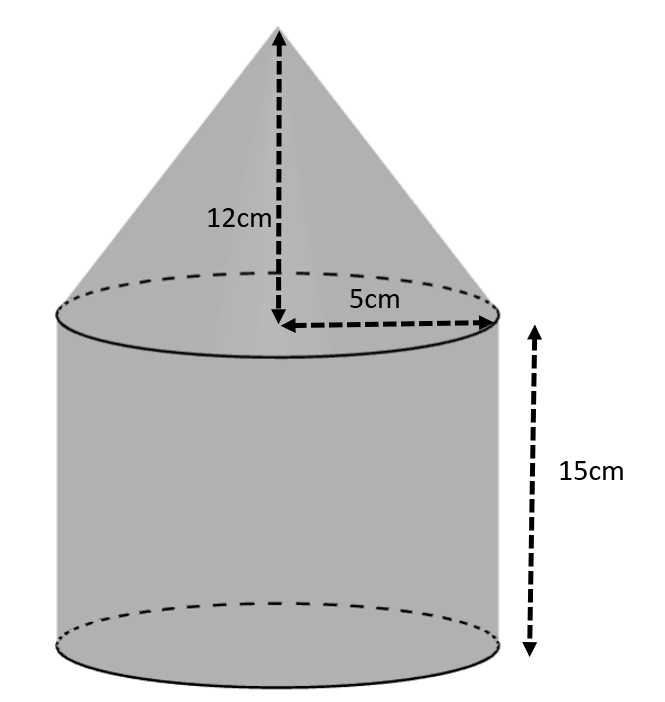


A solid is made up of a cone and a cylinder.
The radius is 5cm, the height of the cone is 12cm and the height of the cylinder is 15cm.
Show that the total surface area of the solid is \(\large 240\pi\)
Hint
There are three surfaces to find: the curved surface of the cone, the curved surface of the cylinder and the circular base.
To find the curved surface area of the cone, you need to find the slant height. Use Pythagoras' Theorem.
Full Solution
 We need to find the slant height of the cone:
We need to find the slant height of the cone:
\(\large l^2=5^2+12^2\\ \large l^2=169\\ \large l=13\)
The surface area of the cone is
\(\large A_{cone}=\pi rl\\ \large A_{cone}=\pi\times5\times 13\\ \large A_{cone}=65\pi\)
The surface area of the cylinder is
\(\large A_{cylinder}=2\pi rh\\ \large A_{cylinder}=2\pi \times 5\times 15\\ \large A_{cylinder}=150\pi\)
The area of the base is
\(\large A_{base}=\pi r^2\\ \large A_{base}=\pi \times 5^2\\ \large A_{base}=25\pi \)
The total surface area is
\(\large =65\pi+150\pi+25\pi\\ \large=240\pi\)



The following diagram shows a circle with centre O and radius 12cm. A and B lie on the circumference of the circle and \(\large AÔB=50°\)
a) Find the area of the minor sector OAB
b) Find the area of the triangle AOB
c) Hence, find the area of the shaded segment
Hint
Full Solution
a) The angle is given in degrees
\(\large A_{sector}= \frac{\theta}{360}\pi r^2\\ \large A_{sector}= \frac{50}{360}\pi \times 12^2\\ \large A_{sector}=20\pi\\ \large A_{sector}\approx62.831...\\ \large A_{sector}\approx62.8cm^2 \)
b) The area of the triangle is
\(\large A_{triangle}= \frac{1}{2} ab \sin C\\ \large A_{triangle}= \frac{1}{2} r^2 \sin \theta\\ \large A_{triangle}= \frac{1}{2} \times12^2 \times\sin 50°\\ \large A_{triangle}\approx55.155...\\ \large A_{triangle}\approx55.2cm^2 \)
c) The area of the segment = area of sector - area of triangle
Be careful to use a higher degree of accuracy for parts a) and b) to give the final answer correct to 3 s.f.
\(\large A_{sector}\approx62.831...-55.155...\\ \large A_{sector}\approx7.68cm^2\)



The following diagram shows a circle with centre O and radius r cm
The area of the shaded sector OAB is \(\large \frac{40\pi}{3}\) cm²
The length of the minor arc AB is \(\large \frac{10\pi}{3}\) cm
a) Find the radius of the circle
b) Find the angle \(\large \theta\) , in radians
Hint
Write an equation for the arc length and another equation for the sector area.
Full Solution
a) The area of the shaded sector OAB is \(\large \frac{40\pi}{3}\) cm²
\(\large A=\frac{1}{2}r^2\theta\\ \large \frac{40\pi}{3}=\frac{1}{2}r^2\theta\\\)
The length of the minor arc AB is \(\large \frac{10\pi}{3}\) cm
\(\large l=r\theta\\ \large \frac{10\pi}{3}=r\theta\)
Let's solve these equations by substituting for \(\large r\theta\) into the area equation
\(\large \frac{1}{2}r^2\theta=\frac{40\pi}{3}\\\)
\(\large \frac{1}{2}r\times r\theta=\frac{40\pi}{3}\\\)
\(\large \frac{1}{2}r\times \frac{10\pi}{3}=\frac{40\pi}{3}\\\)
\(\large \frac{1}{2}r=4\)
r = 8 cm
We can now find the angle by substituting this value into the length equation
\(\large \frac{10\pi}{3}=8\times\theta\\ \large \theta=\frac{10\pi}{24}\\ \large \theta=\frac{5\pi}{12}\)


The following diagram shows a circle with centre O and radius 5cm and another circle with centre P and radius r. The two circles overlap meeting at points A and B. \(\large AÔP=45°\) and \(\large A\hat{P}O=30°\)

a) Show that \(\large r=5\sqrt{2}\) cm
b) Hence, show that the shaded area bounded by the two circles is \(\large \frac{25}{12}(7\pi-6-6\sqrt3)\) cm²
Hint
a) You can use the Sine Rule to find r.

Full Solution
a)
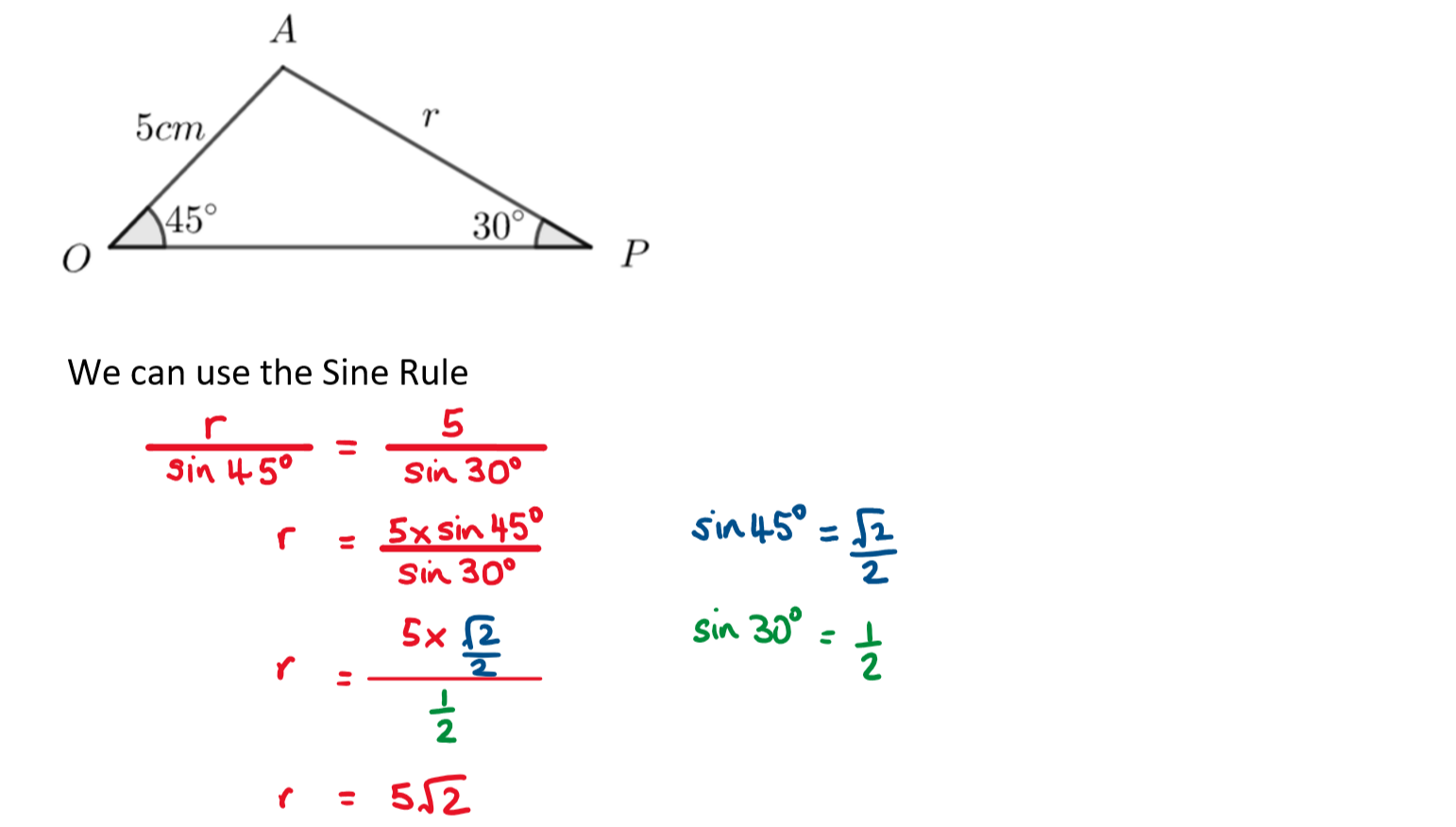
b) Divide the shaded area into two parts.






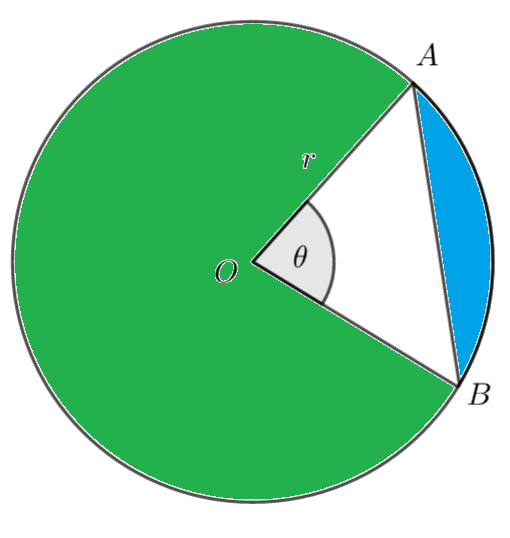
The following diagram shows a circle with centre O and radius r. A and B are points on the circumference of the circle and \(\large A\hat{O} B =\theta\) radians
The area of the green shaded region is three times greater than the area of the blue region.
a) Show that \(\large \sin \theta=\frac{4\theta-2\pi}{3}\)
b) Find the value of \(\large \theta\) , giving your answer correct to 3 significant figures.
Hint
a) The area of the blue segment = area of sector - area of triangle
Full Solution
a)

area of the blue segment = area of sector - area of triangle
\(\large A_{segment}=\frac{1}{2}r^2\theta-\frac{1}{2}r^2\sin\theta\)
 The angle subtended by the the green sector is \(\large 2\pi-\theta\)
The angle subtended by the the green sector is \(\large 2\pi-\theta\)
Area of the green sector is
\(\large A_{sector}=\frac{1}{2}r^2(2\pi-\theta)\)
The area of the green sector = three times the area of the blue segment
\(\large \frac{1}{2}r^2(2\pi-\theta)\) = 3 \(\large (\frac{1}{2}r^2\theta-\frac{1}{2}r^2\sin\theta)\)
\(\large \frac{1}{2}r^2(2\pi-\theta)=\frac{3}{2}r^2(\theta-\sin\theta)\)
\(\large 2\pi-\theta=3(\theta-\sin\theta)\\ \large 2\pi-\theta=3\theta-3\sin\theta\\ \large 3\sin\theta=4\theta-2\pi\\ \large \sin\theta=\frac{4\theta-2\pi}{3}\)
b) We can solve this equation using our graphical equation.You can plot the graphs of \(\large y=\sin\theta\\ \) and \(\large y=\frac{4\theta-2\pi}{3}\) and find the point of intersection
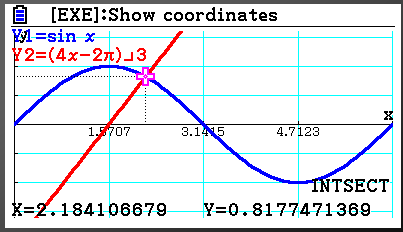
\(\large \theta \approx2.18\)
The diagram looks approaximately like the following



The following diagram shows a circle with centre O and radius r. Points A and B lie on the circumference of the circle and \(\large A\hat{O}B=\theta\) radians. The tangents to the circle A and B intersect at C.
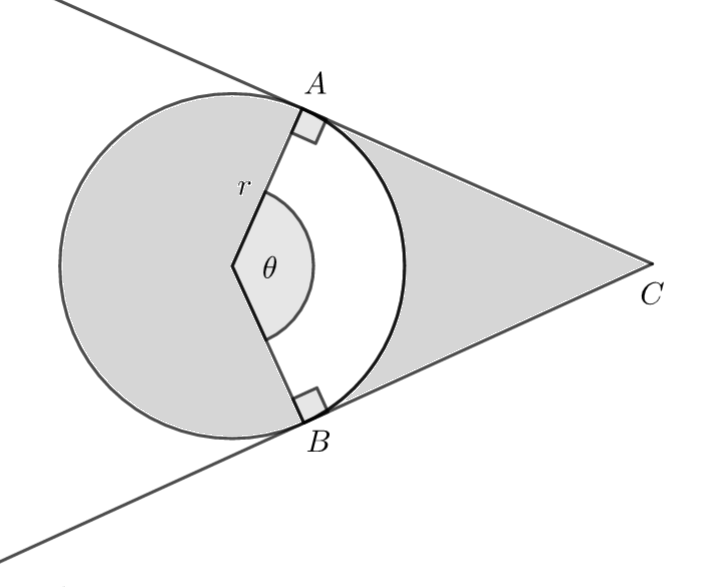
a) Show that \(\large AC=r\tan (\frac{\theta}{2})\)
b) Hence, find the value of \(\large \theta\) when the two shaded regions have an equal area.
Hint
b) Area of red region = Area of kite OACB - Area of sector OAB
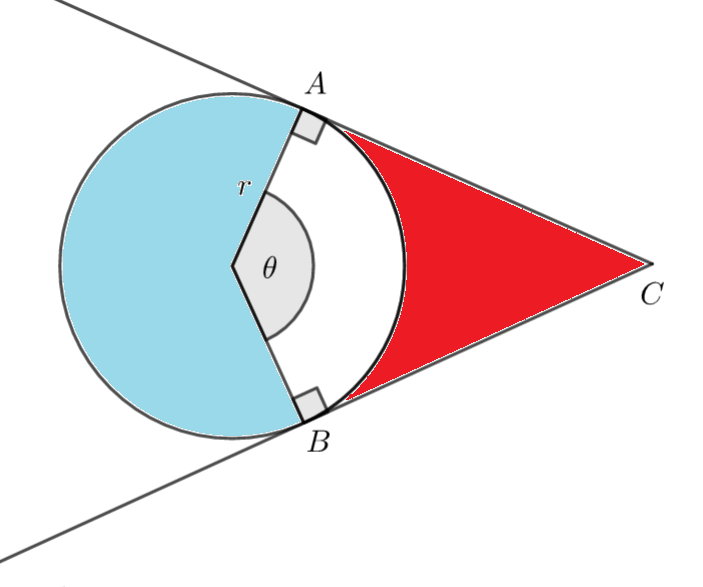
Full Solution
a) Triangle OAC is a right angled triangle, so we can use right-angled triangle trigonometry to work out the length AC (opposite to the angle \(\large\frac{\theta}{2}\)
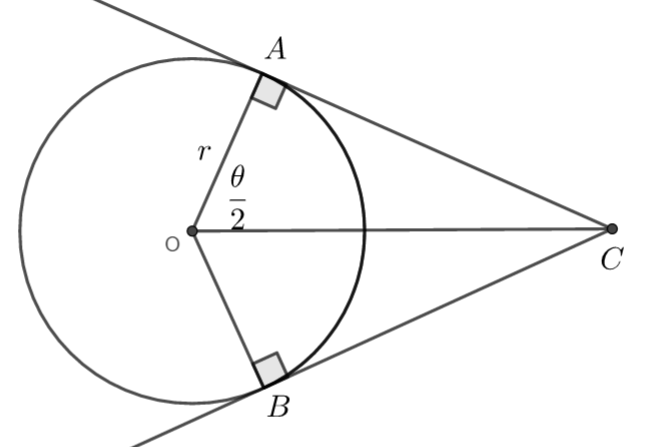
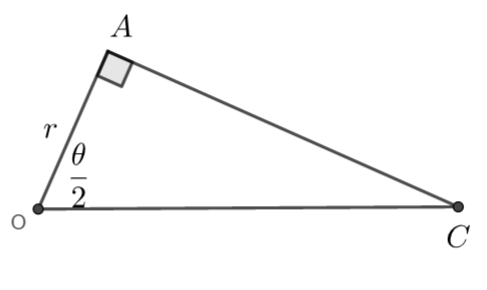
\(\large \tan\frac{\theta}{2}=\frac{AC}{r}\)
\(\large AC=r\tan\frac{\theta}{2}\)
b)
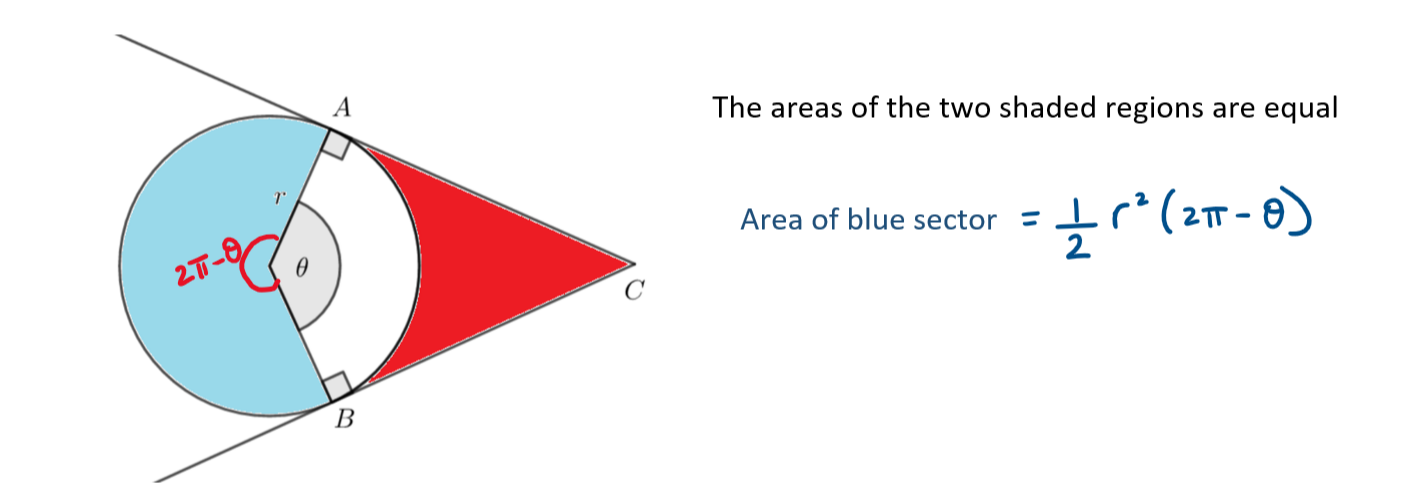
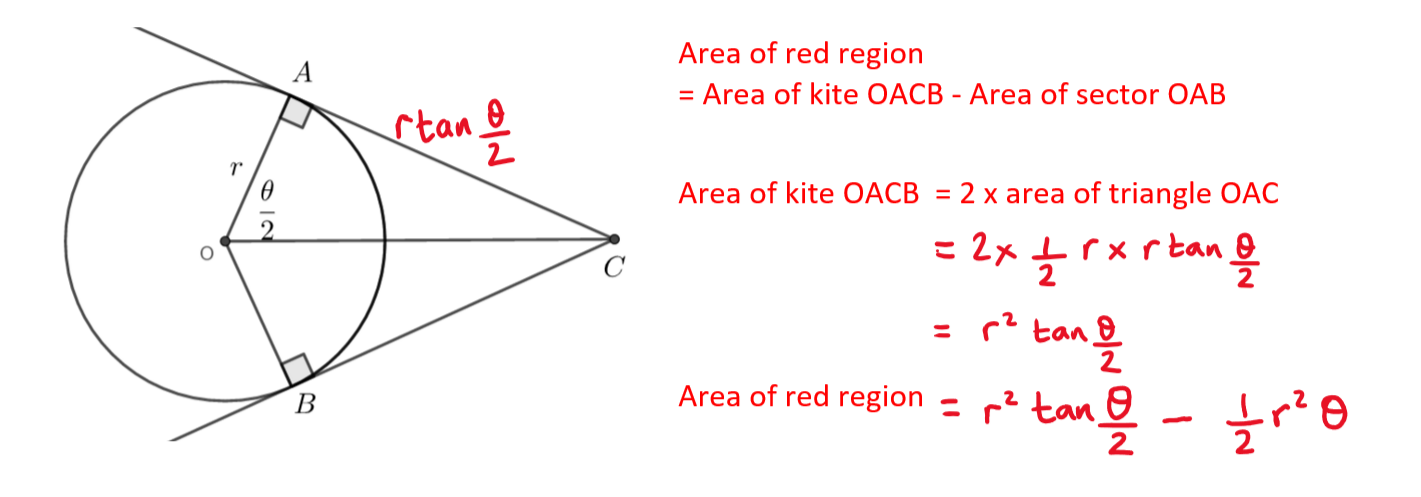



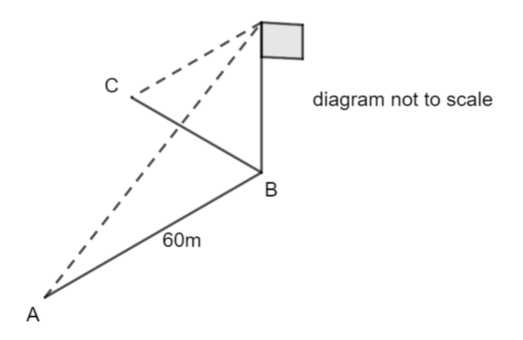
A, B and C are points on horizontal ground.
C is due West of B. A is due South of B. AB = 60m
A flagpole stands vertically at B.
From A, the angle of elevation of the top of the flagpole is 11°.
From B, the angle of elevation of the top of the flagpole is 15°.
Calculate the distance AC giving your answer to 3 significant figures.
Hint
Full Solution
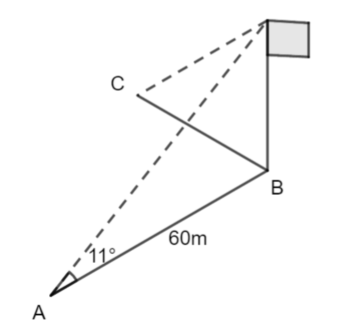 We can find the height of the flagpole
We can find the height of the flagpole
\(\large\tan11°=\frac{h}{60}\\ \large h=60\times\tan11°\\ \large h\approx 11.7\)
use calculator memory to store the exact value of this length
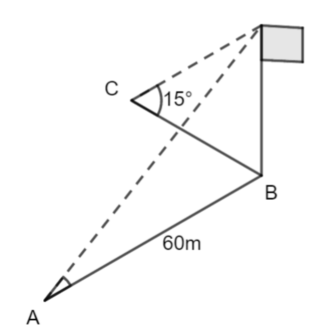 Now we can find the length BC
Now we can find the length BC
\(\large\tan15°=\frac{h}{BC}\\ \large BC=\frac{h}{tan15°}\\ \large BC=\frac{60\times \tan11°}{tan15°}\\\\ \large BC\approx 43.5\)use the exact value of h
use calculator memory to store the exact value of this length
ABC is a right-angled triangle
\(\large AC^2=AB^2+BC^2\\ \large AC^2=60^2+(43.5...)^2\\ \large AC\approx74.1m\)



The diagram shows a cuboid ABCDEFGH. AB = 8 cm, AE = 6 cm and BC = 15 cm.
a) Find the length of AC.
Give your answer correct to 3 significant figures
b) Find the size of the angle between the line EC and the plane ABCD.
Give your answer correct to 1 decimal place.
Hint
b) Here is the right-angled triangled you should consider for this question

Full Solution
a)
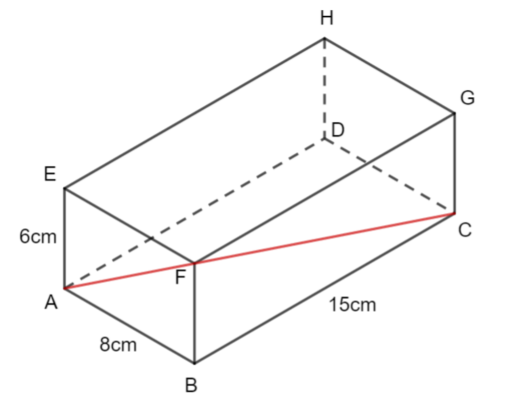
ABC is a right-angled triangle.
Use Pythagoras' Theorem to work out AC.
AC² = 8² + 15²
AC² = 289
AC = 17cm
b)
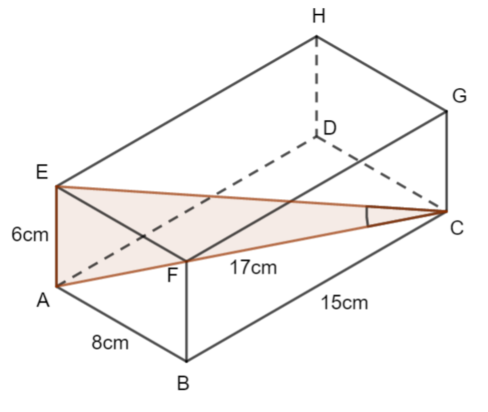 The angle between the line EC and the plane ABCD can be visualised in the diagram.
The angle between the line EC and the plane ABCD can be visualised in the diagram.
EAC is a right-anghled triangle.
We can use trigonometry to work out the angle
\(\large \tan\theta=\frac{6}{17}\\ \large\theta=\tan^{-1}(\frac{6}{17})\\ \large\theta\approx19.4°\)


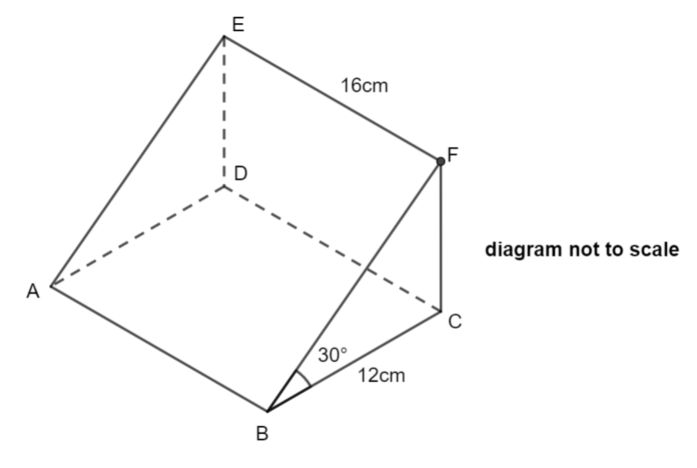
ABCDEF is a prism in which the triangle BCF is the cross section.
BC = 12cm, EF = 16cm, angle CBF = 30° and angle FCB = 90°
The angle AF makes with the plane ABCD is \(\large \theta\).
Show that \(\large \tan\theta=\frac{\sqrt{3}}{5}\)
Hint
The following triangle helps you to see the angle that is required.

Full Solution
We can work out the lengths CF and CA
Use the triangle CBF to work out CF
\(\large \tan30°=\frac{CF}{12} \qquad \qquad \tan30°=\frac{\sqrt{3}}{3}\\ \large \frac{\sqrt{3}}{3}=\frac{CF}{12}\\ \large \frac{12\sqrt{3}}{3}=CF\\ \large CF=4\sqrt{3}\)
Use Pythagoras' Theorem to work out CA
CA² = 12² + 16²
CA² = 400
CA = 20
Now put this information in the diagram
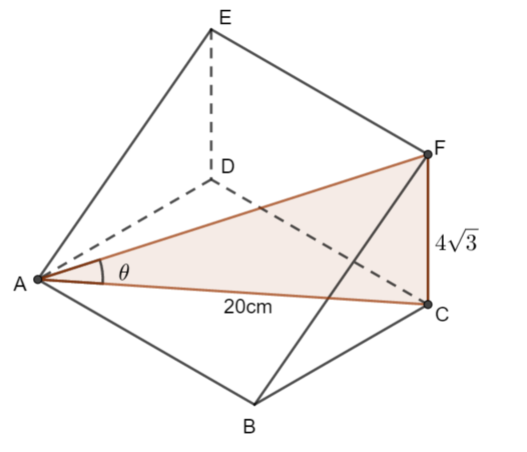
\(\large \tan\theta=\frac{4\sqrt{3}}{20}\\ \large \tan\theta=\frac{\sqrt{3}}{5}\)


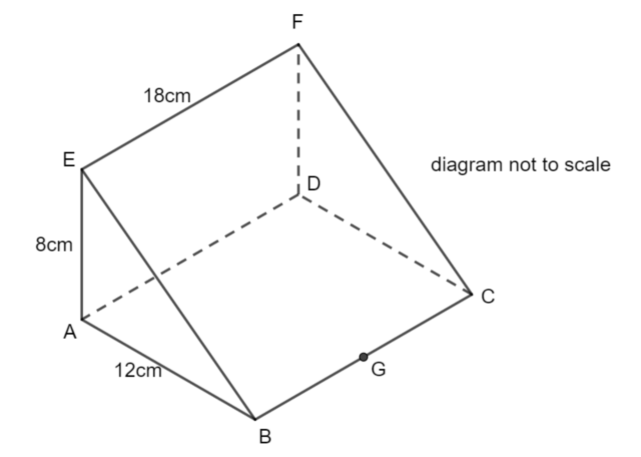
ABCDEFG is a triangular prism.
AB = 12cm, AE = 8cm, EF = 18cm.
Angle BAE = 90°
G is the midpoint of BC.
Calculate the angle between EG and the plane ABCD.
Give your answer correct to 1 decimal place.
Hint
Here is the triangle that contains the angle required
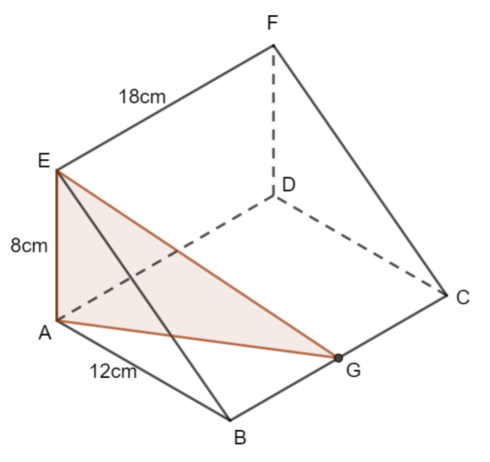
Full Solution
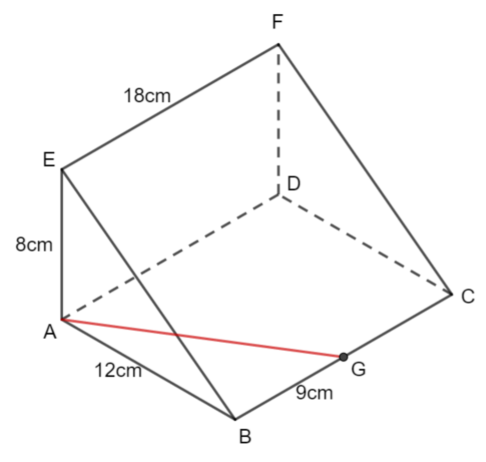 We shoud calculate the length AG using Pythagoras' Theorem.
We shoud calculate the length AG using Pythagoras' Theorem.
G is the midpoint of BC. Therefore BG is 9cm.
AG² = 9² + 12²
AG² = 225
AG = 15
 Here is the angle that we are required to find.
Here is the angle that we are required to find.
It is a right-angled triangle.
\(\large \tan\theta =\frac{8}{15}\\ \large\theta=\tan^{-1}(\frac{8}{15})\\ \large\theta\approx24.0°\)



ABCDEF is a prism.
AB = AE = BE = 6cm. BC = 10cm
Calculate
a) the length EC
b) the angle AEC
c) the angle between EC and the plane ABCD
Give lengths to 3 significant figures and angles to 1 decimal place.
Hint
For parts b) and c), the challenge is to visualise the triangles required to find the angles. The question aims to show you that the angles in part b) and c) are not the same!
b)
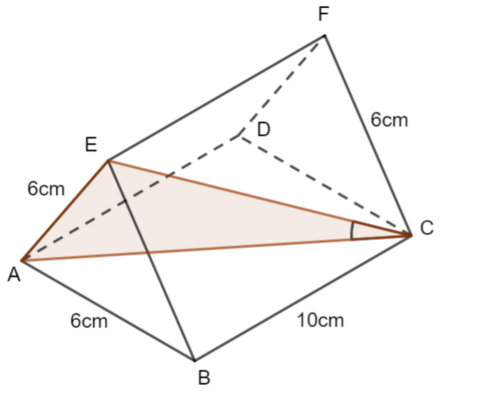
c)
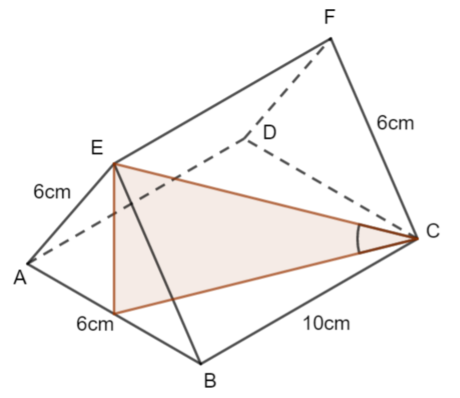
Full Solution
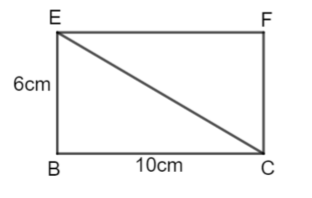 a) WE can find the length EC using Pythagoras' Theorem
a) WE can find the length EC using Pythagoras' Theorem
EC² = 10² + 6²
EC² = 136
\(\large EC = \sqrt{136}\)
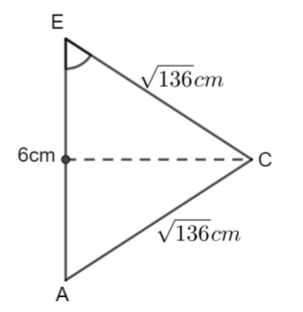 b) The length AC is the same as EC
b) The length AC is the same as EC
We have an isoceles triangle. Use just the top half of the triangle and right-angled trigonometry to find the angle ACE (or you can use the whole triangle and the cosine rule).
\(\large \cos\theta=\frac{3}{\sqrt{136}}\\ \large \theta=\cos^{-1}(\frac{3}{\sqrt{136}})\\ \large \theta\approx75.1°\)
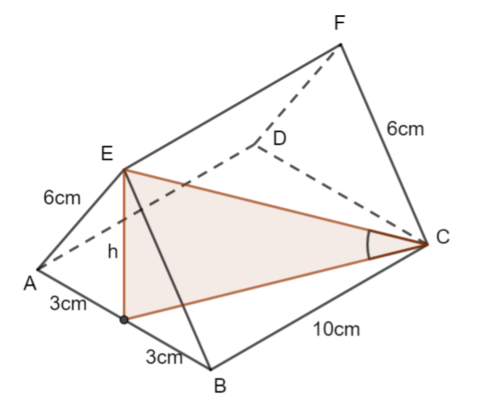 c) The angle between EC and the plane ABCD can be visualised in this diagram.
c) The angle between EC and the plane ABCD can be visualised in this diagram.
We know the length EC =\(\large \sqrt{136}\)
We need to find another legnth in this right-anghled triangle. Find the height of the triangle:
\(\large h^2=6^2-3^2\\ h=\sqrt{27}\)
Let \(\large \alpha\) be the angle between EC and the plane ABCD
\(\large\sin\alpha=\frac{\sqrt{27}}{\sqrt{136}}\\ \large\alpha\approx26.5°\)


The following diagram shows a quadrilateral ABCD.
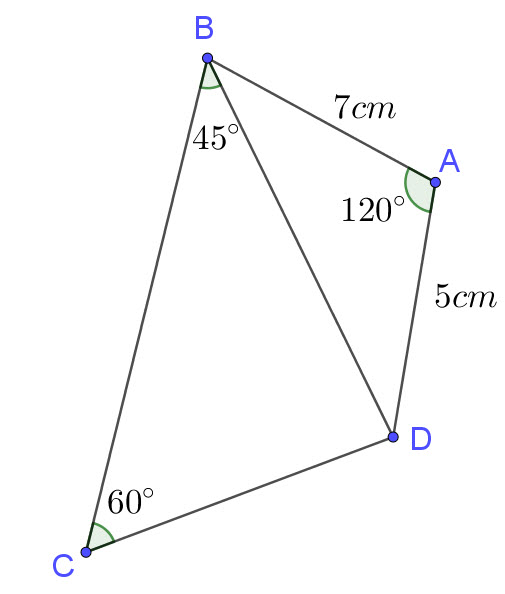
AB = 7cm , AD = 5cm ∠DAB=120° , ∠DBC=45° , ∠BCD=60°
BD = \(\sqrt{a}\)
CD = \(\sqrt{b}\)
\(a,b \in \mathbb{Z}\)
Find a and b
Hint
Full Solution
Print from here


The following diagram shows a quadrilateral ABCD.

AD = x – 1 , BD = x + 1 , DC = 2x and \(\angle CDA\) = 120°
The sum of the area of triangle ADC and triangle BDC is \(4 \sqrt{3}\)
Find x
Hint
Full Solution
Print from here


In a triangle ABC, AB = 8cm, BC = a, AC = b and \(\angle BAC\) = 30°
a) Show that \(b^2-8\sqrt{3}b+64-a^2=0\)
b) Hence find the possible values of a (in cm) for which the triangle has two possible solutions.
Hint
a) Use the cosine rule.
The following applet might help you visualise the triangle. Drag the slider to see the different possible values that a can take
Full Solution
Print from here

Given that \(cosx=-\frac{\sqrt{7}}{3}\) and \(\frac{\pi}{2}\le x\le \pi\) , find the possible values of sinx and cotx
Hint
Draw a circle.
Full Solution
Print from here

If \(tanx=\frac{12}{5}\) and \(\pi\le x\le \frac{3\pi}{2}\) , find the value of cosecx
Hint
Draw a circle.
Full Solution
Print from here


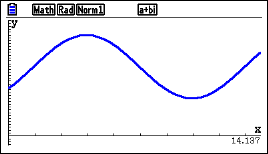
The height h of water, in metres, in a habour is modelled by the function \(\large h(t)=5.5\sin(0.5(t-1.5))+12\) where t is time after midday in hours.
a) Find the initial height of the water.
b) At what time is it when the water reaches this height again?
c) Find the maximum height of the water.
d) How much time is there in between the first and second time that the water at 16 metres?
Give heights to 3 significant figures and times to the nearest minute
Hint
This question gets you to use your calculator to solve problems.
Ensure that your calculator is in radian mode.
Adjust the view window so that you get a good view of the graph
Full Solution
a) 8.25 metres

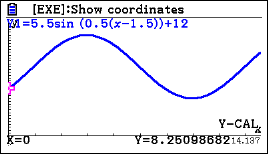
b) 21:17 or 9.17 pm
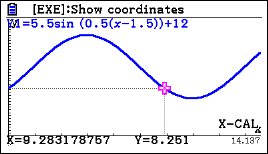 0.283x60 = 17 minutes
0.283x60 = 17 minutes
c) 4.64 meters
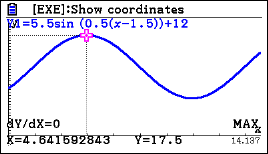
d) 3 hours 2 mins


6.155 - 3.129 = 3.026
0.026 x 60 = 2 mins


The following diagram shows a Ferris wheel.

The height, h metres of a seat above ground after t minutes is given by \(\large h(t)=a\ \cos(bt)+c\) , where a, b and c \(\large \in \mathbf{R}\)
The following graph shows the height of the seat.
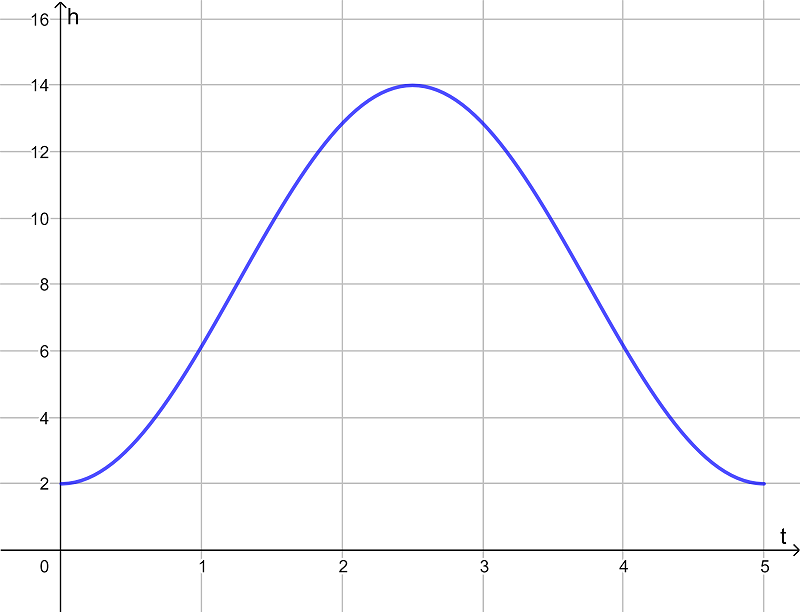
Find the values of a, b and c
Hint
amplitude is |a|
vertical shift = c
Full Solution
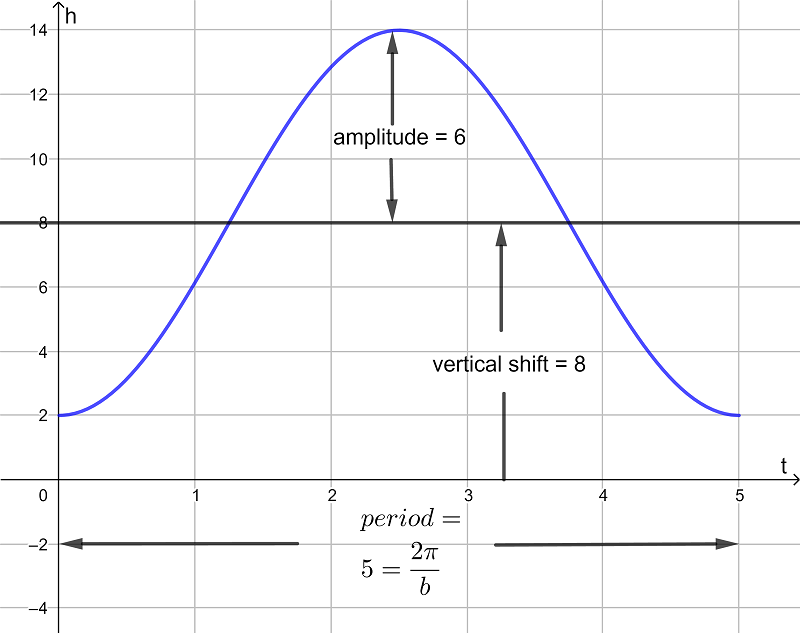
amplitude is |a| = 6 , a = -6
vertical shift = c = 8


Consider a function f, such that \(\large f(x) = 5.6\cos(\frac{\pi}{a}(x-1))+b\) , \(\large 0\le x\le 15\), \(\large a,b\in \mathbf{R}\)
The function f has a local maximum at the point (1 , 8.8) and a local minimum at the point (10 , -2.4)
a) Find the period of the function
b) Hence, find the value of a.
c) Find the value of b.
Hint
For \(\large f(x)=a \cos(b(x+c))+d\)
period = \(\large \frac{2\pi}{b}\)
Full Solution
a) period = 18

b) period = \(\large \frac{2\pi}{\frac{\pi}{a}}\)
\(\large \frac{2\pi}{\frac{\pi}{a}}=18\\ \large 2a = 18\\ \large a = 9\)
c) Use (1 , 8.8)
\(\large5.6\cos(\frac{\pi}{9}(1-1))+b=8.8\\ \large5.6\cos(0)+b=8.8\\ \large5.6+b=8.8\\ \large b=3.2\)


Consider a function f, such that \(\large f(x) = a\sin(\frac{\pi}{15}(x+2))+b\) , \(\large a,b\in \mathbf{R}\)
The function f has passes through the points (10.5 , 5.5) and (15.5 , 2.5)
Find the value of a and the value of b
Hint
This will give you two equations for the unknowns a and b.
Solve these equations
Full Solution
\(\large f(x) = a\cos(\frac{\pi}{15}(x+2))+b\)
(10.5 , 5.5)
\(\large a\sin(\frac{\pi}{15}(10.5+2))+b=5.5\\ \large a\sin(\frac{12.5}{15}\pi)+b=5.5\\ \large a\sin(\frac{25}{30}\pi)+b=5.5\\ \large a\sin(\frac{5}{6}\pi)+b=5.5\\ \large a(0.5)+b=5.5\\ \large a+2b=11\)
(15.5 , 2.5)
\(\large a\sin(\frac{\pi}{15}(15.5+2))+b=2.5\\ \large a\sin(\frac{17.5}{15}\pi)+b=2.5\\ \large a\sin(\frac{35}{30}\pi)+b=2.5\\ \large a\sin(\frac{7}{6}\pi)+b=2.5\\ \large a(-0.5)+b=2.5\\ \large -a+2b=5\)
a + 2b = 11
-a + 2b = 5
4b = 16
b = 4
a= 3


The following diagram shows a ball attached to the end of a spring.
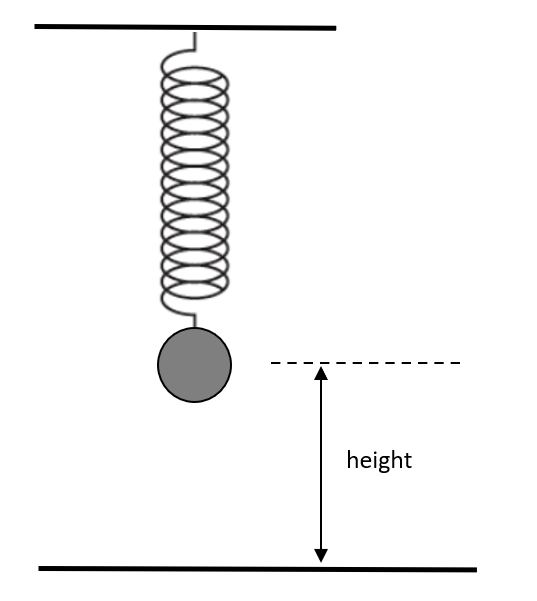
The height, h, in mtres of the ball above the ground t seconds after being released can be modelled by the function
\(\large h(t)=a\cos(\frac{\pi}{b}t)+c\) , \(\large a,b, c\in \mathbf{R}\)
The ball is release from an initial height of 4 metres.
After \(\large \frac{4}{3}\) seconds, the ball is at a height of 1.6 metres.
It takes the ball 4 seconds to return to its initial height.
Find the values of a, b and c.
Hint
If it takes 4 seconds to return to the initial height, then the period = 4
Full Solution
If it takes 4 seconds to return to the initial height, then the period = 4
\(\large \frac{2\pi}{\frac{\pi}{b}}=4\\ \large b=2\)
\(\large h(t)=a\cos(\frac{\pi}{2}t)+c\)
When t = 0, h = 4
\(\large a\cos(0)+c=4\\ \large a+c=4\)
When t = \(\large \frac{4}{3}\), h = 1.6
\(\large a\cos(\frac{\pi}{2}(\frac{4}{3}))+c=1.6\\ \large a\cos(\frac{2\pi}{3})+c=1.6\\ \large a(-0.5)+c=1.6\\ \large -a+2c=3.2\)
a + c = 4
-a + 2c = 3.2
3c = 7.2
c = 2.4
a = 1.6


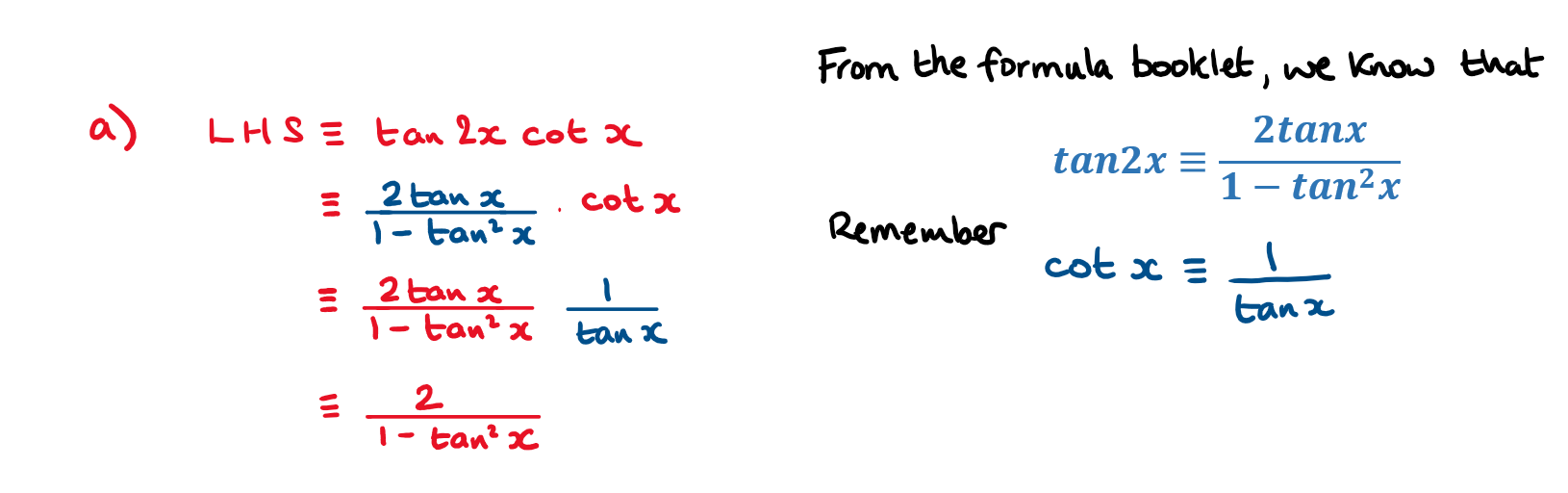
a) Show that the equation \(\large 2 \sin^2x=3 \cos x\) may be written in the form
\(\large 2 \cos^2x+3 \cos x-2=0\)
b) Hence, solve \(\large 2 \sin^2x=3 \cos x\) , for \(\large 0\le x\le2\pi\)
Hint
a) Use \(\large \cos^2 x+\sin ^2x\equiv1\)
b) Use the answer from part a). This is a quadratic equation.
Don't forget that \(\large \cos^2 x\) means \(\large (\cos x)^2\)
Full Solution
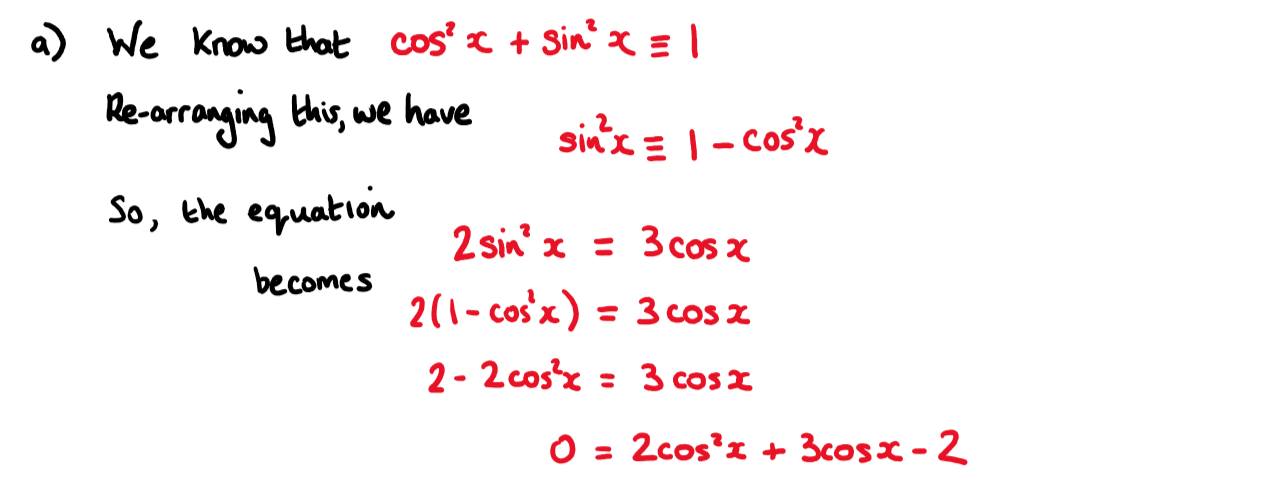

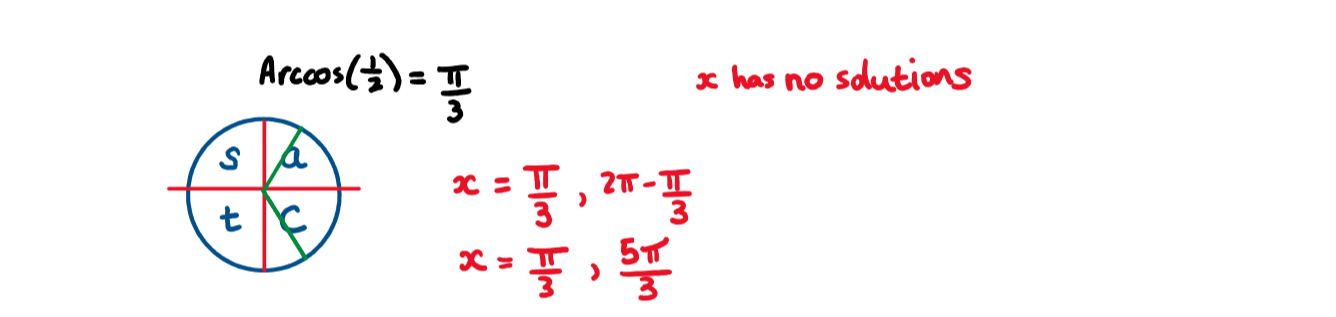



Given that \(x=\frac{2}{cos\theta}\) and \(y=3tan\theta\)
show that \(\frac{x^2}{4}-\frac{y^2}{9}=1\)
Hint
Full Solution
Print from here


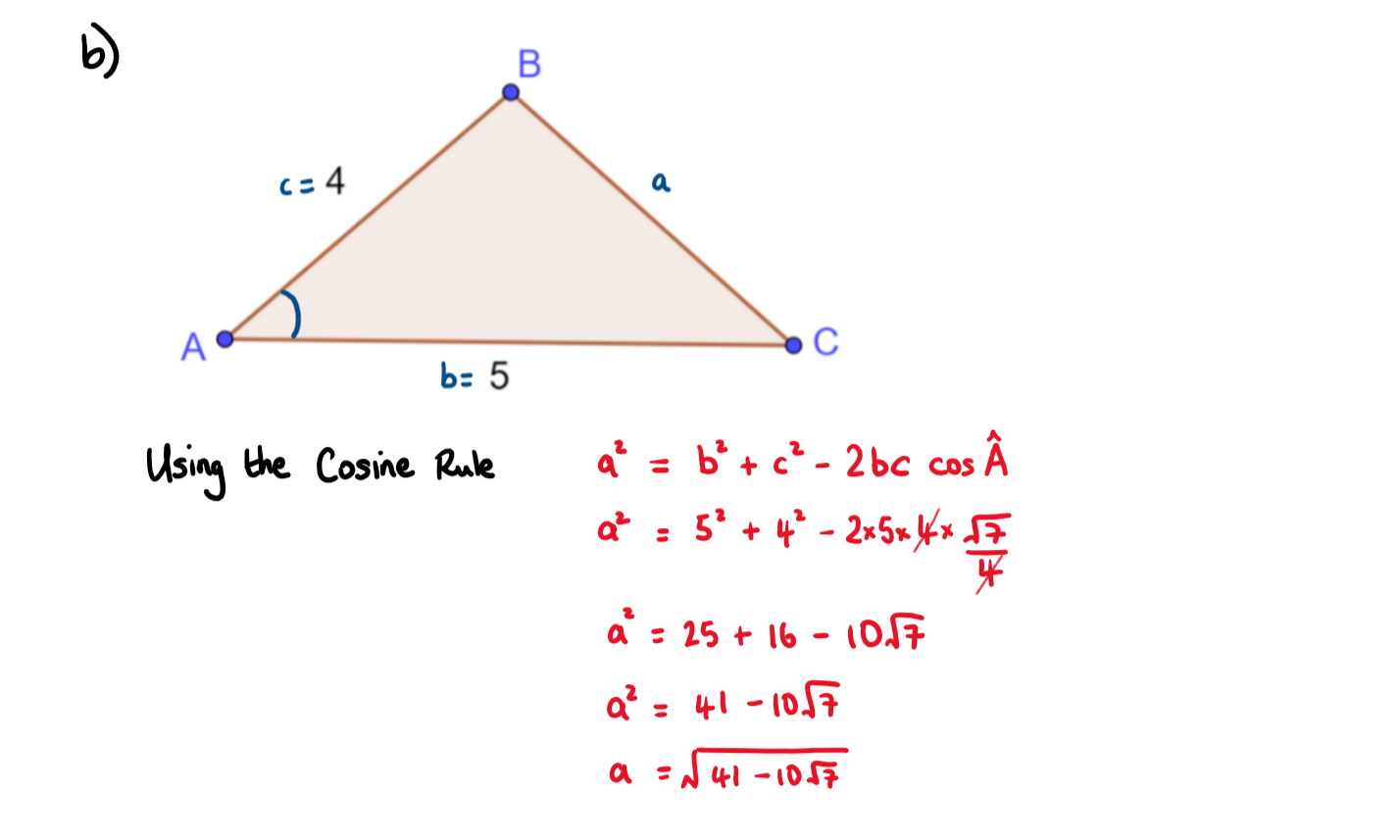
The following diagram shows triangle ABC with AB = 4 and AC = 5
 DIAGRAM NOT TO SCALE
DIAGRAM NOT TO SCALE
a) Given that \(\large \sin \hat A=\frac{3}{4}\), find the value of \(\large \cos \hat A\)
b) Hence, show that the length of \(\large BC=\sqrt{41-10\sqrt{7}}\)
Hint
a) You can use the Pythagorean identity \(\large \cos^2\theta+\sin^2\theta\equiv1\)
b) Use the Cosine Rule, c² = a² + b² - 2ab cosC
Full Solution



Prove that \(\large \frac{\sin ^3\theta}{\tan \theta}+\cos^3\theta\equiv\cos\theta\)
Hint
This is a very difficult proof for SL students!
There is always more than one way to carry out this proof.
Full Solution

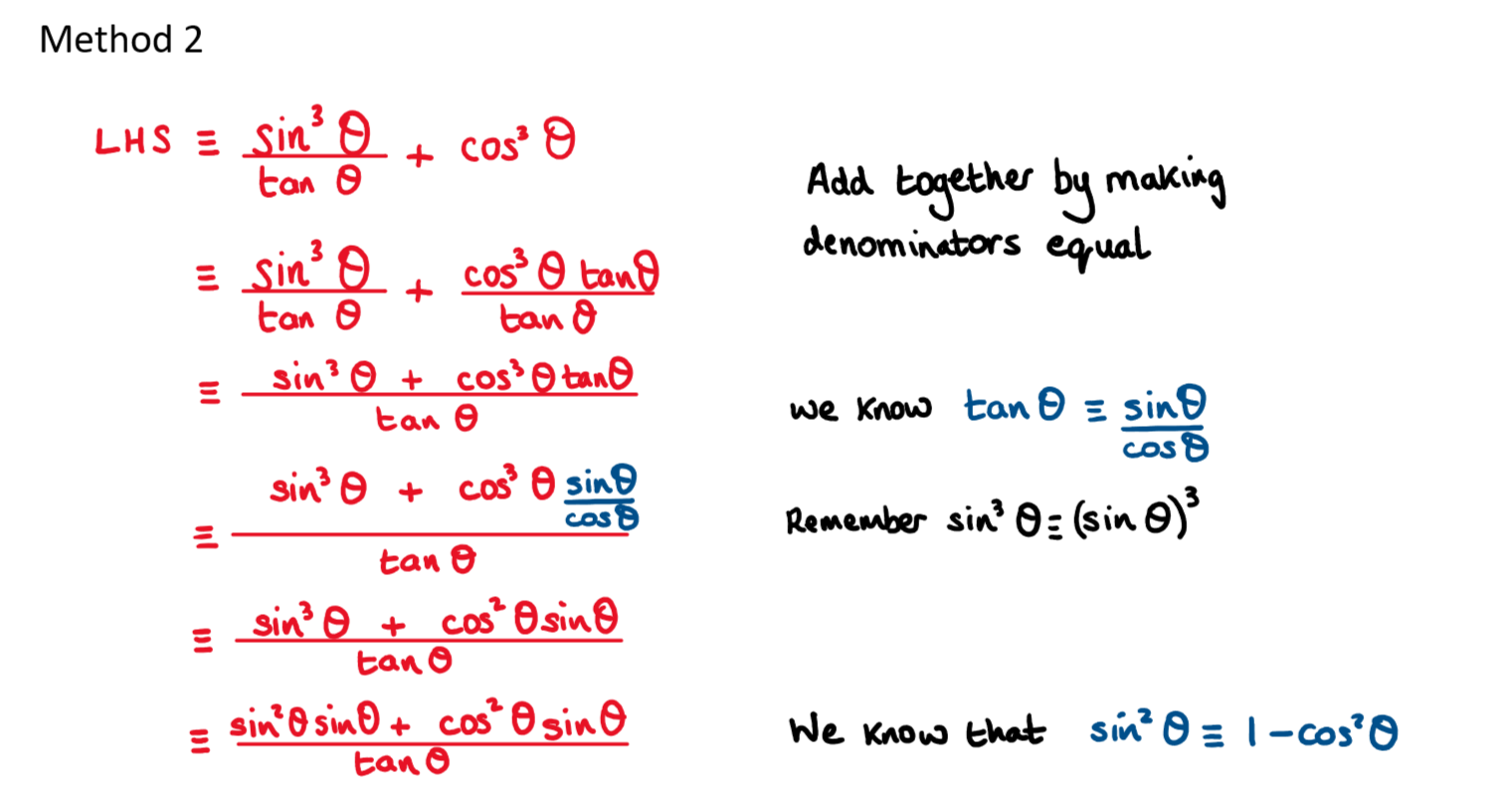


a) Show that \(\large \text{cosec}^2x-\cot ^2x\equiv1\)
b) Hence, prove that \(\large \text{cosec}^4x-\cot^4x\equiv \text{cosec}^2x+\cot ^2x\)
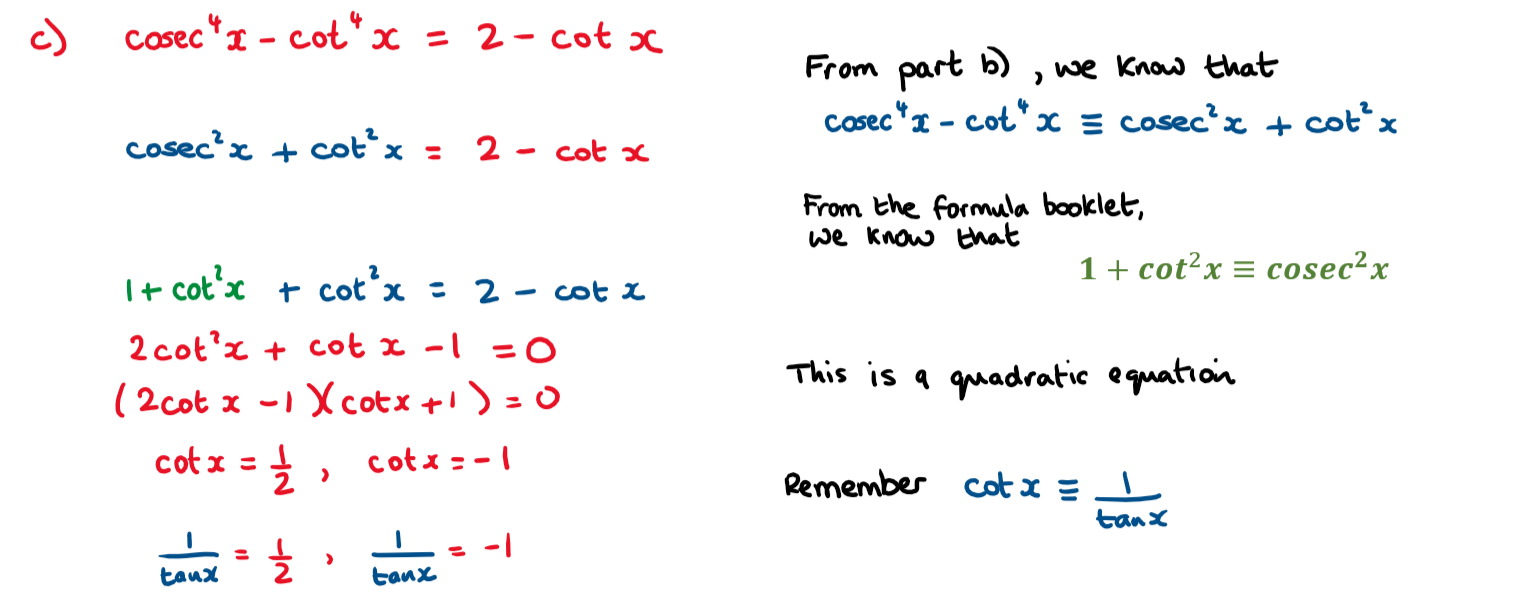
c) Given that \(\large \text{arctan}(2)\approx63.4°\), solve
\(\large \text{cosec}^4x-\cot^4x=2-\cot x\) , for \(\large 0 \le x \le360°\)
Hint
a) Use the identity \(\large 1+\cot^{ 2 }\theta \equiv \text{cosec}^{2}\theta\)
\(\large a^2-b^2\equiv (a-b)(a+b)\\ \)
\(\large a^4-b^4\equiv (a²-b²)(a²+b²)\\ \)
c) Use your answer in part b) to write a quadratic equation for cot x. Again, you will need the identity \(\large 1+\cot^{ 2 }\theta \equiv \text{cosec}^{2}\theta\)
Full Solution
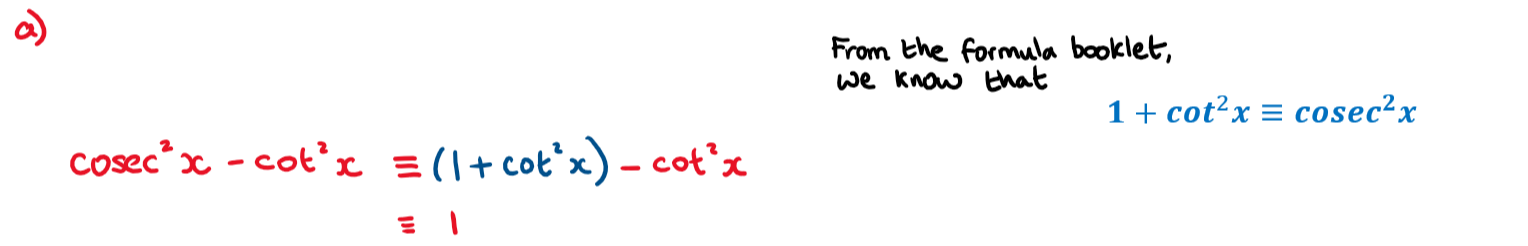
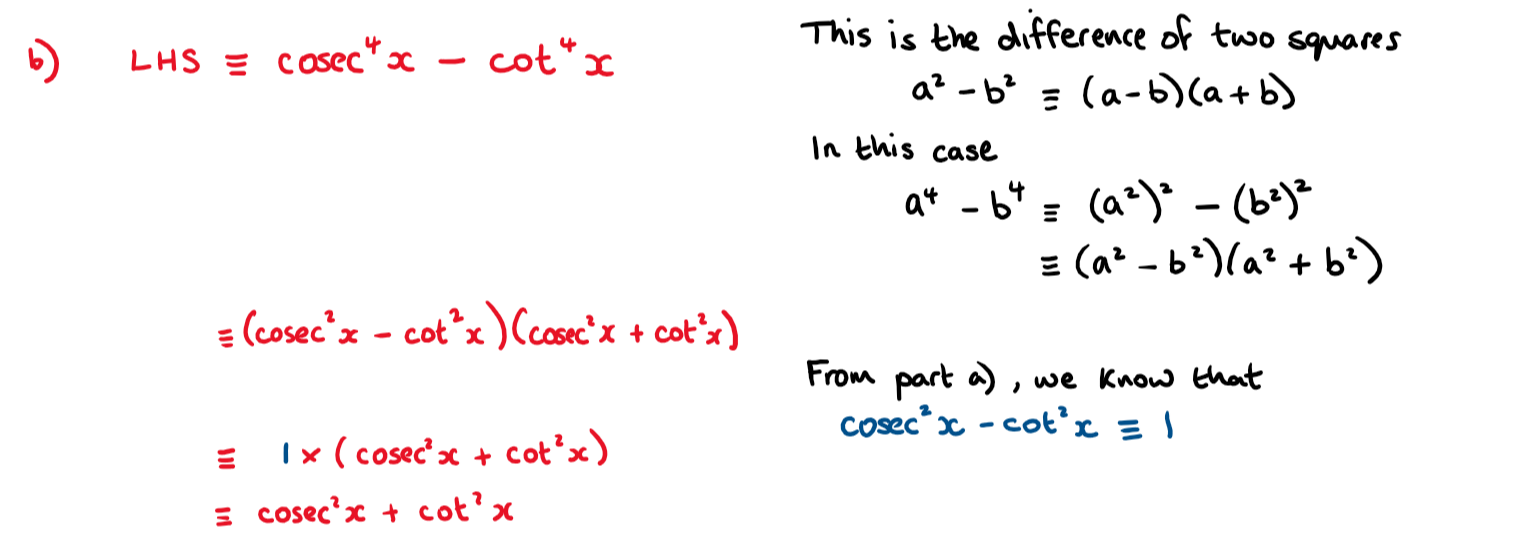
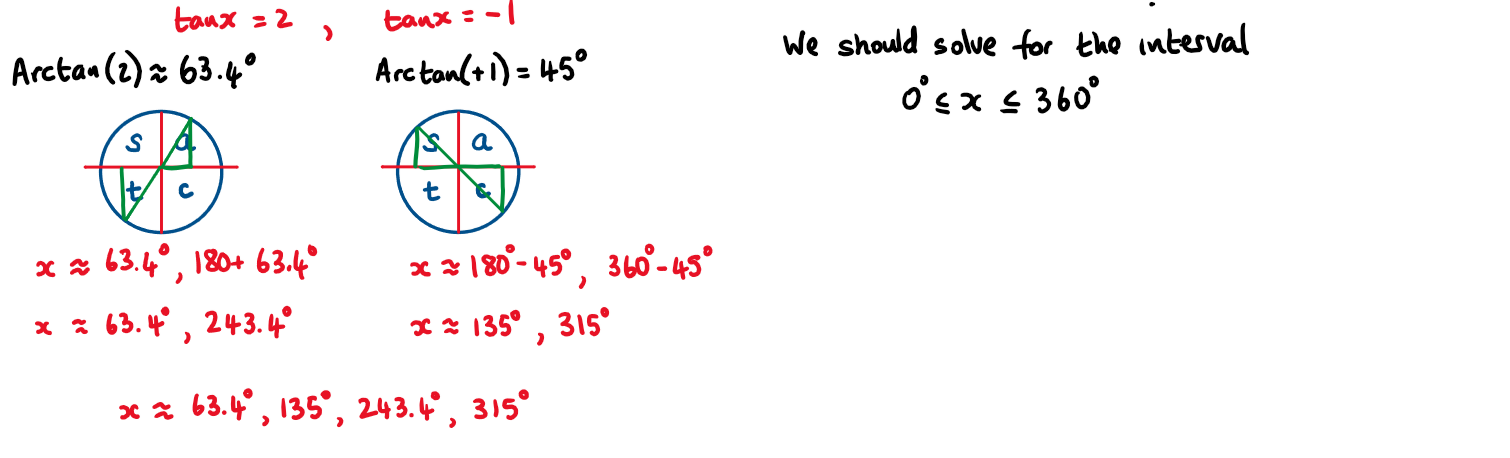

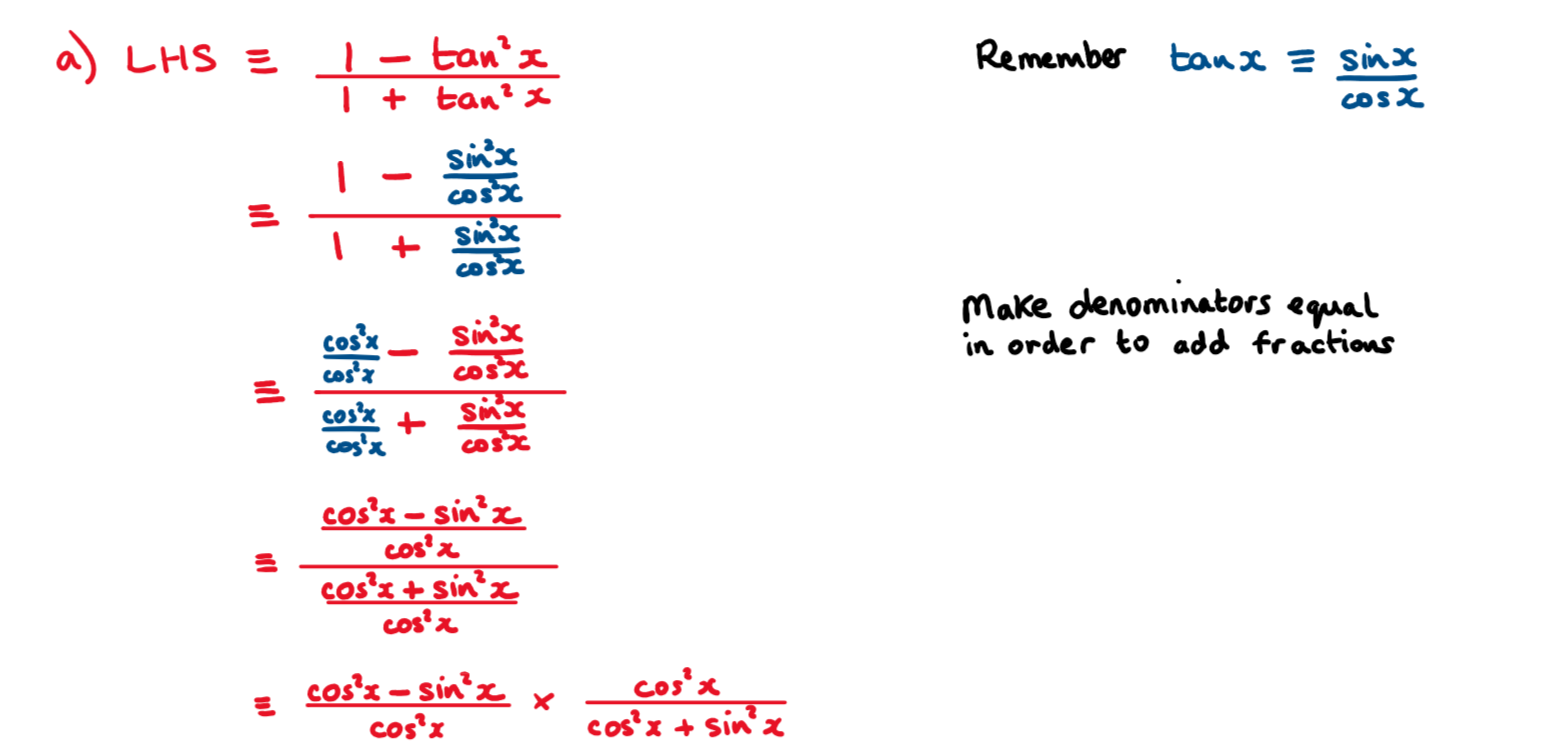
a) Prove that \(\large \frac{1-\tan^2x}{1+\tan^2x}\equiv\cos2x\)
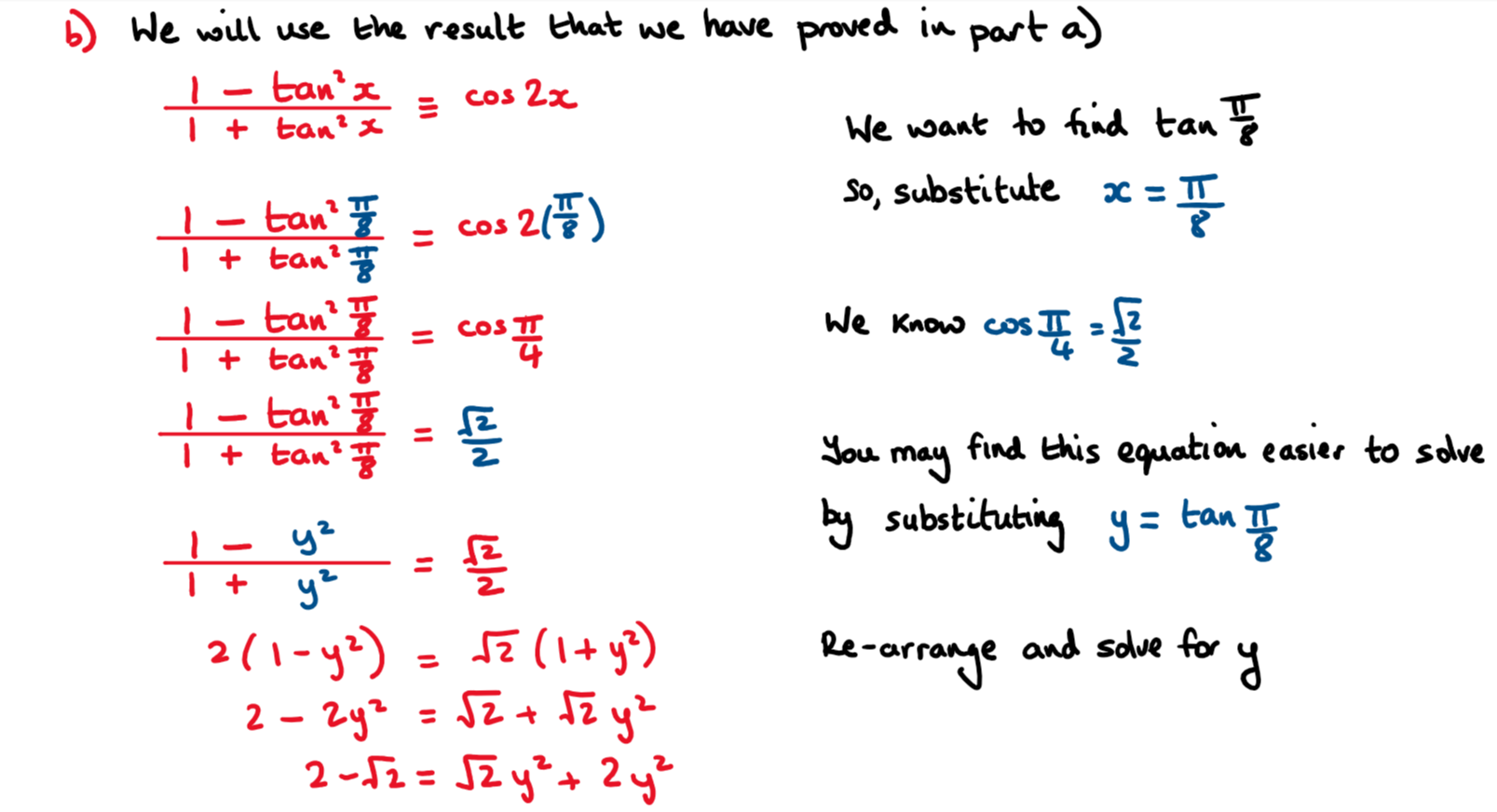
b) Hence, show that
\(\large \tan\frac{\pi}{8}=\sqrt{3-2\sqrt{2}}\)
Hint
a) Start with the left-hand side of the identity.
Use \(\tan x\equiv\frac{\sin x}{\cos x}\)
b) The term hence is important here. You need to use the result proved in part a)
Try substituting \(\large x=\frac{\pi}{8}\)
Full Solution



If \(\large \sin A=\frac{4}{5}\) , where \(\large 0\le A\le\frac{\pi}{2}\)
and \(\large \cos B=-\frac{12}{13}\) , where \(\large \pi \le B\le\frac{3\pi}{2}\)
work out \(\large \cos(B-A)\)
Hint
You will need to work out cosA and sinB
Full Solution

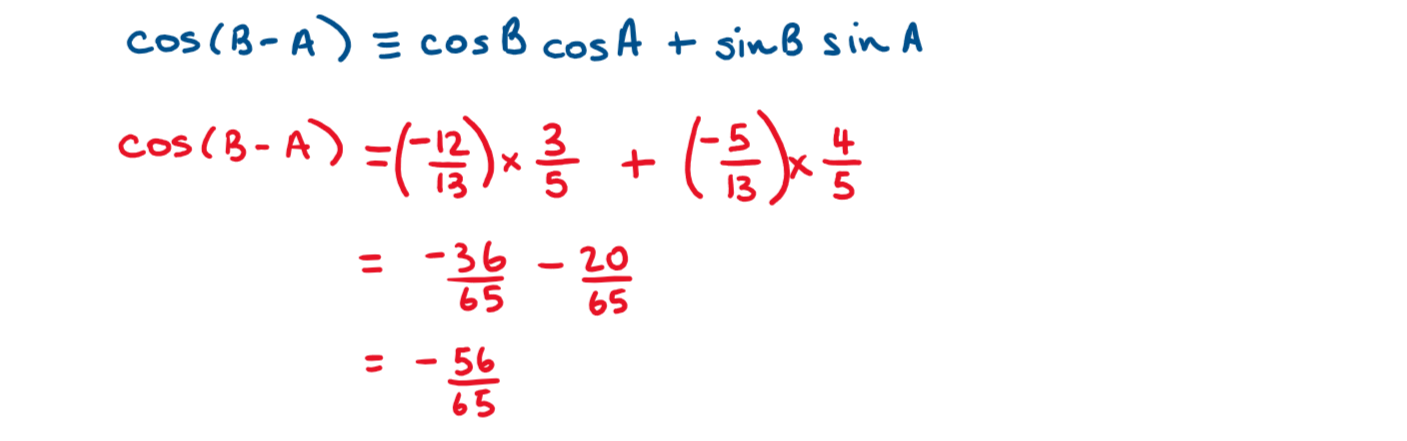

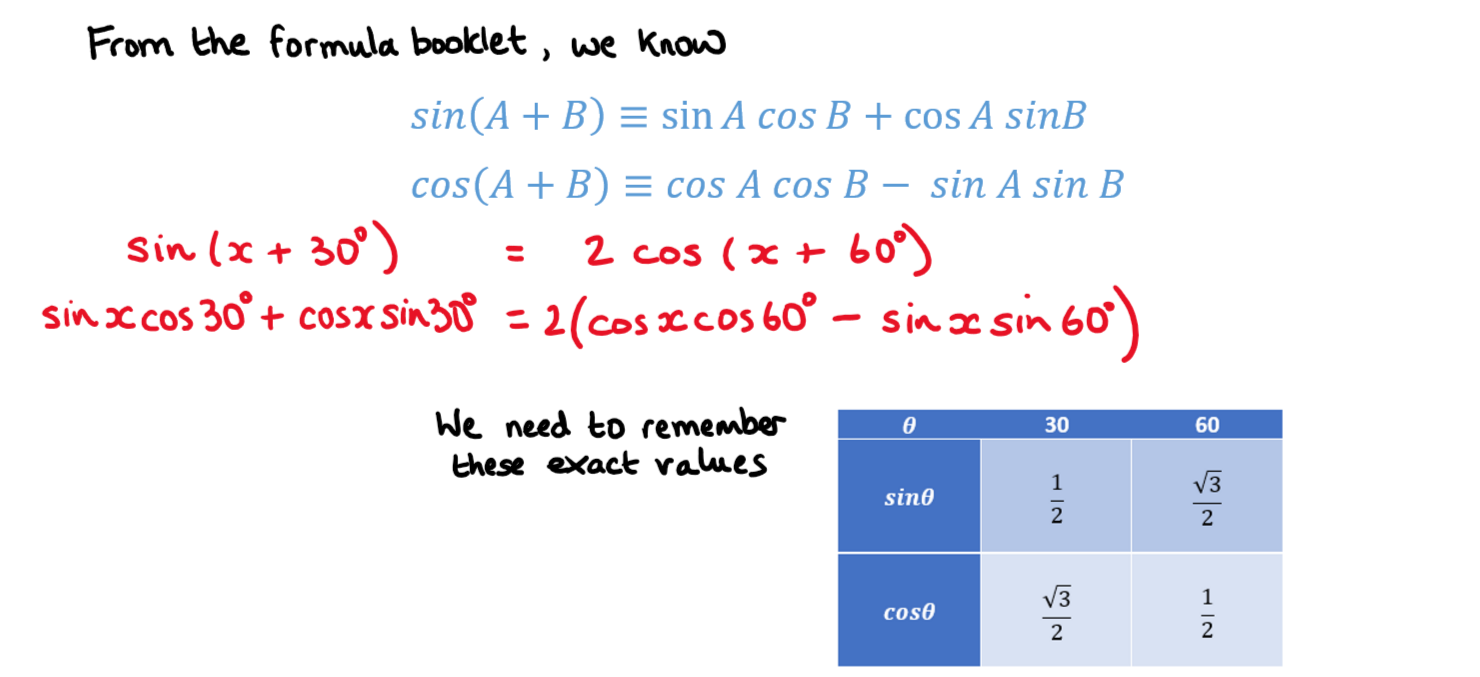
If \(\large \sin(x+30°)=2\cos(x+60°)\), then show that \(\large \tan x=\frac{\sqrt{3}}{9}\)
Hint
Full Solution


a) By writing 15° as 45° - 30° , find the value of sin15°
b) Hence, show that the area of this triangle \(\large =4(\sqrt{3}-1)\)
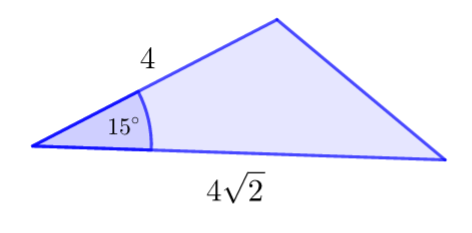
Hint
a) We need to use the compound angle formula to evaluate sin(45°-30°)
Full Solution

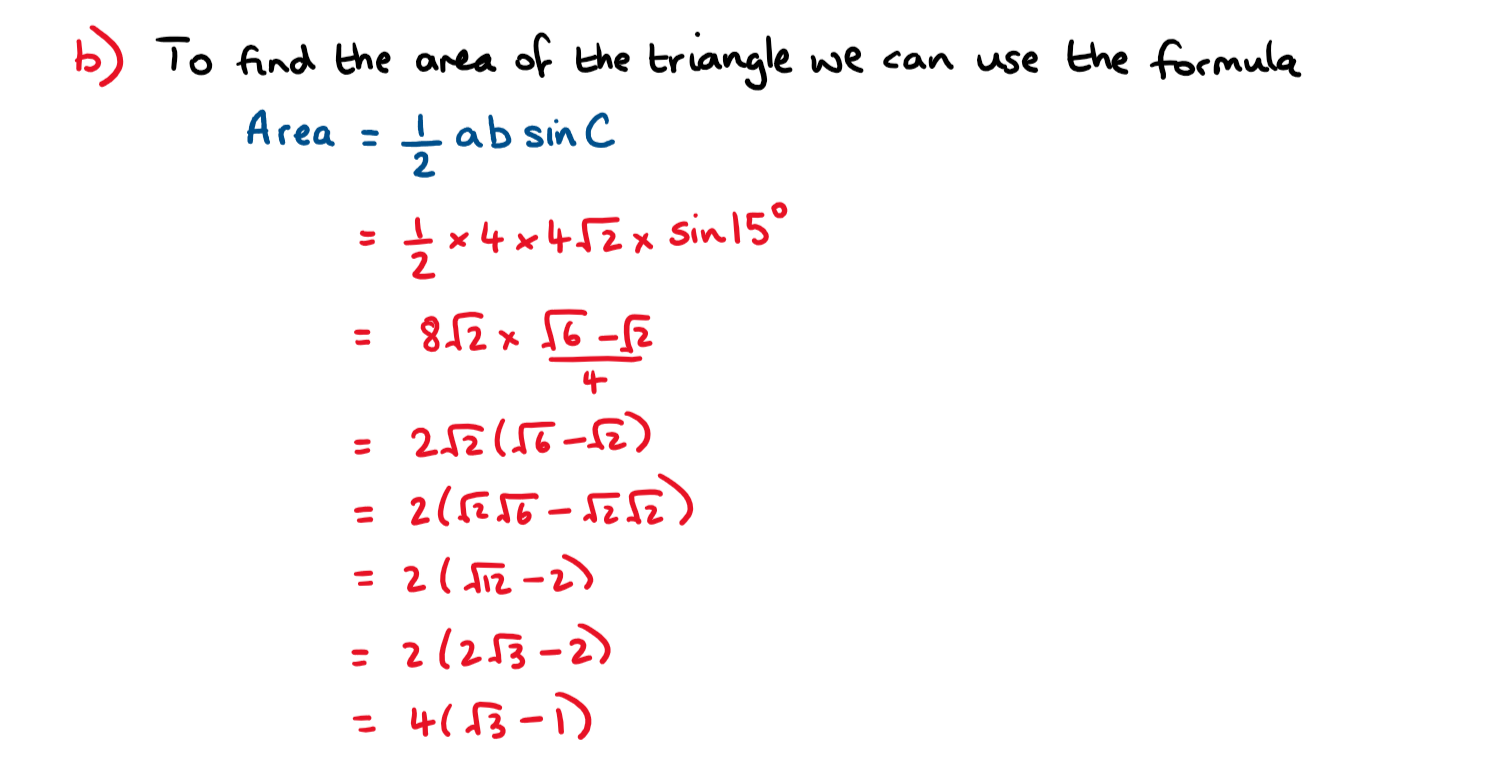

Prove that
\(\large \frac{\sin(A+B)+\sin(A-B)}{\cos(A+B)+\cos(A-B)}=\tan A\)
Hint

Full Solution



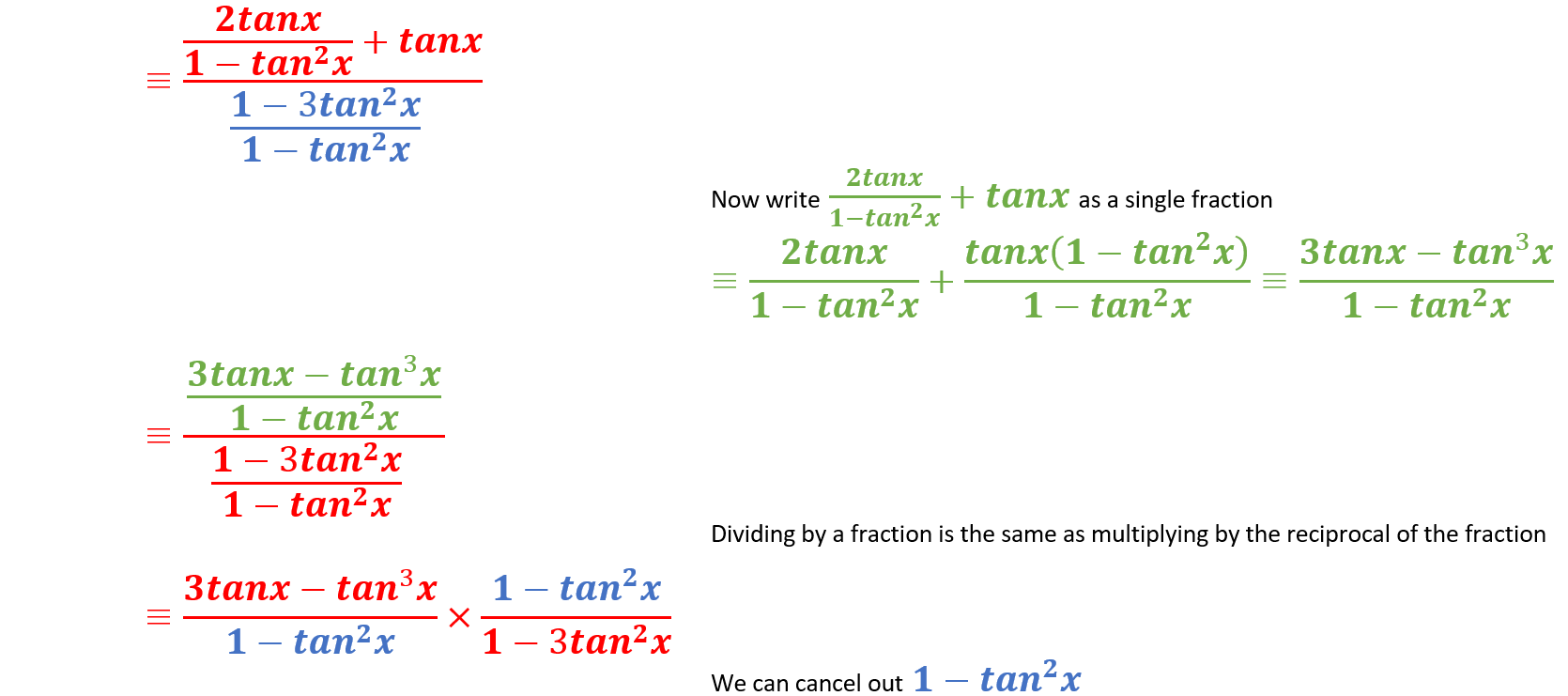
Prove that \(\large \tan 3x\equiv \frac{3\tan x-\tan^3x}{1-3\tan^2x}\)
Hint
Use the compound angle formula to work out \(\large \tan(2x + x)\)
Very careful manipulation is required in this question. Take your time to make sure that you do not make a mistake.
Full Solution




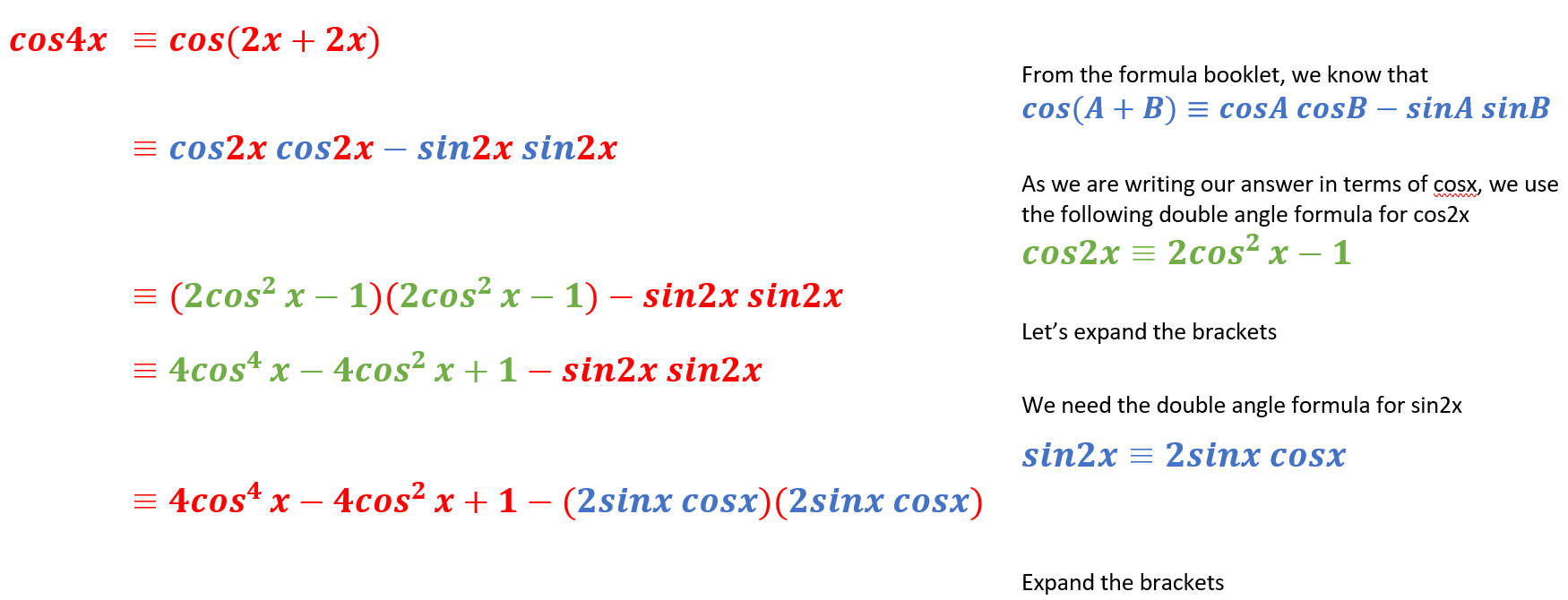
a) Write \(\large \cos4x\) in terms of \(\large\cos x\)
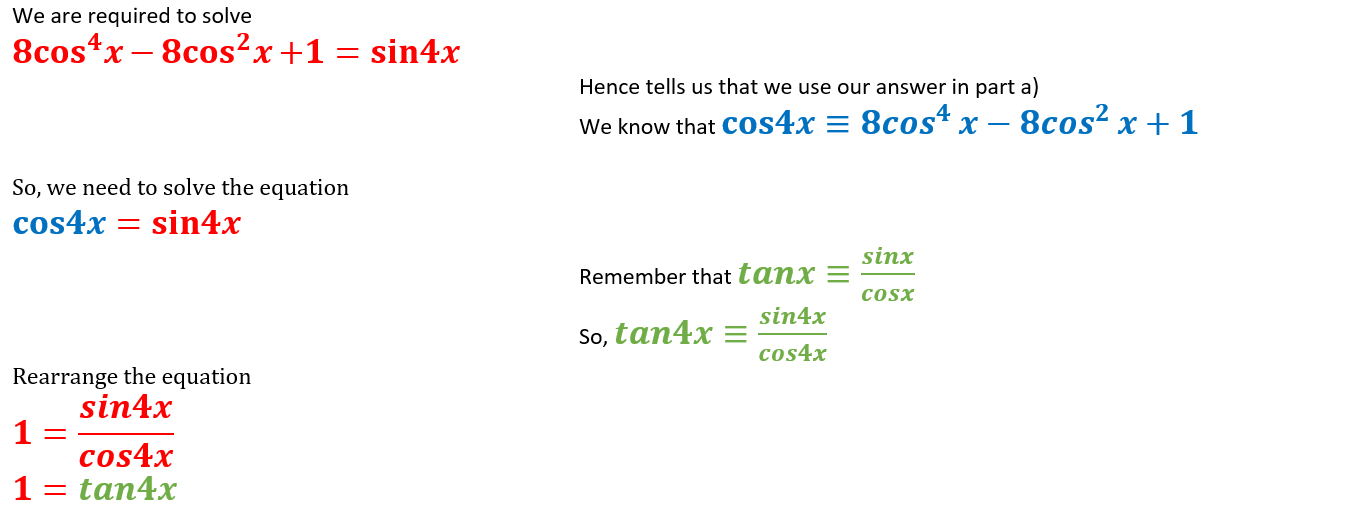
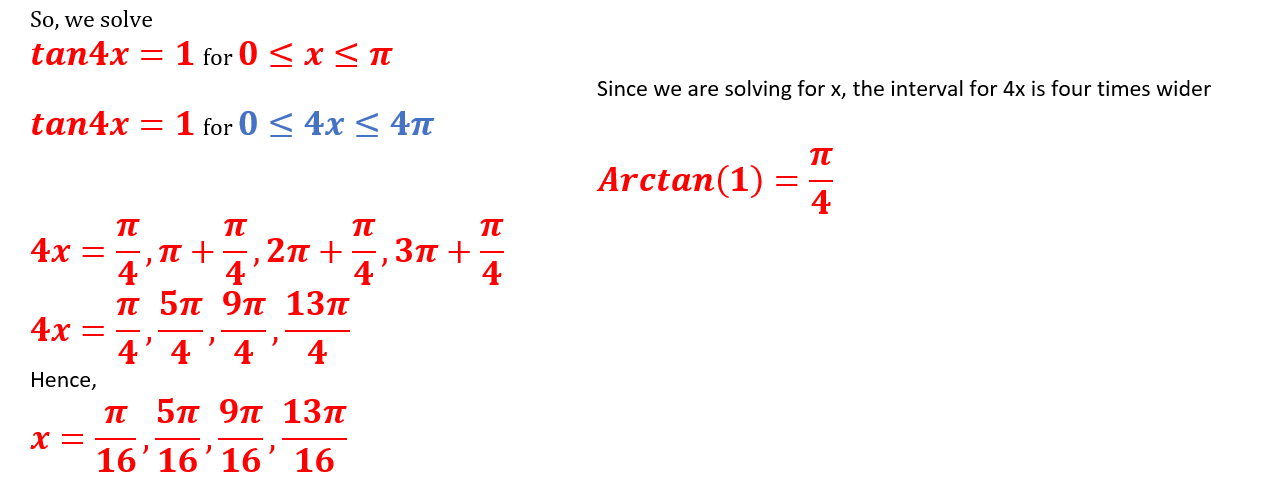
b) Hence, solve \(\large 8\cos^4x-8\cos^2x+1=\sin4x\) , for \(\large 0\le x\le\pi\)
Hint
a) Write \(\large\cos4x\equiv \cos(2x+2x)\) and use the double angle formula for \(\large \cos2x\)
b) Remember that \(\large \tan x\equiv\frac{\sin x}{\cos x}\)
Full Solution
a)
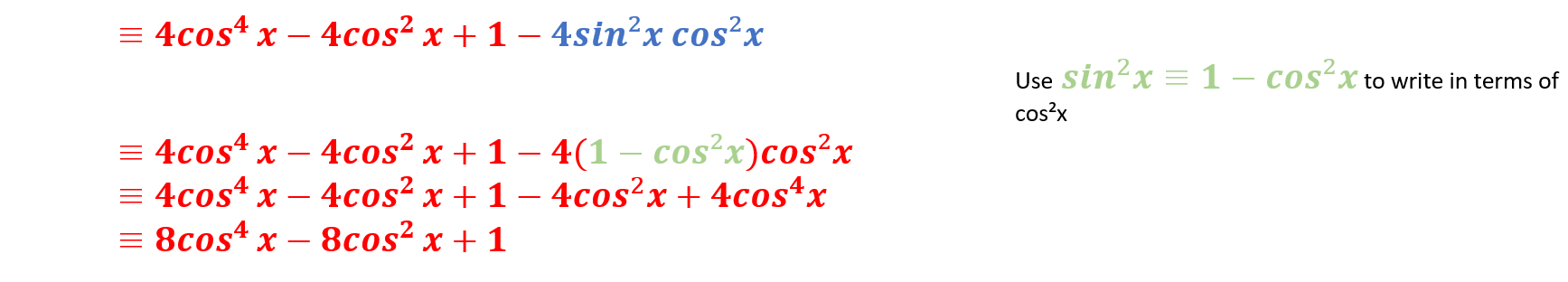
b)


Let f(x) = (cos2x - sin2x)²
a) Show that f(x) can be expressed as 1 - sin4x
b) Let f(x) = 1 - sin4x. Sketch the graph of f for \(0\le x\le \pi \)
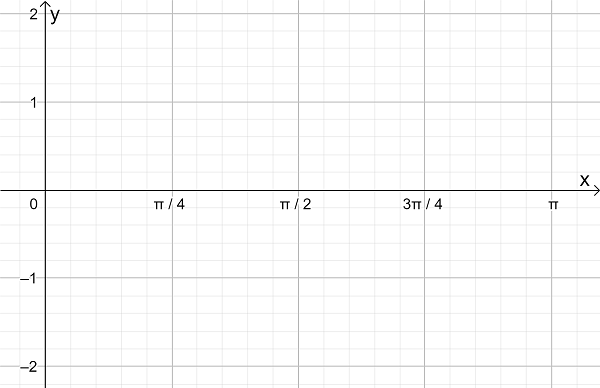
Hint
a) Expand the brackets
Consider the following identities \(cos^{ 2 }\theta +sin^{ 2 }\theta \equiv 1\\ sin2\theta \equiv 2sin\theta cos\theta \)
b) In order to sketch this function, consider the transformations from the graph of y = sinx
Full Solution
Print from here



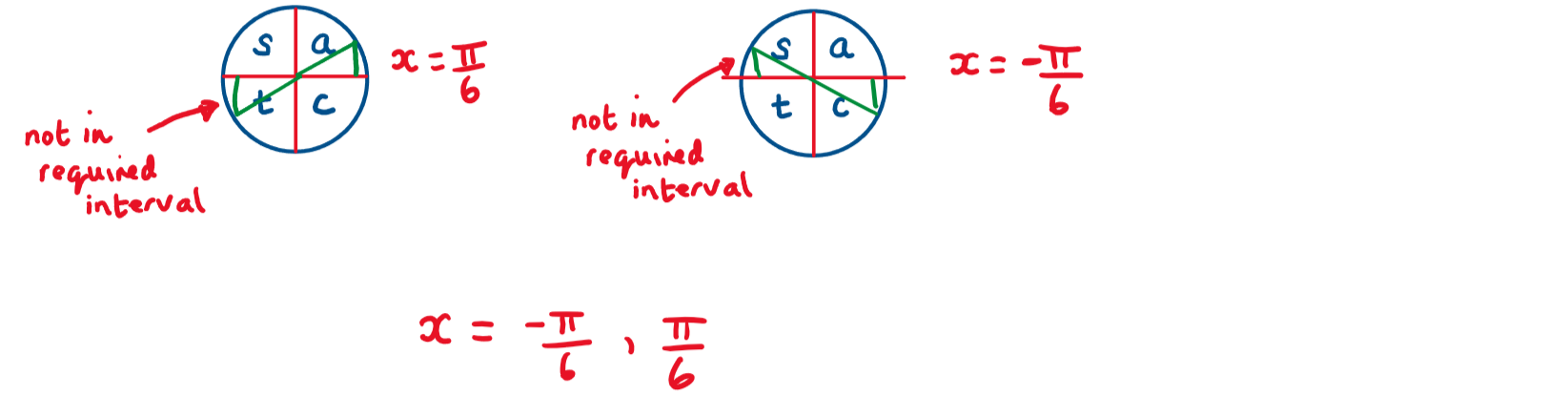
Solve \(cos2θ=sinθ\) for \(0\le \theta \le 2\pi \)
Hint
Full Solution
Print from here



a) Show that \(cos2\theta-3cos\theta+2\equiv 2{ cos }^{ 2 }\theta -3cos\theta +1\)
b) Hence, solve \(cos2\theta-3cos\theta+2=0\) for \(0\le \theta \le 2\pi \)
Hint
Full Solution
Print from here



Let \(cos\theta=\frac{2}{3}\), where \(0\le \theta \le \frac { \pi }{ 2 } \)
Find the value of
a) \(sin\theta\)
b) \(sin2\theta\)
c) \(sin4\theta\)
Hint
a) Draw a triangle and work out \(sin\theta\)
c) Work out \(cos2\theta\) first. \(2\theta\) is obtuse
Full Solution
Print from here


Let f(x)= cosx and g(x) = \(\frac{2x^2}{1-x}\)
a) Show that g∘f(x) = 1 can be written as 2cos²x + cosx - 1 = 0
b) Hence solve g∘f(x)=1 for \(-\pi\le x\le \pi\)
Hint
a) Start by finding the compostive function g∘f(x).
b) This is a quadratic equation. Some students find it easier to factorise by substituting y = cosx and solving for y first.
Full Solution
Print from here


Solve \(\log _{ 3 }{ sinx-\log _{ 3 }{ cosx=0.5 } } \) for \(0\le x\le 2\pi\)
Hint
Use log laws to simlify equation: \(\log _{ a }{ \frac { x }{ y } =\log _{ a }{ x } -\log _{ b }{ y } } \)
Full Solution
Print from here


1 + cosx + cos²x + cos3x + ... = \(2 + \sqrt2\)
Find x given that \(-\frac {\pi}{2}\le x\le \frac {\pi}{2}\)
Hint
1 + cosx + cos²x + cos3x + ... is an infinite geometric series. Can you find the sum?
Full Solution
Print from here

A line \({ L }_{ 1 }\) passes through A(2,0,-3) and B(4,3,2).
a) Find the equation of the line \({ L }_{ 1 }\)
A second line \({ L }_{ 2}\) has equation \(\textbf{r}=\left( \begin{matrix} 2 \\ 3 \\ 5 \end{matrix} \right) +\lambda \left( \begin{matrix} 1 \\ -4 \\ k \end{matrix} \right) \)
b) Given that \({ L }_{ 1 }\) and \({ L }_{ 2 }\)are perpendicular, find k.
Hint
b) If \({ L }_{ 1 }\) and \({ L }_{ 2 }\) are perpendicular, then the scalar product of the direction vectors = 0
Full Solution
Print from here

\(\overrightarrow { AB }\) and \(\overrightarrow { AC }\) are two vectors such that \(\overrightarrow { AB } =\left( \begin{matrix} 3 \\ -1 \\ 2 \end{matrix} \right) \) and \(\overrightarrow { AC } =\left( \begin{matrix} 2 \\ 0 \\ 1 \end{matrix} \right) \)
Find \(\hat { BAC } \) to the nearest degree.
Hint
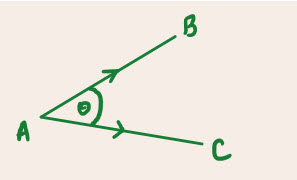
\(cos\theta =\frac { a\cdot b }{ \left| a \right| \left| b \right| } \)
Full Solution
Print from here

The angle between the line \({ L }_{ 1 }\) and \({ L }_{ 2 }\) is \(\frac{\pi}{2}\).
\( { L }_{ 1 }:\quad \frac { x+2 }{ 3 } =2y+1=\frac { 5-z }{ 2 } \)
\( { L }_{ 2 }: \quad x =\frac { y-2}{ 3} =kz \)
Find k.
Hint
\(\frac { x-{ x }_{ 0 } }{ l } =\frac { y-{ y }_{ 0 } }{ m } =\frac { z-{ z }_{ 0 } }{ n } \)
Full Solution
Print from here

Find the angle between the planes \({ \Pi }_{ 1 }\) and \({ \Pi }_{ 2 }\) to the nearest degree.
\({ \Pi }_{ 1 }: 2x-3y+z=0\)
\({ \Pi }_{ 2 }: x+2y+5z=-4\)
Hint
Full Solution
Print from here

Find the value of x for which the vectors \(\left( \begin{matrix} sinx \\ \sqrt{3} \\ 0 \end{matrix} \right) \) and \(\left( \begin{matrix} 4cosx \\-1\\ 2 \end{matrix} \right) \)are perpendicular, \(0\le x\le \frac { \pi }{ 2 } \).
Hint
Full Solution
Print from here

OABC is a parallelogram.
\(\overrightarrow { OA } =\textbf{a}\) \(\overrightarrow { OB } =\textbf{b}\) \(\overrightarrow { OC } =\textbf{a}+\textbf{b}\)
Given that \((\textbf{ a }+\textbf{ b })\cdot (\textbf{ a }-\textbf{ b })=0\) what can you conclude
Hint
- The distributive law holds for the scalar product
- \(\textbf{ a }\cdot \textbf{ a }={ \left| \textbf{a} \right| }^{ 2 }\)
Full Solution
Print from here

 ACB is a right-angled triangle
ACB is a right-angled triangle
\(\overrightarrow { CB } = \textbf {a }\) \(\overrightarrow { AC } = \textbf {b }\)
a) Write \(\overrightarrow { AB } \) in terms of a and b
b) Find \(\textbf{ a }\cdot \textbf{ b }\)
c) Show that \({ \left| \textbf { a+b } \right| }^{ 2 }={ \left| \textbf { a } \right| }^{ 2 }+{ \left| \textbf{ b } \right| }^{ 2 }\) and hence prove Pythagoras' Theorem.
Hint
Full Solution
Print from here

A line L passes through the points A(1,-1,3) and B(3,-4,4)
Point C (x,y,1) also lies on the line L. Find x and y.
Hint
Full Solution
Print from here


A line L passes through the points A(0,2,-4) and B(3,-3,2)
Point C also lies on the line L. Find the coordinates of C given that \(\left| \overrightarrow { AC } \right| =\left| \overrightarrow { AB } \right| \)
Hint
Draw a diagram!
Find the equation of the straight line. What is the value of \(\lambda \) that defines the position of B? Think about what this value should be for C.
Full Solution
Print from here


A line L passes through the points A(0,2,-4) and B(3,-3,2)
Point C also lies on the line L. Find the possible coordinates of C given that \(\left| \overrightarrow { AC } \right| =2\left| \overrightarrow { AB } \right| \)
Hint
Draw a diagram!
Is there just one answer?
Find the equation of the straight line. What is the value of \(\lambda \) that defines the position of B? Think about what this value should be for C.
Full Solution
Print from here

A line L1 passes through the points P(-13,-6,1) and Q(3,2,-3).
A second line L2 has equation \(\textbf{r}=\left( \begin{matrix} 9\\12 \\ 2 \end{matrix} \right) +s\left( \begin{matrix} -3 \\ 2\\4 \end{matrix} \right) \)
- Show that \(\overrightarrow{PQ}=\left( \begin{matrix} 16 \\ 8\\-4 \end{matrix} \right) \)
- Hence, write down the equation L1 in the form \(\textbf{r}=\textbf{a}+t \textbf{b}\)
- The lines L1 and L2 intersect at the point R. Find the coordinates of R.
Hint
Full Solution
Print from here

- Find \(\overrightarrow{AC}\)
- Show that \(\overrightarrow{BD}\) is perpendicular to \(\overrightarrow{AC}\)
- Write down the equation of the line (AC) in the form
- Write down the equation of the line (BD)
- The lines (AC) and (BD) intersect at E. Find the coordinates of E
Hint
b. Use the scalar product to show that the lines are perpendicular
Full Solution
Print from here

Two boats A and B, move so that a time t hours, their positions, in kilometres, are given by
\(\textbf{r}_{A}=\left( \begin{matrix} -2 \\ -12 \end{matrix} \right) +t\left( \begin{matrix} 2 \\ -4 \end{matrix} \right) \)
\(\textbf{r}_{B}=\left( \begin{matrix} 11 \\ -11 \end{matrix} \right) +t\left( \begin{matrix} -2 \\ 3 \end{matrix} \right) \)
- Find the position where the two boats cross.
- Show that the boats do not collide.
Hint
The key to answering this question correctly is to make the assumption that they will have the same position at some time, but that the time is not the same for the two boats.
Use tA and tB
Full Solution
Print from here

Two boats A and B, move so that a time t hours, their positions, in kilometres, are given by
\(\textbf{r}_{A}=\left( \begin{matrix} -2 \\ -12 \end{matrix} \right) +t\left( \begin{matrix} 2 \\ -4 \end{matrix} \right) \)
\(\textbf{r}_{B}=\left( \begin{matrix} 11 \\ -11 \end{matrix} \right) +t\left( \begin{matrix} -2 \\ 3 \end{matrix} \right) \)
- Find the position where the two boats cross.
- Show that the boats do not collide.
Hint
The key to answering this question correctly is to make the assumption that they will have the same position at some time, but that the time is not the same for the two boats.
Use tA and tB
Full Solution
Print from here

Two boats A and B, move so that a time t hours, their positions, in kilometres, are given by
\(\textbf{r}_{A}=\left( \begin{matrix} -2 \\ -12 \end{matrix} \right) +t\left( \begin{matrix} 2 \\ -4 \end{matrix} \right) \)
\(\textbf{r}_{B}=\left( \begin{matrix} 11 \\ -11 \end{matrix} \right) +t\left( \begin{matrix} -2 \\ 3 \end{matrix} \right) \)
- Find the position where the two boats cross.
- Show that the boats do not collide.
Hint
The key to answering this question correctly is to make the assumption that they will have the same position at some time, but that the time is not the same for the two boats.
Use tA and tB
Full Solution
Print from here

The line L1 has equation \(\textbf{r}=\left( \begin{matrix} 2\\-1 \\ 3 \end{matrix} \right) +t\left( \begin{matrix} -1 \\ -2\\k \end{matrix} \right) \)
The line L2 has equation \(\textbf{r}=\left( \begin{matrix} 2\\1 \\ -3 \end{matrix} \right) +t\left( \begin{matrix} 2 \\ -1\\-1 \end{matrix} \right) \)
- The point A(3,1,-1) lies on the line L1. Show that k = 4.
- Show that the lines and L1 are L2 perpendicular.
- Show that the lines and L1 do not L2 intersect.
- The point B lies on the line The point C has coordinates (2,1,-3). ABC forms an isosceles triangles with AC=BC. Find the coordinates of B.
Hint
d. You should be able to work out the length of AC
Consider that B lies on the line L1 . Find the vector \(\overrightarrow{BC}\)

Full Solution
Print from here


During an air show, two planes, A and B, perform a manoeuvre in which their paths cross in a near miss. The two planes are flying at the same altitude.
\(\textbf{ r }_{ A }=\left( \begin{matrix} 150 \\ 320 \end{matrix} \right) +t\left( \begin{matrix} 200 \\ 300 \end{matrix} \right) \)
\(\textbf{ r }_{ B }=\left( \begin{matrix} 875 \\ 110 \end{matrix} \right) +t\left( \begin{matrix} -100 \\ 400 \end{matrix} \right) \)
t = time in seconds. Distances are given in metres.
a) Show that the two planes cross paths, but the planes do not collide
b) Find the distance between the planes when t = 0.
c) Show that the distance d between A and B at any time t can be given by the expression
d = \(\sqrt { 100000{ t }^{ 2 }-477000t+569725 } \)
d) To the nearest metre, find the closest distance that the two planes get to one another.
Hint

a) To decide if the planes collide, find time, t1 when xA=xB . Then find time, t2 when yA=yB. Show t1 \(\neq \) t2
b) Use Pythagoras' Theorem to find the distance between the points
d) Use you calculator!
Full Solution
Print from here

Distances in this question are given in metres
Two brothers, Orville and Wilbur are testing their model airplanes. The position of Orville's airplane t seconds after taking off from ground level is given by
\(\textbf{ r }=\left( \begin{matrix} 12 \\ -19\\0 \end{matrix} \right) +t\left( \begin{matrix} -4 \\ 4\\3 \end{matrix} \right) \)
a) Find the height of the plane after 4 seconds.
b) Wilbur's airplane takes off after Orville's airplane s seconds after taking off is given by
\(\textbf{ r }=\left( \begin{matrix} -26 \\ 25\\0 \end{matrix} \right) +s\left( \begin{matrix} 2 \\ -4\\8 \end{matrix} \right) \)
Find the angle between the two paths.
c) The two airplanes collide at (-20,13,24). How long after Orville’s airplane takes off does Wilbur’s airplane take off?
d) Find the speed of the two airplanes at the moment of the collision.
Hint
Full Solution
Print from here

All distances in this question are in km.
An interceptor missile, M1 is positioned at the origin. A missile, M2 is launched from (-20,7) with velocity \(\left( \begin{matrix} 3 \\ 1 \end{matrix} \right) \) kms-1 . M1 is capable of twice the speed of M2 . How many seconds later must the interceptor missile, M1 be launched if it is to travel the shortest possible distance?
Hint
Full Solution
Print from here

a = \(\left( \begin{matrix} 2 \\ 3 \\ -5 \end{matrix} \right) \) and b = \(\left( \begin{matrix} 3 \\ -2 \\ 4 \end{matrix} \right) \)
Find \(\textbf{a}\times \textbf{b}\)
Hint
\(\textbf{v}\times \textbf{w}=\left( \begin{matrix} { \textbf{v} }_{ 1 } \\ { \textbf{v} }_{ 2 } \\ { \textbf{v} }_{ 3 } \end{matrix} \right) \times \left( \begin{matrix} { \textbf{w} }_{ 1 } \\ { \textbf{w} }_{ 2 } \\ { \textbf{w} }_{ 3 } \end{matrix} \right) =\left( \begin{matrix} { \textbf{v} }_{ 2 }{ \textbf{w} }_{ 3 }-{ \textbf{v} }_{ 3 }{ \textbf{w} }_{ 2 } \\ { \textbf{v} }_{ 3 }{ \textbf{w} }_{ 1 }-{ \textbf{v} }_{ 1 }{ \textbf{w} }_{ 3 } \\ { \textbf{v} }_{ 1 }{ \textbf{w} }_{ 2 }-{ \textbf{v} }_{ 2 }{ \textbf{w} }_{ 1 } \end{matrix} \right)\)
a x b is perpendicular to a and b. Check your answer by showing \((\textbf{a}\times \textbf{b})\cdot \textbf{a}=0\) and \((\textbf{a}\times \textbf{b})\cdot \textbf{b}=0\)
Full Solution
Print from here

a = 3i + 2j + k and b = 2i + 3j + 2k
Find a unit vector that is perpendicular to a and b
Hint
Use the vector product
\(\textbf{v}\times \textbf{w}=\left( \begin{matrix} { \textbf{v} }_{ 1 } \\ { \textbf{v} }_{ 2 } \\ { \textbf{v} }_{ 3 } \end{matrix} \right) \times \left( \begin{matrix} { \textbf{w} }_{ 1 } \\ { \textbf{w} }_{ 2 } \\ { \textbf{w} }_{ 3 } \end{matrix} \right) =\left( \begin{matrix} { \textbf{v} }_{ 2 }{ \textbf{w} }_{ 3 }-{ \textbf{v} }_{ 3 }{ \textbf{w} }_{ 2 } \\ { \textbf{v} }_{ 3 }{ \textbf{w} }_{ 1 }-{ \textbf{v} }_{ 1 }{ \textbf{w} }_{ 3 } \\ { \textbf{v} }_{ 1 }{ \textbf{w} }_{ 2 }-{ \textbf{v} }_{ 2 }{ \textbf{w} }_{ 1 } \end{matrix} \right)\)
A unit vector is a vector with magnitude = 1
a x b is perpendicular to a and b. Check your answer by showing \((\textbf{a}\times \textbf{b})\cdot \textbf{a}=0\) and \((\textbf{a}\times \textbf{b})\cdot \textbf{b}=0\)
Full Solution
Print from here

The area of a parallelogram formed by two adjacent vectors a and b is 7 square units.
a = \(\left( \begin{matrix} -3 \\ 4 \\ k \end{matrix} \right) \) b = \(\left( \begin{matrix} 3 \\ -2 \\ -2 \end{matrix} \right) \)
Find k
Hint
Area of parallelogram = \(\left| \textbf{a}\times \textbf{b} \right| \)
Full Solution
Print from here

Given that \(\textbf{u}\times \textbf{v} = \textbf{u}\times \textbf{w}\) show that \(\textbf{v}- \textbf{w} \) is parallel to \(\textbf{u}\)
Hint
If \(\textbf{a}\times \textbf{b}=0\) then a and b are parallel
Full Solution
\(\textbf{u}\times \textbf{v} = \textbf{u}\times \textbf{w}\)
\(\textbf{u}\times \textbf{v} - \textbf{u}\times \textbf{w}=0\)
\(\textbf{u}\times ( \textbf{v} - \textbf{w})=0\)
Since the vector product is zero, the vectors are parallel

a) For any two vectors v and w prove Lagrange's Identity
\({ \left| \textbf{v}\times \textbf{w} \right| }^{ 2 }+{ \left( \textbf{v}\cdot \textbf{w} \right) }^{ 2 }={ \left| \textbf{v} \right| }^{ 2 }{ \left| \textbf{w} \right| }^{ 2 }\)
b) Hence, find \(\textbf{v}\cdot \textbf{w}\) if
\({ \left| \textbf{v} \right| }=3\)
\({ \left| \textbf{w} \right| }=4\)
\(\textbf{v}\times \textbf{w} =\left( \begin{matrix} -1 \\ 2 \\ 3 \end{matrix} \right) \)
Hint
Look in formula booklet for formulae for \({ \left| \textbf{v}\times \textbf{w} \right| }\) and \(\textbf{v}\cdot \textbf{w}\)
Full Solution
Print from here

The points A, B and C are given by the position vectors a, b and c.
If A, B and C are collinear, show that
\(\textbf{b}\times \textbf{c}=\textbf{a}\times (\textbf{c}-\textbf{b})\)
Hint
If A, B and C are collinear, then \(\overrightarrow { AB } \) is parallel to \(\overrightarrow { BC } \)
What do you know about the vector product of parallel vectors?
Full Solution
Print from here

a and b are vectors
Show that \(|\textbf{a}×\textbf{b}|^{ 2 }+|\textbf{a} ∙\textbf{b}|^{ 2 }=(\textbf{a}\textbf{b})^{ 2 }\)
Hint
Full Solution
Print from here

A plane has vector equation \(\textbf{ r }=\left( \begin{matrix} 1 \\ 2 \\ 0 \end{matrix} \right) +\mu \left( \begin{matrix} 2 \\ 1 \\ 1 \end{matrix} \right) +\lambda \left( \begin{matrix} 3 \\ 0 \\ -1 \end{matrix} \right) \)
Show that the Cartesian equation of the plane is x - 5y + 3z + 9 = 0
Hint
Full Solution
Print from here

The coordinates of points A, B and C are given as (5,4,1) , (5,1,-2) and (1,-1,2) respectively.
a) Find the equation of the plane that passes through A, B and C
b) Find the equation of the plane that is perpendicular to AB and passes through C
Hint
b) The normal to the plane is vector \(\overrightarrow { AB }\)
Full Solution
Print from here

A plane has vector equation \(\textbf{ r }=\left( \begin{matrix} 1 \\ 2 \\ 0 \end{matrix} \right) +\mu \left( \begin{matrix} -2 \\ 0 \\ 5 \end{matrix} \right) +\lambda \left( \begin{matrix} 0 \\ -4 \\ 5 \end{matrix} \right) \)
a) Find the Cartesian equation of the plane
b) The plane meets the x, y and z axes at A, B and C respectively. OABC forms a pyramid. Find the volume of the pyramid.
Hint
b) The plane crosses the x axis when y = z = 0. Find the corrdinates of A. Similarly, find coordinates of B and C.
Full Solution
Print from here

Find the Cartesian equation of the plane that is perpendicular to the plane 2x - y + z = 8 and contains the points A(4,2,-3) and B(6,1,-1).
Hint
Full Solution
Print from here


\({ \Pi }_{ 1 }:\quad \quad x+2y−z=5\\ { \Pi }_{ 2 }:\quad \quad 2x−y+3z=−4\)
Hint
1. Eliminate z and write y in terms of x
3. Write parametric equations
x = ...
y = ...
z = ...
4. Convert to vector form
Full Solution
Print from here

\(\ \ \ x + \ \ y +\ \ z =8\\ \ \ ax − \ y \ \ \quad \ \ \ =3\\ −x+3y+4z=b\)
- There is no unique solution solution to the system of equations. Find the value of a.
- Given that the system can be solved, find the value of b.
Hint
2. Equate the coefficients of y with equation from above and equation 2
Full Solution
Print from here


\({ \Pi }_{ 1 }:\quad 2x+\ y+3z=a\\ { \Pi }_{ 2 }:\quad \ x−2y+2z=−9 \\ { \Pi }_{ 3 }:\quad 3x+4y+4z=−1\)
- Find a
- Find the equation of the straight line in the form \(\textbf {r}=\textbf {a}+\lambda\textbf {b}\) where the components of b are integers
Hint
- Combine the equations to eliminate x
- Equate the coefficients of y and z
Full Solution
Print from here


\(x +2y+kz=−1\\ 2x+ \ y− \ z=3\\ kx−2y+ z=1\)
Hint
Combine the equations to eliminate y
Full Solution
Print from here

The points A and B are given by A(-8,1,-2) and B(-2,-1,2).
A plane Π is defined by the equation \(2x−y−3z=−8\)
- Find a vector equation of the line L passing through the points A and B.
- Find the coordinates of the point of intersection of the line and the plane.
Hint
To find the equation of the line, you will need to find \(\overrightarrow{AB}\)
\(\overrightarrow{AB}=\overrightarrow{OB}-\overrightarrow{OA}\)
Full Solution
Print from here

Consider the plane \(x−2y+4z=−15\) and the line
\(x=3+kλ\\ y=−2+λ\\ z=(2k+6)−2λ\)
The line and the plane are perpendicular. Find
- The value of k
- The coordinates of the point of intersection of the line and the plane.
Hint
If line and plane are perpendicular, then line is parallel to normal to the plane
Full Solution
Print from here

\(Π_{ 1 }\)and \(Π_{ 2 }\) are planes such that
\(Π_{ 1 }:2x−y−2z=0\)
and
\(Π_{ 2 }:−2x+3y+3z=4 \)
L is the intersection of planes \(Π_{ 1 }\) and \(Π_{ 2 }\)
- Find the equation of the line
A third plane \(Π_{ 3 }\) is defined by the equation \(kx+(k−1)y−z=5\)
- Find the value of k such that the line L does not intersect with \(Π_{ 3 }\)
Hint
If the line L does not intersect with \(Π_{ 3 }\) then they must be parallel

Here is a graph of the three planes and the line
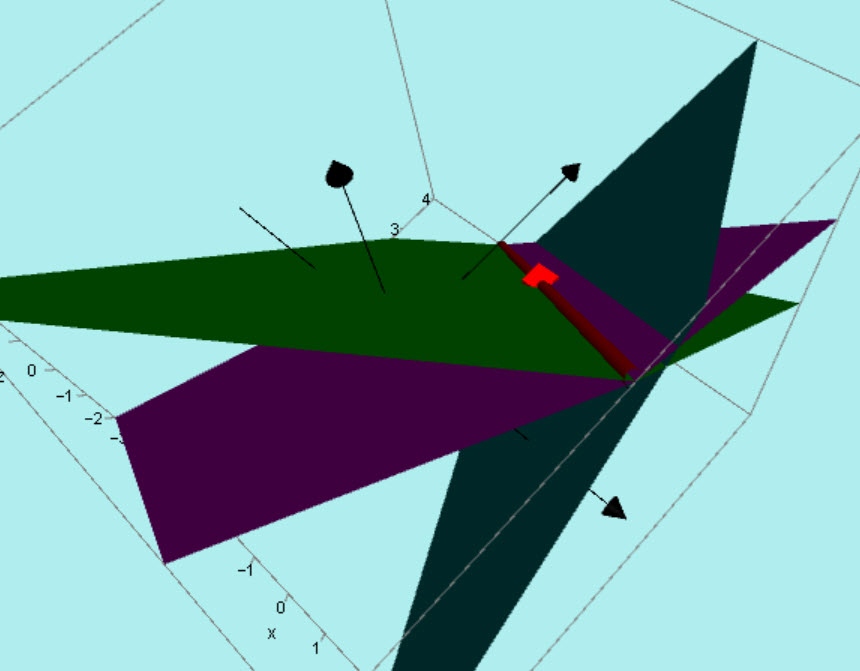
Full Solution
Print from here

The point A (3, 1, –2) is on the line L, which is perpendicular to the plane \(2x−3y−z+9=0\).
- Find the Cartesian equation of the line L.
- Find the point R which is the intersection of the line L and the plane.
- The point A is reflected in the plane. Find the coordinates of the image of A.
Hint
The points A , R and A' lie on the straight line:

Full Solution
Print from here
Feedback
Which of the following best describes your feedback?



 Twitter
Twitter  Facebook
Facebook  LinkedIn
LinkedIn