 On this page we will look at another type of differential equations: linear differential equations in the form y' + P(x)y=Q(x) which can be solved by using an integrating factor. There are many applications of this type of differential equation. For example, in electrical engineering, we can solve Kirchhoff's Laws to model electrical circuits. To be successful with this topic, you will need to have strong foundations in the following areas: integration techniques (especially in the form \(\int\frac{f'(x)}{f(x)} \mathrm{d}x\)) and manipulation of logarithms and exponents. You can get some practice in these areas if you complete all the quizzes on this page before attempting the exam-style questions.
On this page we will look at another type of differential equations: linear differential equations in the form y' + P(x)y=Q(x) which can be solved by using an integrating factor. There are many applications of this type of differential equation. For example, in electrical engineering, we can solve Kirchhoff's Laws to model electrical circuits. To be successful with this topic, you will need to have strong foundations in the following areas: integration techniques (especially in the form \(\int\frac{f'(x)}{f(x)} \mathrm{d}x\)) and manipulation of logarithms and exponents. You can get some practice in these areas if you complete all the quizzes on this page before attempting the exam-style questions.
On this page, you should learn about
- solving \(\large \frac{\text{d}y}{\text{d}x}+P(x)y=Q(x)\)
- using the integrating factor \(I=e^{\int P(x)\mathrm{d}x}\)
In order to use the integrating factor method, the differential equation needs to be in the form \(\large \frac{\text{d}y}{\text{d}x}+P(x)y=Q(x)\). In the following videos we look at how the method works.
In the following video we will look at an easier question that requires the integrating factor method to solve it. In particular, we will look at how the method works and why it works. You will see that you need to be confident in quite a few areas of the HL course to be able to carry it out. In this case, we need to use integration by recognition and integration by parts.
Find the general solution to the differential equation
\(\large \frac{\text{d}y}{\text{d}x}+\frac{y}{x}=\sin x\)
in the form \(\large y=f(x)\)
Notes from the video
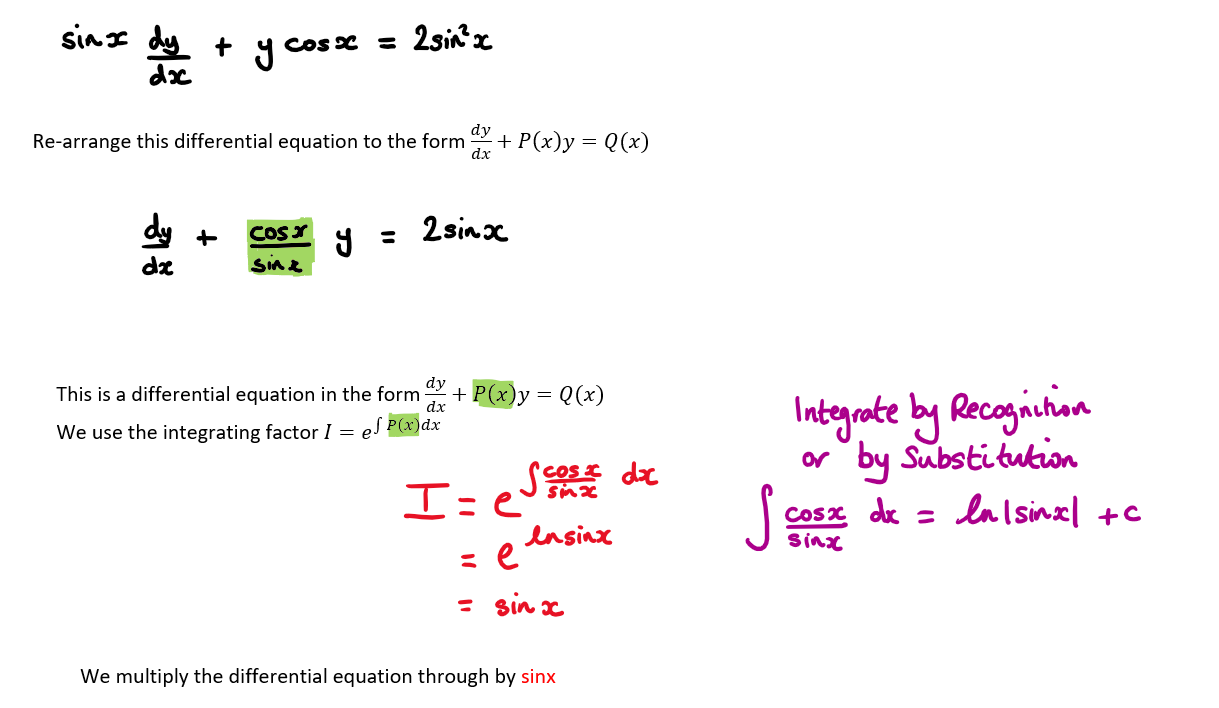
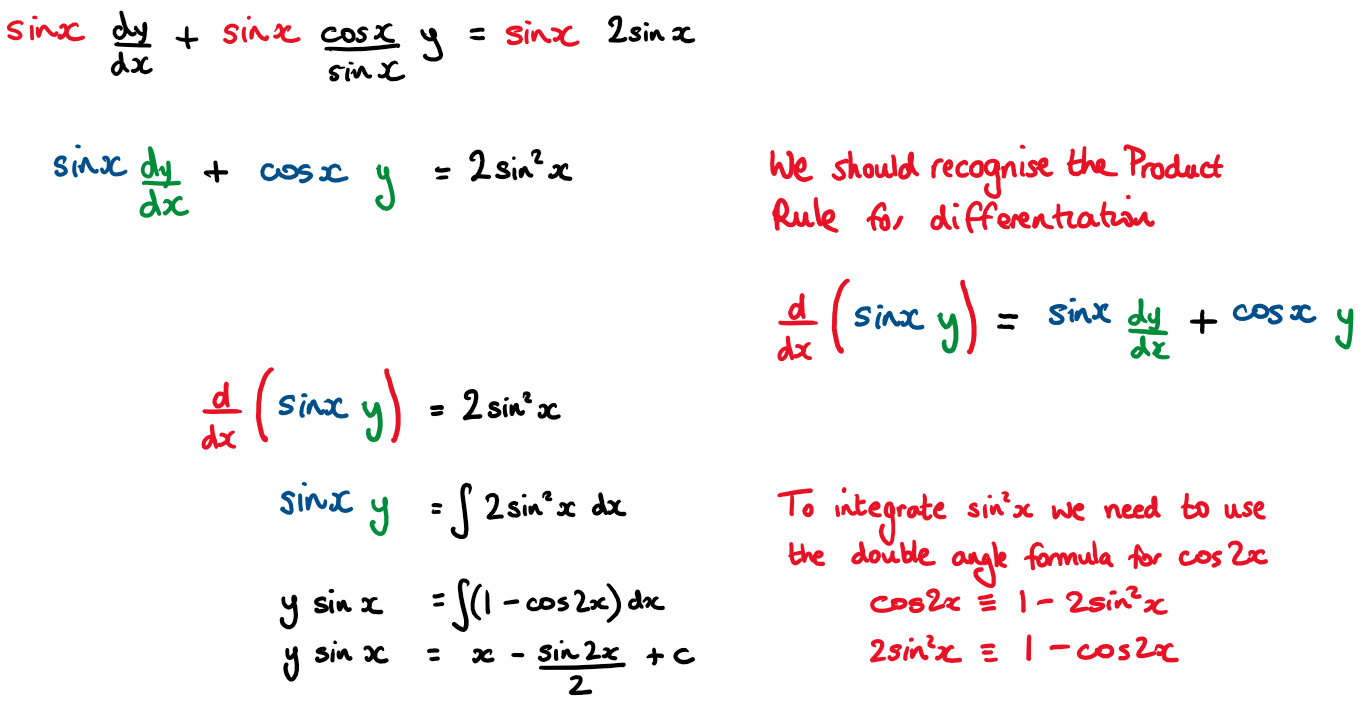
In the following video we look at how we can find the particular solution to a differential equation using an integrating factor. This example is more challenging, since we have to re-arrange the differential equation to put it in the correct form and the integration is more difficult.
Find the particular solution to the differential equation
\(\large \cos x\frac{\text{d}y}{\text{d}x}+{y}\ {\sin x}=\sin 2x\)
given that \(\large y(0)=2\)
Notes from the video
Print from here
To solve differential equations with an integrating factor, we are often required to manipulate exponents of logarithmic functions. This will give you practice in that skill
START QUIZ!
Often differential equations with an integrating factor require us to carry out integrations in the form \(\large\int\frac{f'(x)}{f(x)} \mathrm{d}x=\ln |f(x)|+C\)
The following quiz wil help you practise these type of integrals
START QUIZ!
The integrating factor, \(I\) for the differential equation in the form \(\large\frac{\mathrm{d}y}{\mathrm{d}x}+P(x)y=Q(x)\) can be found by evaluating \(\large I=e^{\int P(x) \mathrm{d}x}\)
The following quiz gives you some practice in finding the integrating factor
START QUIZ!

Consider the following first order differential equation
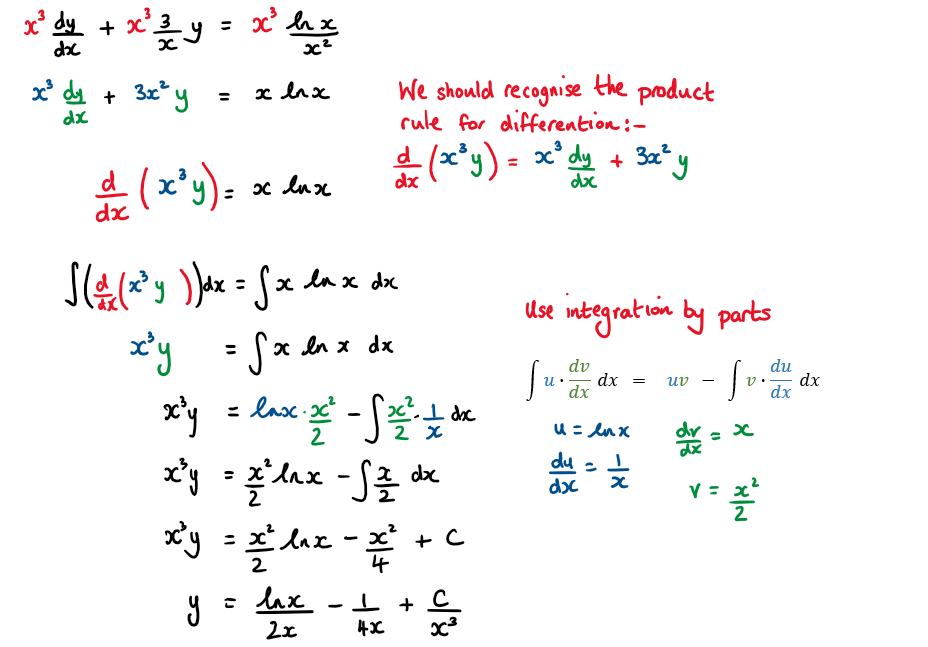
\(\large x\frac{\text{d}y}{\text{d}x}+3y=\frac{lnx}{x}\)
a) Show that x3 is the integrating factor for this differential equation
b) Hence, find the general solution of this differential equation in the form y = f(x)
Hint
Full Solution
How much of Differential Equations - Integrating Factor have you understood?



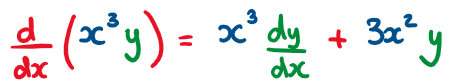
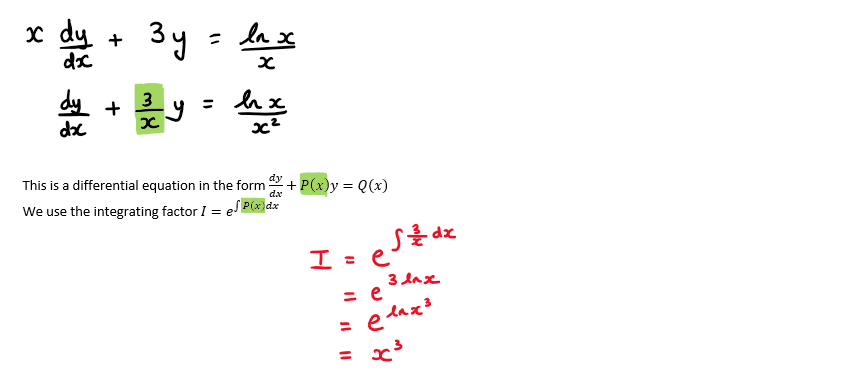

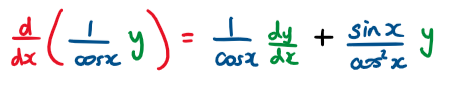
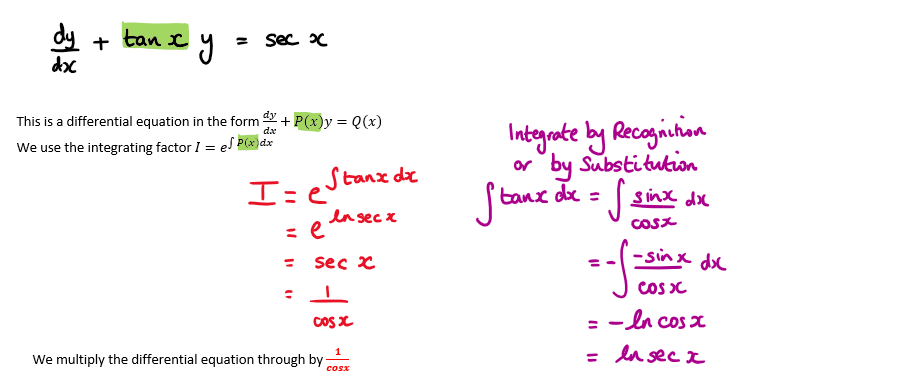

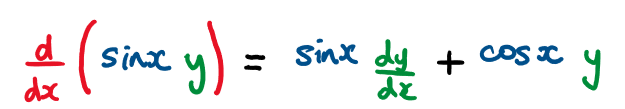

 Twitter
Twitter  Facebook
Facebook  LinkedIn
LinkedIn