
In this page, we will will learn about the Pythagorean Identities. It is actually quite rare that exam questions are solely about these identities, but it is essential that you can use and manipulate them confidently because they are used in so many different parts of the course (so they do come up a lot!). You will learn what they are and how to use them.
Key Concepts
On this page, you should learn about
- the Pythagorean identities
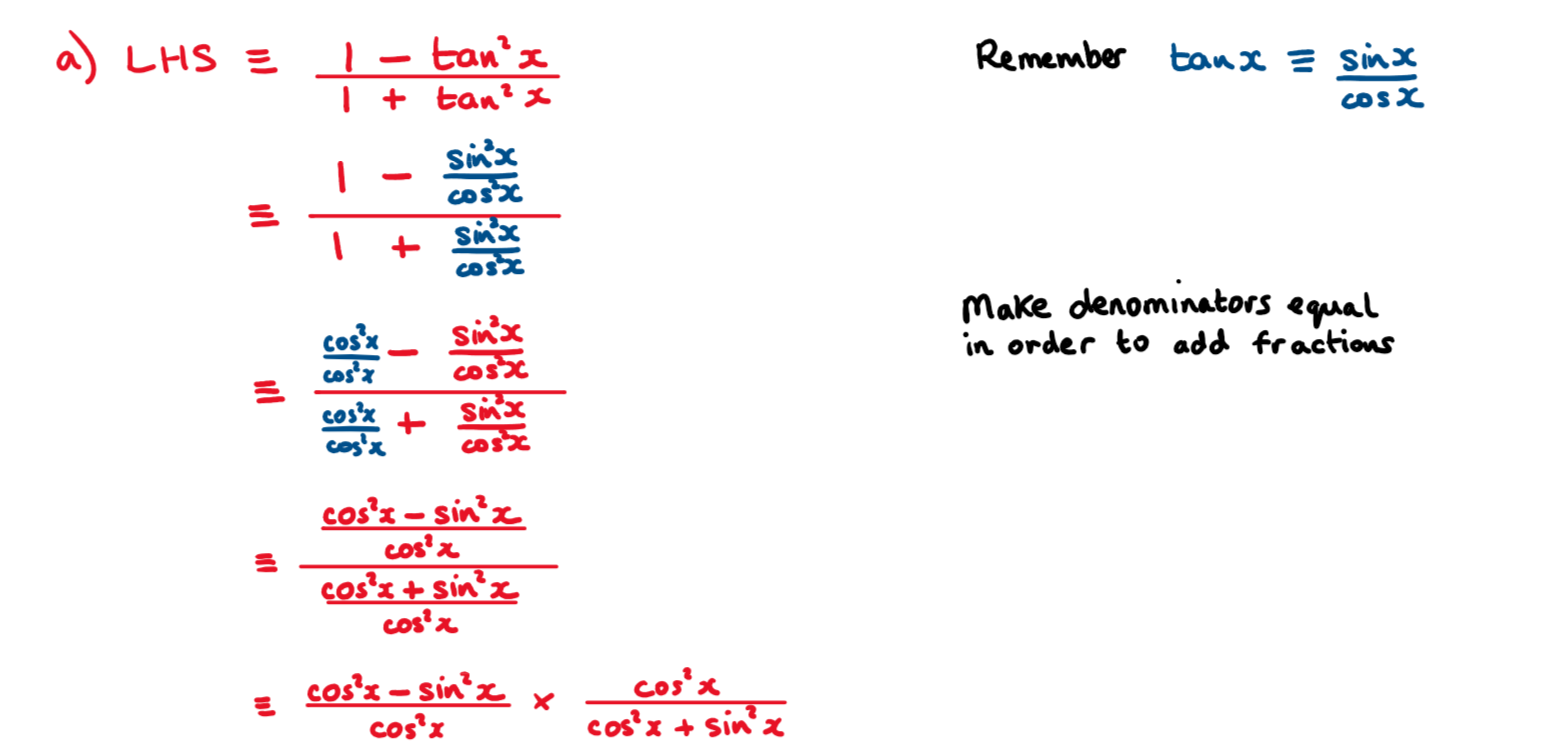
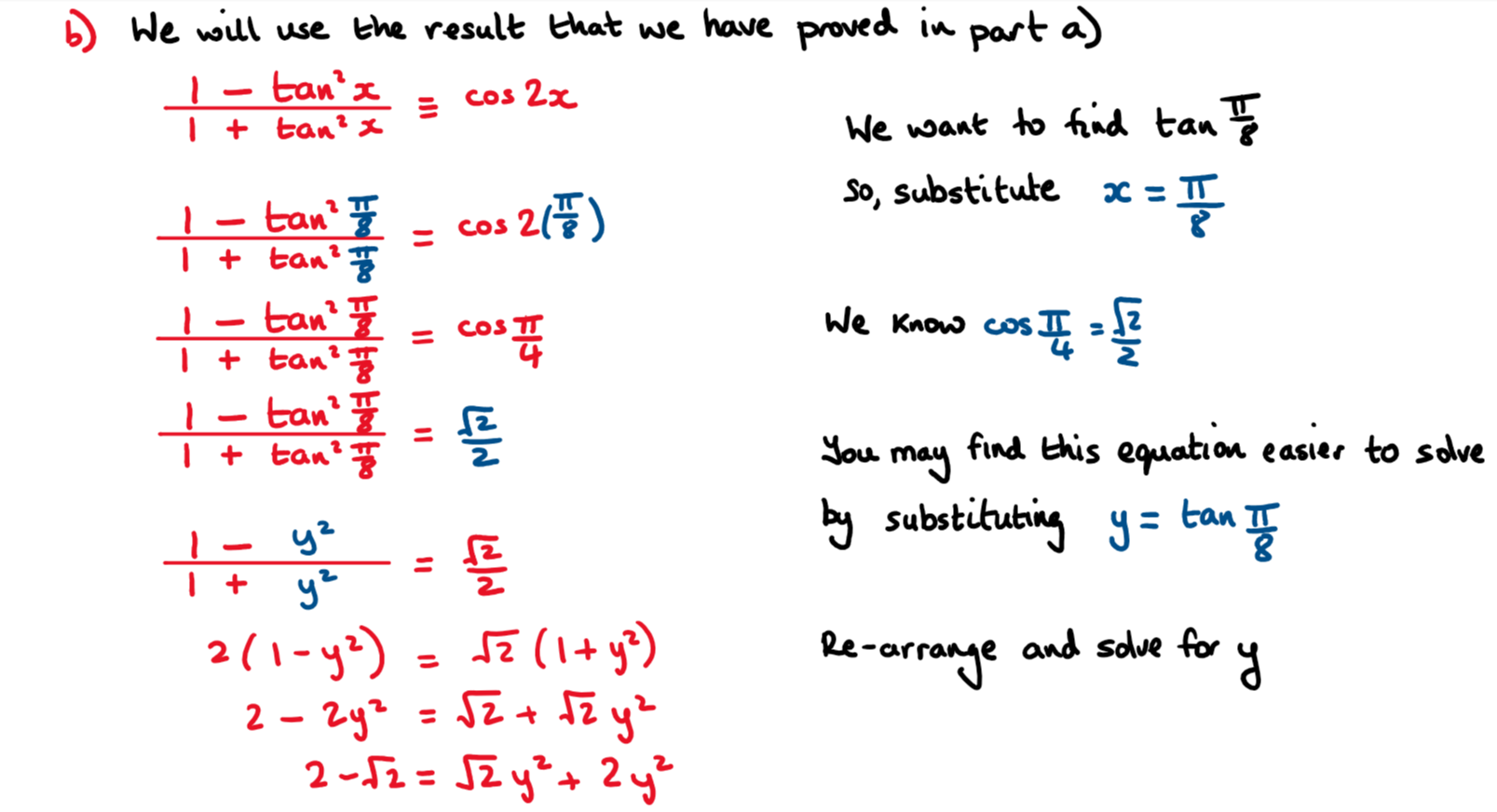
- \(\large \cos^{ 2 }\theta +\sin^{ 2 }\theta \equiv 1\)
- \(\large 1+\tan^{ 2 }\theta \equiv \sec^{2}\theta\)
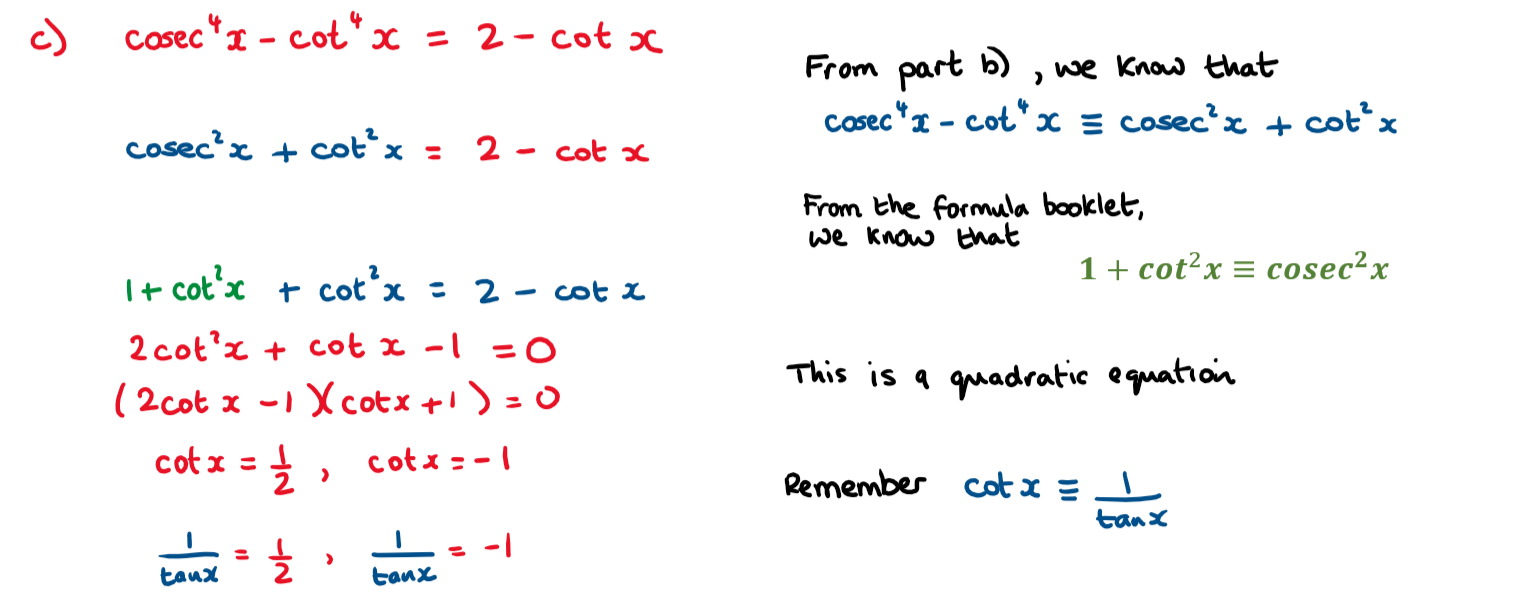
- \(\large 1+\cot^{ 2 }\theta \equiv \text{cosec}^{2}\theta\)
Print from here
MY PROGRESS
How much of Pythagorean Identities HL have you understood?


 Given that cosx = 0.7 , work out sin²x
Given that cosx = 0.7 , work out sin²x

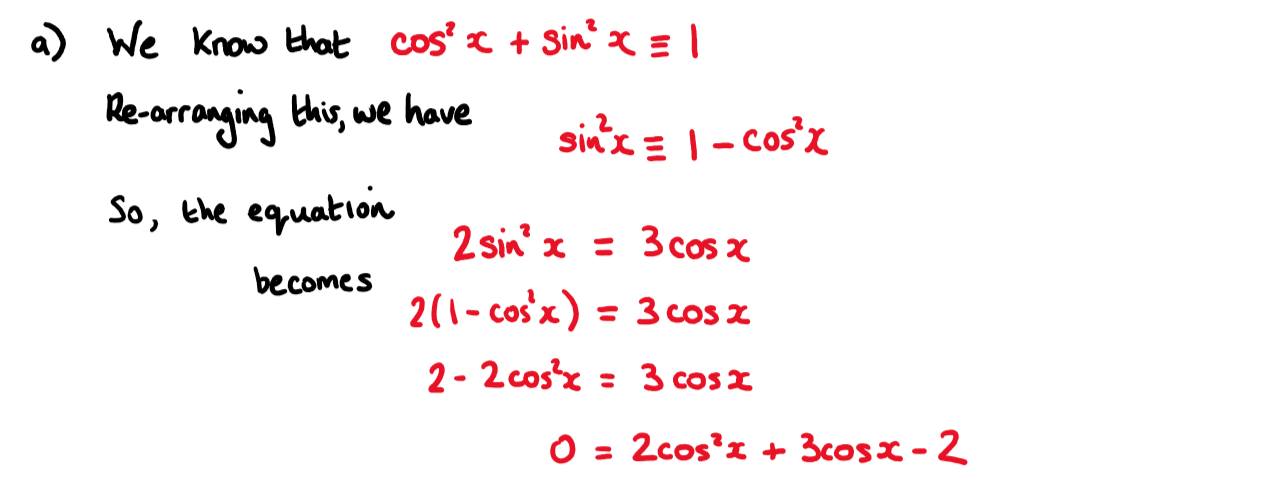

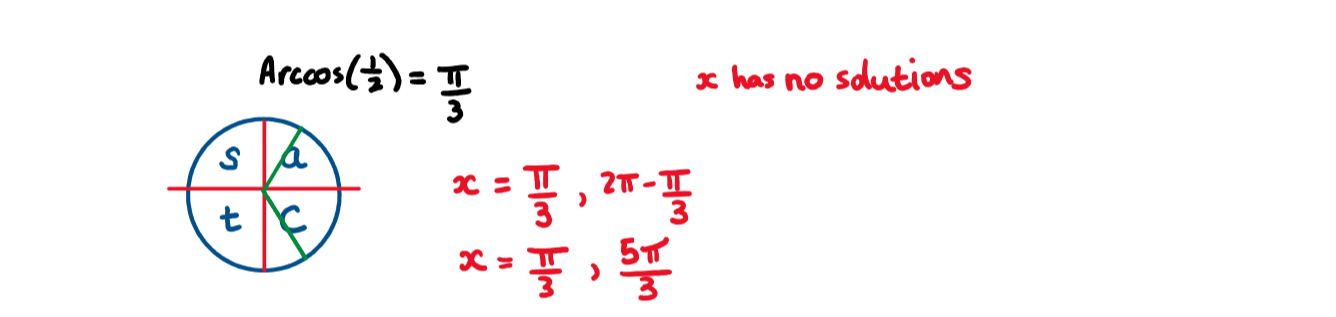


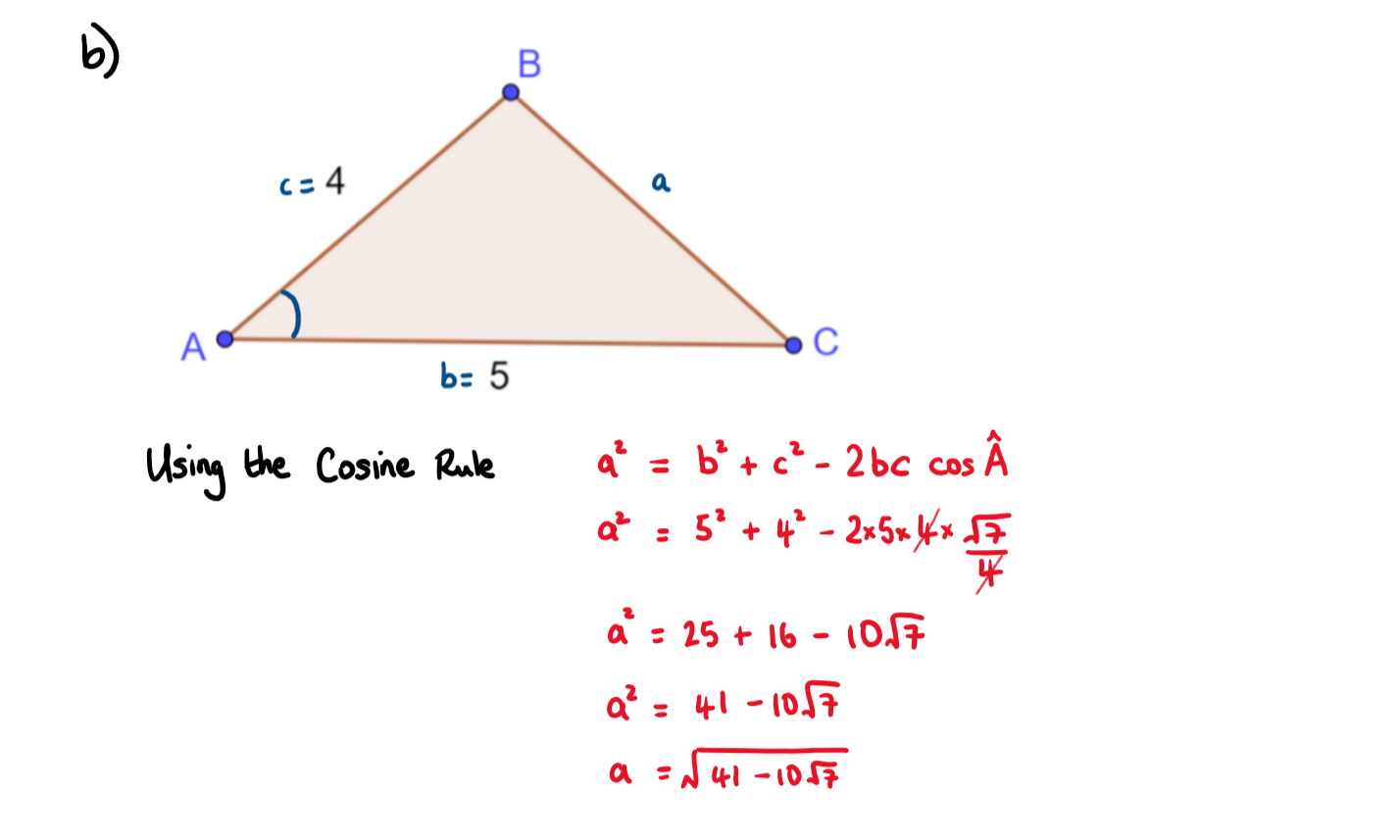
 DIAGRAM NOT TO SCALE
DIAGRAM NOT TO SCALE



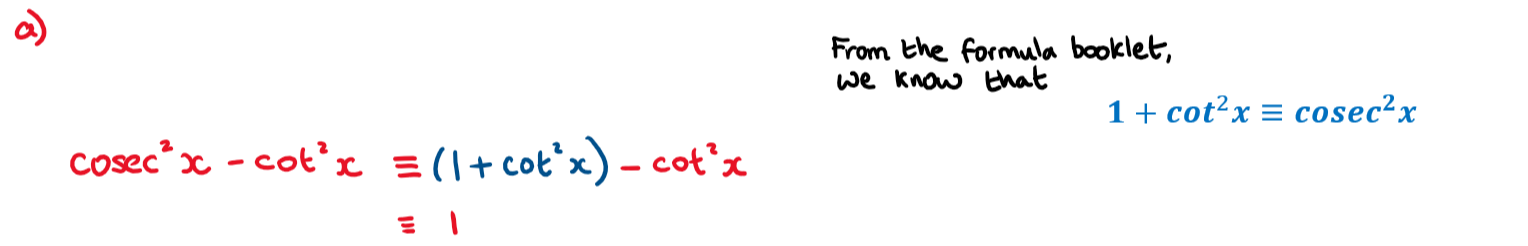
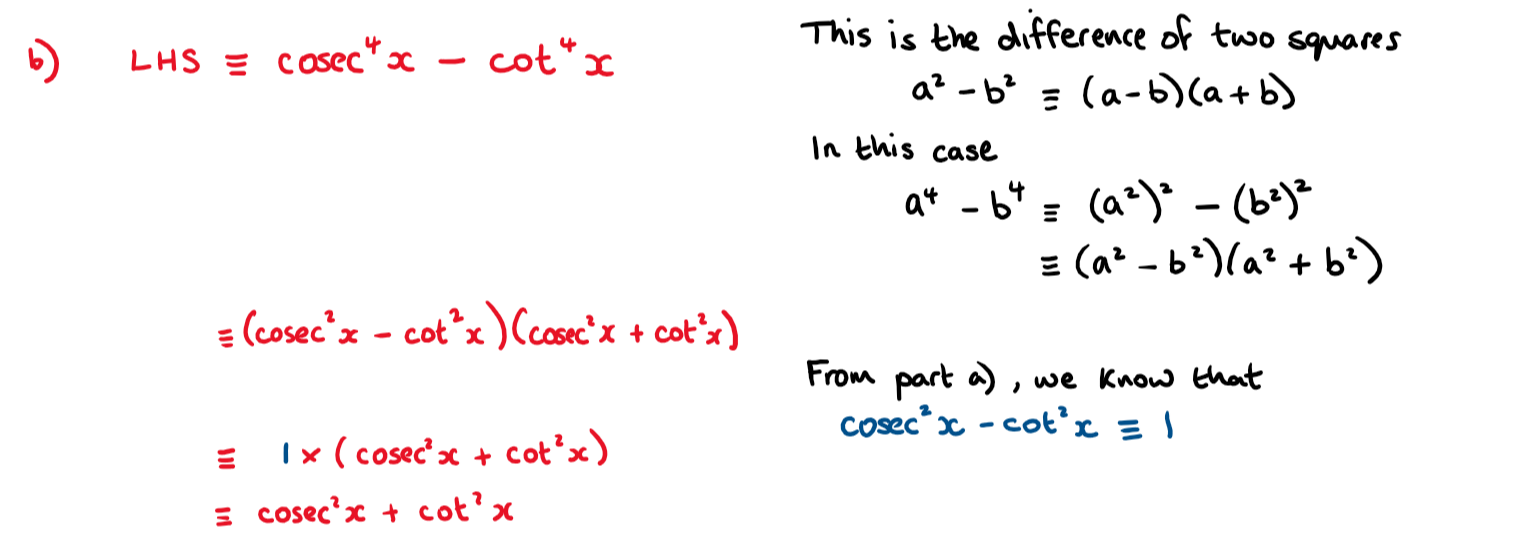


 Twitter
Twitter  Facebook
Facebook  LinkedIn
LinkedIn