 On this page we will look at quadratic expressions and how the different factorised forms link to the shape of the graph. We will also get an understanding about how the discriminant affects not only the number of roots of a quadratic equation, but also how we can use it as a tool to help us solve problems with intersections of graphs.
On this page we will look at quadratic expressions and how the different factorised forms link to the shape of the graph. We will also get an understanding about how the discriminant affects not only the number of roots of a quadratic equation, but also how we can use it as a tool to help us solve problems with intersections of graphs.
On this page, you should learn to
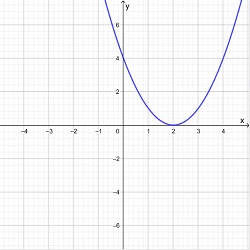
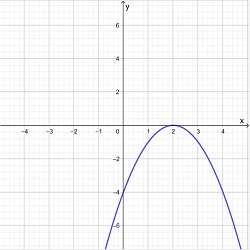
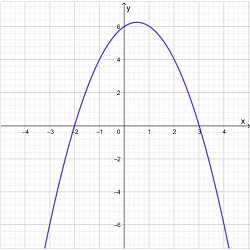
- Understand the quadratic function \(f(x)=ax^2+bx+c\) and its graph
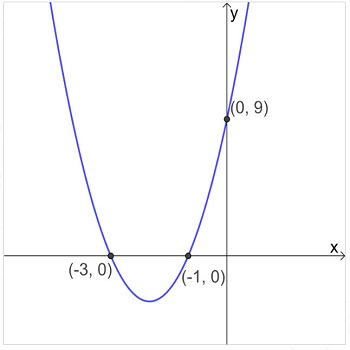
- Find and use the intercept form \(f(x)=a(x-p)(x-q)\)
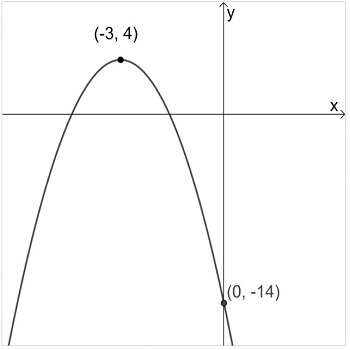
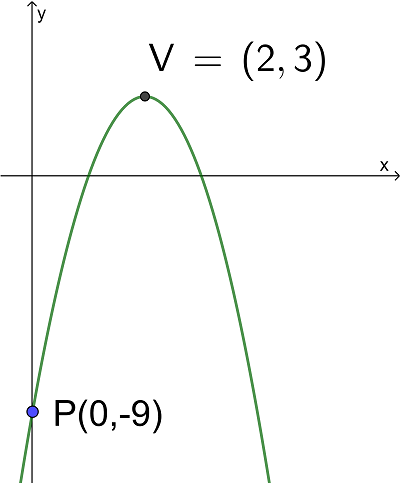
- Find and use the vertex form \(f(x)=a(x-h)^2+k\)
- Solve quadratic equations
- Solve quadratic inequalities
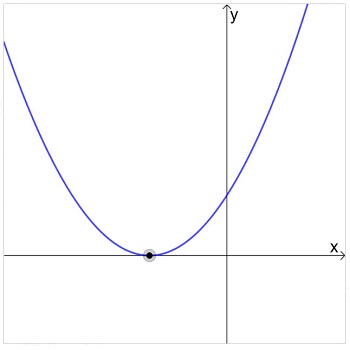
- Use the discriminant \(\Delta =b^2-4ac\) to determine the nature of roots
The following videos will help you understand all the concepts from this page
Print from here
Just for Fun
If you understand the form y=a(x - p)(x - q) of a quadratic equation, then you should be able to play and win this game of angry birds. The angry bird starts at (0 , 0), the pig is at (10 , 0) and the bird must pass through (5,4).
Enter the equation in the space below and press play.
How much of Quadratics have you understood?











 Twitter
Twitter  Facebook
Facebook  LinkedIn
LinkedIn