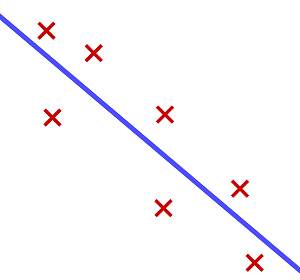
 This page is all about looking for correlations in bivariate data (to see if there is a relationship between x and y variables). You will learn how to calculate r, Pearson’s product-moment correlation coefficient and how to interpret it. We will look at y on x regression as well as x on y regression using them to consider interpolation and extrapolation.
This page is all about looking for correlations in bivariate data (to see if there is a relationship between x and y variables). You will learn how to calculate r, Pearson’s product-moment correlation coefficient and how to interpret it. We will look at y on x regression as well as x on y regression using them to consider interpolation and extrapolation.
On this page, you should learn about
- linear correlation of bi-variate data
- scatter diagrams
- y on x regression line
- x on y regression line
- interpolation and extrapolation
- Pearson' product-moment correlation coefficient, \(r\)
Print from here
Here is a quiz that gets you to estimate the r value for different graphs
START QUIZ!
Here is a quiz that tests your understanding of linear correlation
START QUIZ!


The world record times in seconds for the women’s 100m sprint from 1970 onwards are given below
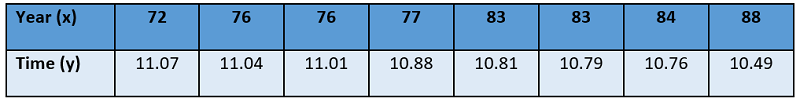
Use your calculator to write down
a) \(\bar{x}\) , the mean year
b) \(\bar{y}\) , the mean time
c) \(r\), Pearson’s product-moment correlation coefficient
The equation of the regression line y on x is y = ax + b
d) Find the values of a and b for these data
e) Show that \((\bar{x},\bar{y})\) lies on this line
f) Use the regression line to estimate the world record time in 2024
Hint
Full Solution
How much of Linear Correlation have you understood?





 Twitter
Twitter  Facebook
Facebook  LinkedIn
LinkedIn