File failed to load: https://library.tis.today/StudyIB/ajax/libs/mathjax/2.7.0/extensions/MathMenu.js

Sums and Products of Roots

  2. Functions
  3. Sums and Products of Roots
    4. 30'

On this page, we learn all about roots of polynomial equations and how to use the formulae for the sum of the roots and the product of the roots. For the polynomial equation, degree n

\(\sum _{ r=1 }^{ n }{ { a }_{ r } } { x }^{ r }\)  , the sum of the roots = \(\frac { { a }_{ n-1 } }{ { a }_{ n } } \) , the product of the roots =\((-1)^n\frac { { a }_{ 0 } }{ { a }_{ n } } \)

Key Concepts

On this page, you should learn about

  • roots of polynomial equations
  • sums and products of roots
 

Essentials

The following videos will help you understand all the concepts from this page

Where do the formulae come from

Below you will find the formula for finding the sum of the roots and the product of the roots of polynomial equations of different degrees, but what are they and where do these formulae come from? We will find out in the video below.

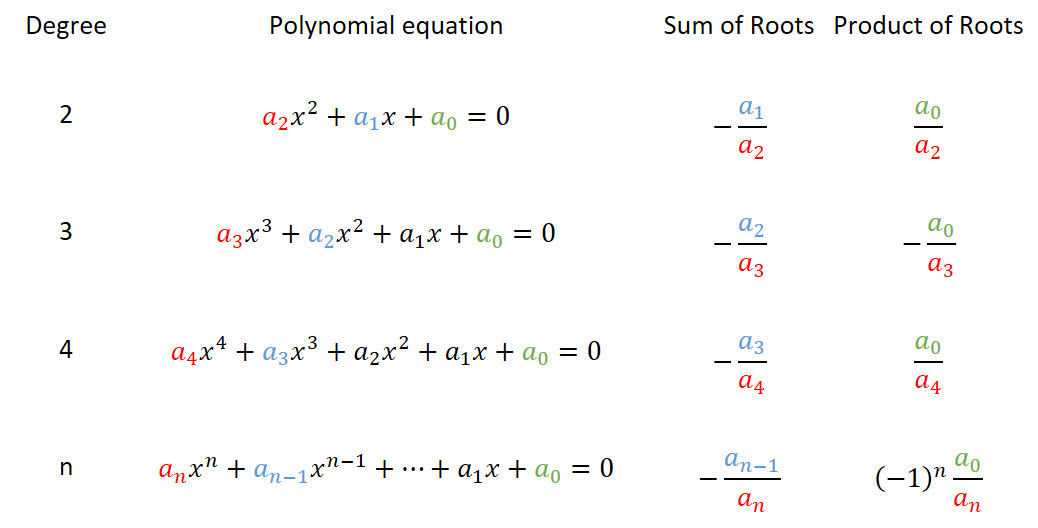
* These formulae do not appear in the formula booklet. You should learn the general case for degree n by heart.

Notes from the video

 

Finding New Polynomials

In the following video, we look at a typical exam-style question in which we are required to find a new polynomial having worked out the sum and product of roots of this equation:

The quadratic equation x² - 4x + 5 = 0 has roots \(\alpha\) and \(\beta\).

a. Without solving the equation, find the value of

i \(\alpha + \beta\)

ii \(\alpha \beta\)

b. Another quadratic equation 5x² + bx + c = 0 , b,c\(\in \mathbb{Z}\) has roots \(\frac{1}{\alpha}\) and \(\frac{1}{\beta}\).

Find the value of b and the value of c.

Notes from the video

 

Problem Solving

The following video is a typical exam-style question. One of the things that IB likes to do in examinations, is to ask a question that requires knowledge from different areas of the course. This can be quite challenging and often something that textbooks do not give you practice in. This question is about sums and products of roots of polynomial equations, but requires some interesting problem-solving skils, since the roots of the equation are terms of an arithmetic sequence. Here is the question:

Consider the equation \(64x^{ 3 }−144x^{ 2 }+92x−15=0\)

  1. Write down the numerical value of the sum and the product of the roots of this equation.
  2. The roots of this equation are three consecutive terms of an arithmetic sequence. Solve the equation.

Notes from the video

 

Complex Roots

In the following video we will look at an example involving complex roots of a polynomial equation. Since the coefficients of the polynomial are real numbers, complex roots must always come in pairs and more than that they must be conjugate pairs - this way two complex numbers can multiply to give a real number.

The conjugate root theorem states that if the complex number \(a+ib\) is a root of a polynomial f(x) in one variable with real coefficients, then the complex conjugate \(a-ib\) also a root of that polynomial.

One root of the equation \(4z^4-4z^3-25z^2+55z-42=0\) is \(1+\frac {\sqrt{3}}{2}i\)

Find the other roots of the equation.

Notes from the video

 
 

Summary

Print from here

text

Test Yourself

Here is a quiz that practises the sums and products of real roots of polynomial


START QUIZ!

text

Exam-style Questions

Question 1

The quadratic equation \(3x^{ 2 }−8x+2=0\) has roots \(\alpha\) and \(\beta\).

a. Without solving the equation, find the value of \(\alpha + \beta\) and \(\alpha \beta\).

b. Another quadratic equation \(3x^{ 2 }+bx+c=0\quad ,\quad b,c\in \mathbb{Z}\) has roots \(\frac{\alpha}{\beta}\) and \(\frac{\beta}{\alpha}\). Find the value of b and c.

Hint

Full Solution

 

Question 2

In the quadratic equation \(px^{ 2 }−45x+25=0\quad ,\quad p\in \mathbb{Z}\) ,one root is two times the other.

Find the value of p.

Hint

Full Solution

 

Question 3

Let \(f(x)=2x^{ 4 }+x^{ 3 }−14x^{ 2 }+5x+6\quad ,\quad x\in \mathbb{R}\)

a. For the polynomial equation f(x) = 0 , find the value of

  1. the sum of the roots
  2. the product of the roots

b. A new polynomial is defined by g(x) = f(x - 2). Find the sum of the roots of the equation g(x) = 0

Hint

Full Solution

 

Question 4

Consider the equation \(8x^{ 3 }−42x^{ 2 }+px−27=0.\)

  1. State
    1. the sum of the roots of the equation
    2. the product of the roots of the equation
  2. The roots of this equation are three consecutive terms of a geometric sequence. Taking the roots to be \(\frac{\alpha}{\beta},\alpha,\alpha\beta\) show that one of the roots is \(\frac{3}{2}\)
  3. Solve the equation.
  4. Find the value of p.

Hint

Full Solution

 

Question 5

One root of the equation z² + bz + c = 0 is 2+3i where \(b,c\in\mathbb{Z}\).

Find the value of b and the value of c.

Hint

Full Solution

 

Question 6

The equation \(2z^{ 4 }−9z^{ 3 }+pz^{ 2 }+qz−174=0 \quad,\quad p,q\in\mathbb{Z}\) has two real roots \(\alpha\) and \(\beta\) and two complex roots \(\gamma\) and \(\delta\) where \(\gamma=2-5i\).

a. Show that \(\alpha+\beta=\frac{1}{2}.\)

b. Find \(\alpha\beta\).

c. Hence find the two real roots α and β.

d. Find the values of p and q.

Hint

Full Solution

 
 
MY PROGRESS

How much of Sums and Products of Roots have you understood?