


On this page, you should learn about
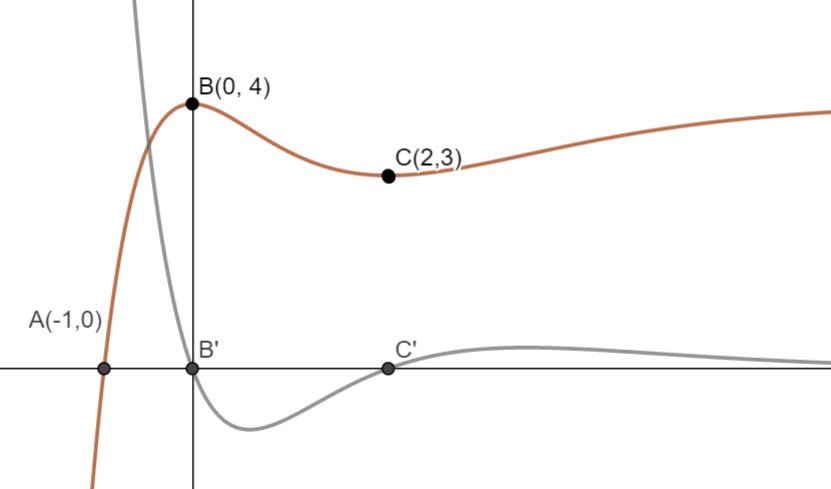
- graphs of \(f,f'\ \mathrm{and} \ f''\)
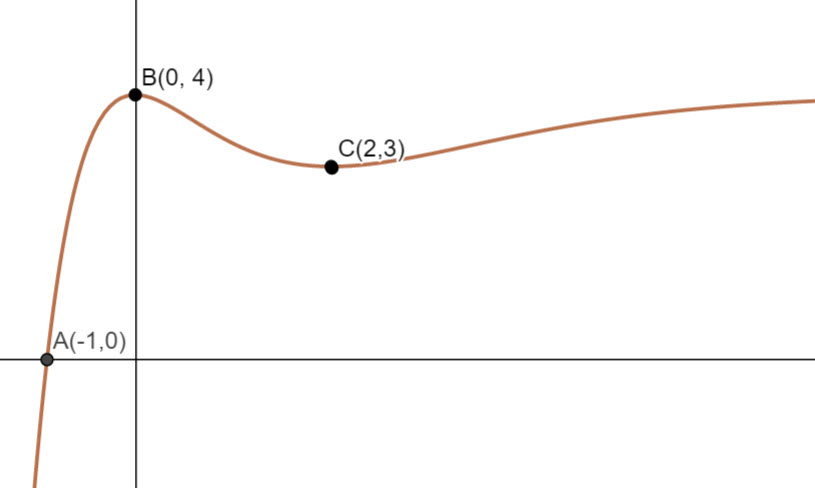
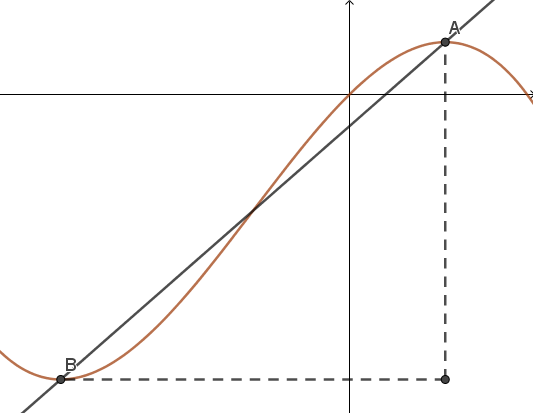
- local maximum and minimum points
- stationary points of inflexion
- non-stationary points of inflexion
The following videos will help you understand all the concepts from this page
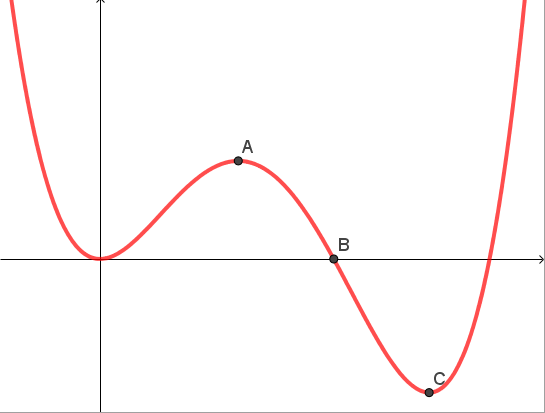
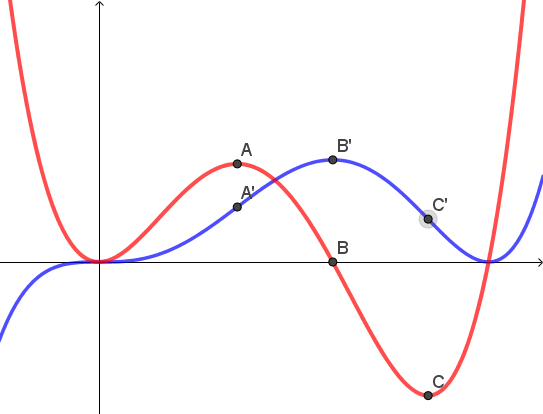
In the following video we shall see how to sketch graphs of a gradient function
You can get some practice of sketching gradient functions by trying the activity below. This works best if you have a device with a tactile screen so that you can sketch the graphs on screen. If this is not the case, you can print a copy from here and draw with a pencil.
- Choose the pen tool and sketch the gradient function
- Check your answer by clicking in the check box
- Turn off the answer and delete your sketch before moving on to the next question
Print from here
How much of Graphs and Derivatives have you understood?













 Twitter
Twitter  Facebook
Facebook  LinkedIn
LinkedIn