9.1.3 Calculating Energy Changes in SHM

Calculating Energy Changes in SHM

There are many equations to learn in the topic of simple harmonic motion
The key equations are summarised below:

9-1-3-equations-for-shm-1-ib-hl

Pd0TczGl_9-1-3-equations-for-shm-2

9-1-3-equations-for-shm-3

A summary of the equations related to simple harmonic motion. The green stars indicate equations which are not included in the IB data booklet

Worked Example

The graph shows the potential energy, E_P, for a particle oscillating with SHM. The particle has mass 45 g.

9-1-3-worked-example-1

(a)

Use the graph to determine the amplitude and hence, the period of oscillation.

(b)

Calculate the maximum speed which the particle achieves.

Part (a)

Step 1: List the known quantities

- - Mass of the particle, m = 45 g = 45 × 10⁻³ kg

Step 2: Use the graph to determine the maximum potential energy of the particle

9-1-3-worked-example-1-solution

- - Maximum potential energy, E_Pmax = 60 mJ = 60 × 10⁻³ J

Step 3: Determine the amplitude of the oscillation

- - The amplitude of the motion, x₀, is the maximum displacement
  - At the maximum displacement, the particle is at its highest point, hence this is the position of maximum potential energy
  - From the graph, when E_P = E_Pmax:

x₀² = 8.0 cm = 0.08 m

- - Therefore, the amplitude is:

x₀ = √0.08 = 0.28 m

Step 4: Write down the equation for the potential energy of an oscillator

$E_{P} = \frac{1}{2} m ω^{2} {x_{0}}^{2}$

Step 5: Rearrange the equation for angular velocity, ω:

$ω^{2} = \frac{2 E_{P}}{m {x_{0}}^{2}}$

$ω = \sqrt{\frac{2 E_{P}}{m {x_{0}}^{2}}}$

Step 6: Substitute the known values and calculate ω

$ω = \sqrt{\frac{2 \times (60 \times 10^{- 3})}{(45 \times 10^{- 3}) \times 0.08}}$

ω = 5.77 rad s⁻¹

Step 7: Write down the relation between period, T, and angular velocity, ω

$T = \frac{2 π}{ω}$

Step 8: Determine the time period of the oscillation

$T = \frac{2 π}{5.77}$ = 1.1 s

Part (b)

Step 1: List the known quantities

- - Angular velocity, ω = 5.77 rad s⁻¹
  - Amplitude, x₀ = 0.28 m

Step 2: Write down the equation for the maximum speed of an oscillator

v_max = ωx₀

Step 3: Substitute in the known quantities

v_max = 5.77 × 0.28 = 1.6 m s⁻¹

Worked Example

A student investigated the behaviour of a 200 g mass oscillating on a spring, and produced the graph shown.

9-1-3-worked-example-2

(a)

Determine the values for amplitude and time period

(b)

Hence find the maximum kinetic energy of the oscillating mass.

Part (a)

Step 1: Read the values of amplitude and time period from the graph

Step 2: State the values of amplitude and time period

- - Amplitude, x₀ = 0.3 m
  - Time period, T = 2.0 s

Part (b)

Step 1: List the known quantities

- - Mass of the oscillator, m = 200 g = 0.2 kg

Step 2: Write down the equation for the maximum speed of an oscillator

v_max = ωx₀

Step 3: Write down the equation relating angular speed and time period

$ω = \frac{2 π}{T}$

Step 4: Combine the two equations and calculate the maximum speed

$v_{m a x} = \frac{2 π x_{0}}{T} = \frac{2 π \times 0.3}{2.0}$

v_max = 0.942 m s⁻¹

Step 5: Use the maximum speed to calculate the maximum kinetic energy of the oscillating mass

E_{K m a x} = \frac{1}{2} m {v_{m a x}}^{2}

E_Kmax = 0.5 × 0.2 × 0.942² = 0.1 J

Worked Example

A ball of mass 23 g is held between two fixed points A and B by two stretch helical springs, as shown in the diagram below.

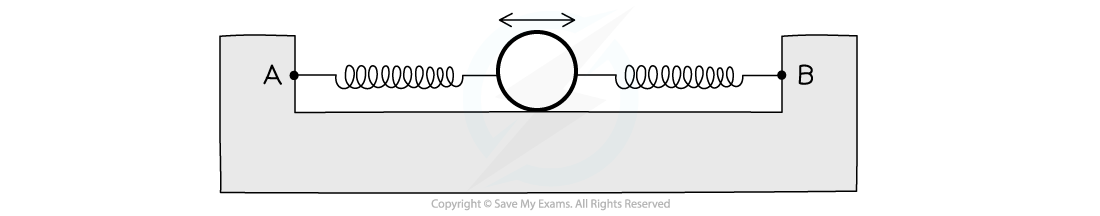Worked example horizontal mass on spring, downloadable AS & A Level Physics revision notes The ball oscillates along the line AB with simple harmonic motion of frequency 4.8 Hz and amplitude 1.5 cm.

Calculate the total energy of the oscillations.

Step 1: Write down all known quantities

- Mass, m = 23 g = 23 × 10^–3 kg
- Amplitude, x₀ = 1.5 cm = 0.015 m
- Frequency, f = 4.8 Hz

Step 2: Write down the equation for the total energy of SHM oscillations:

$E = \frac{1}{2} m ω^{2} {x_{0}}^{2}$

Step 3: Write an expression for the angular frequency

$ω = 2 π f$

Step 4: Substitute values into the SHM energy equation

$E = \frac{1}{2} m {(2 π f)}^{2} {x_{0}}^{2}$

E = 0.5 × (23 × 10⁻³) × (2π × 4.8)² × (0.015)²

E = 2.354 × 10⁻³ = 2.4 mJ (2 s.f.)

Exam Tip

There are a large number of equations associated with SHM. Most of them are given in the data booklet which you will be given to use in the exam

Make sure you are familiar with the equations, as you will probably need to use several different ones to solve the longer questions.

DP IB Physics: HL

Revision Notes

9.1.3 Calculating Energy Changes in SHM

Calculating Energy Changes in SHM

Worked Example

Worked Example

Worked Example

Exam Tip

Author: Lindsay

Resources

Members

Company

Quick Links