The Uncertainty Principle
- The quantisation of energy levels and their associated wave functions leads to some surprising outcomes
- One of these outcomes is known as the uncertainty principle
- The uncertainty principle states that:
- This leads to two consequences related to:
- Position and momentum
- Energy and time
Position & Momentum
- One example is that it is not possible to know the position and the momentum of a quantum particle precisely
- This can be represented in equation form as:
- Where:
- Δx = change in displacement
- Δpx = change in momentum
- h = Planck's constant
- This equation shows that:
- The better the position of the particle is known, the less precise is the knowledge of its momentum
- The value of Planck’s constant is small so this limitation only becomes important for small particles within the quantum regime - it does not apply in the Newtonian mechanics that you have used to calculate momentum for larger objects
Energy & Time
- There is a similar relationship between energy and time where both cannot be known precisely
- Where:
- ΔE = change in energy
- Δt = change in time
- h = Planck's constant
Wavefunctions
- This principle can be demonstrated by looking at the wavefunctions associated with quantum particles that are nearly 'free'
- For example, those associated with the energy levels in a 'swimming pool' potential energy well
- The graph of wavefunction vs position shows that while the momentum is known precisely the position of the particle is unknown
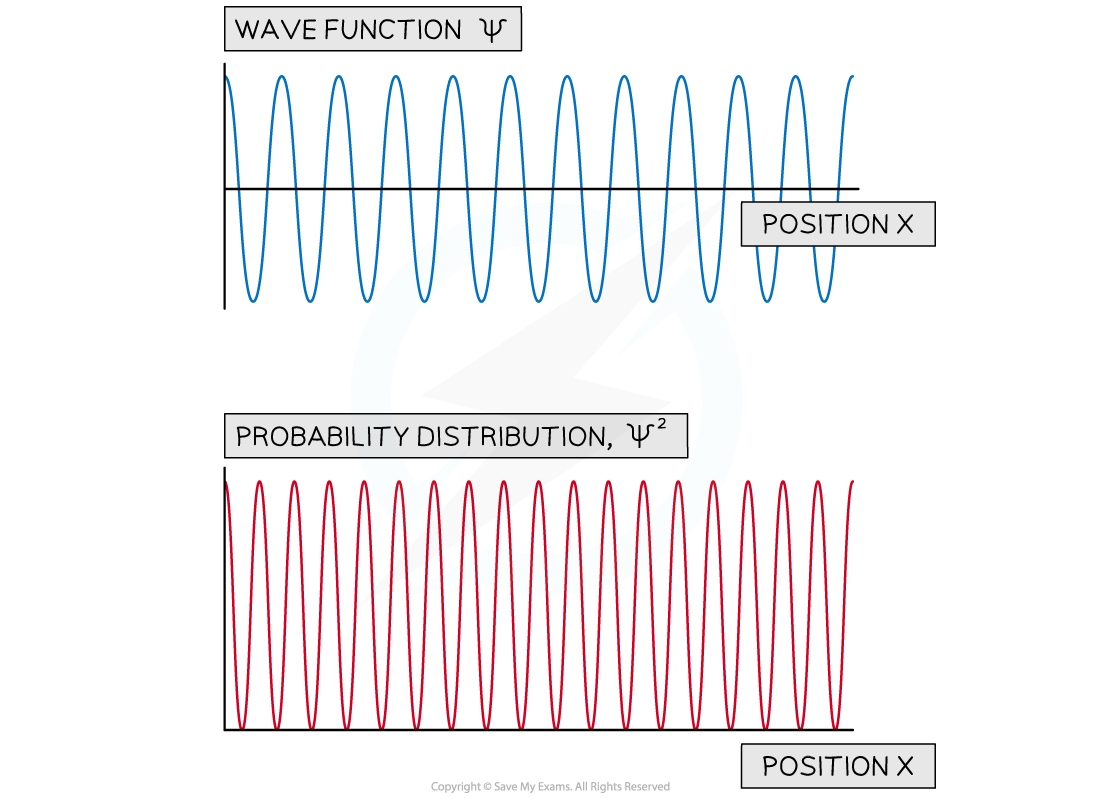
The wave function of a free particle with a fixed energy. The wave function shows it has a single value of momentum while the probability distributions shows that the particle can be anywhere in space.
- For a particle in a 'swimming pool' potential well, its energy levels are close together
- Hence, the wave function can be a mixture of the wavefunctions associated with a number of closely spaced energy levels
- For a range of five neighbouring energy levels, the wavefunction can be obtained by adding the individual wavefunctions
- This is a result of the principle of superposition
- This gives rise to a wave packet, meaning the particle is more likely to be in a particular region of space
- This can be shown by a graph of the probability distribution vs position
- This helps to visualise how the approximate uncertainty in the position, Δx and momentum Δpx can be determined
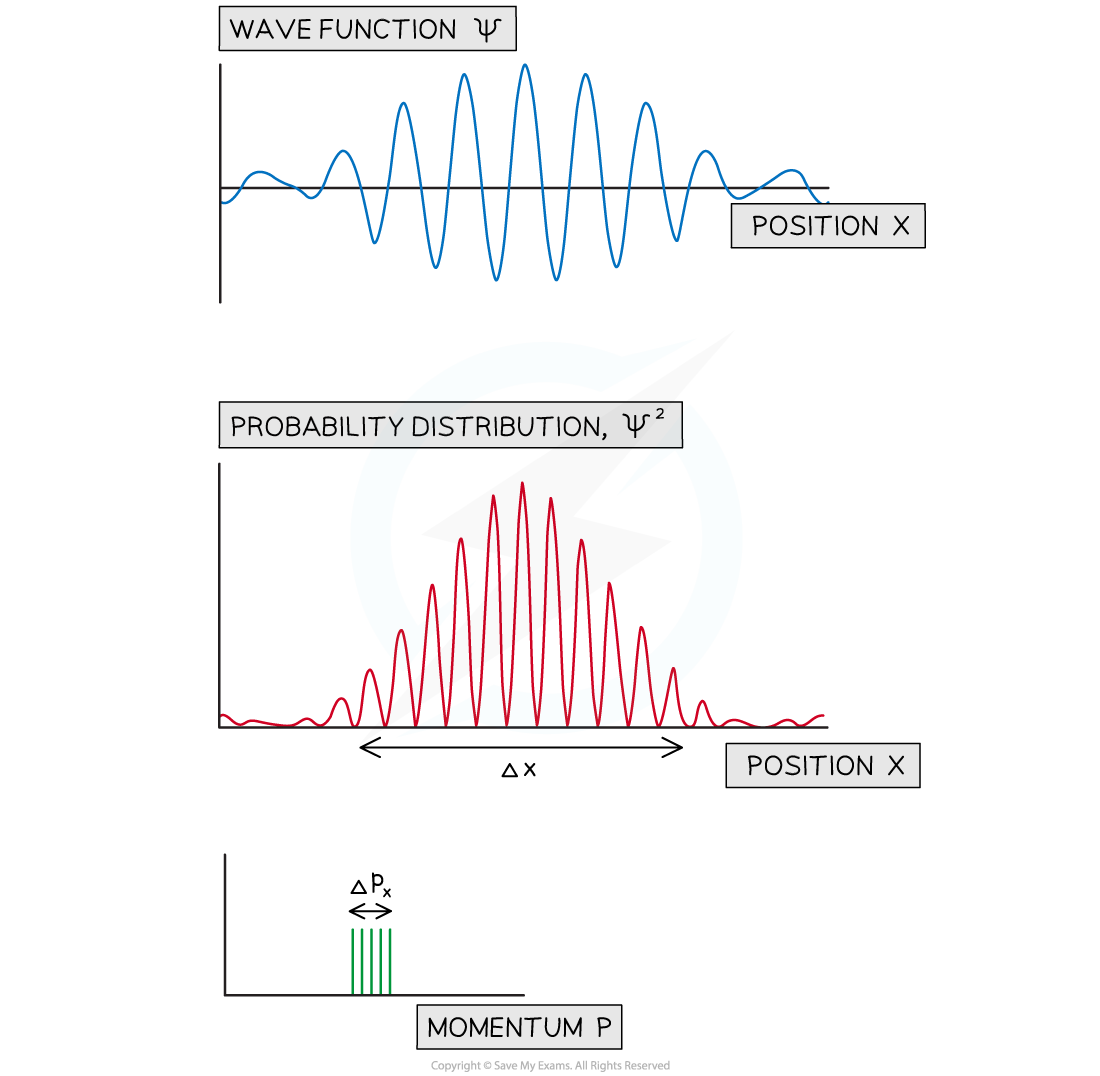
A wave packet made from the wavefunctions associated with five closely spaced energy levels. The probability distribution is shown along with the uncertainty in position and momentum.
- Consider the wavefunctions associated with five and ten energy levels with the probability distribution and the estimates of Δx and Δpx
- Comparing the two sets of graphs, it can be seen that:
- As the momentum becomes less well known the position becomes more well known
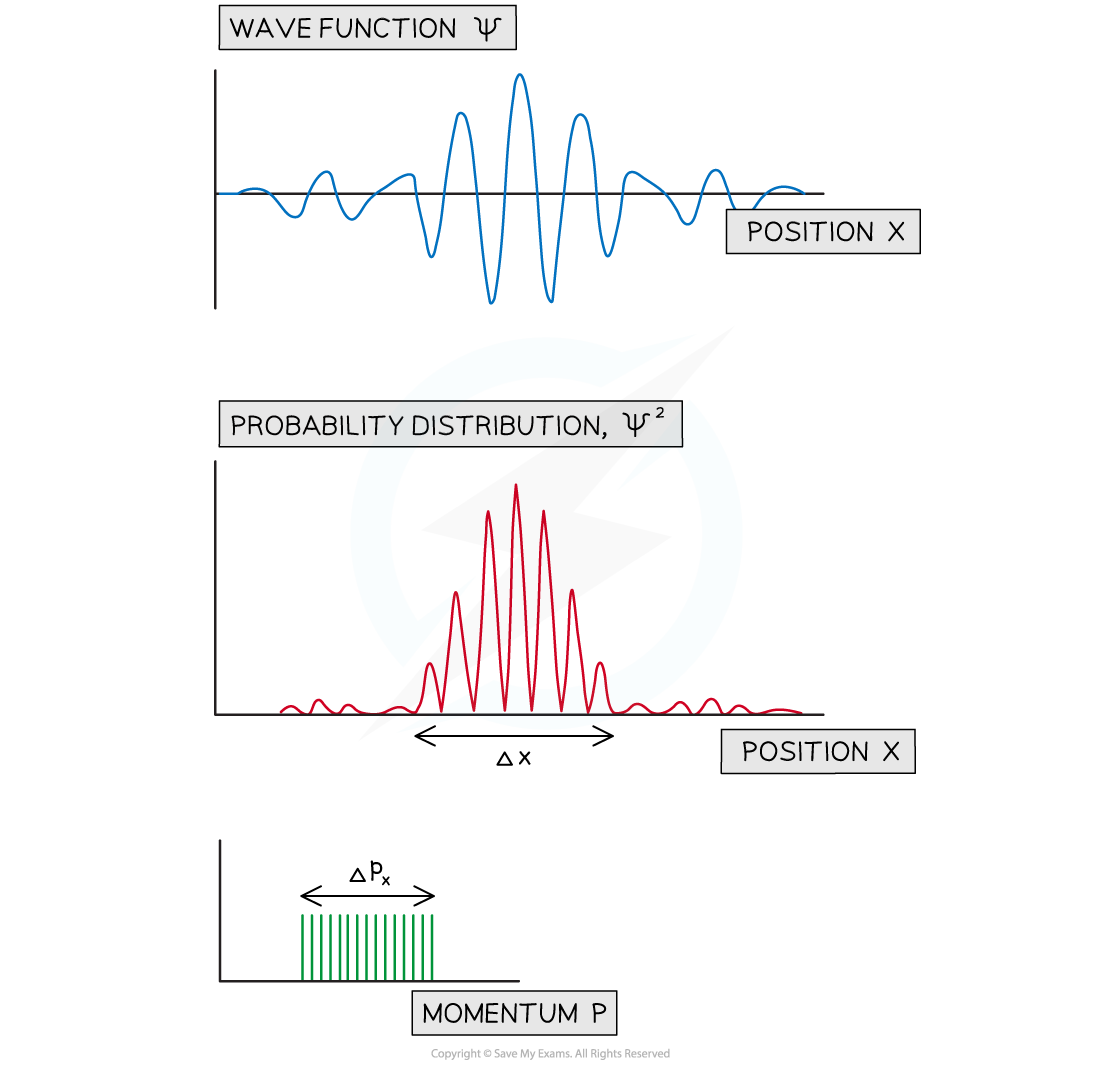
A wave packet made from the wavefunctions associated with five closely spaced energy levels. The probability distribution is shown along with the uncertainty in position and momentum.
Worked Example
A narrow beam of electrons with velocity 106 m s−1 are directed towards a slit which is 10-9 m wide.
The electrons are observed on a screen placed 2.0 m from the slit.
Estimate the length of the area of the screen where electrons will be seen in appreciable numbers.
Step 1: Write down the uncertainty equation for position and momentum
Step 2: Identify the known uncertainty from the question
-
- The slit is 10−9 m wide, so the uncertainty in the vertical component of the position of the electrons at the slit, Δx = 10−9
Step 3: Rearrange the equation to find Δp and calculate
Step 4: Calculate the momentum, p
-
- Mass of electron, m = 9.1 × 10−31 kg
-
- Momentum, p = mv = 9.1 × 10−31 × 106 = 9.1 × 10−25 N s
Step 5: Substitute change in momentum (step 3) and momentum (step 4) into the equation for change in direction
Step 6: Apply the deviation of angle to find the length on the screen
-
- Distance from slits to screen, D = 2.0 m
- Angle of deviation from line of beam, θ = 0.06 rad
-
- Therefore:
length on screen = 2θ × D = (2 × 0.06) × 2.0 = 0.24 m
Step 7: Write the final answer out in full
-
- The length of the screen where appreciable numbers of electrons are expected to be observed = 24 cm
