12.1.10 Tunnelling

• Single potential wells lead to quantised energy levels and their associated wavefunctions
  • The wavefunction extends throughout space

• However, for infinitely deep square wells the wavefunctions are localised within the well region
  • The probability of finding the quantum particle at the barrier is zero

• For a finite barrier, the wavefunction can penetrate the barrier
  • So, the particle has some probability of being in a “classically forbidden region”

• If there are two well-like regions, the solution of Schrodinger’s equation gives an energy level and wavefunction that extends over the whole region of the potential well

• When the red wave function is squared it gives the probability of finding the particle in a particular region of space
• Since the wave function extends through the barrier this means there is a finite probability of finding the particle in either of the two well regions

A thin barrier or classically forbidden region can result in tunnelling

• Consequently, if a quantum particle were placed in the narrow well on the right, it is possible at some time later to find it in the region on the left
  • The particle is said to have tunnelled through the narrow barrier

Tunnelling & Alpha Decay

• The strong nuclear force within the nucleus is represented by the square well
  • While the -dependence of the Coulombic repulsion dominates outside the well

• Nucleons in the nucleus have quantised energy levels and wave functions
  • An alpha particle can gain energy and occupy an excited energy level where the barrier width is smaller

• As a result, the alpha particle can tunnel through the classically forbidden region
  • This greatly increases the probability of the alpha particle being emitted

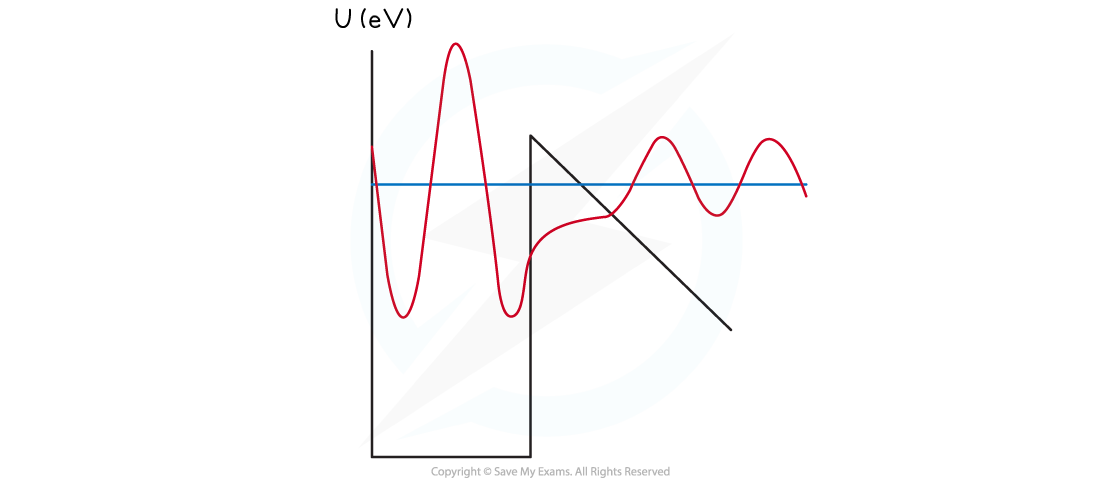
Alpha decay through quantum mechanical tunnelling

Uses of Quantum Tunnelling

• Quantum tunnelling is utilised in several systems, for example in:
  • Semiconductor devices
  • Fusion reactions in the Sun
  • A scanning tunnelling microscope

• In one mode of operation of a scanning tunnelling microscope, a sharp point, one atom thick, is maintained close to a surface
  • This is so that a small tunnelling current between the tip and the surface remains constant
  • In this case, the gap between the tip and the sample surface acts as the barrier that the electrons must tunnel through

• The tip is moved up and down and across the surface by piezoelectric transducers allowing the sample surface to be mapped out

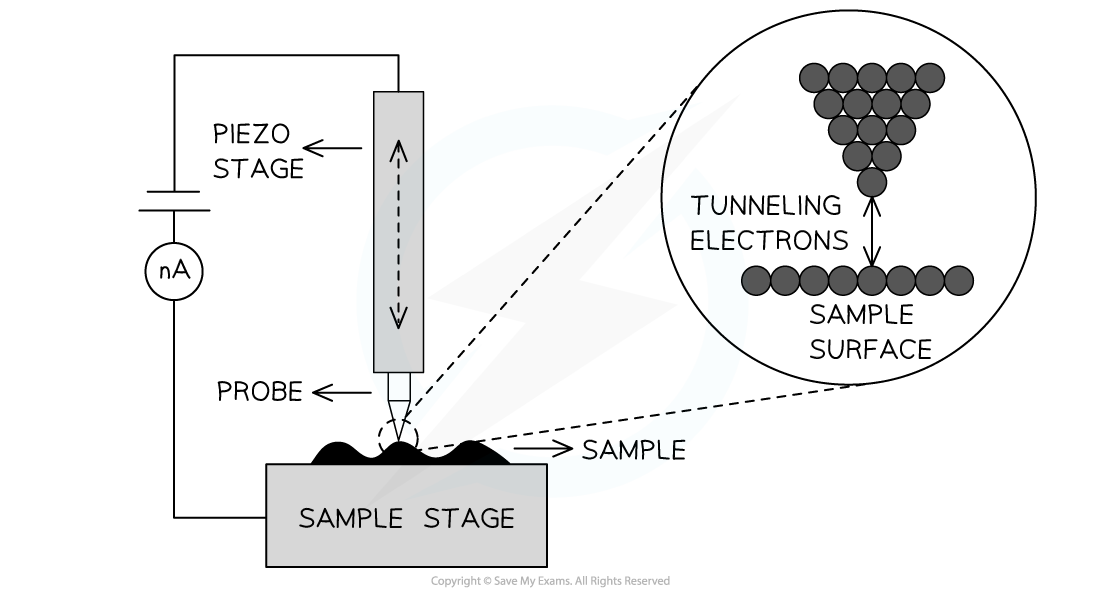Simplified schematic of a scanning tunnelling microscope