1.3.2 Combining & Resolving Vectors

• Vectors are represented by an arrow
  • The arrowhead indicates the direction of the vector
  • The length of the arrow represents the magnitude

Combining Vectors

• Vectors can be combined by adding or subtracting them to produce the resultant vector
  • The resultant vector is sometimes known as the ‘net’ vector (eg. the net force)

• There are two methods that can be used to combine vectors: the triangle method and the parallelogram method

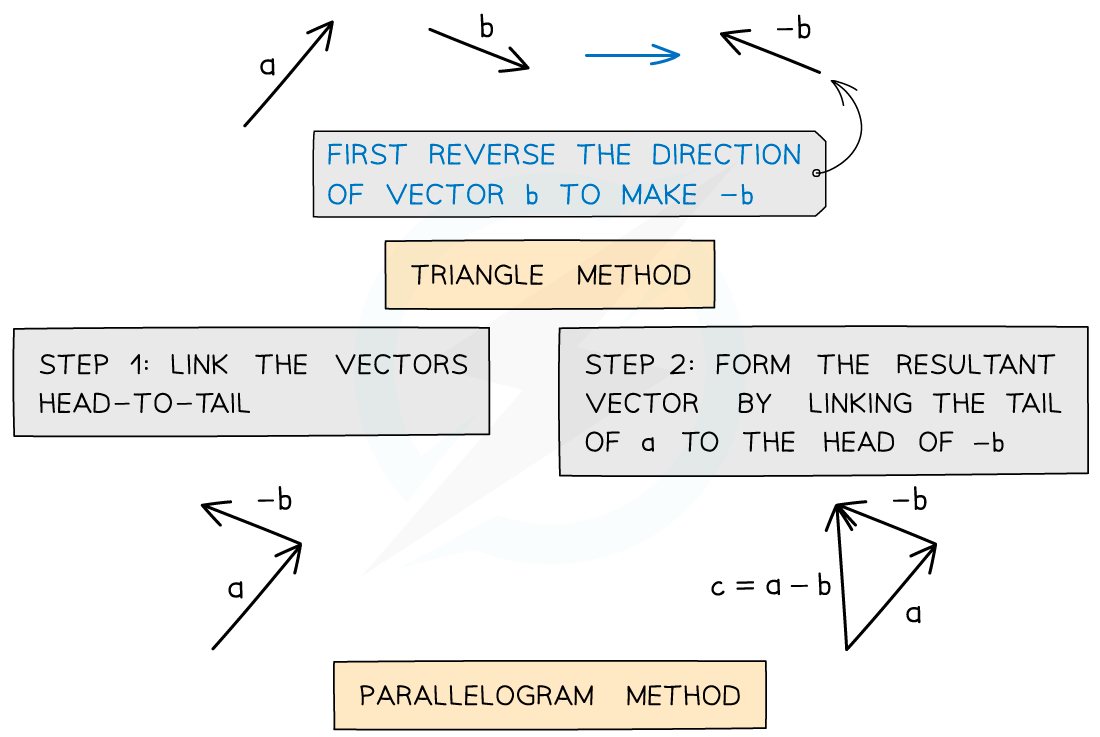
• To combine vectors using the triangle method:
  • Step 1: link the vectors head-to-tail
  • Step 2: the resultant vector is formed by connecting the tail of the first vector to the head of the second vector

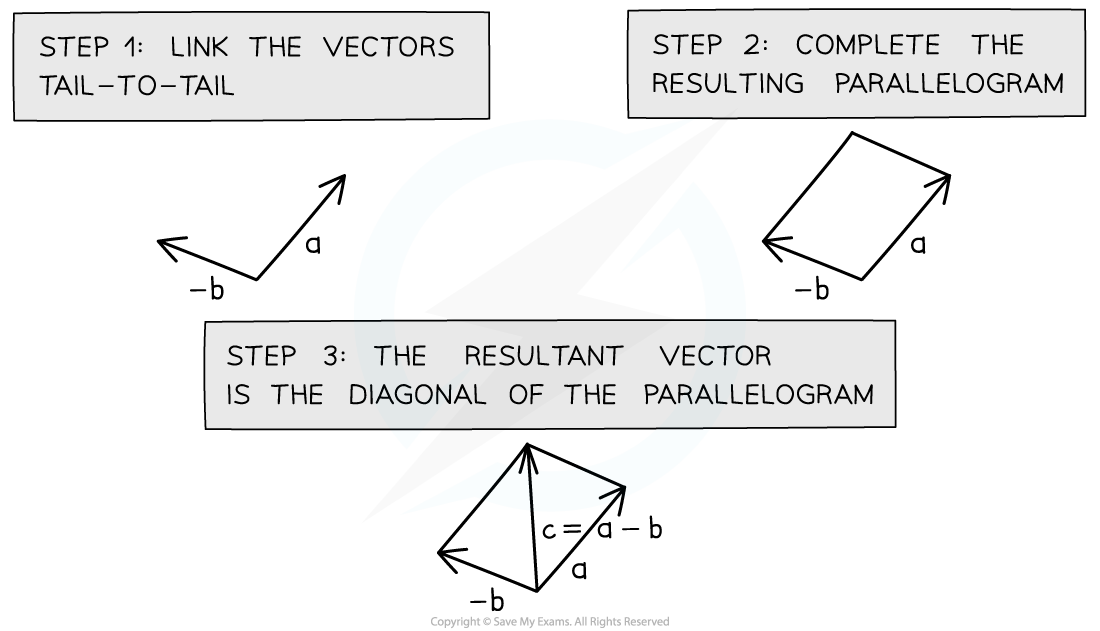
• To combine vectors using the parallelogram method:
  • Step 1: link the vectors tail-to-tail
  • Step 2: complete the resulting parallelogram
  • Step 3: the resultant vector is the diagonal of the parallelogram

Resolving Vectors

• Two vectors can be represented by a single resultant vector
  • Resolving a vector is the opposite of adding vectors

• A single resultant vector can be resolved
  • This means it can be represented by two vectors, which in combination have the same effect as the original one

• When a single resultant vector is broken down into its parts, those parts are called components
• For example, a force vector of magnitude F and an angle of θ to the horizontal is shown below

The resultant force F at an angle θ to the horizontal

• It is possible to resolve this vector into its horizontal and vertical components using trigonometry

The resultant force F can be split into its horizontal and vertical components

• For the horizontal component, F_x = F cos θ
• For the vertical component, F_y = F sin θ

Step 4: State the final answer complete with direction

R = 2√34 = 11.66 = 12 km

θ = 59° east and upwards from the horizontal