Gravitational Field Strength
- There is a universal force of attraction between all matter with mass
- This force is known as the ‘force due to gravity’ or the weight
- The Earth’s gravitational field is responsible for the weight of all objects on Earth
- A gravitational field is defined as:
A region of space where a test mass experiences a force due to the gravitational attraction of another mass
- The direction of the gravitational field is always towards the centre of the mass causing the field
- Gravitational forces cannot be repulsive
- Gravity has an infinite range, meaning it affects all objects in the universe
- There is a greater gravitational force around objects with a large mass (such as planets)
- There is a smaller gravitational force around objects with a small mass (almost negligible for atoms)
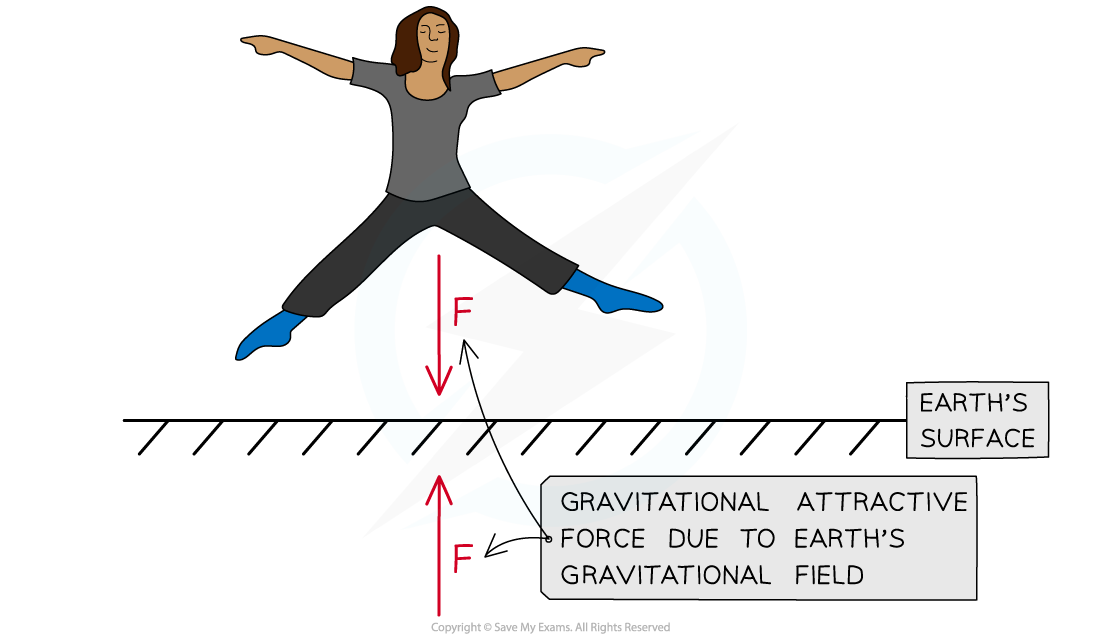
The Earth's gravitational field produces an attractive force. The force of gravity is always attractive
- The gravitational field strength at a point is defined as:
The force per unit mass experienced by a test mass at that point
- This can be written in equation form as:
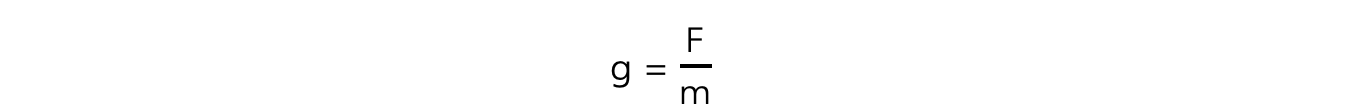
- Where:
- g = gravitational field strength (N kg−1)
- F = force due to gravity, or weight (N)
- m = mass of test mass in the field (kg)
- This equation shows that:
- On planets with a large value of g, the gravitational force per unit mass is greater than on planets with a smaller value of g
- An object's mass remains the same at all points in space
- However, on planets such as Jupiter, the weight of an object will be greater than on a less massive planet, such as Earth
- This means the gravitational force would be so high that humans, for example, would not be unable to fully stand up
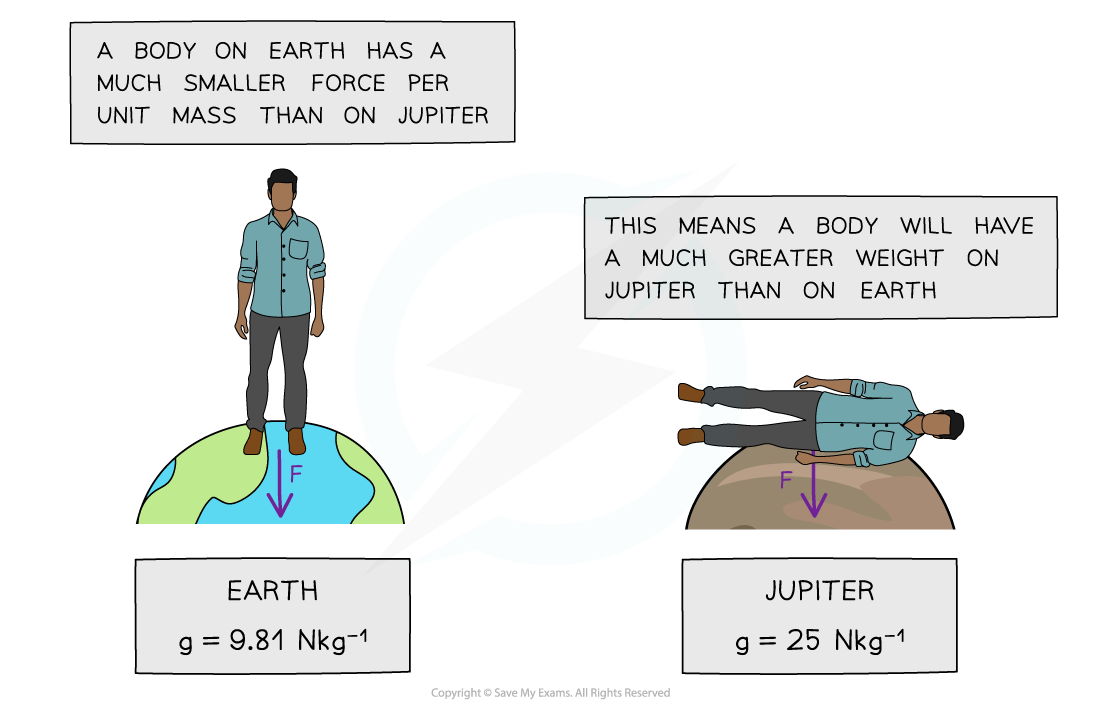
A person’s weight on Jupiter would be so large that a human would be unable to fully stand up
- Factors that affect the gravitational field strength at the surface of a planet are:
- The radius r (or diameter) of the planet
- The mass M (or density) of the planet
- This can be shown by equating the equation F = mg with Newton's law of gravitation:
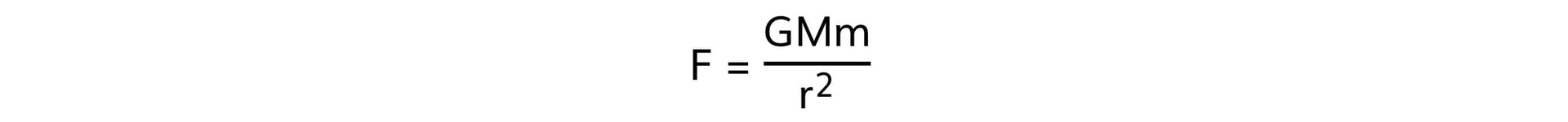
- Substituting the force F with the gravitational force mg leads to:

- Cancelling the mass of the test mass, m, leads to the equation:
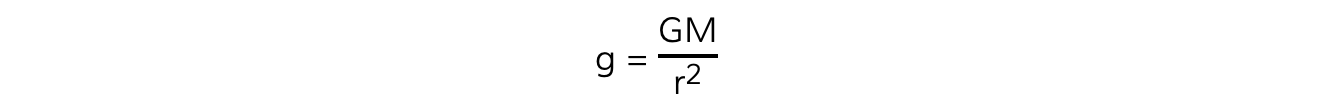
- Where:
- G = Newton's Gravitational Constant
- M = mass of the body causing the field (kg)
- r = distance from the mass where you are calculating the field strength (m)
- This equation shows that:
- The gravitational field strength g depends only on the mass of the body M causing the field
- Hence, objects with any mass m in that field will experience the same gravitational field strength
- The gravitational field strength g is inversely proportional to the square of the radial distance, r2
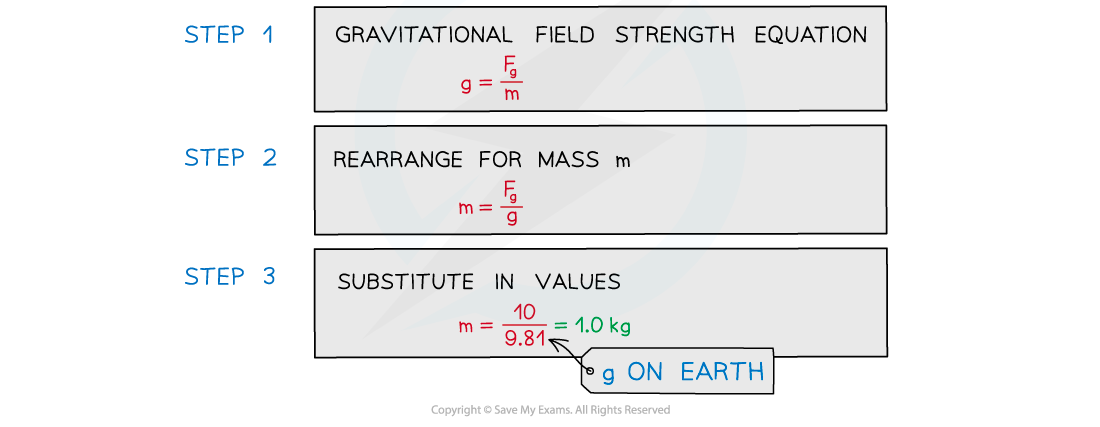
Worked Example
Calculate the mass of an object with weight 10 N on Earth.
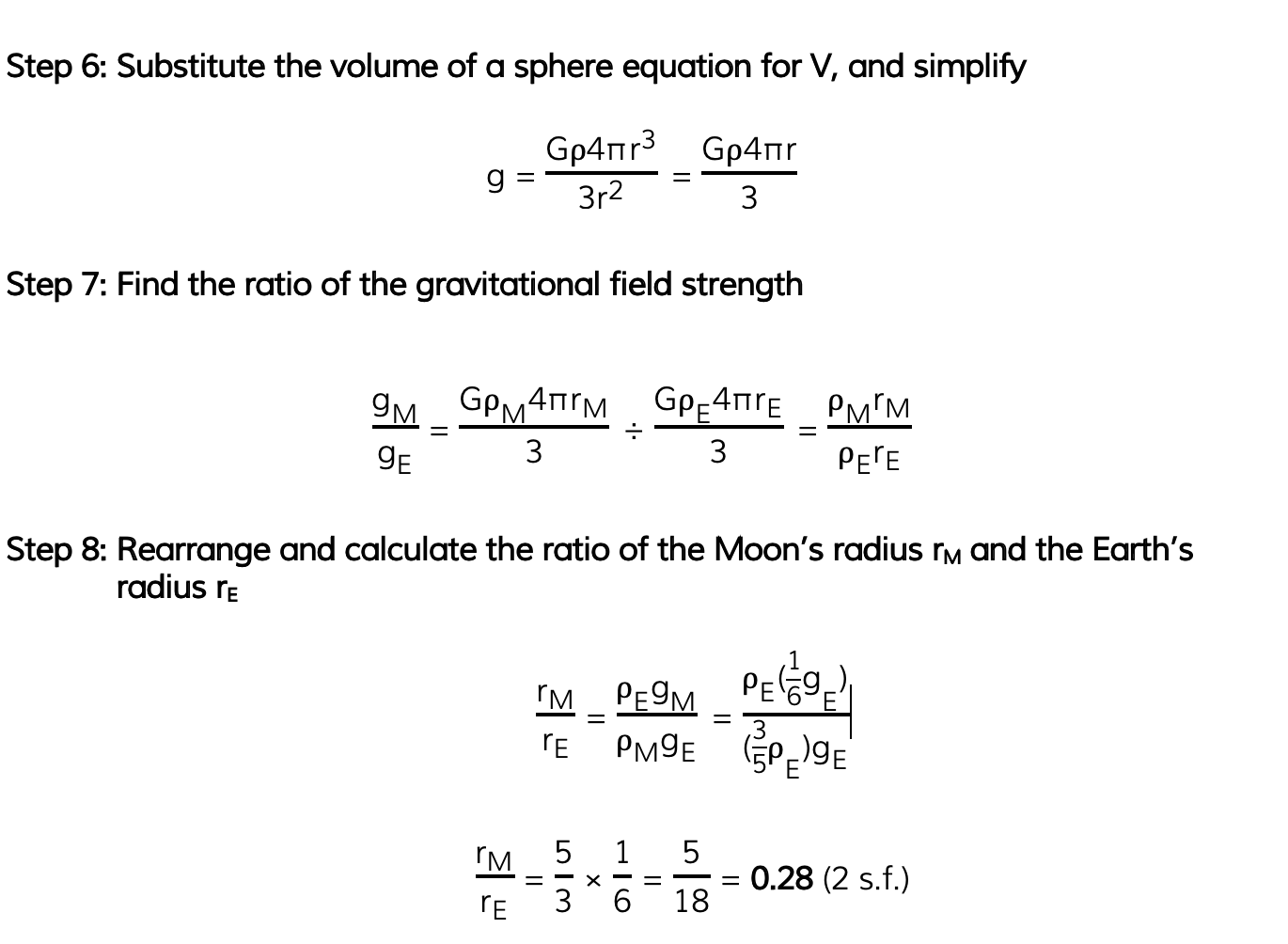
Worked Example
The mean density of the Moon is 3/5 times the mean density of the Earth. The gravitational field strength on the Moon is 1/6 of the value on Earth.
Determine the ratio of the Moon's radius rM and the Earth's radius rE.

Exam Tip
There is a big difference between g and G (sometimes referred to as ‘little g’ and ‘big G’ respectively), g is the gravitational field strength and G is Newton’s gravitational constant. Make sure not to use these interchangeably! Remember the equation density ρ = mass m ÷ volume V, which may come in handy with some calculations
Resultant Gravitational Field Strength
- In a similar way to other vectors, such as force or velocity, the gravitational field strength due to two bodies can be determined
- This is because gravitational field strength is a vector, meaning it has both a magnitude and direction
- The resultant gravitational field strength is, therefore, the vector sum of the gravitational field strength due to each body
Worked Example
A planet is equidistant from two stars in a binary system. Each star has a mass of 5.0 × 1030 kg and the planet is at a distance of 3.0 × 1012 m from each star. Calculate the magnitude of the resultant gravitational field strength at the position of the planet.

Step 1: List the known quantities
- Mass of one star, M = 5.0 × 1030 kg
- Distance between one star and the planet, r = 3.0 × 1012 m
- Angle between the gravitational field strength and the planet, θ = 42°
Step 2: Write out the equation for gravitational field strength
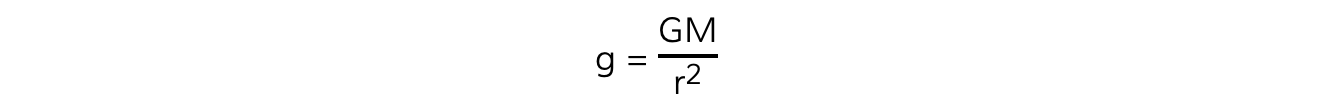
Step 3: Calculate the gravitational field strength due to one star
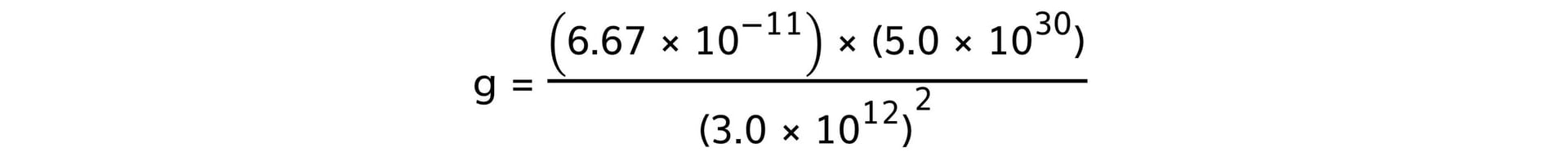
g = 3.7 × 10−5 N kg−1
Step 4: Resolve the vectors vertically
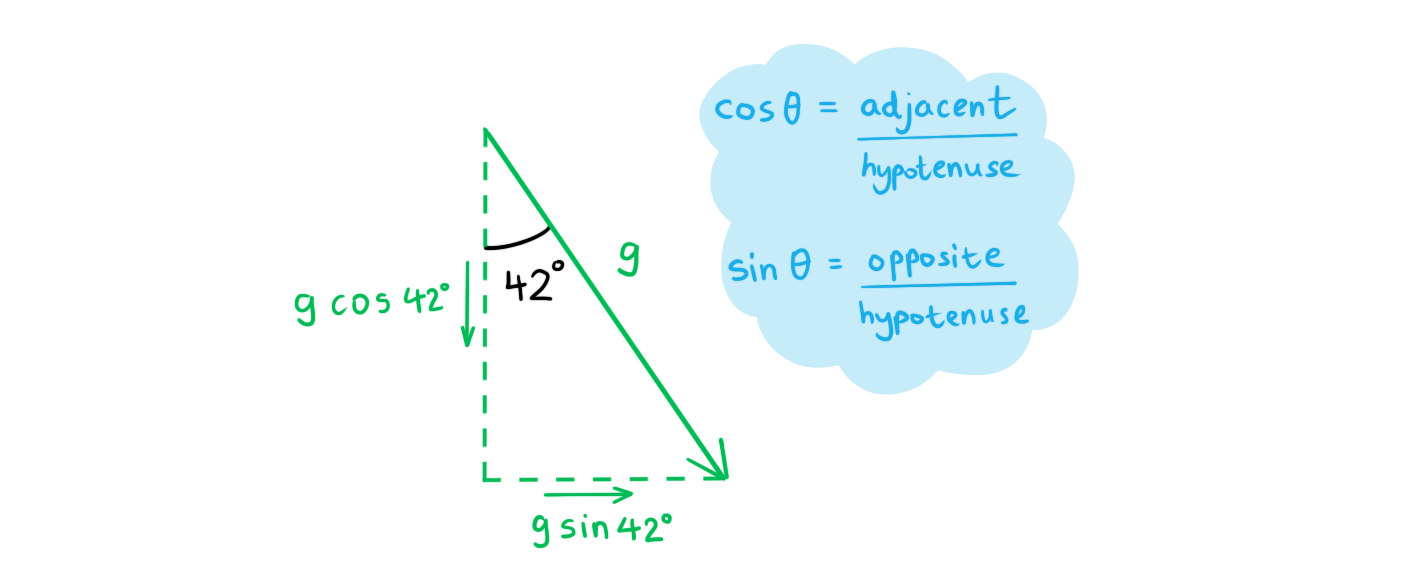
- The vertical component of each vector is g cos 42°
Step 5: Use vector addition to determine the resultant gravitational field strength
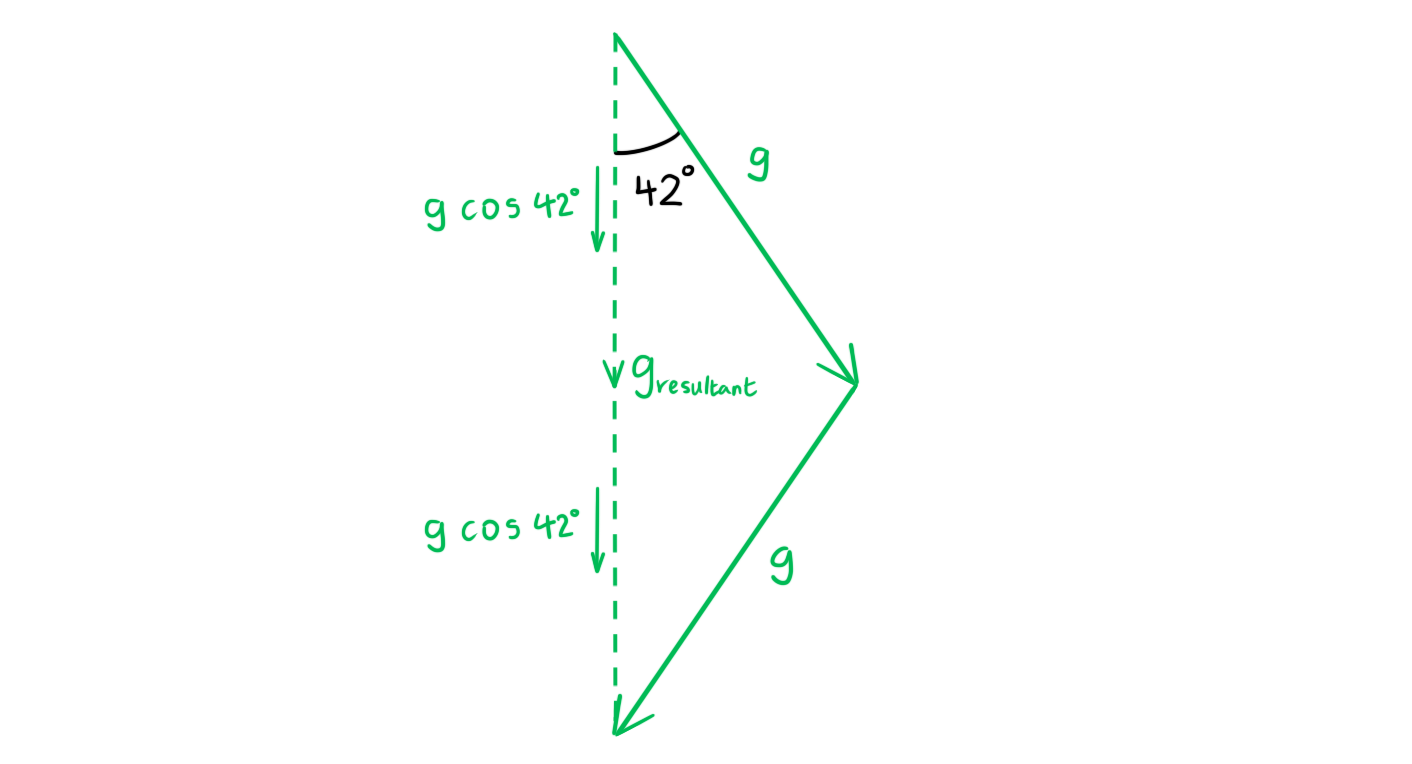
gresultant = g cos 42° + g cos 42° = 2g cos 42°
gresultant = 2 × (3.7 × 10−5) × cos 42°
gresultant = 5.5 × 10−5 N kg−1
Exam Tip
Don't worry, for calculation questions involving resultant gravitational field strength - only two bodies along a straight line will be tested!
