2.2.1 Free-Body Diagrams

• In physics, during force interactions, it is common to represent situations as simply as possible without losing information
  • When considering force interactions objects can be represented as point particles
  • These point particles should be placed at the center of mass of the object

• Force vectors that act upon that objects should be drawn with their tail on that point particle
  • The length of the force vector corresponds to its strength
  • The longer the vector, the greater the force magnitude

• The below example shows the forces acting on an object when pushed to the right over a rough surface

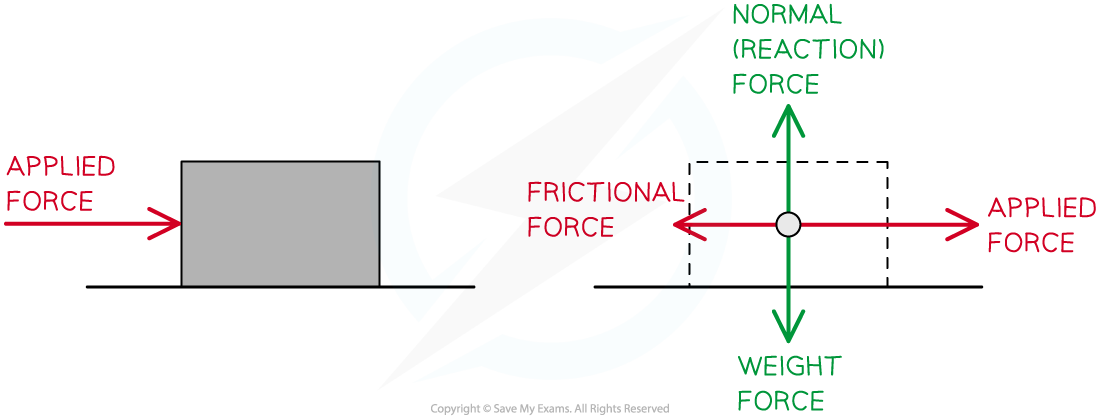
Point particle representation of the forces acting on a moving object

• The below example shows an object sitting on a slope in equilibrium

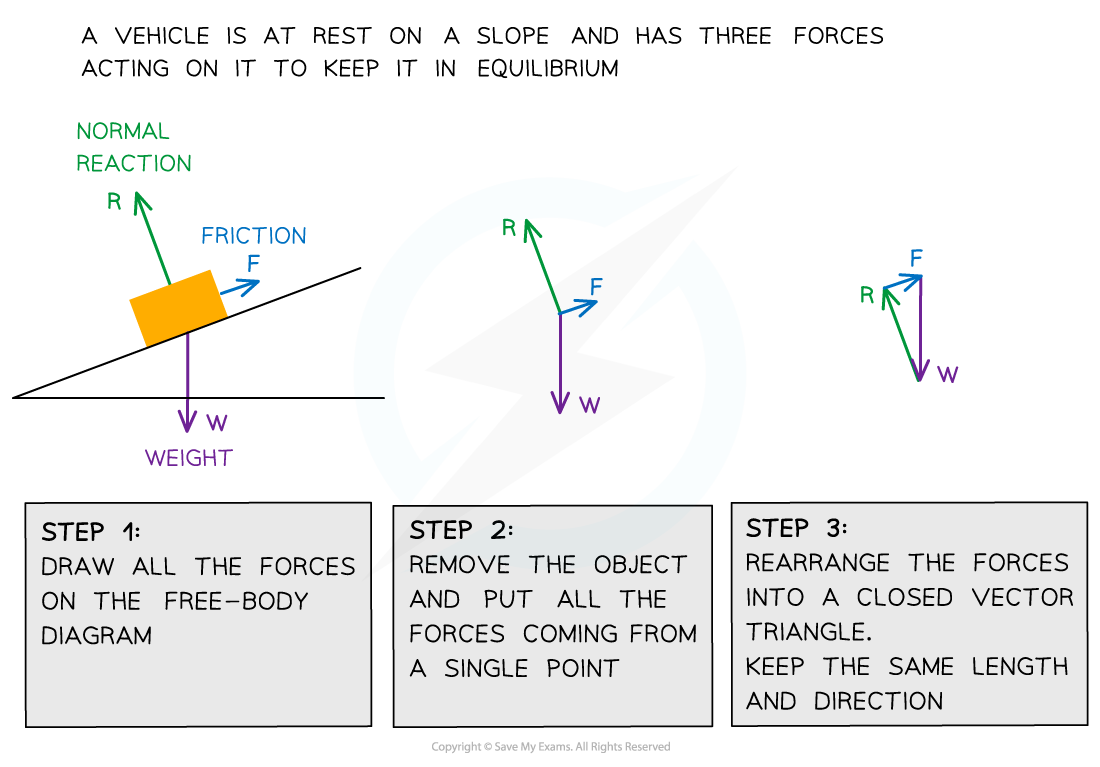
Three forces on an object in equilibrium form a closed vector triangle

• The below example shows the forces acting on an object suspended from a stationary rope

Free-body diagram of an object suspended from a stationary rope

• Free body diagrams are useful for modeling the forces that are acting on an object
• Each force is represented as a vector arrow, where each arrow:
  • Is scaled to the magnitude of the force it represents
  • Points in the direction that the force acts
  • Is labelled with the name of the force it represents or an appropriate symbol

• Free body diagrams can be used:
  • To identify which forces act in which plane
  • To resolve the net force in a particular direction

• The rules for drawing a free-body diagram are the following:
  • Rule 1: Draw a point in the centre of mass of the body
  • Rule 2: Draw the body free from contact with any other object
  • Rule 3: Draw the forces acting on that body using vectors with correct direction and proportional length

Free body diagrams can be used to show the various forces acting on objects

• You must be able to apply the following forces with their symbols to free-body diagrams:
  • Weight (W)
  • Tension (T)
  • Normal Reaction Force (N)
  • Upthrust (U)
  • Frictional Forces (F_r)

Part (a)

A picture frame hanging from a nail:

• • • The size of the arrows should be such that the 3 forces would make a closed triangle as they are balanced

Part (b)

A box sliding down a slope:

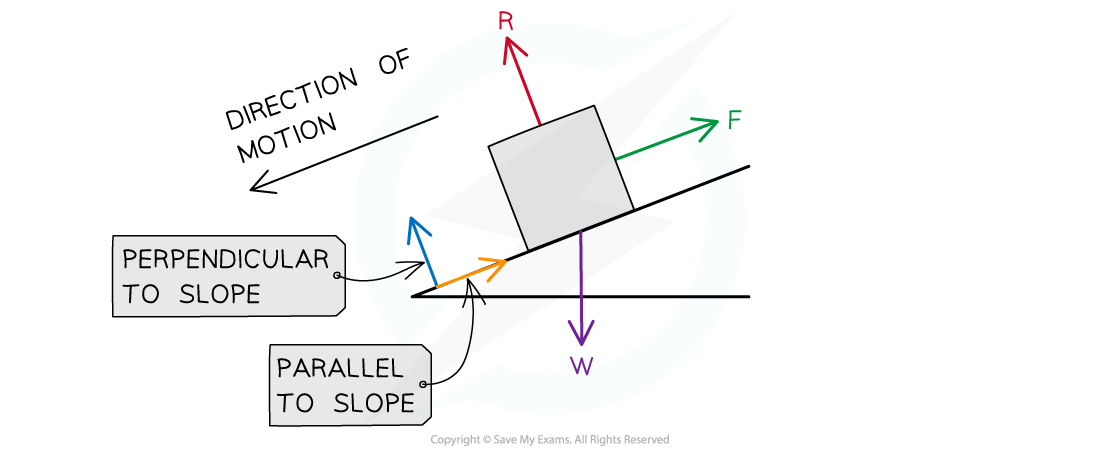
• • There are three forces acting on the box:
    • The normal contact force, R, acts perpendicular to the slope
    • Friction, F, acts parallel to the slope and in the opposite direction to the direction of motion
    • Weight, W, acts down towards the Earth

Step 1: Draw the object in a simplified diagram

Step 2: Identify all of the forces acting upon the object in the question, including any forces that may be implied

• • Weight: 30 N down
  • Upthrust from the water (since the object is floating): 30 N up
  • Applied force: 35 N to the right
  • Resistive force: 5 N to the left

Step 3: Draw in all of the force vectors (arrows), making sure the arrows start at the object and are directed away

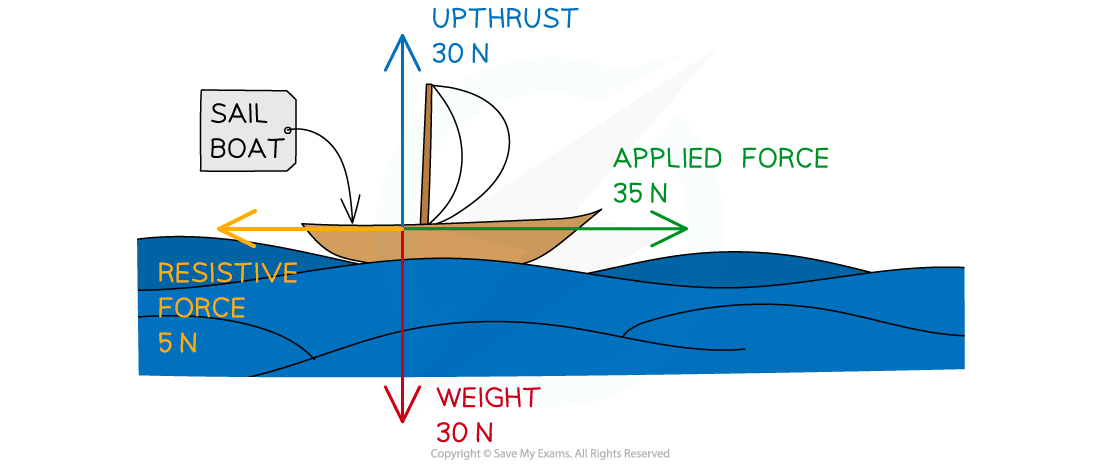
• • An approximation can be made as to the final resultant force due to all of the forces
    • Decide whether the resultant force is approximately up or down
    • Decide whether the resultant force is approximately left or right
    • For example, the resultant force is directed up and to the right