4.9.2 Confidence Interval for the Mean

What is a confidence interval?

• It is impossible to find the exact value of the population mean when taking a sample
• The mean of a sample is called a point estimate
• The best we can do is find an interval for which the exact value is likely to lie
• This is called the confidence interval for the mean
• The confidence level of a confidence interval is the probability that the interval contains the population mean
• Be careful with the wording – the population mean is a fixed value so it does not make sense to talk about the probability that it lies within an interval
• Instead we talk about the probability of an interval containing the mean
• Suppose samples were collected and a 95% confidence interval for the population mean was constructed for each sample then for every 100 intervals we would expect on average 95 of them to contain the mean
• 95 out of 100 is not guaranteed – it is possible that all of them could contain the mean
• It is also possible (though very unlikely) that none of them contains the mean

How do I find a confidence interval for the population mean (μ)?

• You will be given data using a sample from a population
• The population will be normally distributed
• If not then the sample size should be large enough so you can use the Central Limit Theorem
• You will use the interval functions on your calculator
• Use a z-interval if the population variance is known σ²
• On your GDC enter:
• the standard deviation σ and the confidence level α%
• EITHER the raw data
• OR the sample mean  and the sample size n

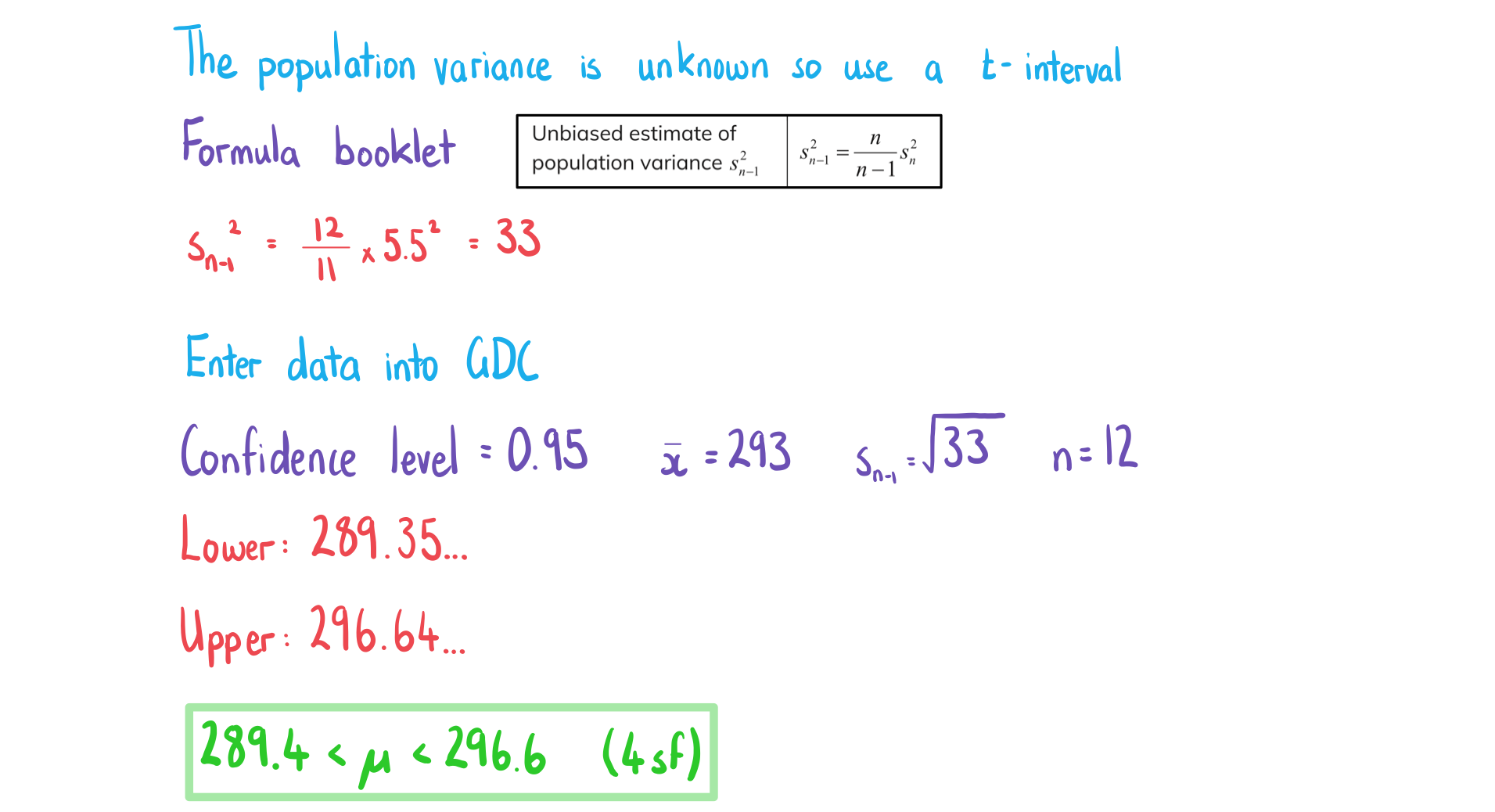
• Use a t-interval if the population variance is unknown
  • In this case the test uses the unbiased estimate for the variance 
  • On your GDC enter:

• the confidence level α%
• EITHER the raw data
• OR the sample mean , the value of s_n-1 and the sample size n
• Your GDC will give you the lower and upper bounds of the interval
• It can be written as a < μ < b

What affects the width of a confidence interval?

• The width of a confidence interval is the range of the values in the interval
• The confidence level affects the width
• Increasing the confidence level will increase the width
• Decreasing the confidence level with decrease the width
• The size of the sample affects the width
• Increasing the sample size will decrease the width
• Decreasing the sample size will increase the width

How can I interpret a confidence interval?

• After you have found a confidence interval for μ you might be expected to comment on the claim for a value of μ
• If the claimed value is within the confidence interval then there is not enough evidence to reject the claim
• Therefore the claim is supported
• If the claimed value is outside the interval then there is sufficient evidence to reject the claim
• The value is unlikely to be correct