2.1.1 Equations of a Straight Line

Equations of a Straight Line

How do I find the gradient of a straight line?

Find two points that the line passes through with coordinates (x₁, y₁) and (x₂, y₂)
The gradient between these two points is calculated by

$m = \frac{y_{2} - y_{1}}{x_{2} - x_{1}}$

- This is given in the formula booklet
The gradient of a straight line measures its slope
- A line with gradient 1 will go up 1 unit for every unit it goes to the right
- A line with gradient -2 will go down two units for every unit it goes to the right

What are the equations of a straight line?

$y = m x + c$
- This is the gradient-intercept form
- It clearly shows the gradient m and the y-intercept (0, c)
$y - y_{1} = m (x - x_{1})$
- This is the point-gradient form
- It clearly shows the gradient m and a point on the line (x₁, y₁)
$a x + b y + d = 0$
- This is the general form
- You can quickly get the x-intercept $(- \frac{d}{a}, 0)$ and y-intercept $(0, - \frac{d}{b})$

How do I find an equation of a straight line?

You will need the gradient
- If you are given two points then first find the gradient
It is easiest to start with the point-gradient form
- then rearrange into whatever form is required
  - multiplying both sides by any denominators will get rid of fractions
You can check your answer by using your GDC
- Graph your answer and check it goes through the point(s)
- If you have two points then you can enter these in the statistics mode and find the regression line $y = a x + b$

Exam Tip

A sketch of the graph of the straight line(s) can be helpful, even if not demanded by the question
- Use your GDC to plot them
Ensure you state equations of straight lines in the format required
- Usually $y = m x + c$ or $a x + b y + d = 0$
- Check whether coefficients need to be integers (they usually are for $a x + b y + d = 0$ )

Worked Example

The line $l$ passes through the points $(- 2, 5)$ and $(6, - 7)$ .

Find the equation of $l$ , giving your answer in the form $a x + b y + d = 0$ where $a, b$ and $c$ are integers to be found.

2-1-1-ib-ai-sl-equation-of-a-line-we-solution

Parallel Lines

How are the equations of parallel lines connected?

Parallel lines are always equidistant meaning they never intersect
Parallel lines have the same gradient
- If the gradient of line l₁is m₁ and gradient of line l₂is m₂then...
  - $m_{1} = m_{2} \Rightarrow l_{1} & l_{2} are parallel$
  - $l_{1} & l_{2} are parallel \Rightarrow m_{1} = m_{2}$
To determine if two lines are parallel:
- Rearrange into the gradient-intercept form $y = m x + c$
- Compare the coefficients of $x$
- If they are equal then the lines are parallel

Parallel & Perpendicular Gradients Notes Diagram 1

Worked Example

The line $l$ passes through the point $(4, - 1)$ and is parallel to the line with equation $2 x - 5 y = 3$ .

Find the equation of $l$ , giving your answer in the form $y = m x + c$ .

2-1-1-ib-ai-sl-parallel-lines-we-solution

Perpendicular Lines

How are the equations of perpendicular lines connected?

Perpendicular lines intersect at right angles
The gradients of two perpendicular lines are negative reciprocals
- If the gradient of line l₁is m₁ and gradient of line l₂is m₂then...
  - $m_{1} \times m_{2} = - 1 \Rightarrow l_{1} & l_{2} are perpendicular$
  - $l_{1} & l_{2} are perpendicular \Rightarrow m_{1} \times m_{2} = - 1$
To determine if two lines are perpendicular:
- Rearrange into the gradient-intercept form $y = m x + c$
- Compare the coefficients of $x$
- If their product is -1 then they are perpendicular
Be careful with horizontal and vertical lines
- $x = p$ and $y = q$ are perpendicular where p and q are constants

Parallel & Perpendicular Gradients Notes Diagram 3

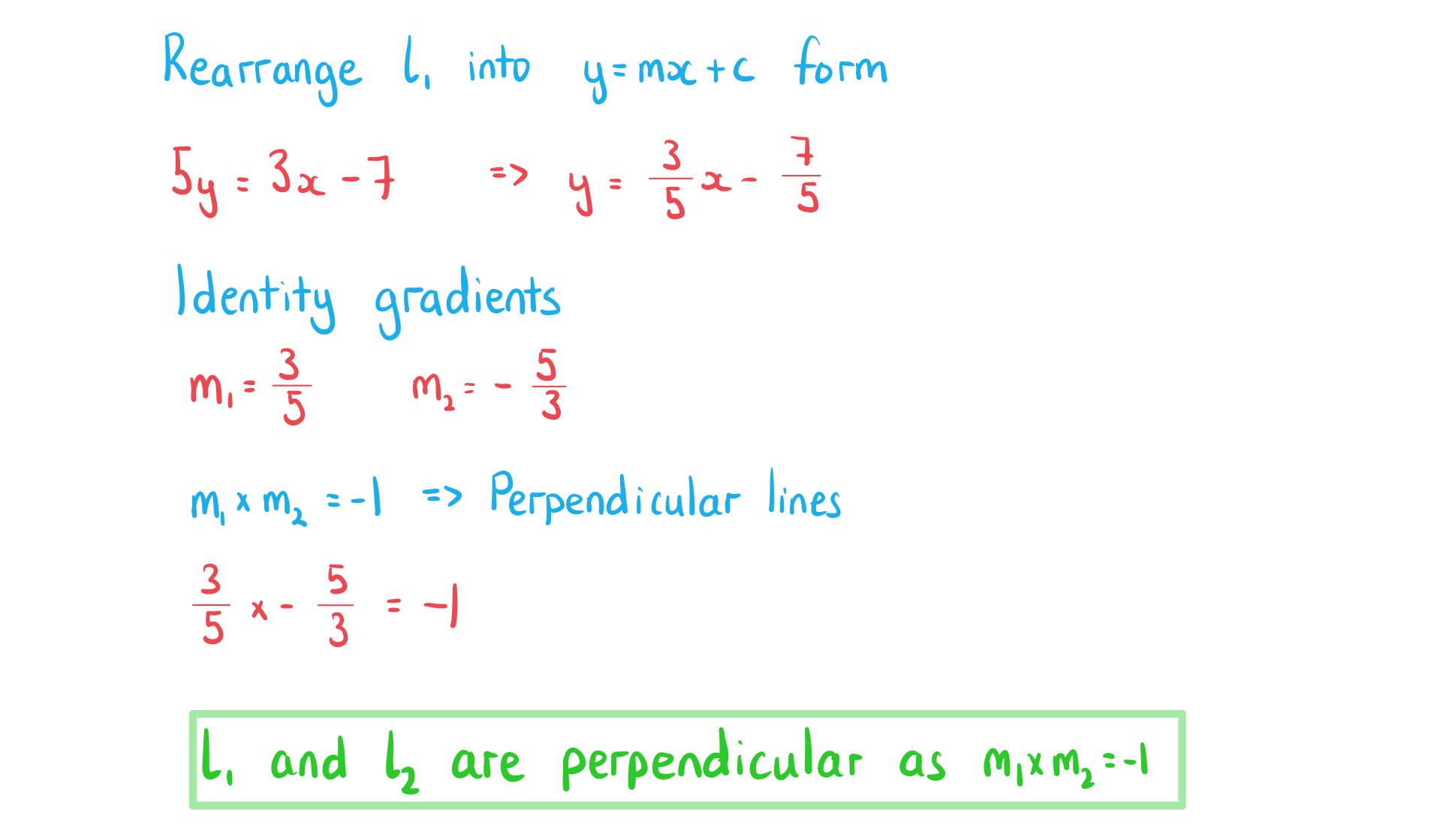
Worked Example

The line $l_{1}$ is given by the equation $3 x - 5 y = 7$ .

The line $l_{2}$ is given by the equation $y = \frac{1}{4} - \frac{5}{3} x$ .

Determine whether $l_{1}$ and $l_{2}$ are perpendicular. Give a reason for your answer.

2-1-1-ib-ai-sl-perpendicular-lines-we-solution

DP IB Maths: AI HL

Revision Notes

2.1.1 Equations of a Straight Line

Equations of a Straight Line

How do I find the gradient of a straight line?

What are the equations of a straight line?

How do I find an equation of a straight line?

Exam Tip

Worked Example

Parallel Lines

How are the equations of parallel lines connected?

Worked Example

Perpendicular Lines

How are the equations of perpendicular lines connected?

Worked Example

Author: Daniel

Resources

Members

Company

Quick Links