| Date | May 2017 | Marks available | 3 | Reference code | 17M.2.sl.TZ2.9 |
| Level | SL only | Paper | 2 | Time zone | TZ2 |
| Command term | Find | Question number | 9 | Adapted from | N/A |
Question
A ship is sailing north from a point A towards point D. Point C is 175 km north of A. Point D is 60 km north of C. There is an island at E. The bearing of E from A is 055°. The bearing of E from C is 134°. This is shown in the following diagram.
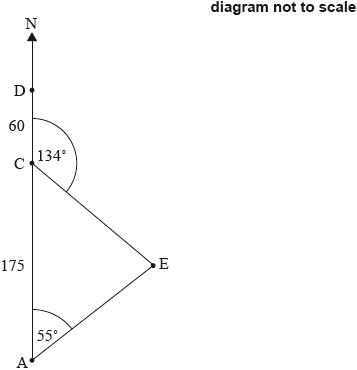
Find the bearing of A from E.
Finds CE.
Find DE.
When the ship reaches D, it changes direction and travels directly to the island at 50 km per hour. At the same time as the ship changes direction, a boat starts travelling to the island from a point B. This point B lies on (AC), between A and C, and is the closest point to the island. The ship and the boat arrive at the island at the same time. Find the speed of the boat.
Markscheme
valid method (M1)
eg180+55, 360−90−35
235° (accept S55W, W35S) A1 N2
[2 marks]
valid approach to find AˆEC (may be seen in (a)) (M1)
egAˆEC=180−55−AˆCE, 134=E+55
correct working to find AˆEC (may be seen in (a)) (A1)
eg180−55−46, 134−55, AˆEC=79∘
evidence of choosing sine rule (seen anywhere) (M1)
egasinA=bsinB
correct substitution into sine rule (A1)
egCEsin55∘=175sinAˆEC
146.034
CE=146 (km) A1 N2
[5 marks]
evidence of choosing cosine rule (M1)
egDE2=DC2+CE2−2×DC×CE×cosθ
correct substitution into right-hand side (A1)
eg602+146.0342−2×60×146.034cos134
192.612
DE=193 (km) A1 N2
[3 marks]
valid approach for locating B (M1)
egBE is perpendicular to ship’s path, angle B=90
correct working for BE (A1)
egsin46∘=BE146.034, BE=146.034sin46∘, 105.048
valid approach for expressing time (M1)
egt=ds, t=dr, t=192.61250
correct working equating time (A1)
eg146.034sin46∘r=192.61250, s105.048=50192.612
27.2694
27.3 (km per hour) A1 N3
[5 marks]

