The following diagram shows the chord [AB] in a circle of radius 8 cm, where \({\text{AB}} = 12{\text{ cm}}\).

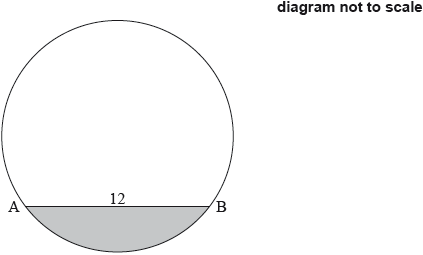
Find the area of the shaded segment.

attempt to find the central angle or half central angle     (M1)

eg\(\,\,\,\,\,\), cosine rule, right triangle

correct working     (A1)

eg\(\,\,\,\,\,\)\(\cos \theta  = \frac{{{8^2} + {8^2} - {{12}^2}}}{{2 \bullet 8 \bullet 8}},{\text{ }}{\sin ^{ - 1}}\left( {\frac{6}{8}} \right),{\text{ }}0.722734,{\text{ }}41.4096^\circ ,{\text{ }}\frac{\pi }{2} - {\sin ^{ - 1}}\left( {\frac{6}{8}} \right)\)

correct angle \({\rm{A\hat OB}}\) (seen anywhere) 

eg\(\,\,\,\,\,\)\({\text{1.69612, }}97.1807^\circ ,{\text{ 2}} \times {\text{si}}{{\text{n}}^{ - 1}}\left( {\frac{6}{8}} \right)\)     (A1)

correct sector area

eg\(\,\,\,\,\,\)\(\frac{1}{2}(8)(8)(1.70),{\text{ }}\frac{{97.1807}}{{360}}(64\pi ),{\text{ }}54.2759\)     (A1) 

area of triangle (seen anywhere)     (A1) 

eg\(\,\,\,\,\,\)\(\frac{1}{2}(8)(8)\sin 1.70,{\text{ }}\frac{1}{2}(8)(12)\sin 0.722,{\text{ }}\frac{1}{2} \times \sqrt {64 - 36}  \times 12,{\text{ }}31.7490\)

appropriate approach (seen anywhere)     (M1)

eg\(\,\,\,\,\,\)\({A_{{\text{triangle}}}} - {A_{{\text{sector}}}}\), their sector-their triangle

22.5269

area of shaded region \( = 22.5{\text{ }}({\text{c}}{{\text{m}}^2})\)     A1     N4

Note:     Award M0A0A0A0A1 then M1A0 (if appropriate) for correct triangle area without any attempt to find an angle in triangle OAB.

[7 marks]