Show that \({\text{AC}} = 7{\text{ cm}}\).

The shape in the following diagram is formed by adding a semicircle with diameter [AC] to the triangle.

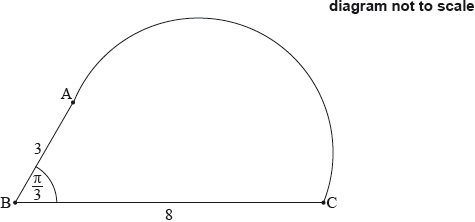
Find the exact perimeter of this shape.

evidence of choosing the cosine rule     (M1)

eg\(\,\,\,\,\,\)\({c^2} = {a^2} + {b^2} - ab\cos C\)

correct substitution into RHS of cosine rule     (A1)

eg\(\,\,\,\,\,\)\({3^2} + {8^2} - 2 \times 3 \times 8 \times \cos \frac{\pi }{3}\)

evidence of correct value for \(\cos \frac{\pi }{3}\) (may be seen anywhere, including in cosine rule)     A1

eg\(\,\,\,\,\,\)\(\cos \frac{\pi }{3} = \frac{1}{2},{\text{ A}}{{\text{C}}^2} = 9 + 64 - \left( {48 \times \frac{1}{2}} \right),{\text{ }}9 + 64 - 24\)

correct working clearly leading to answer     A1

eg\(\,\,\,\,\,\)\({\text{A}}{{\text{C}}^2} = 49,{\text{ }}b = \sqrt {49} \)

\({\text{AC}} = 7{\text{ (cm)}}\)     AG     N0

Note:     Award no marks if the only working seen is \({\text{A}}{{\text{C}}^2} = 49\) or \({\text{AC}} = \sqrt {49} \) (or similar).

[4 marks]

correct substitution for semicircle     (A1)

eg\(\,\,\,\,\,\)\({\text{semicircle}} = \frac{1}{2}(2\pi  \times 3.5),{\text{ }}\frac{1}{2} \times \pi  \times 7,{\text{ }}3.5\pi \)

valid approach (seen anywhere)     (M1)

eg\(\,\,\,\,\,\)\({\text{perimeter}} = {\text{AB}} + {\text{BC}} + {\text{semicircle, }}3 + 8 + \left( {\frac{1}{2} \times 2 \times \pi  \times \frac{7}{2}} \right),{\text{ }}8 + 3 + 3.5\pi \)

\(11 + \frac{7}{2}\pi {\text{ }}( = 3.5\pi  + 11){\text{ (cm)}}\)     A1     N2

[3 marks]