| Date | May 2014 | Marks available | 3 | Reference code | 14M.2.sl.TZ1.1 |
| Level | SL only | Paper | 2 | Time zone | TZ1 |
| Command term | Find | Question number | 1 | Adapted from | N/A |
Question
The following diagram shows triangle ABC.
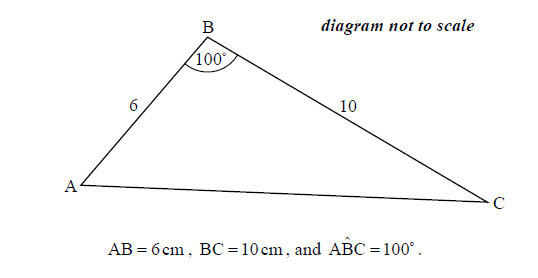
Find AC.
[3]
a.
Find BˆCA.
[3]
b.
Markscheme
evidence of choosing cosine rule (M1)
eg AC2=AB2+BC2−2(AB)(BC)cos(AˆBC)
correct substitution into the right-hand side (A1)
eg 62+102−2(6)(10)cos100∘
AC=12.5234
AC=12.5 (cm) A1 N2
[3 marks]
a.
evidence of choosing a valid approach (M1)
eg sine rule, cosine rule
correct substitution (A1)
eg sin(BˆCA)6=sin100∘12.5, cos(BˆCA)=(AC)2+102−622(AC)(10)
BˆCA=28.1525
BˆCA=28.2∘ A1 N2
[3 marks]
b.
Examiners report
[N/A]
a.
[N/A]
b.

