DP Mathematics: Applications and Interpretation Questionbank

Topic 4—Statistics and probability
Description
[N/A]Directly related questions
-
21M.2.SL.TZ2.1b.iv:
d.
-
21M.2.AHL.TZ2.2d.ii:
Let N be the number of cats weighing over 4.62 kg.
Find the variance of N.
-
21M.2.SL.TZ2.4d:
It is found that 12 of the cats weigh more than x kg. Estimate the value of x.
-
21M.2.AHL.TZ2.2b:
Find the proportion of male Persian cats weighing between 5.5 kg and 6.5 kg.
-
21M.2.AHL.TZ2.2d.i:
Find the probability that exactly one of them weighs over 4.62 kg.
-
21M.2.AHL.TZ2.5c:
Find the probability that over a 12-month period, there will be exactly 3 months when Bill does not visit the garden.
-
21M.3.AHL.TZ1.2c.ii:
Find rs for the seven employees working in the international department.
-
21M.3.AHL.TZ1.2e.ii:
The tests are performed at the 5% significance level.
Assuming that:- there is no correlation between the marks in any of the sections and scores in any of the attributes,
- the outcome of each hypothesis test is independent of the outcome of the other hypothesis tests,
find the probability that at least one of the tests will be significant.
-
21M.3.AHL.TZ1.2d.i:
State the name of this type of test for reliability.
-
21M.3.AHL.TZ2.1a.ii:
Describe one criticism that can be made about the validity of Juliet’s investigation.
-
21M.3.AHL.TZ2.1d.i:
State why the hypothesis test should be one-tailed.
-
21M.3.AHL.TZ2.1c.ii:
Determine the value of r, Pearson’s product-moment correlation coefficient, for these remaining responses.
-
21M.3.AHL.TZ2.1f.iii:
Perform the test, using a 5% significance level, and state your conclusion in context.
-
21M.3.AHL.TZ2.1e.iii:
Find the value of c, of d and of e.
-
20N.1.AHL.TZ0.F_13a.i:
Calculate the value of r, the product moment correlation coefficient of the sample.
-
20N.2.AHL.TZ0.H_9c:
A packet is randomly selected. Given that the packet has a weight greater than 105 g, find the probability that it has a weight greater than 110 g.
-
20N.1.SL.TZ0.S_8a:
Find the value of a.
-
20N.1.SL.TZ0.S_8c:
Find the value of b.
-
20N.1.SL.TZ0.S_8e:
The first 150 athletes that completed the race won a prize.
Given that an athlete took between 22 and m minutes to complete the 5 km race, calculate the probability that they won a prize.
-
20N.2.SL.TZ0.S_9a:
Find the probability that it will take Fiona between 15 minutes and 30 minutes to walk to the bus stop.
-
20N.2.SL.TZ0.S_9b:
Find σ.
-
20N.2.SL.TZ0.S_9e:
This year, Fiona will go to school on 183 days.
Calculate the number of days Fiona is expected to arrive on time.
-
20N.1.SL.TZ0.T_10a:
Write down H0, the null hypothesis for this test.
-
20N.1.SL.TZ0.T_7b:
Using this information, write down an equation in p and q.
-
20N.1.SL.TZ0.T_7d.i:
Using your answers to parts (b) and (c), find the height of Flower A.
-
20N.2.SL.TZ0.T_1b.ii:
Calculate y, the mean time to fully charge the robot.
-
20N.2.SL.TZ0.T_1d.ii:
Describe the correlation between the wind speed and the time to fully charge the robot.
-
20N.2.SL.TZ0.T_2a.ii:
Find the value of b.
-
EXN.1.SL.TZ0.4a:
Find the number of potatoes in the sample with a weight of more than 200 grams.
-
EXN.1.AHL.TZ0.16a:
Find the probability that more than 7 people arrive at the ride before Shunsuke.
-
EXN.2.AHL.TZ0.6f:
Find the probability of a Type II error in the owner’s test.
-
21M.1.SL.TZ1.2c:
the standard deviation.
-
21M.1.AHL.TZ1.14c:
Find the probability that a bag selected at random weighs more than 1 kg.
-
21M.1.AHL.TZ1.11b:
Write down the null and alternative hypotheses.
-
21M.1.SL.TZ2.11b.i:
Write down the degrees of freedom for this test.
-
21M.1.AHL.TZ2.9b.i:
State the null and alternative hypotheses for this test.
-
21M.1.AHL.TZ2.10c:
Find a 95% confidence interval for μ. You may assume that all conditions for a confidence interval have been met.
-
21M.1.AHL.TZ2.15a:
State the null and alternative hypotheses for the test.
-
21M.2.SL.TZ1.1i.ii:
Interpret your result.
-
21M.2.AHL.TZ1.4b.i:
Find the equation of the least squares regression quadratic curve for these four points.
-
21N.1.SL.TZ0.9c.ii:
Find the minimum score needed to obtain a grade 5.
-
21N.1.SL.TZ0.9b:
Find an expression for a in terms of b.
-
21N.1.AHL.TZ0.12a:
Use the data in the second table to find the value of m and the value of b for the regression line, ln x=m(ln d)+b.
-
21N.2.SL.TZ0.1a.iv:
prefers a tablet or is 14–16 years old.
-
21N.2.SL.TZ0.3a.i:
maximum value of h.
-
21N.2.SL.TZ0.5a.ii:
a dart lands more than 15 cm from O.
-
21N.2.AHL.TZ0.3d.i:
Find Arianne’s expected score in the competition.
-
21N.2.AHL.TZ0.7a.ii:
Using the data from Loreto’s sample, perform the hypothesis test at a 5% significance level to determine if Loreto should employ extra staff.
-
21N.3.AHL.TZ0.2g.ii:
Use Euler’s method with a step length of 0.5 minutes to estimate the maximum value of H.
-
22M.1.SL.TZ1.8c:
A bag that weighs more than k grams is rejected by the factory for being overweight. The factory rejects 2% of bags for being overweight.
Find the value of k.
-
22M.1.AHL.TZ1.9b:
A statistician in the company suggests it would be fairer if the company passes the inspection when the mean weight of five randomly chosen bags is greater than 950 g.
Find the probability of passing the inspection if the statistician’s suggestion is followed.
-
22M.1.SL.TZ2.10a:
Determine the probability that a Fuji apple selected at random will be a large apple.
-
22M.2.SL.TZ1.3d.ii:
b.
-
SPM.1.SL.TZ0.9b:
Calculate the p-value for this test.
-
22M.2.SL.TZ2.1f:
Use your graphic display calculator to find an estimate of the mean reaction time.
-
SPM.1.SL.TZ0.9c:
State, giving a reason, whether Ms Calhoun should accept the null hypothesis.
-
22M.2.SL.TZ2.1d.i:
Write down the value of a.
-
SPM.1.SL.TZ0.9a.i:
State the null hypothesis.
-
SPM.2.AHL.TZ0.6a:
Write down a transition matrix T representing the movements between the two companies in a particular year.
-
SPM.3.AHL.TZ0.1c.ii:
Find the standard deviation of the changes.
-
EXM.2.AHL.TZ0.12b.i:
Use all the coordinates in the table to find the equation of the least squares cubic regression curve.
-
EXM.3.AHL.TZ0.4a.ii:
Explain why for any transition state diagram the sum of the out degrees of the directed edges from a vertex (state) must add up to +1.
-
EXM.3.AHL.TZ0.4b:
Write down the transition matrix M, for this Markov chain problem.
-
EXM.2.SL.TZ0.5a:
State the name for this type of sampling technique.
-
22M.2.AHL.TZ2.4e.i:
c.
It is not required to state units for this value.
-
EXM.1.AHL.TZ0.19c:
Calculate Pearson’s product moment correlation coefficient for this data.
-
EXM.1.AHL.TZ0.21c.i:
Write down the coefficient of determination, R2.
-
EXM.1.AHL.TZ0.21d:
Hence predict the temperature of the water after 3 minutes.
-
EXM.3.AHL.TZ0.7d.i:
Show that lny=qx+lnp.
-
EXM.3.AHL.TZ0.8b:
Show that the expected frequency for 20 < x ≤ 4 is 31.5 correct to 1 decimal place.
-
EXM.3.AHL.TZ0.7d.iii:
By finding the equation of a suitable regression line, show that p=1.83 and q=0.986.
-
EXM.3.AHL.TZ0.9f.ii:
c.
-
22M.3.AHL.TZ2.1c:
Find the value of a, of b, and of c. Give your answers to 3 decimal places.
-
EXM.1.AHL.TZ0.55b:
By calculating an appropriate χ2 statistic, test, at the 5% significance level, whether or not the binomial distribution gives a good fit to these data.
-
EXM.1.SL.TZ0.11a:
Test this claim at the 5% level of significance.
-
EXM.1.AHL.TZ0.55a.i:
Calculate the mean number of brown eggs in a box.
-
EXM.2.AHL.TZ0.27b:
Find the mean and standard deviation of the sample data in the table above. Show how you arrived at your answers.
-
EXM.2.AHL.TZ0.28b.ii:
Some time later, the actual value of μ is 503. Find the probability of a Type II error.
-
18N.1.AHL.TZ0.H_1a:
By drawing a Venn diagram, or otherwise, find P(A∪B).
-
18M.1.SL.TZ2.T_9a.iii:
Write down an expression, in set notation, for the shaded region represented by Diagram 3.
-
18M.2.SL.TZ1.S_5b:
Given that P((A ∪ B)′ ) = 0.19, find P(A | B′ ).
-
18M.2.SL.TZ2.T_1c.ii:
Find the value of y.
-
17M.2.AHL.TZ2.H_5c:
Find the probability that the 1st July was calm given that the 3rd July is windy.
-
16N.1.SL.TZ0.S_5a:
Find P(B).
-
17M.1.SL.TZ1.T_7b:
Find the probability that Sara’s baggage arrives in London.
-
19M.2.SL.TZ1.T_3c.iii:
For these data, write down the upper quartile.
-
19M.2.SL.TZ1.T_3f:
Write down the number of degrees of freedom for this test.
-
19M.2.SL.TZ2.T_1e.i:
Find the probability that the student does not take the Spanish class.
-
19M.2.SL.TZ2.T_1e.ii:
Find the probability that neither of the two students take the Spanish class.
-
18N.2.SL.TZ0.S_2b:
Another athlete on this sports team has a hand length of 21.5 cm. Use the regression equation to estimate the height of this athlete.
-
18M.1.SL.TZ1.T_4b:
Using the equation of the regression line, estimate the concentration of dissolved oxygen in the river when the temperature is 18 °C.
-
18M.1.SL.TZ2.T_1a:
Plot and label the point M(ˉm,ˉp) on the scatter diagram.
-
18M.2.SL.TZ1.T_2c:
Write down the number of degrees of freedom.
-
18M.2.SL.TZ1.T_2d.ii:
Write down the associated p-value.
-
18M.2.SL.TZ1.T_2f:
Write down the probability that this flight arrived on time.
-
18M.2.SL.TZ1.T_2h:
Two flights are chosen at random from those which were slightly delayed.
Find the probability that each of these flights travelled at least 5000 km.
-
18M.2.SL.TZ2.T_2b.ii:
Use the cumulative frequency curve to find the lower quartile.
-
18M.2.SL.TZ1.S_8a:
Find the value of a and of b.
-
17M.1.SL.TZ2.S_8b.i:
Find the amount of money an employee earned for working 40 hours;
-
18M.1.AHL.TZ1.H_3a:
Find the value of a and the value of b.
-
16N.2.SL.TZ0.T_1c:
Plot the point (ˉx, ˉy) on your scatter diagram and label this point M.
-
19M.1.SL.TZ1.T_5a:
Complete the cumulative frequency table.
-
19M.1.SL.TZ1.T_5c:
A customer buys a large bouquet.
Find the probability that there are 12 roses in this bouquet.
-
18M.2.SL.TZ2.T_3b.ii:
Find the value of q.
-
17M.2.SL.TZ2.T_1a.i:
If the teacher chooses a response at random, find the probability that it is a response to the Calculus question;
-
17M.2.SL.TZ2.T_1b:
The teacher groups the responses by topic, and chooses two responses to the Logic question. Find the probability that both are not satisfactory.
-
19M.2.SL.TZ1.S_10d:
Ted will always have another turn if he expects an increase to his winnings.
Find the least value of w for which Ted should end the game instead of having another turn.
-
17N.2.SL.TZ0.T_1b.ii:
Write down, for N, the mid-interval value of the modal class.
-
17N.2.SL.TZ0.T_1g:
State the result of the test. Give a reason for your answer.
-
17M.1.SL.TZ1.S_4c:
Jim’s model is d=−2.24t+105, for 0⩽t⩽20. Use his model to predict the decrease in temperature for any 2 minute interval.
-
17M.1.SL.TZ1.T_6d:
State the conclusion for this test. Give a reason for your answer.
-
17M.1.SL.TZ2.T_6b:
Write down the number of leaves with a length less than or equal to 8 cm.
-
17M.2.SL.TZ1.T_5f.i:
Calculate the probability that a student chosen at random spent at least 90 minutes preparing for the test.
-
17M.2.SL.TZ2.S_2a.ii:
Write down the value of r.
-
17N.2.SL.TZ0.S_4b:
Write down P(X=2).
-
17M.2.SL.TZ2.S_10a.ii:
Find p.
-
17M.2.SL.TZ2.S_10b.ii:
Explain why the probability of drawing three white marbles is 16.
-
17M.2.AHL.TZ2.H_10e:
Find E(Y).
-
19M.2.AHL.TZ1.H_9c.i:
A customer is selected at random. Find the probability that the customer is male and buys a sandwich.
-
18N.3.AHL.TZ0.Hsp_1b:
Var(4X−3Y).
-
18N.3.AHL.TZ0.Hsp_1c:
E(X2−Y2).
-
17N.2.AHL.TZ0.H_4:
It is given that one in five cups of coffee contain more than 120 mg of caffeine.
It is also known that three in five cups contain more than 110 mg of caffeine.Assume that the caffeine content of coffee is modelled by a normal distribution.
Find the mean and standard deviation of the caffeine content of coffee. -
17M.2.SL.TZ1.S_9d:
Given that Y>9, find P(Y<13).
-
18N.1.SL.TZ0.S_9a.ii:
Find the probability, in terms of n, that the game will end on her second draw.
-
18M.2.SL.TZ1.S_2b:
Calculate the expected value of the score.
-
19M.1.SL.TZ1.S_1c:
Find P(A′∪B).
-
18N.1.SL.TZ0.T_14a:
Find the value of k.
-
17M.1.SL.TZ2.S_3c:
Find P(93<X<107).
-
18N.2.AHL.TZ0.H_10a.i:
Using this distribution model, find P(X<60).
-
18N.1.SL.TZ0.T_8b:
Find the probability that Jorgé chooses a red disc.
-
18N.2.SL.TZ0.T_2c.i:
Find the probability that this student studies Mathematics.
-
19M.1.SL.TZ2.T_11b.i:
Write down the elements that belong to A∩B′.
-
19M.1.SL.TZ1.S_9c:
Write down the standardized value for x=1.
-
18N.2.SL.TZ0.S_9c:
A randomly selected participant has a reaction time greater than 0.65 seconds. Find the probability that the participant is in Group X.
-
18M.2.AHL.TZ2.H_3a:
Sketch the probability density function for X, and shade the region representing P(μ − 2σ < X < μ + σ).
-
18N.2.AHL.TZ0.H_10e:
Now suppose that Archie received exactly 20 emails in total in a consecutive two day period. Show that the probability that he received exactly 10 of them on the first day is independent of λ.
-
18M.1.AHL.TZ2.H_3a:
Find the value of p.
-
18M.1.AHL.TZ2.H_3b.i:
Find μ, the expected value of X.
-
19M.1.AHL.TZ1.H_10a.i:
Find the remainder when p(x) is divided by (x−2).
-
18M.3.AHL.TZ0.Hsp_3d:
Another model of smartphone whose battery life may be assumed to be normally distributed with mean μ hours and standard deviation 1.2 hours is tested. A researcher measures the battery life of six of these smartphones and calculates a confidence interval of [10.2, 11.4] for μ.
Calculate the confidence level of this interval.
-
18M.1.SL.TZ1.T_13a.ii:
Find the probability that a student, chosen at random arrives between 45 minutes and 55 minutes after the school opens.
-
18M.1.SL.TZ1.T_13b:
A second school, Mulberry Park, also opens at 08:00 every morning. The arrival times of the students at this school follows exactly the same distribution as Malthouse school.
Given that, on one morning, 15 students arrive at least 60 minutes after the school opens, estimate the number of students at Mulberry Park school.
-
17M.2.AHL.TZ1.H_9c:
Find the standard deviation of the times taken by female runners.
-
17M.2.AHL.TZ2.H_3b:
Calculate the new value of μ giving your answer correct to two decimal places.
-
17N.2.SL.TZ0.T_4c:
Copy and complete the tree diagram.
-
17N.2.SL.TZ0.T_4e:
Find the probability that the liquid turns blue.
-
17N.2.SL.TZ0.T_4g:
Estimate the number of employees, from this 38, who are allergic to nuts.
-
18N.3.AHL.TZ0.Hsp_3a.i:
Find the probability that a fish from this lake will have a weight of more than 560 grams.
-
19M.3.AHL.TZ0.Hsp_3c:
Find an unbiased estimate for σ2.
-
18N.3.AHL.TZ0.Hsp_2b:
Assuming that T is normally distributed, find
(i) the 90% confidence interval for the mean time taken to travel to work by the workers of this company,
(ii) the 95% confidence interval for the mean time taken to travel to work by the workers of this company.
-
18M.3.AHL.TZ0.Hsp_1a:
Find the probability that a randomly chosen male bird weighs between 4.75 kg and 4.85 kg.
-
17N.3.AHL.TZ0.Hsp_4a:
State suitable hypotheses to investigate whether or not U, V are independent.
-
17M.3.AHL.TZ0.Hsp_1b:
Find unbiased estimates of μ and σ2.
-
16N.2.AHL.TZ0.H_11b:
Find the values of the constants a and b.
-
18N.2.SL.TZ0.S_10b.ii:
During the interval p < t < q, he volume of water in the container increases by k m3. Find the value of k.
-
18N.2.SL.TZ0.S_10c:
When t = 0, the volume of water in the container is 2.3 m3. It is known that the container is never completely full of water during the 4 hour period.
Find the minimum volume of empty space in the container during the 4 hour period.
-
19N.3.AHL.TZ0.Hsp_3a:
State the central limit theorem as applied to a random sample of size n, taken from a distribution with mean μ and variance σ2.
-
19N.3.AHL.TZ0.Hsp_3c.iii:
Given that the probability that Josie makes a Type II error is 0.25, find the value of μ, giving your answer correct to three significant figures.
-
17M.2.SL.TZ2.S_8c.ii:
Find the the rate of change of f at B.
-
19N.3.AHL.TZ0.Hsp_1b:
Peter uses the regression line of y on x as y=0.248x+83.0 and calculates that a student with a Mathematics test score of 73 will have a running time of 101 seconds. Comment on the validity of his calculation.
-
19N.2.AHL.TZ0.H_2a:
Calculate the probability that Audrey will run at least two marathons in a particular year.
-
19N.1.SL.TZ0.T_4c:
Write down an element that belongs to (A∪B)′∩C.
-
17N.1.AHL.TZ0.H_10b.i:
Determine the mean of X.
-
17N.1.AHL.TZ0.H_10a:
Show that the probability that Chloe wins the game is 38.
-
21M.2.SL.TZ2.1c.ii:
will test negative.
-
21M.2.SL.TZ2.1b.i:
a.
-
21M.2.SL.TZ2.1c.iii:
has the disease given that they tested negative.
-
21M.2.SL.TZ2.1d:
The medical centre finds the actual number of positive results in their sample is different than predicted by the tree diagram. Explain why this might be the case.
-
21M.2.SL.TZ2.4a:
Sketch a diagram showing the above information.
-
21M.2.AHL.TZ2.2a:
Sketch a diagram showing the above information.
-
21M.2.AHL.TZ2.5b.ii:
during the first and third month only.
-
21M.2.AHL.TZ2.5d:
After the first year, a number of baby magpies start to visit Hank’s garden. It may be assumed that each of these baby magpies visits the garden randomly and independently, and that the number of times each baby magpie visits the garden per month is modelled by a Poisson distribution with mean 2.1.
Determine the least number of magpies required, including Bill, in order that the probability of Hank’s garden having at least 30 magpie visits per month is greater than 0.2.
-
21M.2.AHL.TZ2.4b:
Find a prediction for the ratio of the number of patients Doctor Black will have, compared to Doctor Green, after two years.
-
21M.3.AHL.TZ1.2c.i:
Without calculation, explain why it might not be appropriate to calculate a correlation coefficient for the whole sample of 18 employees.
-
21M.3.AHL.TZ2.1e.i:
Use Juliet’s data to find the value of a and of b.
-
20N.2.AHL.TZ0.H_5a.ii:
Find the probability that at least three people from the sample took a holiday in the Lake District in 2019.
-
20N.2.AHL.TZ0.H_9e:
Packets are delivered to supermarkets in batches of 80. Determine the probability that at least 20 packets from a randomly selected batch have a weight less than 95 g.
-
20N.1.SL.TZ0.T_14c:
Given that Andre did not become the champion, find the probability that he lost in the semi-final.
-
20N.1.SL.TZ0.T_6a.i:
Find the probability that the first ball chosen is labelled A.
-
20N.1.SL.TZ0.T_7d.ii:
Using your answers to parts (b) and (c), find the height of Flower D.
-
20N.2.SL.TZ0.T_1a:
On graph paper, draw a scatter diagram to show the results of Don’s investigation. Use a scale of 1 cm to represent 2 units on the x-axis, and 1 cm to represent 5 units on the y-axis.
-
20N.2.SL.TZ0.T_2e:
Hence determine whether the events in parts (d)(i) and (d)(ii) are independent. Justify your reasoning.
-
20N.2.SL.TZ0.T_6g:
Emlyn claims the results from Saturday and Sunday show that his expected number of successful shots will be more than Johan’s.
Determine if Emlyn’s claim is correct. Justify your reasoning.
-
18M.2.SL.TZ1.T_5c:
Copy the probability tree diagram and write down the relevant probabilities along the branches.
-
EXN.1.SL.TZ0.4b.i:
Find the median weight.
-
EXN.1.SL.TZ0.1b:
The Principal selects the students for the sample by asking those who took part in a previous survey if they would like to take part in another. She takes the first of those who reply positively, up to the maximum needed for the sample.
State which two of the sampling methods listed below best describe the method used.
Stratified Quota Convenience Systematic Simple random
-
EXN.1.SL.TZ0.8d:
State a more appropriate model for the water temperature in the lake over an extended period of time. You are not expected to calculate any parameters.
-
EXN.1.SL.TZ0.12b:
Find the value of u1.
-
EXN.1.SL.TZ0.12c:
A game is played in which the arrow attached to the centre of the disc is spun and the sector in which the arrow stops is noted. If the arrow stops in sector 1 the player wins 10 points, otherwise they lose 2 points.
Let X be the number of points won
Find E(X).
-
EXN.2.SL.TZ0.4c.iv:
Determine the conclusion of the test, clearly justifying your answer.
-
EXN.2.AHL.TZ0.3a:
Use the given value of sn to find the value of sn-1.
-
EXN.2.AHL.TZ0.6d:
Find the critical region for this test.
-
EXN.2.AHL.TZ0.6c:
Write down the null and alternative hypotheses for the test.
-
21M.1.SL.TZ1.6b:
Calculate the p-value for this test.
-
21M.1.SL.TZ2.11a:
Find an estimate for how many copies the vendor expects to sell each day.
-
21M.1.SL.TZ2.11b.ii:
Write down the conclusion to the test. Give a reason for your answer.
-
21M.2.SL.TZ1.4c:
State the null and alternative hypotheses.
-
21M.2.AHL.TZ1.5a:
It is sunny today. Find the probability that it will be sunny in three days’ time.
-
21N.1.SL.TZ0.1a:
For this data, find the value of the Pearson’s product-moment correlation coefficient, r.
-
21N.1.SL.TZ0.1d:
A 57-year-old male also ran in the 5000 m race.
Use the equation of the regression line to estimate the time he took to complete the 5000 m race.
-
21N.1.SL.TZ0.11c.ii:
c.
-
21N.1.AHL.TZ0.12b:
Assuming that the model found in part (a) remains valid, estimate the percentage of trees in stock when d=25.
-
21N.2.SL.TZ0.3b.i:
Find the time, in seconds, it takes for the blade [BC] to make one complete rotation under these conditions.
-
21N.2.SL.TZ0.5d.i:
Find the probability that Arianne scores at least 5 points in the competition.
-
21N.2.AHL.TZ0.3a.i:
a dart lands less than 13 cm from O.
-
21N.2.AHL.TZ0.3d.iii:
Find the probability that Arianne scores at least 5 points and less than 8 points.
-
21N.2.AHL.TZ0.3c:
Find the probability that Arianne does not score a point on a turn of three darts.
-
21N.3.AHL.TZ0.2e:
Use the general solution from part (d) and the initial condition h(0)=3.2 to predict the value of T.
-
21N.3.AHL.TZ0.2f:
Find this new height.
-
22M.1.SL.TZ1.7d:
Find the p-value for the test.
-
22M.1.SL.TZ1.10c:
Given that Karl has two socks of the same colour find the probability that he has two brown socks.
-
22M.1.AHL.TZ2.12a:
Find the probability of making a Type I error when weighing a male cuttlefish.
-
22M.2.SL.TZ1.3a.i:
the upper quartile.
-
22M.2.SL.TZ1.3e.i:
Find the value of the Spearman’s rank correlation coefficient rs.
-
22M.2.SL.TZ1.5b.ii:
Find the maximum number of tickets that could be sold if the expected number of passengers who arrive to board the flight must be less than or equal to 72.
-
22M.2.AHL.TZ1.3e.i:
their mean standardized score.
-
22M.2.AHL.TZ1.3e.ii:
the standard deviation of their standardized score.
-
SPM.1.SL.TZ0.9a.ii:
State the alternative hypothesis.
-
22M.2.SL.TZ2.1a.i:
median reaction time.
-
22M.2.SL.TZ2.1e:
Write down the modal class from the table.
-
SPM.1.SL.TZ0.13c:
Find the probability he will choose a male student at most 9 times.
-
SPM.1.AHL.TZ0.12:
Product research leads a company to believe that the revenue (R) made by selling its goods at a price (p) can be modelled by the equation.
R(p)=cpedp, c, d∈R
There are two competing models, A and B with different values for the parameters c and d.
Model A has c = 3, d = −0.5 and model B has c = 2.5, d = −0.6.
The company experiments by selling the goods at three different prices in three similar areas and the results are shown in the following table.
The company will choose the model with the smallest value for the sum of square residuals.
Determine which model the company chose.
-
SPM.1.AHL.TZ0.16a:
State a suitable null and alternative hypotheses for Tom’s test.
-
SPM.1.AHL.TZ0.17a:
Find the probability he will choose a female student 8 times.
-
SPM.1.AHL.TZ0.17b:
The Head of Year, Mrs Smith, decides to select a student at random from the year group to read the notices in assembly. There are 80 students in total in the year group. Mrs Smith calculates the probability of picking a male student 8 times in the first 20 assemblies is 0.153357 correct to 6 decimal places.
Find the number of male students in the year group.
-
SPM.2.AHL.TZ0.6b:
Find the eigenvalues and corresponding eigenvectors of T.
-
EXM.1.SL.TZ0.3c:
Calculate Spearman’s rank correlation coefficient for this data.
-
EXM.1.AHL.TZ0.21c.ii:
Interpret what the value of R2 tells you about the model.
-
EXM.2.SL.TZ0.5b:
Calculate the number of volunteers in the sample under the age of 30.
-
EXM.1.SL.TZ0.5c:
Give an example of a set of data with 7 numbers in it that does have an outlier, justify this fact by stating the Interquartile Range.
-
EXM.1.AHL.TZ0.19a:
State the name of this test for reliability.
-
EXM.3.AHL.TZ0.7c.ii:
Find the value of this area.
-
EXM.3.AHL.TZ0.8a.i:
Find unbiased estimates for the population mean.
-
EXM.3.AHL.TZ0.7d.ii:
Hence explain how a straight line graph could be drawn using the coordinates in the table.
-
EXM.3.AHL.TZ0.9g:
Hence predict the total number of people infected by this disease after several months.
-
EXM.3.AHL.TZ0.9h:
Use the logistic model to find the day when the rate of increase of people infected is greatest.
-
22M.3.AHL.TZ2.1f:
By considering the claims of both Aimmika and Nichakarn, explain whether the advertising was beneficial to the store.
-
EXM.1.AHL.TZ0.57b:
The number of cars passing a certain point in a road was recorded during 80 equal time intervals and summarized in the table below.
Carry out a χ2 goodness of fit test at the 5% significance level to decide if the above data can be modelled by a Poisson distribution.
-
EXM.1.AHL.TZ0.56b.i:
Write down appropriate hypotheses.
-
EXM.1.AHL.TZ0.59b.i:
State suitable hypotheses.
-
EXM.2.AHL.TZ0.27d:
In order to test for the goodness of fit, the test statistic was calculated to be 1.0847. Show how this was done.
-
EXM.2.AHL.TZ0.27e:
State your hypotheses, critical number, decision rule and conclusion (using a 5% level of significance).
-
EXM.2.AHL.TZ0.28b.i:
Find the significance level of this procedure.
-
18M.1.SL.TZ2.T_7a:
State the number of boys who answered questions in Portuguese.
-
16N.1.SL.TZ0.T_12a:
Complete the tree diagram below.
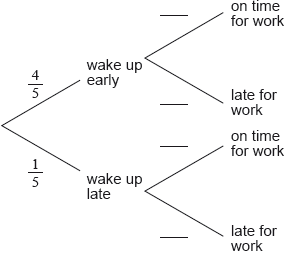
-
17M.1.SL.TZ1.T_7a:
Complete the tree diagram.
-
19M.2.SL.TZ1.T_3b:
Write down the modal number of pets.
-
19M.2.SL.TZ1.T_3e.ii:
State the alternative hypothesis.
-
19M.2.SL.TZ1.T_3i:
State the conclusion for this test. Give a reason for your answer.
-
19M.2.SL.TZ2.S_1a.ii:
Find the correlation coefficient.
-
19M.2.SL.TZ2.T_1d:
State whether or not H0 should be rejected. Justify your statement.
-
19M.2.AHL.TZ1.H_3b.ii:
the standard deviation.
-
18N.2.SL.TZ0.T_1c.i:
Use the regression line y on x to estimate Jerome’s examination score.
-
16N.2.SL.TZ0.T_2b:
Show that x=3.
-
18M.1.SL.TZ1.T_2a:
Find the value of x.
-
18M.2.SL.TZ1.T_2b:
Calculate the expected frequency of flights travelling at most 500 km and arriving slightly delayed.
-
18M.2.SL.TZ1.T_2d.i:
Write down the χ2 statistic.
-
18M.2.SL.TZ2.T_2b.iii:
Use the cumulative frequency curve to find the upper quartile.
-
19M.1.SL.TZ2.T_12a.ii:
q.
-
17M.1.SL.TZ2.S_8a.ii:
Write down the number of employees who worked 50 hours or less.
-
16N.2.SL.TZ0.T_1e:
Write down the equation of the regression line y on x for these eight male students.
-
16N.1.SL.TZ0.T_2b:
Draw a box-and-whisker diagram on the grid below to represent the Vitamin C content, in milligrams, for this sample.

-
18M.2.SL.TZ1.S_9b.i:
Find the standardized value for 289 g.
-
17M.1.AHL.TZ1.H_10b.ii:
By considering the graph of f write down the median of X;
-
18M.1.SL.TZ1.S_2a:
Find the value of the interquartile range.
-
17N.2.SL.TZ0.T_1e.ii:
Write down the number of degrees of freedom.
-
17M.1.SL.TZ1.T_1a:
Complete the following table.
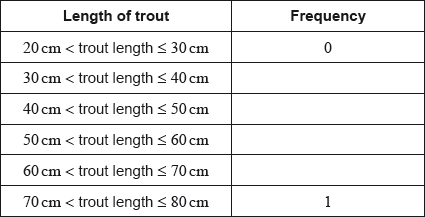
-
17M.2.SL.TZ1.T_5b:
Find the median test grade of the students.
-
17M.2.AHL.TZ2.H_10b:
Find E(X).
-
19M.2.AHL.TZ1.H_9c.ii:
A female customer is selected at random. Find the probability that she buys a sandwich.
-
18N.3.AHL.TZ0.Hsp_1a:
E(2X+7Y).
-
17M.2.SL.TZ1.S_9b:
Find the value of μ.
-
18N.1.SL.TZ0.S_9a.i:
Find the probability, in terms of n, that the game will end on her first draw.
-
18N.1.SL.TZ0.S_9b.i:
third draw.
-
19M.1.SL.TZ1.T_12c:
Write down the probability that the second spin is yellow, given that the first spin is blue.
-
17N.1.SL.TZ0.T_13b:
Find the value of k.
-
17N.2.SL.TZ0.S_7:
The heights of adult males in a country are normally distributed with a mean of 180 cm and a standard deviation of σ cm. 17% of these men are shorter than 168 cm. 80% of them have heights between (192−h) cm and 192 cm.
Find the value of h.
-
16N.2.SL.TZ0.T_4d:
Calculate the value of w.
-
19M.1.SL.TZ1.S_9d:
It is also known that P(x > 2) = b.
Find s.
-
18N.2.AHL.TZ0.H_10b.i:
an estimate for the mean number of emails received per working day.
-
16N.2.SL.TZ0.T_6g:
Find the value of this minimum area.
-
16N.2.SL.TZ0.T_6h:
Find the least number of cans of water-resistant material that will coat the area in part (g).
-
17M.2.SL.TZ2.S_B10c:
Jill plays the game nine times. Find the probability that she wins exactly two prizes.
-
17M.2.AHL.TZ1.H_9a:
Find the probability that a runner selected at random will complete the marathon in less than 3 hours.
-
17N.1.SL.TZ0.T_7a:
Complete the Venn diagram for these students.
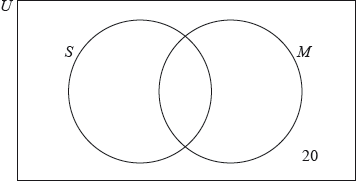
-
17N.1.SL.TZ0.T_7c:
Determine whether the events S and M are independent.
-
16N.2.AHL.TZ0.H_8b:
Find P(|X−μ|<1.2σ).
-
17N.2.SL.TZ0.T_4d:
Find the probability that this adult is allergic to nuts and the liquid turns blue.
-
18N.3.AHL.TZ0.Hsp_3c.i:
State suitable hypotheses for the test.
-
17N.3.AHL.TZ0.Hsp_2b.ii:
Interpret your p-value at the 5% level of significance, justifying your conclusion.
-
19N.3.AHL.TZ0.Hsp_3b:
Jack takes a random sample of size 100 and calculates that ˉx=60.2. Find an approximate 90 % confidence interval for μ.
-
19N.1.SL.TZ0.T_13b:
Write down the modal grade of the eggs.
-
19N.1.SL.TZ0.T_9c:
Sungwon will play the game for 11 turns.
Find the expected number of times the score on a turn is greater than 4.
-
21M.2.SL.TZ2.1f:
Find the total number of patients who visited the centre during this day.
-
21M.2.AHL.TZ2.4c:
Find a matrix P, with integer elements, such that T=PD P−1, where D is a diagonal matrix.
-
21M.3.AHL.TZ1.2a:
Use an appropriate test, at the 5% significance level, to determine whether a new employee staying with the firm is independent of their interview rating. State the null and alternative hypotheses, the p-value and the conclusion of the test.
-
21M.3.AHL.TZ1.2c.iii:
Hence comment on the validity of the written assessment as a measure of the level of performance of employees in this department. Justify your answer.
-
21M.3.AHL.TZ2.1c.i:
Calculate the mean annual income for these remaining responses.
-
21M.3.AHL.TZ2.1d.iii:
The critical value for this test, at the 5% significance level, is 0.549. Juliet assumes that the population is bivariate normal.
Determine whether there is significant evidence of a positive correlation between annual income and happiness. Justify your answer.
-
21M.3.AHL.TZ2.1e.iv:
Find the coefficient of determination for each of the two models she considers.
-
20N.3.AHL.TZ0.Hsp_3b:
Find the least value of n required to ensure that the width of the confidence interval is less than 2 grams.
-
20N.1.SL.TZ0.T_3b:
Use your graphic display calculator to find an estimate of the standard deviation of the weights of mangoes from this harvest.
-
20N.1.SL.TZ0.T_6b:
Find the probability that the second ball chosen is labelled A, given that the first ball chosen was labelled N.
-
20N.2.SL.TZ0.T_2a.i:
Find the value of a.
-
20N.2.SL.TZ0.T_6b:
Show that m=15.7.
-
20N.2.SL.TZ0.T_6d:
Find the value of x.
-
18M.1.SL.TZ2.T_7c:
Two girls are selected at random.
Calculate the probability that one girl answered questions in Mandarin and the other answered questions in Hindi.
-
EXN.1.SL.TZ0.3b.i:
Find the p-value for the owner’s test.
-
EXN.1.SL.TZ0.1a:
Calculate the number of grade 12 students who should be in the sample.
-
EXN.1.SL.TZ0.8a:
Assuming the data follows a linear model for this period, find the regression line of T on m for the remaining data.
-
EXN.1.SL.TZ0.8c.i:
Explain why your line should not be used to estimate the value of m at which the temperature is 10.0 °C.
-
EXN.1.SL.TZ0.8b:
Use your line to find an estimate for the water temperature on the first day of May.
-
EXN.1.SL.TZ0.8c.ii:
Explain in context why your line should not be used to predict the value for December (month 12).
-
EXN.1.SL.TZ0.10d:
Find the probability it will rain at least once while Paula is outside.
-
EXN.1.SL.TZ0.10b:
Find the probability it will rain in each of the three hours Paula is working outside.
-
EXN.1.AHL.TZ0.12c.i:
Use your answer to part (b) to write down the value of n to the nearest integer.
-
EXN.1.AHL.TZ0.12b:
Find the equation of the least squares regression line of log10 P against log10 d.
-
EXN.1.AHL.TZ0.12a:
Explain why this graph indicates that P is inversely proportional to dn.
-
EXN.2.SL.TZ0.1c:
Which of the correlation coefficients would you recommend is used to assess whether or not there is an association between total number of minutes late and distance from school? Fully justify your answer.
-
EXN.2.SL.TZ0.4c.ii:
Write down the null and alternative hypotheses.
-
EXN.3.AHL.TZ0.1e.ii:
Hence find upper and lower bounds for the number of fish in the lake when t=8.
-
EXN.2.AHL.TZ0.6e:
Find the mean and standard deviation of the mass of the melons for this year.
-
21M.1.SL.TZ1.6c:
State whether the result of the test supports Arriane’s claim. Justify your reasoning.
-
21M.1.SL.TZ1.6a:
State, in words, the null hypothesis.
-
21M.1.AHL.TZ2.10d:
Suggest, with justification, a valid conclusion that Talha could make.
-
21M.1.AHL.TZ2.10a:
Find an unbiased estimate for the mean number (μ) of chocolates per packet.
-
21M.1.AHL.TZ2.13c:
Comment on your answer to part (b), referring to at least one limitation of the model.
-
21M.2.SL.TZ1.1a:
State which of the two sampling methods, systematic or quota, Jason has used.
-
21M.2.SL.TZ1.1g:
State whether it is valid to use the regression line y on x for Jason’s estimate. Give a reason for your answer.
-
21M.2.SL.TZ1.1i.i:
Calculate the value of rs.
-
21M.2.SL.TZ1.4a.i:
in less than 6.5 m.
-
21M.2.SL.TZ1.4a.ii:
in more than 7 m.
-
21M.2.AHL.TZ1.5b:
Find the eigenvalues and eigenvectors of T.
-
21M.2.AHL.TZ1.4b.ii:
By considering the gradient of this curve when x=4, explain why it may not be a good model.
-
21N.1.SL.TZ0.11c.i:
b.
-
21N.1.SL.TZ0.9c.i:
Find the number of students who obtained a grade 5.
-
21N.1.AHL.TZ0.14c:
The sacks of potatoes are transported in crates. There are 10 sacks in each crate and the weights of the sacks of potatoes are independent of each other.
Find the probability that the total weight of the sacks of potatoes in a crate exceeds 500 kg.
-
21N.2.SL.TZ0.3b.ii:
Calculate the angle, in degrees, that the blade [BC] turns through in one second.
-
21N.2.AHL.TZ0.3d.iv:
Given that Arianne scores at least 5 points, find the probability that Arianne scores less than 8 points.
-
21N.3.AHL.TZ0.2b.i:
Find the equation of the least squares quadratic regression curve.
-
19M.1.SL.TZ2.T_5a:
Using the given information, complete the following Venn diagram.
-
22M.1.SL.TZ1.7c:
Write down the expected frequency of rolling a 1.
-
22M.1.SL.TZ1.8b:
Find the probability that a randomly chosen bag is rejected for being underweight.
-
22M.1.SL.TZ2.10b:
Find the value of k.
-
22M.1.SL.TZ2.8c.ii:
State what your conclusion means in context.
-
22M.2.SL.TZ1.5a:
The airline sells 74 tickets for this flight. Find the probability that more than 72 passengers arrive to board the flight.
-
SPM.1.AHL.TZ0.9a:
Find sn−1 for this sample.
-
SPM.2.SL.TZ0.3e.i:
Find the value of the Spearman’s rank correlation coefficient, rs.
-
SPM.2.AHL.TZ0.6e:
Hence write down the number of customers that company X can expect to have in the long term.
-
22M.3.AHL.TZ2.1e.i:
By finding a critical value, perform this test at a 5 % significance level.
-
EXM.2.AHL.TZ0.12c.ii:
Find the value of this area.
-
EXM.1.SL.TZ0.3d:
State what conclusion Charles can make from the answer in part (c).
-
EXM.2.AHL.TZ0.12c.i:
Write down an expression for the area enclosed by the cubic regression curve, the x-axis, the y-axis and the line x=10.
-
EXM.3.AHL.TZ0.4g:
Explain how your answer to part (f) fits with your answer to part (c).
-
EXM.3.AHL.TZ0.4c.ii:
Explain which part of the transition state diagram confirms this.
-
EXM.1.AHL.TZ0.20b:
Calculate Pearson’s product moment correlation coefficient for this data.
-
EXM.2.SL.TZ0.5f:
Find the p-value for this t-test.
-
EXM.3.AHL.TZ0.4h:
Find the minimum number of tosses of the coin that Abi will have to make to be at least 95% certain of having finished the game by reaching state C.
-
EXM.2.SL.TZ0.5g:
State the conclusion of this test, in context, giving a reason.
-
EXM.1.SL.TZ0.5b:
Hence, show that a data set with only 5 numbers in it cannot have any outliers.
-
EXM.2.SL.TZ0.5c.ii:
The current drug.
-
EXM.3.AHL.TZ0.8d:
Use the normal distribution model to find the score required to pass.
-
EXM.3.AHL.TZ0.9b.i:
the number of new people infected on day 6.
-
EXM.3.AHL.TZ0.7d.iv:
Hence find the area enclosed by the exponential function, the x-axis, the y-axis and the line x=4.4.
-
EXM.3.AHL.TZ0.8a.ii:
Find unbiased estimates for the population Variance.
-
22M.2.AHL.TZ2.5d.ii:
in the long term.
-
22M.3.AHL.TZ1.1b.iii:
Write down the value of R2 for this model.
-
22M.3.AHL.TZ1.1a.iii:
Explain why it would not be appropriate to conduct a hypothesis test on the value of r found in (a)(ii).
-
22M.3.AHL.TZ1.1f.i:
Use linear regression to estimate the value of k and of L.
-
22M.3.AHL.TZ2.1d.i:
Write down the number of degrees of freedom for her test.
-
EXM.1.SL.TZ0.10a:
Showing all steps clearly, test whether the die is fair
(i) at the 5% level of significance;
(ii) at the 1% level of significance.
-
EXM.1.AHL.TZ0.59b.ii:
Calculate the value of the χ2 statistic and state your conclusion using a 10% level of significance.
-
EXM.2.AHL.TZ0.26b:
The data from the two samples above are combined to form a single set of data. The following frequency table gives the observed frequencies for the combined sample. The data has been divided into five intervals.
Test, at the 5% level, whether the combined data can be considered to be a sample from a normal population with a mean of 380.
-
18M.1.SL.TZ1.T_10b:
Find the value of x.
-
18M.2.SL.TZ1.S_5a:
Find P(A ∩ B′ ).
-
18M.2.SL.TZ1.T_5d.i:
A contestant is chosen at random. Find the probability that this contestant fell into a trap while attempting to pass through a door in the second wall.
-
19M.2.SL.TZ1.T_1b.i:
Write down, for this set of data the mean temperature difference from 37 °C, ˉx.
-
19M.2.SL.TZ1.T_1b.ii:
Write down, for this set of data the mean number of heartbeats per minute, ˉy.
-
19M.2.SL.TZ1.T_3d:
Write down the ratio of teenagers to non-teenagers in its simplest form.
-
19M.2.SL.TZ1.T_3g:
Calculate the expected number of teenagers that prefer cats.
-
19M.2.SL.TZ2.T_1c.i:
the expected frequency of female students who chose to take the Chinese class.
-
16N.2.SL.TZ0.T_2a:
Draw a Venn diagram to represent the given information, using sets labelled B, C and H.
-
18M.1.SL.TZ2.T_12a:
Write down the mid-interval value for 10 ≤ t < 15.
-
18M.2.SL.TZ2.S_1a.ii:
Write down the correlation coefficient.
-
19M.1.SL.TZ2.T_12c:
Use the cumulative frequency curve to find an estimate for the number of students who worked at most 35 hours per month.
-
16N.2.SL.TZ0.S_8d:
(i) Find the number of students who spent between 25 and 30 hours browsing the Internet.
(ii) Given that 10% of the students spent more than k hours browsing the Internet, find the maximum value of k.
-
19M.1.SL.TZ1.T_11c:
Any suitcase that weighs more than k kg is identified as excess baggage.
19.6 % of the suitcases at this airport are identified as excess baggage.Find the value of k.
-
18M.2.SL.TZ2.T_3d.ii:
For this test find the p-value.
-
17M.2.SL.TZ2.T_1c:
State the null hypothesis for this test.
-
17M.2.SL.TZ2.T_3e:
State a reason why the regression line C on x is appropriate to model the relationship between these variables.
-
17M.2.SL.TZ2.T_3g:
Draw the regression line C on x on the scatter diagram.
-
16N.1.SL.TZ0.T_6b:
For this test, write down the χ2 statistic.
-
19M.2.SL.TZ2.S_9a:
Show that event A and event D are not independent.
-
18M.2.AHL.TZ2.H_8b:
It is further given that P(X ≤ 1) = 0.09478 correct to 4 significant figures.
Determine the value of n and the value of p.
-
18N.2.AHL.TZ0.H_3b:
Find the probability that a randomly selected Infiglow battery will have a life of at least 15 hours.
-
17N.2.SL.TZ0.S_8a:
Write down the value of a and of b.
-
17N.2.SL.TZ0.T_1c.i:
Use your graphic display calculator to estimate the mean of N;
-
17N.2.SL.TZ0.T_1d:
Find the expected frequency of students choosing the Science category and obtaining 31 to 40 correct answers.
-
17N.2.SL.TZ0.T_1e.i:
Write down the null hypothesis for this test;
-
17N.2.SL.TZ0.T_1f.ii:
Write down the χ2 statistic.
-
17M.1.SL.TZ1.T_6b:
Write down the number of degrees of freedom for this test.
-
17M.1.SL.TZ2.T_7a:
Write down an equation, in terms of x and y, for the total number of times the die was rolled.
-
17M.2.SL.TZ1.T_5e:
Given that the first student chosen at random scored a grade 5 or higher, find the probability that both students scored a grade 6.
-
17N.2.SL.TZ0.S_4a:
Find the value of k.
-
17M.2.SL.TZ2.S_10a.i:
Find q.
-
17M.2.AHL.TZ2.H_10a:
Show that a=32 and b=112.
-
19M.2.SL.TZ1.S_9a:
Find the gradient of L.
-
19M.2.SL.TZ1.S_9d.iii:
Hence or otherwise, find the obtuse angle formed by the tangent line to f at x=8 and the tangent line to f at x=2.
-
19M.2.AHL.TZ1.H_9a.ii:
only a sandwich.
-
18N.1.SL.TZ0.T_8a:
Complete the tree diagram.
-
18N.2.SL.TZ0.T_2a.iii:
Find the number of students in the school that study both Biology and Mathematics.
-
18N.2.SL.TZ0.T_2b.i:
Write down n(S∩(M∪B)).
-
18N.2.SL.TZ0.T_2b.ii:
Write down n(B∩M∩S′).
-
19M.1.SL.TZ1.T_7b:
In the table indicate which two of the given statements are true by placing a tick (✔) in the right hand column.
-
16N.2.SL.TZ0.T_4c:
Calculate the manufacturer’s expected daily recycling cost.
-
19M.1.SL.TZ1.S_9a:
Find P(−1.6 < z < 2.4). Write your answer in terms of a and b.
-
17M.2.SL.TZ1.S_4b.ii:
Hence, find the probability that fewer than k students are left handed.
-
19M.1.AHL.TZ1.H_10b:
Prove that p(x) has only one real zero.
-
19M.1.AHL.TZ1.H_10c:
Write down the transformation that will transform the graph of y=p(x) onto the graph of y=8x3−12x2+16x−24.
-
16N.2.AHL.TZ0.H_3b:
Given that P(X=2)=0.241667 and P(X=3)=0.112777, use part (a) to find the value of μ.
-
19M.2.AHL.TZ2.H_3b:
Calculate the probability that Iqbal passes at least two of the papers he attempts.
-
17M.2.AHL.TZ2.H_3a:
Given that μ=253 and σ=1.5 find the probability that a randomly chosen packet of biscuits is underweight.
-
17M.1.SL.TZ1.T_4c.ii:
Write down n(W∩S).
-
17M.1.SL.TZ2.T_10b.ii:
Find the probability that at least one of Francesco’s light bulbs is defective.
-
18N.3.AHL.TZ0.Hsp_3b.i:
State the distribution of your test statistic, including the parameter.
-
18N.3.AHL.TZ0.Hsp_3b.ii:
Find the p-value for the test.
-
19M.3.AHL.TZ0.Hsp_2c:
At the start of the day, one employee, Amanda, has a queue of four customers. A second employee, Brian, has a queue of three customers. You may assume they work independently.
Find the probability that Amanda’s queue will be dealt with before Brian’s queue.
-
19M.3.AHL.TZ0.Hsp_3e.ii:
Write down the conclusion reached.
-
18M.3.AHL.TZ0.Hsp_1b:
Find the probability that the weight of a randomly chosen male bird is more than twice the weight of a randomly chosen female bird.
-
17N.3.AHL.TZ0.Hsp_2b.i:
Use a two-tailed test to determine the p-value for the above results.
-
18N.3.AHL.TZ0.Hsp_2a.i:
Find unbiased estimates of the mean of T.
-
16N.3.AHL.TZ0.Hsp_2d:
Calculate the probability that he makes a Type II error.
-
17M.3.AHL.TZ0.Hsp_1c.i:
Carry out an appropriate test and state the p-value obtained.
-
EXM.2.SL.TZ0.6a:
Use a chi-squared goodness of fit test to investigate whether or not, at the 5 % level of significance, the N(0, 1) distribution can be used to model these results.
-
17M.1.SL.TZ1.S_6a.i:
Write down the gradient of the curve of f at P.
-
17M.1.SL.TZ1.S_9b:
Find the value of a.
-
19N.3.AHL.TZ0.Hsp_1a.i:
State suitable hypotheses H0 and H1 to test Peter’s claim, using a two-tailed test.
-
19N.1.SL.TZ0.T_12c:
It is known that 20% of the runners took more than 28 minutes and less than k minutes to complete the race.
Find the value of k.
-
19N.1.SL.TZ0.T_6c:
Explain why it is valid to use the regression equation to estimate the airfare between Hong Kong and Tokyo.
-
19N.2.SL.TZ0.T_1b:
Write down the number of degrees of freedom.
-
19N.2.SL.TZ0.T_1f.i:
Calculate the probability that the customer is an adult.
-
17M.1.AHL.TZ2.H_7a:
The random variable X has the Poisson distribution Po(m). Given that P(X>0)=34, find the value of m in the form lna where a is an integer.
-
21M.2.SL.TZ2.1e:
Draw a Venn diagram to illustrate this information, placing all relevant information on the diagram.
-
21M.2.SL.TZ2.1c.i:
will not have the disease and will test positive.
-
21M.2.AHL.TZ2.2e:
A cat is selected at random from all 160 cats.
Find the probability that the cat was female, given that its weight was over 4.7 kg.
-
21M.2.SL.TZ2.4e:
Ten of the cats are chosen at random. Find the probability that exactly one of them weighs over 6.25 kg.
-
21M.3.AHL.TZ1.2b:
Show that 11 employees are selected for the sample from the national department.
-
21M.3.AHL.TZ2.1a.i:
Describe one way in which Juliet could improve the reliability of her investigation.
-
21M.3.AHL.TZ2.1b:
Juliet classifies response K as an outlier and removes it from the data. Suggest one possible justification for her decision to remove it.
-
21M.3.AHL.TZ2.1f.ii:
State the null and alternative hypotheses for this test.
-
20N.2.AHL.TZ0.H_2:
Jenna is a keen book reader. The number of books she reads during one week can be modelled by a Poisson distribution with mean 2.6.
Determine the expected number of weeks in one year, of 52 weeks, during which Jenna reads at least four books.
-
20N.2.AHL.TZ0.H_9a:
Find the probability that a randomly selected packet has a weight less than 100 g.
-
20N.1.SL.TZ0.S_1a.ii:
Find the value of v.
-
20N.2.SL.TZ0.S_2b:
Using the regression equation, estimate the number of hot chocolates that Lucy will sell on a day when the maximum temperature is 12°C.
-
20N.1.SL.TZ0.T_3a:
Write down the modal group for these data.
-
20N.1.SL.TZ0.T_6a.ii:
Find the probability that the first ball chosen is labelled A or labelled N.
-
20N.1.SL.TZ0.T_6c:
Find the probability that both balls chosen are labelled N.
-
20N.1.SL.TZ0.T_7c:
Write down a second equation in p and q.
-
20N.2.SL.TZ0.T_1e.iii:
Hence or otherwise estimate the charging time when the wind speed is 27 km h-1.
-
20N.2.SL.TZ0.T_2d.i:
Find the probability that a randomly selected student visited the rollercoasters.
-
20N.2.SL.TZ0.T_6a:
Sketch a diagram to represent this information.
-
20N.2.SL.TZ0.T_6c.ii:
Find the probability that Emlyn plays more than 20 minutes in a game.
-
EXN.1.SL.TZ0.10c:
Find the probability it will not rain while Paula is outside.
-
EXN.1.AHL.TZ0.5a:
Find the transition matrix for the maze.
-
EXN.1.AHL.TZ0.5b:
A scientist sets up the robot and then leaves it moving around the maze for a long period of time.
Find the probability that the robot is in room B when the scientist returns.
-
EXN.1.AHL.TZ0.12c.ii:
Find an expression for P in terms of d.
-
EXN.1.AHL.TZ0.16b:
Find the probability there will be space for him on the 9.01 car.
-
EXN.2.AHL.TZ0.4e:
Find the sum of the square residuals for Jorge’s model using the values t=1, 2, 3, 4.
-
EXN.2.AHL.TZ0.6b.ii:
have a total mass greater than 6.0 kg.
-
EXN.3.AHL.TZ0.1c.ii:
State an assumption that is being made for X to be considered as following a binomial distribution.
-
EXN.3.AHL.TZ0.1d.i:
Show that an estimate for Var(X) is 38.25.
-
21M.1.SL.TZ1.2b:
the mean.
-
21M.1.SL.TZ1.10c:
Find the expected score of a game.
-
21M.1.SL.TZ2.5a.i:
the minimum number of sick days taken during the year.
-
21M.1.SL.TZ2.5a.ii:
the lower quartile.
-
21M.1.AHL.TZ2.13a:
Determine the transition matrix for this graph.
-
21M.2.SL.TZ1.1b:
Write down the median time to read the book.
-
21N.1.AHL.TZ0.9a:
Complete the following transition diagram to represent this information.
-
21N.1.AHL.TZ0.9b:
Katie works for 180 days in a year.
Find the probability that Katie cycles to work on her final working day of the year.
-
21N.2.SL.TZ0.1b:
State the null and alternative hypotheses.
-
21N.2.SL.TZ0.1d.i:
Write down the χ2 test statistic.
-
21N.2.SL.TZ0.3e.ii:
Find the time, in seconds, that point C is above a height of 100 m, during each complete rotation.
-
21N.2.SL.TZ0.3c.i:
Write down the amplitude of the function.
-
21N.2.AHL.TZ0.7b.ii:
Perform the test, clearly stating the conclusion in context.
-
21N.3.AHL.TZ0.2g.i:
Show that dHdt≈0.2514-0.009873t-0.1405√H, where 0≤t≤T.
-
19M.1.SL.TZ2.T_5b:
Find the number of surveyed students who did not like any of the three flavours.
-
22M.1.SL.TZ1.8a:
Write down the percentage of bags that weigh more than 500 g.
-
22M.1.AHL.TZ1.15b:
Find the probability of a Type I error.
-
22M.1.SL.TZ2.2c:
Two different applicants are chosen at random from the original group.
Find the probability that both applicants applied to the Arts programme.
-
22M.1.SL.TZ2.8b:
Calculate the p-value for this test.
-
22M.1.AHL.TZ2.9a:
Find an unbiased estimate of the population mean of d.
-
22M.1.AHL.TZ2.9c.ii:
Given that all assumptions for this test are satisfied, carry out an appropriate hypothesis test. State and justify your conclusion. Use a 5% significance level.
-
22M.1.AHL.TZ2.12c:
Find the probability of making an error using the zoologist’s method.
-
22M.1.AHL.TZ2.12b:
Find the probability of making a Type II error when weighing a female cuttlefish.
-
22M.2.SL.TZ1.5b.i:
Write down the expected number of passengers who will arrive to board the flight if 72 tickets are sold.
-
22M.2.AHL.TZ1.3a:
Name the type of sampling that best describes the method used by the Principal.
-
SPM.1.SL.TZ0.12b:
Calculate the expected score.
-
SPM.1.AHL.TZ0.9b:
Find a 95 % confidence interval for the population mean, giving your answer to 4 significant figures.
-
SPM.1.AHL.TZ0.10:
In a coffee shop, the time it takes to serve a customer can be modelled by a normal distribution with a mean of 1.5 minutes and a standard deviation of 0.4 minutes.
Two customers enter the shop together. They are served one at a time.
Find the probability that the total time taken to serve both customers will be less than 4 minutes.
Clearly state any assumptions you have made.
-
SPM.1.AHL.TZ0.16c:
The average number of fish caught in an hour is actually 2.5.
Find the probability of a Type II error.
-
SPM.3.AHL.TZ0.1e.i:
Use an appropriate test to determine whether there is evidence, at the 5 % significance level, that the students in school B have improved more than those in school A.
-
SPM.2.SL.TZ0.3c.ii:
State whether this estimate is reliable. Justify your answer.
-
SPM.3.AHL.TZ0.1g:
Use an appropriate test to determine whether showing an improvement is independent of gender.
-
EXM.2.AHL.TZ0.12b.ii:
Write down the coefficient of determination.
-
EXM.1.AHL.TZ0.15b:
Find the equation of the regression line of ln(T−25) on t.
-
EXM.1.AHL.TZ0.17a:
Write down the transition matrix for this Markov chain.
-
EXM.3.AHL.TZ0.4e:
Find v1,v2,v3,v4.
-
EXM.1.AHL.TZ0.18b:
Find the steady state probability vector for M in terms of a and b.
-
EXM.1.SL.TZ0.7b:
State the hypotheses for this t-test.
-
EXM.1.AHL.TZ0.20a:
State the name of this test for validity.
-
EXM.3.AHL.TZ0.8g.i:
Find the probability of making a Type I error.
-
22M.3.AHL.TZ2.1e.ii:
Hence state the probability of a Type I error for this test.
-
EXM.1.AHL.TZ0.59a:
Calculate unbiased estimates of the population mean and the population variance.
-
EXM.1.SL.TZ0.9:
Six coins are tossed simultaneously 320 times, with the following results.
At the 5% level of significance, test the hypothesis that all the coins are fair.
-
EXM.1.AHL.TZ0.56b.ii:
Carry out a test at the 1% significance level, and state your conclusion.
-
EXM.2.AHL.TZ0.29a:
A horse breeder records the number of births for each of 100 horses during the past eight years. The results are summarized in the following table:
Stating null and alternative hypotheses carry out an appropriate test at the 5% significance level to decide whether the results can be modelled by B (6, 0.5).
-
EXM.2.AHL.TZ0.28a.i:
State suitable hypotheses for testing this belief.
-
EXM.2.AHL.TZ0.29b:
Without doing any further calculations, explain briefly how you would carry out a test, at the 5% significance level, to decide if the data can be modelled by B(6, p), where p is unspecified.
-
18M.1.SL.TZ2.T_9a.ii:
Write down an expression, in set notation, for the shaded region represented by Diagram 2.
-
18M.1.SL.TZ2.T_9b.ii:
Shade, on the Venn diagram, the region represented by the set J∩K.
-
18M.2.SL.TZ1.T_5a:
Write down the probability that Ayako avoids the trap in this wall.
-
18M.2.SL.TZ2.T_1c.i:
Find the value of x.
-
18M.2.SL.TZ2.T_1e:
Find n((C∪B)∩P′).
-
17N.1.SL.TZ0.S_1b:
Find the probability that exactly one of the selected balls is green.
-
17M.1.SL.TZ2.T_2d:
Write down the value of n(F).
-
19M.2.SL.TZ1.T_1c:
Plot and label the point M(ˉx, ˉy) on the scatter diagram.
-
19M.2.SL.TZ1.T_1d.i:
Use your graphic display calculator to find the Pearson’s product–moment correlation coefficient, r.
-
19M.2.SL.TZ1.T_3c.ii:
For these data, write down the lower quartile.
-
18N.1.SL.TZ0.T_4b:
Calculate the expected number of male engineers.
-
18N.1.SL.TZ0.T_4d:
Abhinav rejects H0.
State a reason why Abhinav is incorrect in doing so.
-
18M.1.SL.TZ1.T_4a.ii:
For these data, find the equation of the regression line y on x.
-
18M.2.SL.TZ1.T_2g:
Given that this flight was not heavily delayed, find the probability that it travelled between 500 km and 5000 km.
-
18M.2.SL.TZ2.S_1a.i:
Find the value of a and of b.
-
19M.1.SL.TZ2.T_12b:
On the same grid, complete the cumulative frequency curve for these data.
-
16N.2.SL.TZ0.S_8c:
During week 3 each student spent 5% less time browsing the Internet than during week 1. For week 3, find
(i) the median;
(ii) the variance.
-
16N.2.SL.TZ0.T_1h:
Write down a reason why this estimate is not reliable.
-
18M.2.SL.TZ2.T_3a.i:
Find the probability that a basketball player has a weight that is less than 61 kg.
-
18M.2.SL.TZ2.T_3b.i:
Sketch a normal curve to represent this probability.
-
17N.1.SL.TZ0.T_5b:
Draw the regression line on the scatter diagram.
-
17M.2.SL.TZ2.T_1g:
State the conclusion of this χ2 test. Give a reason for your answer.
-
18M.2.SL.TZ1.S_9c:
To the nearest gram, find the minimum weight of an orange that the grocer will buy.
-
18M.2.SL.TZ1.S_9e:
The grocer selects two boxes at random.
Find the probability that the grocer buys more than half the oranges in each box.
-
19M.2.SL.TZ1.S_10a.ii:
Find the probability of rolling two or more red faces.
-
19M.2.SL.TZ1.S_10b:
Show that, after a turn, the probability that Ted adds exactly $10 to his winnings is 13.
-
17M.1.AHL.TZ1.H_10a:
Find the value of k.
-
17M.1.AHL.TZ1.H_10c.ii:
Hence state the interquartile range of X.
-
17N.2.SL.TZ0.S_8c:
Write down the number of low production hives.
-
17N.2.SL.TZ0.T_1b.i:
Write down, for N, the modal class;
-
17M.1.SL.TZ2.T_7b:
Using the mean score, write down a second equation in terms of x and y.
-
17M.2.SL.TZ1.T_1a:
Find the range of the average body weights for these seven species of mammal.
-
17M.2.SL.TZ1.T_1b.ii:
For the data from these seven species describe the correlation between the average body weight and the average weight of the brain.
-
17M.2.SL.TZ2.S_2b:
Use the regression equation to estimate the number of visitors on a day when the maximum temperature is 15 °C.
-
18M.2.SL.TZ2.T_2d:
Write down the percentage of buses that travelled a distance greater than the upper quartile.
-
18M.2.AHL.TZ1.H_10b:
Find P(X<1).
-
17M.2.AHL.TZ2.H_10f:
Find P(Y⩾3).
-
17M.2.SL.TZ1.S_9c:
Find λ.
-
19M.2.AHL.TZ2.H_10c:
Hence find the expected number of times per day that Steffi is fed at Will’s house.
-
19M.2.AHL.TZ2.H_10e:
Show that the expected number of occasions per year on which Steffi visits Will’s house and is not fed is at least 30.
-
18M.2.SL.TZ2.S_10a.i:
Write down the value of k.
-
16N.1.AHL.TZ0.H_10b:
(i) show that P(A)=13;
(ii) hence find P(B).
-
19M.1.SL.TZ1.T_7a:
Place the numbers 2π,−5,3−1 and 232 in the correct position on the Venn diagram.
-
18N.1.SL.TZ0.S_10a:
Find the coordinates of P.
-
18N.1.SL.TZ0.S_10b.ii:
Hence, find the equation of L in terms of a.
-
18N.2.SL.TZ0.S_9d:
Ten of the participants with reaction times greater than 0.65 are selected at random. Find the probability that at least two of them are in Group X.
-
16N.2.SL.TZ0.T_6b:
Express this volume in cm3.
-
17M.2.SL.TZ1.S_4b.i:
Hence, find the probability that exactly k students are left handed;
-
18M.2.AHL.TZ2.H_3b:
Find the value of P(μ − 2σ < X < μ + σ).
-
17N.2.AHL.TZ0.H_2b:
Find P(A).
-
18M.1.AHL.TZ2.H_3b.ii:
Find P(X > μ).
-
17N.2.AHL.TZ0.H_6b:
Find the expected number of weeks in the year in which Lucca eats no bananas.
-
18M.3.AHL.TZ0.Hsp_3a:
State suitable hypotheses for a two-tailed test.
-
19M.2.AHL.TZ2.H_2a:
Calculate the probability that, on a randomly selected day, Timmy makes a profit.
-
18M.1.SL.TZ2.S_8b:
Find the probability that Pablo leaves home before 07:00 and is late for work.
-
18M.1.SL.TZ2.S_8c:
Find the probability that Pablo is late for work.
-
19M.2.AHL.TZ2.H_2b:
The shop is open for 24 days every month.
Calculate the probability that, in a randomly selected month, Timmy makes a profit on between 5 and 10 days (inclusive).
-
18M.2.SL.TZ2.S_10d.i:
Find the expected number of bags in this crate that contain at most one small apple.
-
17M.1.SL.TZ1.T_4b:
Determine whether rock-climbing is offered by the school in the fall/autumn trimester.
-
18M.2.AHL.TZ1.H_8:
Each of the 25 students in a class are asked how many pets they own. Two students own three pets and no students own more than three pets. The mean and standard deviation of the number of pets owned by students in the class are 1825 and 2425 respectively.
Find the number of students in the class who do not own a pet.
-
19M.3.AHL.TZ0.Hsp_2b:
Find the probability that the time taken by an employee to deal with a queue of three customers is less than nine minutes.
-
18N.3.AHL.TZ0.Hsp_2a.ii:
Find unbiased estimates of the variance of T.
-
18M.3.AHL.TZ0.Hsp_1c:
Two randomly chosen male birds and three randomly chosen female birds are placed on a weighing machine that has a weight limit of 18 kg. Find the probability that the total weight of these five birds is greater than the weight limit.
-
19M.1.SL.TZ2.S_9b:
Line L passes through the origin and has a gradient of tanθ. Find the equation of L.
-
19M.1.SL.TZ2.S_9c:
Find the derivative of f.
-
17M.2.AHL.TZ2.H_1b:
Calculate the mean score.
-
17M.3.AHL.TZ0.Hsp_1a:
State suitable hypotheses to test the inspector’s claim.
-
19N.3.AHL.TZ0.Hsp_3c.ii:
Write down the probability that Josie makes a Type I error.
-
17M.2.SL.TZ2.S_8b.ii:
Write down the rate of change of f at A.
-
19N.1.SL.TZ0.T_6a:
Use your graphic display calculator to find the equation of the regression line y on x.
-
19N.1.SL.TZ0.T_6b:
Use your regression equation to estimate the cost of a flight from Hong Kong to Tokyo with Galois Airways.
-
19N.1.SL.TZ0.T_9b:
Find the probability that Sungwon scores greater than 4 on both of her first two turns.
-
19N.2.SL.TZ0.T_1c:
Show that the expected number of children who chose shrimp is 31, correct to two significant figures.
-
19N.2.SL.TZ0.T_1e:
State the conclusion for this test. Give a reason for your answer.
-
17N.1.AHL.TZ0.H_10b.ii:
Determine the variance of X.
-
17M.2.AHL.TZ1.H_5b:
Find the probability that exactly seven rooms will have fewer than three faults in the carpet.
-
17M.2.AHL.TZ1.H_5a:
Find the probability that the carpet laid in the first room has fewer than three faults.
-
21M.2.SL.TZ2.4b:
Find the proportion of male Persian cats weighing between 5.5 kg and 6.5 kg.
-
21M.2.SL.TZ2.1b.ii:
b.
-
21M.2.SL.TZ2.1a:
State the sampling method being used.
-
21M.2.SL.TZ2.1b.iii:
c.
-
21M.2.AHL.TZ2.2c:
Determine the expected number of cats in this group that have a weight of less than 5.3 kg.
-
21M.2.AHL.TZ2.4e:
Hence, or otherwise, determine the expected ratio of the number of patients Doctor Black would have compared to Doctor Green in the long term.
-
21M.3.AHL.TZ1.2d.iii:
Hence comment on the reliability of the written assessment.
-
20N.1.AHL.TZ0.F_13a.iii:
State whether your p-value suggests that X and Y are independent.
-
20N.1.AHL.TZ0.F_13b:
Given a further value x=5.2 from the distribution of X, Y, predict the corresponding value of y. Give your answer to one decimal place.
-
20N.2.AHL.TZ0.H_5b:
From a random sample of n holidaymakers, the probability that at least one of them took a holiday in the Lake District in 2019 is greater than 0.999.
Determine the least possible value of n.
-
20N.2.AHL.TZ0.H_9d:
From a random sample of 500 packets, determine the number of packets that would be expected to have a weight lying within 1.5 standard deviations of the mean.
-
20N.3.AHL.TZ0.Hsp_3d:
Given that the weights of the broccoli actually follow a normal distribution with mean 392 grams and variance 80 grams2, find the probability of Anjali making a Type II error.
-
20N.2.SL.TZ0.S_2a.ii:
Write down the correlation coefficient.
-
20N.2.SL.TZ0.T_1f:
Don concluded from his investigation: “There is no causation between wind speed and the time to fully charge the robot”.
In the context of the question, briefly explain the meaning of “no causation”.
-
20N.2.SL.TZ0.T_2c:
Write down the value of n( R∩W) .
-
20N.2.SL.TZ0.T_6c.i:
Find the probability that Emlyn plays between 13 minutes and 18 minutes in a game.
-
EXN.1.AHL.TZ0.6b:
Find the probability it will not rain while Paula is outside.
-
EXN.1.SL.TZ0.10a:
Write down the probability it rains during Paula’s lunch break.
-
EXN.3.AHL.TZ0.1f:
Given this result, comment on the validity of the linear model used in part (a).
-
21M.1.SL.TZ1.2a:
the mode.
-
21M.1.SL.TZ2.5b:
Paul claims that this box and whisker diagram can be used to infer that the percentage of employees who took fewer than six sick days is smaller than the percentage of employees who took more than eleven sick days.
State whether Paul is correct. Justify your answer.
-
21M.2.SL.TZ1.1c:
Calculate the interquartile range.
-
21M.2.AHL.TZ1.5d:
Hence find the long-term percentage of sunny days in Vokram.
-
21N.1.AHL.TZ0.14b:
Find the lower quartile of the weights of the sacks of potatoes.
-
21N.2.SL.TZ0.1d.iii:
State the conclusion for the test in context. Give a reason for your answer.
-
21N.2.SL.TZ0.1c:
Write down the number of degrees of freedom.
-
21N.2.SL.TZ0.3e.i:
Find the height of C above the ground when t=2.
-
21N.2.SL.TZ0.3f.i:
At any given instant, find the probability that point C is visible from Tim’s window.
-
21N.2.SL.TZ0.5a.i:
a dart lands less than 13 cm from O.
-
21N.2.SL.TZ0.5c:
Find the probability that Arianne does not score a point on a turn of three darts.
-
21N.2.SL.TZ0.5b:
Find the probability that Arianne throws two consecutive darts that land more than 15 cm from O.
-
21N.2.AHL.TZ0.7a.i:
Write down null and alternative hypotheses for Loreto’s test.
-
21N.2.AHL.TZ0.3b:
Find the probability that Arianne throws two consecutive darts that land more than 15 cm from O.
-
21N.3.AHL.TZ0.2a.iii:
Suggest why Eva’s use of the linear regression equation in this way could be unreliable.
-
21N.3.AHL.TZ0.2b.iii:
Hence, write down a suitable domain for Eva’s function h(t)=pt2+qt+r.
-
21N.3.AHL.TZ0.2a.ii:
Interpret the meaning of parameter a in the context of the model.
-
21N.3.AHL.TZ0.2d:
By solving the differential equation dhdt=-R2√70 560h, show that the general solution is given by h=17 640(c-R2t)2, where c∈ℝ.
-
21N.3.AHL.TZ0.2c:
Show that dhdt=-R2√70 560h.
-
22M.1.SL.TZ1.7b:
Write down the degrees of freedom.
-
22M.1.SL.TZ1.10b:
Find the probability that Karl takes two socks of the same colour.
-
22M.1.AHL.TZ1.9a:
Find the probability that the company fails the inspection.
-
22M.1.AHL.TZ1.11b:
Using your answer to (a), or otherwise, find the long-term probability of the switch being in state A. Give your answer in the form cd, where c, d∈ℤ+.
-
22M.1.SL.TZ2.5c:
Determine the probability that fewer than 7 people will pass this polygraph test.
-
22M.1.SL.TZ2.8c.i:
State the conclusion of the test. Justify your answer.
-
22M.1.SL.TZ2.5a:
Calculate the expected number of people who will pass this polygraph test.
-
22M.1.SL.TZ2.5b:
Calculate the probability that exactly 4 people will fail this polygraph test.
-
22M.1.AHL.TZ2.9b:
Find an unbiased estimate of the population variance of d.
-
22M.2.SL.TZ1.3f:
When calculating the ranks, Chester incorrectly read the Netherlands’ score as 478. Explain why the value of the Spearman’s rank correlation rs does not change despite this error.
-
22M.2.AHL.TZ1.3b.i:
the mean mark.
-
22M.2.AHL.TZ1.3c:
Perform an appropriate test at the 5% significance level to see if the mean marks achieved by the students in the school are higher than the national standard. It can be assumed that the marks come from a normal population.
-
SPM.1.SL.TZ0.12c:
Jae Hee plays the game twice and adds the two scores together.
Find the probability Jae Hee has a total score of −3.
-
22M.2.SL.TZ2.1b:
Find the estimated number of teenagers who have a reaction time greater than 0.4 seconds.
-
SPM.2.SL.TZ0.3a.ii:
Using the value of r, interpret the relationship between Stan’s score and Minsun’s score.
-
SPM.3.AHL.TZ0.1b:
State why comparing only the final IB points of the students from the two schools would not be a valid test for the effectiveness of the two different teaching methods.
-
SPM.3.AHL.TZ0.1d:
Use a paired t-test to determine whether there is significant evidence that the students in school A have improved their IB points since the start of the course.
-
22M.3.AHL.TZ2.1a.i:
Find the mean and variance for the sample data given in the table.
-
EXM.3.AHL.TZ0.4f:
Hence, deduce the form of vn.
-
EXM.1.SL.TZ0.7d:
State the conclusion of this test, in context, giving a reason.
-
22M.2.AHL.TZ2.4e.ii:
A.
It is not required to state units for this value.
-
EXM.1.AHL.TZ0.17c:
Find the steady state probability vector for this Markov chain.
-
EXM.3.AHL.TZ0.4a.i:
Draw a transition state diagram for this Markov chain problem.
-
EXM.1.AHL.TZ0.21b:
Find the equation of the least squares exponential regression curve for 28−T.
-
EXM.1.SL.TZ0.7a:
State an assumption that Anita is making, in order to use a t-test.
-
EXM.3.AHL.TZ0.7b.ii:
Write down the coefficient of determination.
-
22M.3.AHL.TZ1.1b.iv:
Hence comment on the suitability of the model from (b)(ii) in comparison with the linear model found in part (a).
-
EXM.2.AHL.TZ0.24a:
Show that this data leads to an estimated value of p=0.4.
-
EXM.1.SL.TZ0.8a:
Copy and complete the table, showing how you arrived at your answers.
-
EXM.1.AHL.TZ0.55a.ii:
Hence estimate p, the probability that a randomly chosen egg is brown.
-
EXM.1.AHL.TZ0.58a:
Find the exact value of the mean of this distribution.
-
EXM.2.AHL.TZ0.27c:
Most of the expected frequencies have been calculated in the third column. (Frequencies have been rounded to the nearest integer, and frequencies in the first and last classes have been extended to include the rest of the data beyond 15 and 225. Find the values of a, b and c and show how you arrived at your answers.
-
EXM.2.AHL.TZ0.27a.ii:
the level of significance of a hypothesis test.
-
EXM.2.AHL.TZ0.28a.ii:
Calculate the mean of these data and hence estimate the value of p.
-
18M.1.SL.TZ2.T_9a.i:
Write down an expression, in set notation, for the shaded region represented by Diagram 1.
-
18M.2.SL.TZ2.T_1a.ii:
Write down the value of b.
-
18M.2.SL.TZ2.T_1b.i:
Use the tree diagram to find the probability that an employee encountered traffic and was late for work.
-
18M.2.SL.TZ2.T_1b.ii:
Use the tree diagram to find the probability that an employee was late for work.
-
18M.2.SL.TZ2.T_1b.iii:
Use the tree diagram to find the probability that an employee encountered traffic given that they were late for work.
-
18M.2.SL.TZ2.T_1d:
Find the number of employees who, in the last year, did not travel to work by car, bicycle or public transportation.
-
16N.1.SL.TZ0.T_3a:
In the table indicate whether the given statements are True or False.
-
19M.2.SL.TZ1.T_3a:
Write down the total number of people, from this group, who are pet owners.
-
19M.2.SL.TZ2.S_1a.i:
Write down the value of a and of b.
-
18N.2.SL.TZ0.T_1a.ii:
Use your graphic display calculator to write down ˉy, the mean examination score.
-
18M.1.SL.TZ1.T_4a.i:
For these data, find Pearson’s product-moment correlation coefficient, r.
-
18M.1.SL.TZ2.T_1b:
Draw the line of best fit, by eye, on the scatter diagram.
-
18M.1.SL.TZ2.T_1c:
Using your line of best fit, estimate the physics test score for a student with a score of 20 in their mathematics test.
-
18M.2.SL.TZ1.T_2a:
State the alternative hypothesis.
-
18M.2.SL.TZ2.T_2b.i:
Use the cumulative frequency curve to find the median distance.
-
16N.2.AHL.TZ0.H_1b:
Find the value of Var(X).
-
16N.2.SL.TZ0.T_1g:
Use the given equation of the regression line to estimate the number of IB Diploma points that this girl obtained.
-
18M.2.SL.TZ2.T_3e:
State a conclusion for this test. Justify your answer.
-
17M.2.SL.TZ2.T_1f:
Use your graphic display calculator to find the χ2 statistic for this data.
-
17M.2.SL.TZ2.T_3a:
Draw a scatter diagram for this data. Use a scale of 2 cm for 5000 folders on the horizontal axis and 2 cm for 10 000 Euros on the vertical axis.
-
17M.2.SL.TZ2.T_3b.ii:
Write down, for this set of data the mean production cost, ˉC.
-
17M.2.SL.TZ2.T_3c:
Label the point M(ˉx, ˉC) on the scatter diagram.
-
16N.1.SL.TZ0.T_6c:
State, giving a reason, whether the null hypothesis should be rejected.
-
19M.1.AHL.TZ1.H_6a:
P(X>μ+5).
-
19M.2.SL.TZ2.S_9b.i:
Find P(A∩D′).
-
18M.2.SL.TZ1.S_9d:
Find the probability that the grocer buys more than half the oranges in a box selected at random.
-
18M.2.AHL.TZ2.H_8a:
Find the least possible value of n.
-
19M.2.SL.TZ1.S_10c.i:
Write down the value of x.
-
17M.1.AHL.TZ1.H_10b.i:
By considering the graph of f write down the mean of X;
-
17N.2.SL.TZ0.S_8b:
Use this regression line to estimate the monthly honey production from a hive that has 270 bees.
-
17N.2.SL.TZ0.S_8e:
Adam decides to increase the number of bees in each low production hive. Research suggests that there is a probability of 0.75 that a low production hive becomes a regular production hive. Calculate the probability that 30 low production hives become regular production hives.
-
17N.2.SL.TZ0.T_1a:
State whether N is a discrete or a continuous variable.
-
17N.2.SL.TZ0.T_1c.ii:
Use your graphic display calculator to estimate the standard deviation of N.
-
17M.1.SL.TZ1.S_4b:
Jim describes the correlation as very strong. Circle the value below which best represents the correlation coefficient.
0.9920.2510−0.251−0.992
-
17M.1.SL.TZ1.T_6a:
Write down the value of x.
-
17M.2.SL.TZ1.T_1c:
Write down the equation of the regression line y on x, in the form y=mx+c.
-
17M.2.SL.TZ1.T_1e:
Find the percentage error in your estimate in part (d).
-
17M.2.SL.TZ1.T_5c:
Find the interquartile range.
-
17M.2.SL.TZ1.T_5d:
Find the probability that this student scored a grade 5 or higher.
-
18M.2.AHL.TZ1.H_10c:
Given that P(s<X<0.8)=2×P(2s<X<0.8), and that 0.25 < s < 0.4 , find the value of s.
-
17N.2.SL.TZ0.S_4c:
Find P(X=2|X>0).
-
17M.2.SL.TZ2.S_10b.i:
Write down the probability of drawing three blue marbles.
-
17M.2.AHL.TZ2.H_10c:
Find Var(X).
-
19M.2.SL.TZ1.S_9d.i:
Find (f∘f)(x).
-
19M.2.AHL.TZ2.H_10d:
In any given year of 365 days, the probability that Steffi does not visit Will for at most n days in total is 0.5 (to one decimal place). Find the value of n.
-
18N.1.SL.TZ0.S_9b.ii:
fourth draw.
-
18M.2.SL.TZ1.S_2a:
Find the value of k.
-
18M.2.SL.TZ1.S_2c:
The die is rolled 80 times. On how many rolls would you expect to obtain a three?
-
19M.1.SL.TZ1.S_1a:
Find the value of p.
-
19M.1.SL.TZ1.S_1b:
Find the value of q.
-
18N.1.SL.TZ0.T_14b:
Find the probability that a randomly chosen student will be accepted by Marron College.
-
18N.1.SL.TZ0.T_14c:
Given that Naomi attends Marron College, find the probability that she achieved a mark of at least 500 on the test.
-
17M.1.SL.TZ2.S_3b:
Find P(100<X<107).
-
17M.1.SL.TZ2.T_11b:
Find the value of a.
-
17M.1.SL.TZ2.T_11c:
Estimate the interquartile range of the distribution.
-
19M.1.SL.TZ2.T_14a.ii:
Find the price that is two standard deviations above the mean price.
-
18N.2.SL.TZ0.T_2c.iii:
Find the probability that this student is taught in Spanish, given that the student studies Biology.
-
19M.2.SL.TZ1.S_3b:
The graph of f has a horizontal tangent line at x=0 and at x=a. Find a.
-
17M.1.SL.TZ1.S_1a.ii:
Find the value of q.
-
16N.1.AHL.TZ0.H_10a:
Show that P(A∪B)=P(A)+P(A′∩B).
-
16N.2.SL.TZ0.S_5a:
Given that 5.3% of newborn babies have a low birth weight, find w.
-
16N.2.SL.TZ0.T_4b:
(i) Find the probability that a box of cereal, chosen at random, is sold.
(ii) Calculate the manufacturer’s expected daily income from these sales.
-
19M.1.SL.TZ1.S_9b:
Given that z > −1.6, find the probability that z < 2.4 . Write your answer in terms of a and b.
-
16N.2.SL.TZ0.T_6d:
Show that A=πr2+1000000r.
-
16N.2.SL.TZ0.T_6f:
Using your answer to part (e), find the value of r which minimizes A.
-
16N.2.AHL.TZ0.H_3a:
Show that P(X=x+1)=μx+1×P(X=x), x∈N.
-
18M.1.SL.TZ1.T_13a.i:
Find the probability that a student, chosen at random arrives at least 60 minutes after the school opens.
-
18M.1.SL.TZ2.S_8e:
Two days next week Pablo will drive to work. Find the probability that he will be late at least once.
-
18M.2.SL.TZ2.S_10d.ii:
Find the probability that at least 48 bags in this crate contain at most one small apple.
-
17M.1.SL.TZ2.T_10a:
Write down the probability that a light bulb produced by Home Shine is not defective.
-
17M.1.SL.TZ2.T_10b.i:
Find the probability that both light bulbs are not defective.
-
17N.2.SL.TZ0.T_4f:
Find the probability that the tested adult is allergic to nuts given that the liquid turned blue.
-
18N.3.AHL.TZ0.Hsp_3c.ii:
Find the product-moment correlation coefficient r.
-
18N.3.AHL.TZ0.Hsp_3d:
Use an appropriate regression line to estimate the weight of a fish with length 360 mm.
-
19M.3.AHL.TZ0.Hsp_3b:
Find an unbiased estimate for μ.
-
19M.3.AHL.TZ0.Hsp_3e.i:
Find the p-value for the test.
-
16N.2.AHL.TZ0.H_11d:
(i) Hence show that X has two modes m1 and m2.
(ii) State the values of m1 and m2.
-
16N.2.AHL.TZ0.H_11e:
Determine the minimum value of x such that the probability Kati receives at least one free gift is greater than 0.5.
-
19N.3.AHL.TZ0.Hsp_3c.i:
Find the critical region for Josie’s test, giving your answer correct to two decimal places.
-
17M.1.SL.TZ1.S_9c:
The line y=kx−5 is a tangent to the curve of f. Find the values of k.
-
17M.2.SL.TZ2.S_8d:
Let R be the region enclosed by the graph of f , the x-axis, the line x=b and the line x=a. The region R is rotated 360° about the x-axis. Find the volume of the solid formed.
-
18M.1.SL.TZ1.S_7:
Consider f(x), g(x) and h(x), for x∈R where h(x) = (f∘g)(x).
Given that g(3) = 7 , g′ (3) = 4 and f ′ (7) = −5 , find the gradient of the normal to the curve of h at x = 3.
-
19N.1.SL.TZ0.T_12a:
Write down the probability that the runner completed the race in more than 28 minutes.
-
19N.1.SL.TZ0.T_4a:
Write down n(B).
-
19N.1.SL.TZ0.T_9a:
Find the probability that Sungwon’s score on her first turn is greater than 4.
-
17M.1.AHL.TZ2.H_7b:
The random variable Y has the Poisson distribution Po(2m). Find P(Y>1) in the form b−lncc where b and c are integers.
-
20N.1.AHL.TZ0.F_13a.ii:
Assuming that the distribution of X, Y is bivariate normal with product moment correlation coefficient ρ, calculate the p-value of your result when testing the hypotheses H0 : ρ=0 ; H1 : ρ>0.
-
20N.2.AHL.TZ0.H_9b:
The probability that a randomly selected packet has a weight greater than w grams is 0.444. Find the value of w.
-
20N.3.AHL.TZ0.Hsp_3a:
Assuming that the shopkeeper’s claim is correct, find the probability that the weight of six randomly chosen carrots is more than two times the weight of one randomly chosen broccoli.
-
20N.1.SL.TZ0.S_1a.i:
Find the value of t.
-
20N.1.SL.TZ0.S_8b:
Write down the value of the median distance in kilometres (km).
-
20N.2.SL.TZ0.S_3b.i:
Find the value of p which gives the largest value of E(X).
-
20N.2.SL.TZ0.S_9d:
Find the probability that Fiona will arrive on time.
-
20N.1.SL.TZ0.T_10b:
Find the expected number of short trips when it rained.
-
20N.2.SL.TZ0.T_1c:
Plot and label the point M on your scatter diagram.
-
20N.2.SL.TZ0.T_1d.i:
Calculate r, Pearson’s product–moment correlation coefficient.
-
EXN.1.SL.TZ0.3a:
Find the probability that an apple from the tree has a weight greater than 90 grams.
-
EXN.1.SL.TZ0.4c:
The weight of the smallest potato in the sample is 20 grams and the weight of the largest is 400 grams.
Use the scale shown below to draw a box and whisker diagram showing the distribution of the weights of the potatoes. You may assume there are no outliers.
-
EXN.1.AHL.TZ0.6a:
Write down the probability it rains during Paula’s lunch break.
-
EXN.2.SL.TZ0.4a:
Find the probability of scoring a six when rolling the novelty die.
-
EXN.2.AHL.TZ0.3b:
Find the value of a and the value of b, giving your answers correct to one decimal place.
-
EXN.2.AHL.TZ0.6b.i:
both have a mass greater than 3.0 kg.
-
21M.1.SL.TZ1.10b.ii:
a player scores 6, given that they scored at least 3.
-
21M.1.AHL.TZ1.1:
George goes fishing. From experience he knows that the mean number of fish he catches per hour is 1.1. It is assumed that the number of fish he catches can be modelled by a Poisson distribution.
On a day in which George spends 8 hours fishing, find the probability that he will catch more than 9 fish.
-
21M.1.AHL.TZ1.14b:
Find the standard deviation of the weights of these bags of apples.
-
21M.1.SL.TZ2.8b:
Find the p-value for this test.
-
21M.1.SL.TZ2.8c:
Write down the conclusion to the test. Give a reason for your answer.
-
21M.1.AHL.TZ2.9b.ii:
Write down the degrees of freedom for this test.
-
21M.1.AHL.TZ2.9a:
Find an estimate for how many copies the vendor expects to sell each day.
-
21M.1.AHL.TZ2.9b.iii:
Write down the conclusion to the test. Give a reason for your answer.
-
21M.1.AHL.TZ2.13b:
If the mouse was left to wander indefinitely, use your graphic display calculator to estimate the percentage of time that the mouse would spend at point F.
-
21M.1.AHL.TZ2.15c:
Sheila finds 126 coffees were sold during the 5-hour period.
State Sheila’s conclusion to the test. Justify your answer.
-
21M.2.SL.TZ1.1e:
Describe the correlation.
-
21M.2.SL.TZ1.4e:
State the conclusion of the test. Give a reason for your answer.
-
21N.1.SL.TZ0.1c:
Write down the equation of the regression line of t on x, in the form t=ax+b.
-
21N.2.SL.TZ0.1d.ii:
Write down the p-value.
-
21N.2.SL.TZ0.5d.ii:
Find the probability that Arianne scores at least 5 points and less than 8 points.
-
21N.2.SL.TZ0.5d.iii:
Given that Arianne scores at least 5 points, find the probability that Arianne scores less than 8 points.
-
21N.2.AHL.TZ0.3a.ii:
a dart lands more than 15 cm from O.
-
21N.3.AHL.TZ0.2a.i:
Find the equation of the regression line of h on t.
-
21N.3.AHL.TZ0.2b.ii:
Find the value of k.
-
19M.1.SL.TZ2.T_5c:
A student is chosen at random from the surveyed students.
Find the probability that this student likes kiwi fruit smoothies given that they like mango smoothies.
-
22M.1.AHL.TZ1.12d:
Find P(Y>100).
-
22M.1.SL.TZ2.2b:
Find the probability that the applicant applied for the Arts programme.
-
22M.1.SL.TZ2.2a:
Find the probability that a randomly chosen applicant from this group was accepted by the university.
-
22M.2.SL.TZ1.3b:
Determine if the Netherlands’ score is an outlier for this data. Justify your answer.
-
22M.2.SL.TZ1.3d.i:
a.
-
SPM.1.SL.TZ0.3a:
State whether the data is discrete or continuous.
-
22M.2.AHL.TZ1.3b.ii:
the standard deviation of the marks.
-
SPM.1.SL.TZ0.6c:
State, giving a reason, whether the null hypothesis should be accepted.
-
22M.2.SL.TZ2.1c:
Determine the 90th percentile of the reaction times from the cumulative frequency graph.
-
22M.2.SL.TZ2.1d.ii:
Write down the value of b.
-
22M.2.SL.TZ2.1g:
Suggest how, if at all, the estimated mean and estimated median reaction times will change if the errors are corrected. Justify your response.
-
SPM.1.SL.TZ0.6b:
Calculate the p-value for this test.
-
SPM.3.AHL.TZ0.1h:
If you were to repeat the test performed in part (e) intending to compare the quality of the teaching between the two schools, suggest two ways in which you might choose your sample to improve the validity of the test.
-
SPM.2.SL.TZ0.3a.i:
Write down the value of the Pearson’s product–moment correlation coefficient, r.
-
SPM.2.AHL.TZ0.6c:
Hence write down matrices P and D such that T = PDP−1.
-
SPM.2.AHL.TZ0.6d:
Find an expression for the number of customers company X has after n years, where n∈N.
-
SPM.3.AHL.TZ0.1f.ii:
Hence, find the expected improvement between predicted and final points for an increase of one unit in effort grades, giving your answer to one decimal place.
-
EXM.1.AHL.TZ0.15c.i:
find the value of a and of b.
-
EXM.1.SL.TZ0.3b:
Explain why it is appropriate to use Spearman’s rank correlation coefficient to measure the strength of the relationship between P and d.
-
EXM.1.AHL.TZ0.15a:
Show that ln(T−25)=bt+lna.
-
EXM.1.SL.TZ0.2a:
Calculate Spearman’s rank correlation coefficient for this data.
-
EXM.1.AHL.TZ0.15c.ii:
predict the temperature of the metal rod after 3 minutes.
-
EXM.1.AHL.TZ0.20c:
Hence determine, with a reason, if the new exam is a valid indicator of future performance.
-
EXM.3.AHL.TZ0.4c.i:
Find the steady state probability vector for this Markov chain problem.
-
EXM.3.AHL.TZ0.4d:
Explain why having a steady state probability vector means that the matrix M must have an eigenvalue of λ=1.
-
EXM.1.SL.TZ0.5a:
Recalling definitions, such as the Lower Quartile is the n+14th piece of data with the data placed in order, find an expression for the Interquartile Range.
-
EXM.1.AHL.TZ0.21a:
Explain why 28−T can be modeled by an exponential function.
-
EXM.3.AHL.TZ0.7a.i:
Use the trapezoidal rule to find an estimate for the area.
-
EXM.3.AHL.TZ0.7a.ii:
With reference to the shape of the graph, explain whether your answer to part (a)(i) will be an over-estimate or an underestimate of the area.
-
EXM.3.AHL.TZ0.9e:
Give two reasons why the prediction in part (b)(ii) might be lower than 14.
-
EXM.3.AHL.TZ0.8g.ii:
Given that p=0.2 find the probability of making a Type II error.
-
EXM.3.AHL.TZ0.7b.i:
Use all the coordinates in the table to find the equation of the least squares cubic regression curve.
-
EXM.3.AHL.TZ0.7c.i:
Write down an expression for the area enclosed by the cubic function, the x-axis, the y-axis and the line x=4.4.
-
EXM.3.AHL.TZ0.8e:
Perform a suitable test, at the 5% significance level, to determine if there is a difference between the mean scores of males and females. You should clearly state your hypotheses, the p-value and your conclusion.
-
EXM.3.AHL.TZ0.9a:
Use an exponential regression to find the value of a and of b, correct to 4 decimal places.
-
EXM.3.AHL.TZ0.9f.i:
L.
-
22M.3.AHL.TZ2.2h:
Show that Jonas’s network satisfies the requirement of there being less than a 2% probability of the network failing after a power surge.
-
22M.3.AHL.TZ2.1b:
State one assumption that Aimmika needs to make about the sales of bags of rice to support her belief that it follows a Poisson distribution.
-
EXM.2.AHL.TZ0.24b:
Stating null and alternative hypotheses, carry out an appropriate test at the 5 % level to decide whether the farmer’s claim can be justified.
-
EXM.1.AHL.TZ0.56a:
Calculate the mean number of eggs laid by these birds.
-
EXM.1.SL.TZ0.10b:
Explain what is meant by “level of significance” in part (a).
-
EXM.1.AHL.TZ0.58b:
Test, at the 5% level of significance, whether or not the data can be modelled by a Poisson distribution.
-
EXM.2.AHL.TZ0.26a:
The scientists wish to investigate the claim that Group B gain weight faster than Group A. Test this claim at the 5% level of significance, noting which hypothesis test you are using. You may assume that the weight gain for each group is normally distributed, with the same variance, and independent from each other.
-
EXM.2.AHL.TZ0.28a.iii:
Calculate an appropriate value of χ2 and state your conclusion, using a 1% significance level.
-
18M.1.SL.TZ2.T_9b.i:
Shade, on the Venn diagram, the region represented by the set (H∪I)′.
-
18M.2.SL.TZ1.T_5d.ii:
A contestant is chosen at random. Find the probability that this contestant fell into a trap.
-
17M.2.AHL.TZ1.H_1b:
Find P(A′∩B).
-
17M.2.AHL.TZ2.H_5a:
Draw a tree diagram to represent this information for the first three days of July.
-
17M.2.AHL.TZ2.H_5b:
Find the probability that the 3rd July is calm.
-
16N.1.SL.TZ0.S_5b:
Find P(A∪B).
-
16N.1.SL.TZ0.T_3b:
On the Venn diagram, shade the region A∩(B∪C)′.
-
17M.1.SL.TZ2.T_2b:
Complete the Venn diagram using the above information.
-
19M.1.SL.TZ2.T_6c:
The health inspector visits two school cafeterias. She inspects the same number of meals at each cafeteria. The data is shown in the following box-and-whisker diagrams.
Meals prepared in the school cafeterias are required to have less than 10 grams of sugar.
State, giving a reason, which school cafeteria has more meals that do not meet the requirement.
-
19M.2.SL.TZ1.T_3c.i:
For these data, write down the median number of pets.
-
19M.2.SL.TZ2.S_1b:
Use the regression equation to estimate the BMI of an adult man whose waist size is 95 cm.
-
18N.1.SL.TZ0.T_4c:
Find the p-value for this test.
-
18M.1.SL.TZ1.T_6c.i:
Write down the mid-interval value for the 100 < x ≤ 150 group.
-
16N.2.SL.TZ0.T_2d:
Find the probability that this person
(i) went on at most one trip;
(ii) went on the coach trip, given that this person also went on both the helicopter trip and the boat trip.
-
18M.1.SL.TZ2.T_12b.i:
Write down the total number of customers in terms of k.
-
18M.2.SL.TZ2.T_2a:
Find the number of buses that travelled a distance between 15000 and 20000 kilometres.
-
18M.2.SL.TZ1.S_8b:
Use the regression equation to estimate the value of y when x = 3.57.
-
18M.2.SL.TZ1.S_8c:
The relationship between x and y can be modelled using the formula y = kxn, where k ≠ 0 , n ≠ 0 , n ≠ 1.
By expressing ln y in terms of ln x, find the value of n and of k.
-
16N.2.SL.TZ0.S_8a:
Find the mean number of hours spent browsing the Internet.
-
18M.2.SL.TZ2.T_3a.ii:
In a training session there are 40 basketball players.
Find the expected number of players with a weight less than 61 kg in this training session.
-
18M.2.SL.TZ2.T_3c:
Given that P(W > k) = 0.225 , find the value of k.
-
17N.1.SL.TZ0.T_1a.ii:
For the students in this group write down the median age.
-
17N.1.SL.TZ0.T_5a:
Find the mean number of hours that the people surveyed watch television per week.
-
17M.2.SL.TZ2.T_1a.iii:
If the teacher chooses a response at random, find the probability that it is a satisfactory response, given that it is a response to the Calculus question.
-
17M.2.SL.TZ2.T_3h:
Use the equation of the regression line to estimate the least number of folders that the factory needs to sell in a month to exceed its production cost for that month.
-
18M.2.SL.TZ1.S_9a:
Find the probability that an orange weighs between 289 g and 310 g.
-
18M.2.SL.TZ1.S_9b.ii:
Hence, find the value of σ.
-
17M.1.AHL.TZ1.H_10b.iii:
By considering the graph of f write down the mode of X.
-
17M.1.AHL.TZ1.H_10d:
Calculate P(X⩽4|X⩾3).
-
17N.2.SL.TZ0.S_8d.i:
Find the value of k;
-
17M.1.SL.TZ1.T_6c:
Use your graphic display calculator to find the χ2 statistic for this test.
-
17M.2.SL.TZ1.T_1b.i:
For the data from these seven species calculate r, the Pearson’s product–moment correlation coefficient;
-
17M.2.SL.TZ1.T_5a.i:
Calculate the mean test grade of the students;
-
18M.2.SL.TZ2.T_2f:
Find the value of m.
-
18M.2.AHL.TZ1.H_10a:
Show that a=23.
-
17M.2.SL.TZ2.S_10d:
Grant plays the game until he wins two prizes. Find the probability that he wins his second prize on his eighth attempt.
-
19M.2.SL.TZ1.S_9b:
Find u.
-
19M.2.SL.TZ1.S_9d.ii:
Hence, write down f−1(x).
-
16N.2.SL.TZ0.S_10a:
(i) Find the value of c.
(ii) Show that b=π6.
(iii) Find the value of a.
-
19M.2.AHL.TZ1.H_9b.ii:
Find the probability that more than 100 cakes will be sold on a typical day.
-
19M.2.AHL.TZ2.H_10a:
Find the probability that on a randomly selected day, Steffi does not visit Will’s house.
-
19M.1.SL.TZ1.T_12a:
Find the probability that both spins are yellow.
-
17N.1.SL.TZ0.T_13a:
Find the probability that this applicant took at least 40 minutes to complete the test.
-
17M.1.SL.TZ2.T_11a:
Write down the probability that the mass of one of these corncobs is greater than 400 grams.
-
19M.1.SL.TZ2.T_14b:
Find the probability that the price of a kilogram of tomatoes, chosen at random, will be between 2.00 and 3.00 euro.
-
19M.1.SL.TZ2.T_14c:
To stimulate reasonable pricing, the city offers a free permit to the sellers whose price of a kilogram of tomatoes is in the lowest 20 %.
Find the highest price that a seller can charge and still receive a free permit.
-
18N.2.SL.TZ0.T_2a.ii:
Find the number of students in the school that study Mathematics in English.
-
19M.2.SL.TZ1.S_3a:
Find f′(x).
-
19M.1.SL.TZ2.T_11a:
Write down the elements that belong to A∩B.
-
16N.2.SL.TZ0.S_5b:
A newborn baby has a low birth weight.
Find the probability that the baby weighs at least 2.15 kg.
-
18N.2.AHL.TZ0.H_10a.ii:
Using this distribution model, find the standard deviation of X.
-
16N.2.SL.TZ0.T_6a:
Write down a formula for A, the surface area to be coated.
-
16N.2.SL.TZ0.T_6e:
Find dAdr.
-
17N.2.AHL.TZ0.H_2c:
Hence show that events A′ and B are independent.
-
18M.2.AHL.TZ1.H_4a:
Find the probability that a wolf selected at random is at least 5 years old.
-
18M.2.AHL.TZ1.H_4b:
Eight wolves are independently selected at random and their ages recorded.
Find the probability that more than six of these wolves are at least 5 years old.
-
19M.1.AHL.TZ1.H_10a.ii:
Find the remainder when p(x) is divided by (x−3).
-
18M.2.AHL.TZ2.H_9a.iii:
Given that more than 5 taxis arrive during T, find the probability that exactly 7 taxis arrive during T.
-
19M.2.AHL.TZ2.H_3a:
Complete the given probability tree diagram for Iqbal’s three attempts, labelling each branch with the correct probability.
-
18M.3.AHL.TZ0.Hsp_3c:
Find the probability of making a Type II error.
-
18M.2.SL.TZ2.S_10b:
Find P(M < 95) .
-
17M.2.AHL.TZ1.H_9b:
Calculate T1.
-
17M.2.AHL.TZ2.H_3c:
Calculate the new value of σ.
-
17M.1.SL.TZ1.T_4d:
Write down, in terms of F, W and S, an expression for the set which contains only archery, baseball, kayaking and surfing.
-
17N.2.SL.TZ0.T_4a:
Find the probability that this person is not allergic to nuts.
-
18N.3.AHL.TZ0.Hsp_3a.ii:
The maximum weight a hand net can hold is 6 kg. Find the probability that a catch of 11 fish can be carried in the hand net.
-
18N.3.AHL.TZ0.Hsp_3b.iii:
State the conclusion of the test, justifying your answer.
-
18N.3.AHL.TZ0.Hsp_3c.iii:
State the p-value and interpret it in this context.
-
16N.1.AHL.TZ0.H_1:
Find the coordinates of the point of intersection of the planes defined by the equations x+y+z=3, x−y+z=5 and x+y+2z=6.
-
16N.3.AHL.TZ0.Hsp_2a:
Find
(i) E(X);
(ii) Var(X).
-
16N.2.AHL.TZ0.H_11a:
Show that P(X=3)=0.001 and P(X=4)=0.0027.
-
EXM.2.SL.TZ0.6b.i:
a Type I error.
-
17M.2.SL.TZ2.S_8a:
Find the value of p.
-
19N.1.SL.TZ0.T_12b:
Calculate the probability that the runner completed the race in less than 26 minutes.
-
19N.2.SL.TZ0.T_1d.i:
the χ2 statistic.
-
19N.2.SL.TZ0.T_1d.ii:
the p-value.
-
21M.2.AHL.TZ2.4d:
Hence, show that the long-term transition matrix T∞ is given by T∞=(10171017717717).
-
21M.2.AHL.TZ2.5b.i:
on exactly 12 occasions.
-
21M.3.AHL.TZ2.1d.ii:
State the null and alternative hypotheses for this test.
-
21M.3.AHL.TZ2.1e.ii:
Interpret, referring to income and happiness, what the value of a represents.
-
20N.3.AHL.TZ0.Hsp_3c:
Find the significance level for this test.
-
20N.1.SL.TZ0.S_8d:
Find m.
-
20N.2.SL.TZ0.S_3b.ii:
Hence, find the largest value of E(X).
-
20N.1.SL.TZ0.T_14a:
Complete the values in the tree diagram.
-
20N.2.SL.TZ0.T_1b.i:
Calculate , the mean wind speed.
-
20N.2.SL.TZ0.T_1e.i:
Write down the equation of the regression line on , in the form .
-
20N.2.SL.TZ0.T_1e.ii:
Draw this regression line on your scatter diagram.
-
20N.2.SL.TZ0.T_2d.ii:
Find the probability that a randomly selected student visited the virtual reality rides.
-
20N.2.SL.TZ0.T_6e:
Find the probability he plays between and in one game and more than in the other game.
-
EXN.1.SL.TZ0.4b.ii:
Find the lower quartile.
-
EXN.1.SL.TZ0.4b.iii:
Find the upper quartile.
-
EXN.1.SL.TZ0.12a:
Write down the value of .
-
EXN.1.AHL.TZ0.6d:
Given it rains at least once while Paula is outside find the probability that it rains during her lunch hour.
-
EXN.2.SL.TZ0.4b:
Find the probability of scoring more than sixes when this die is rolled times.
-
EXN.2.SL.TZ0.4c.iii:
State the degrees of freedom for the test.
-
EXN.2.AHL.TZ0.3c:
Find the value of the test statistic for this test.
-
EXN.2.AHL.TZ0.3e:
It is given that the critical value for this test is .
State the conclusion of the test in context. Use your answer to part (c) to justify your conclusion.
-
EXN.3.AHL.TZ0.1d.ii:
Hence show that the variance of the proportion of marked fish in the sample, , is .
-
EXN.3.AHL.TZ0.1e.i:
Taking the value for the variance given in (d) (ii) as a good approximation for the true variance, find the upper and lower bounds for the proportion of marked fish in the lake.
-
EXN.2.AHL.TZ0.6a:
Find the probability that a melon selected at random will have a mass greater than .
-
EXN.3.AHL.TZ0.1c.i:
Write down the value of and the value of .
-
21M.1.SL.TZ1.10a:
Complete the table to show the probability distribution of .
-
21M.1.AHL.TZ1.11a:
Identify the type of sampling used by the restaurant manager.
-
21M.1.AHL.TZ1.11c:
Find the -value for the test.
-
21M.1.SL.TZ2.5a.iii:
the median.
-
21M.1.AHL.TZ2.15b:
Find the probability that Sheila will make a type I error in her test conclusion.
-
21M.2.SL.TZ1.1f:
Find the percentage score calculated by Jason.
-
21M.2.SL.TZ1.4b.ii:
and .
-
21M.2.AHL.TZ1.4c:
Find the equation of the new model.
-
21N.1.SL.TZ0.9a:
Find the median of the scores obtained.
-
21N.2.SL.TZ0.1a.iii:
prefers a laptop given that they are years old.
-
21N.2.SL.TZ0.1a.i:
prefers a tablet.
-
21N.2.SL.TZ0.3c.ii:
Find the period of the function.
-
21N.2.SL.TZ0.3a.ii:
minimum value of .
-
21N.2.SL.TZ0.3f.ii:
The wind speed increases. The blades rotate at twice the speed, but still at a constant rate.
At any given instant, find the probability that Tim can see point from his window. Justify your answer.
-
21N.2.SL.TZ0.3d:
Sketch the function for , clearly labelling the coordinates of the maximum and minimum points.
-
21N.2.AHL.TZ0.7b.i:
Write down null and alternative hypotheses for this test.
-
22M.1.SL.TZ1.7a:
Write down the null and alternative hypotheses.
-
22M.1.SL.TZ1.10a:
Complete the tree diagram.
-
22M.1.AHL.TZ1.15a:
Assuming the null hypothesis to be true, state the distribution of .
-
22M.1.SL.TZ2.7b:
With reference to the box and whisker diagrams, state one aspect that may support the researcher’s opinion and one aspect that may counter it.
-
22M.1.AHL.TZ2.9c.i:
State the alternative hypothesis.
-
22M.2.SL.TZ1.3c:
State whether it would be appropriate for Chester to use the equation of a regression line for on to predict a country’s Eurovision score. Justify your answer.
-
SPM.1.SL.TZ0.3b:
Find the value of .
-
SPM.1.SL.TZ0.13a:
Find the probability that on any given day Mr Burke chooses a female student to answer a question.
-
SPM.1.AHL.TZ0.9c:
The bags are labelled as being 1.5 kg mass. Comment on this claim with reference to your answer in part (b).
-
SPM.2.SL.TZ0.3f:
The Commissioner believes Minsun’s score for competitor G is too high and so decreases the score from 9.5 to 9.1.
Explain why the value of the Spearman’s rank correlation coefficient does not change.
-
SPM.2.SL.TZ0.3e.ii:
Comment on the result obtained for .
-
SPM.3.AHL.TZ0.1a:
Identify a test that might have been used to verify the null hypothesis that the predictions from the standardized test can be modelled by a normal distribution.
-
SPM.2.SL.TZ0.3b:
Write down the equation of the regression line on .
-
SPM.3.AHL.TZ0.1c.i:
Find the mean change.
-
EXM.1.SL.TZ0.2b:
State what conclusion Kayla can make from the answer in part (a).
-
EXM.1.SL.TZ0.3a:
Explain why it is not appropriate to use Pearson’s product moment correlation coefficient to measure the strength of the relationship between and .
-
EXM.1.AHL.TZ0.18a:
Show that is always an eigenvalue for M and find the other eigenvalue in terms of and .
-
22M.2.AHL.TZ2.5a.i:
Write down the value of .
-
22M.2.AHL.TZ2.5d.i:
when .
-
EXM.1.AHL.TZ0.19d:
Hence determine, with a reason, if the survey is reliable.
-
EXM.1.SL.TZ0.7c:
Find the p-value for this t-test.
-
EXM.2.SL.TZ0.5d:
State an assumption that the company is making, in order to use a t-test.
-
EXM.3.AHL.TZ0.8f:
Perform a suitable test, at the 5% significance level, to determine if it is easier to achieve a distinction on the new exam. You should clearly state your hypotheses, the critical region and your conclusion.
-
EXM.3.AHL.TZ0.9b.ii:
the day when the total number of people infected will be greater than 1000.
-
EXM.3.AHL.TZ0.9f.iii:
.
-
EXM.3.AHL.TZ0.9d.ii:
Perform a goodness of fit test at the 5% significance level. You should clearly state your hypotheses, the p-value, and your conclusion.
-
EXM.3.AHL.TZ0.9c:
Use your answer to part (a) to show that the model predicts 16.7 people will be infected on the first day.
-
22M.3.AHL.TZ1.1a.ii:
Write down the value of , Pearson’s product-moment correlation coefficient.
-
22M.3.AHL.TZ2.1a.ii:
Hence state why Aimmika believes her data follows a Poisson distribution.
-
22M.3.AHL.TZ2.1d.ii:
Perform the goodness of fit test and state, with reason, a conclusion.
-
EXM.1.SL.TZ0.8b:
Test the hypothesis at the 5% level of significance.
-
EXM.2.AHL.TZ0.27a.i:
a goodness of fit test (a complete explanation required);
-
18M.1.SL.TZ1.T_10c:
Write down the value of .
-
18M.2.SL.TZ1.T_5b:
Find the probability that only one of Ayako and Natsuko falls into a trap while attempting to pass through a door in the first wall.
-
17M.2.AHL.TZ1.H_1a:
Calculate ;
-
16N.1.SL.TZ0.T_12b:
Find the value of .
-
17M.1.SL.TZ2.T_2c:
Find the number of children who play only football.
-
19M.2.SL.TZ1.T_3e.i:
State the null hypothesis.
-
19M.2.SL.TZ2.T_1a:
Write down the null hypothesis, H0 , for this test.
-
19M.2.SL.TZ2.T_1b:
State the number of degrees of freedom.
-
18N.1.SL.TZ0.T_4a:
State the null hypothesis, H0, for this test.
-
18N.2.SL.TZ0.T_1a.iii:
Use your graphic display calculator to write down r , Pearson’s product–moment correlation coefficient.
-
19M.1.SL.TZ2.T_12a.i:
.
-
18M.2.AHL.TZ1.H_6:
The mean number of squirrels in a certain area is known to be 3.2 squirrels per hectare of woodland. Within this area, there is a 56 hectare woodland nature reserve. It is known that there are currently at least 168 squirrels in this reserve.
Assuming the population of squirrels follow a Poisson distribution, calculate the probability that there are more than 190 squirrels in the reserve.
-
18M.1.AHL.TZ1.H_3b:
Find the expected value of T.
-
16N.2.AHL.TZ0.H_1a:
Determine the value of .
-
16N.2.SL.TZ0.S_8b:
During week 2, the students worked on a major project and they each spent an additional five hours browsing the Internet. For week 2, write down
(i) the mean;
(ii) the standard deviation.
-
16N.2.SL.TZ0.T_1a:
On graph paper, draw a scatter diagram for these data. Use a scale of 2 cm to represent 5 hours on the -axis and 2 cm to represent 10 points on the -axis.
-
16N.2.SL.TZ0.T_1b:
(i) , the mean number of hours spent on social media;
(ii) , the mean number of IB Diploma points.
-
16N.2.SL.TZ0.T_1f:
Draw the regression line, from part (e), on your scatter diagram.
-
19M.1.SL.TZ1.T_5b:
Write down the probability that a bouquet of roses sold is not small.
-
17M.2.SL.TZ2.T_1a.ii:
If the teacher chooses a response at random, find the probability that it is a satisfactory response to the Calculus question;
-
17M.2.SL.TZ2.T_1d:
Show that the expected frequency of satisfactory Calculus responses is 12.
-
17M.2.SL.TZ2.T_3b.i:
Write down, for this set of data the mean number of folders produced, ;
-
17M.2.SL.TZ2.T_3f:
Use your graphic display calculator to find the equation of the regression line on .
-
16N.1.SL.TZ0.T_6a:
Find the expected frequency of the patients who became infected whilst in Nightingale ward.
-
19M.2.SL.TZ2.S_9b.ii:
Given that all passengers for a flight arrive on time, find the probability that the flight does not depart on time.
-
18N.2.AHL.TZ0.H_3a:
Find the value of and the value of .
-
19M.2.SL.TZ1.S_10a.i:
Find the probability of rolling exactly one red face.
-
19M.2.SL.TZ1.S_10c.ii:
Hence, find the value of .
-
17M.1.AHL.TZ1.H_10c.i:
Show that .
-
17N.2.SL.TZ0.T_1f.i:
Write down the -value for the test;
-
17M.1.SL.TZ1.S_4a.i:
Write down the independent variable.
-
17M.1.SL.TZ1.S_4a.ii:
Write down the boiling temperature of the liquid.
-
17M.1.SL.TZ2.T_6a:
Write down the median length of these leaves.
-
17M.1.SL.TZ2.T_7c:
Find the value of and of .
-
17M.2.SL.TZ1.T_5f.ii:
Calculate the expected number of students that spent at least 90 minutes preparing for the test.
-
18M.2.SL.TZ2.T_2c:
Hence write down the interquartile range.
-
18M.2.SL.TZ2.T_2e:
Find the number of buses that travelled a distance less than or equal to 12 000 km.
-
17M.2.SL.TZ2.S_10b.iii:
The bag contains a total of ten marbles of which are white. Find .
-
19M.2.SL.TZ1.S_9c:
Find the acute angle between and .
-
16N.2.SL.TZ0.S_10c:
(i) Find .
(ii) Hence or otherwise, find the maximum positive rate of change of .
-
19M.2.AHL.TZ1.H_9a.i:
both a sandwich and a cake.
-
19M.2.AHL.TZ1.H_9b.i:
Find the expected number of cakes sold on a typical day.
-
16N.1.AHL.TZ0.H_2b:
Find the expected value of .
-
17M.2.SL.TZ1.S_9a:
Find .
-
19M.2.AHL.TZ2.H_10b:
Copy and complete the probability distribution table for Y.
-
19M.1.SL.TZ1.T_12b:
Find the probability that at least one of the spins is yellow.
-
19M.2.SL.TZ2.S_9c:
Find the value of .
-
19M.2.SL.TZ2.S_9d:
All flights have two pilots. Find the percentage of flights where both pilots flew more than 30 hours last week.
-
17N.1.SL.TZ0.T_13c:
Estimate the number of applicants who completed the test in less than 25 minutes.
-
19M.1.SL.TZ2.T_14a.i:
On the following diagram, shade the region representing the probability that the price of a kilogram of tomatoes, chosen at random, will be higher than 3.22 euro.
-
18N.2.SL.TZ0.T_2a.i:
Find the number of students in the school that are taught in Spanish.
-
18N.2.SL.TZ0.T_2c.ii:
Find the probability that this student studies neither Biology nor Mathematics.
-
17M.1.SL.TZ1.S_1a.i:
Find the value of ;
-
19M.1.SL.TZ2.T_11b.ii:
Write down .
-
18N.1.SL.TZ0.S_10b.i:
Find .
-
18N.1.SL.TZ0.S_10c:
The graph of has a local minimum at the point Q. The line L passes through Q.
Find the value of .
-
16N.2.SL.TZ0.T_6c:
Write down, in terms of and , an equation for the volume of this water container.
-
18M.2.AHL.TZ2.H_3c:
Find the value of k for which P(μ − kσ < X < μ + kσ) = 0.5.
-
17N.2.AHL.TZ0.H_2a:
Find .
-
19M.1.AHL.TZ1.H_10d:
The random variable follows a Poisson distribution with a mean of and .
Find the value of .
-
18M.2.AHL.TZ2.H_9a.ii:
Find the most likely number of taxis that would arrive during T.
-
19M.2.AHL.TZ2.H_3c:
Find the probability that Iqbal passes his third paper, given that he passed only one previous paper.
-
16N.2.SL.TZ0.S_7a:
Write down the probability that the first disc selected is red.
-
16N.2.SL.TZ0.S_7b:
Let be the number of red discs selected. Find the smallest value of for which .
-
17N.3.AHL.TZ0.Hsp_2a:
Determine unbiased estimates for and .
-
18M.3.AHL.TZ0.Hsp_3b:
Find the critical region for testing at the 5 % significance level.
-
17N.1.SL.TZ0.T_7b:
One of the students who joined the sports club is chosen at random. Find the probability that this student joined both clubs.
-
17M.1.SL.TZ1.T_4a:
Write down the number of sporting activities offered by the school during its school year.
-
17M.1.SL.TZ2.T_10c:
Write down an expression, in terms of , for the probability that at least one of Deborah’s three light bulbs is defective.
-
17N.2.SL.TZ0.T_4b:
Find the probability that both people chosen are not allergic to nuts.
-
19M.3.AHL.TZ0.Hsp_2a:
Find the probability that the time taken for a randomly chosen customer to be dealt with by an employee is greater than 180 seconds.
-
17N.1.SL.TZ0.S_5a:
Find .
-
19M.1.SL.TZ2.S_9a:
Find the value of .
-
19M.1.SL.TZ2.S_9d:
The following diagram shows the graph of for 0 ≤ ≤ 3. Line is a tangent to the graph of at point P.
Given that is parallel to , find the -coordinate of P.
-
17N.3.AHL.TZ0.Hsp_4b:
Find the least value of for which the test concludes that .
-
17M.2.AHL.TZ2.H_1a:
One of the players is chosen at random. Find the probability that this player’s score was 5 or more.
-
16N.3.AHL.TZ0.Hsp_2b:
Explain why a normal distribution can be used to give an approximate model for .
-
17M.3.AHL.TZ0.Hsp_1c.ii:
Using a 10% significance level and justifying your answer, state your conclusion in context.
-
16N.2.AHL.TZ0.H_11c:
Deduce that for .
-
EXM.2.SL.TZ0.6b.ii:
a Type II error.
-
18N.2.SL.TZ0.S_10a:
Find the volume of the container.
-
18N.2.SL.TZ0.S_10b.i:
Find the value of and of .
-
17M.1.SL.TZ1.S_6a.ii:
Find the equation of the normal to the curve of at P.
-
17M.1.SL.TZ1.S_6b:
Determine the concavity of the graph of when and justify your answer.
-
17M.2.SL.TZ2.S_8c.i:
Find the coordinates of B.
-
19N.3.AHL.TZ0.Hsp_1a.ii:
Carry out a suitable test at the 5 % significance level. With reference to the -value, state your conclusion in the context of Peter’s claim.
-
19N.1.SL.TZ0.T_13a:
State whether the weight of the eggs is a continuous or discrete variable.
-
19N.1.SL.TZ0.T_13d:
The mean weight of these eggs is 64.9 grams, correct to three significant figures.
Use the table and your answer to part (c) to find the smallest possible number of eggs that could be within one standard deviation of the mean.
-
19N.1.SL.TZ0.T_15a:
Write down the median rehearsal time.
-
19N.1.SL.TZ0.T_15b:
State whether Stephen is correct. Give a reason for your answer.
-
19N.1.SL.TZ0.T_15c:
On days, Stephen practiced exactly minutes.
Find the possible values of .
-
19N.2.SL.TZ0.T_1f.iii:
Given that the customer is a child, calculate the probability that they chose pasta or fish.
-
21M.2.SL.TZ2.4c:
Determine the expected number of cats in this group that have a weight of less than .
-
21M.2.AHL.TZ2.4a:
Write down a transition matrix indicating the annual population movement between clinics.
-
21M.3.AHL.TZ1.2d.ii:
For the data in this table, test the null hypothesis, , against the alternative hypothesis, , at the significance level. You may assume that all the requirements for carrying out the test have been met.
-
21M.3.AHL.TZ1.2e.i:
Write down the number of tests they carry out.
-
21M.3.AHL.TZ1.2e.iii:
The firm obtains a significant result when comparing section of the written assessment and attribute . Interpret this result.
-
21M.3.AHL.TZ2.1e.vi:
Juliet decides to use the coefficient of determination to choose between these two models.
Comment on the validity of her decision.
-
21M.3.AHL.TZ2.1f.i:
State the name of the test which Juliet should use.
-
21M.3.AHL.TZ2.1e.v:
Hence compare the two models.
-
20N.2.AHL.TZ0.H_5a.i:
State two assumptions made in order for this model to be valid.
-
20N.1.SL.TZ0.S_1b:
Find the probability that a randomly selected student from the class plays tennis or volleyball, but not both.
-
20N.2.SL.TZ0.S_2a.i:
Find the value of and of .
-
20N.2.SL.TZ0.S_3a:
Find an expression for in terms of .
-
20N.2.SL.TZ0.S_9c:
Find the probability that the bus journey takes less than minutes.
-
20N.1.SL.TZ0.T_10c:
The -value for this test is .
State the conclusion to Isaac’s test. Justify your reasoning.
-
20N.1.SL.TZ0.T_14b:
Find the value of .
-
20N.1.SL.TZ0.T_3c:
On the grid below, draw a histogram for the data in the table.
-
20N.1.SL.TZ0.T_7a:
Find the height of Flower .
-
20N.2.SL.TZ0.T_2b:
Find the number of students who visited at least two types of main attraction.
-
20N.2.SL.TZ0.T_6f:
Find the expected number of successful shots Emlyn will make on Monday, based on the results from Saturday and Sunday.
-
EXN.1.SL.TZ0.3b.ii:
The test is performed at the significance level.
State the conclusion of the test, giving a reason for your answer.
-
EXN.1.AHL.TZ0.6c:
Find the probability it will rain at least once while Paula is outside.
-
EXN.2.SL.TZ0.4c.i:
Find the expected frequency for each of the numbers if the manufacturer’s claim is true.
-
EXN.2.AHL.TZ0.3d:
Determine the degrees of freedom for Dana’s test.
-
21M.1.SL.TZ1.10b.i:
a player scores at least in a game.
-
21M.1.AHL.TZ1.11d:
State the conclusion of the test. Give a reason for your answer.
-
21M.1.AHL.TZ1.14a:
Find the mean weight of a bag of apples.
-
21M.1.SL.TZ2.8a:
Write down the null and alternative hypotheses.
-
21M.1.AHL.TZ2.10b:
Use the formula to determine an unbiased estimate for the variance of the number of chocolates per packet.
-
21M.2.SL.TZ1.1d:
Determine whether Jason is correct. Support your reasoning.
-
21M.2.SL.TZ1.1h:
Copy and complete the information in the following table.
-
21M.2.SL.TZ1.4b.i:
and .
-
21M.2.SL.TZ1.4d:
Find the -value for the test.
-
21M.2.AHL.TZ1.5c.i:
Write down the matrix .
-
21M.2.AHL.TZ1.5c.ii:
Write down the matrix .
-
21N.1.SL.TZ0.1b:
Comment on your answer to part (a), using the information that Eduardo found.
-
21N.1.SL.TZ0.11a:
Write down the value of .
-
21N.1.SL.TZ0.11b:
Find an expression, in terms of , for the probability of a person not having blue eyes and having fair hair.
-
21N.1.AHL.TZ0.14a:
Find the probability that a sack is under its labelled weight.
-
21N.2.SL.TZ0.1a.ii:
is years old and prefers a mobile phone.
-
21N.2.AHL.TZ0.3d.ii:
Find the probability that Arianne scores at least points in the competition.
-
22M.1.SL.TZ1.7e:
State the conclusion of the test. Give a reason for your answer.
-
22M.1.AHL.TZ1.15c:
Find the probability of a Type II error, if the number of cars now follows a Poisson distribution with a mean of cars per minute.
-
22M.1.SL.TZ2.7a:
Show that the test score of would not be considered an outlier.
-
22M.1.SL.TZ2.8a:
State the alternative hypothesis.
-
22M.2.SL.TZ1.3e.ii:
Interpret the value obtained for .
-
22M.2.SL.TZ1.5c:
Find, to the nearest integer, the expected increase or decrease in the money made by the airline if they decide to sell tickets rather than .
-
22M.2.SL.TZ1.3a.ii:
the interquartile range.
-
22M.2.SL.TZ1.3d.iii:
.
-
22M.2.AHL.TZ1.3d:
State one reason why the test might not be valid.
-
SPM.1.SL.TZ0.3c:
It was not possible to ask every person in the school, so the Headmaster arranged the student names in alphabetical order and then asked every 10th person on the list.
Identify the sampling technique used in the survey.
-
SPM.1.SL.TZ0.13b:
Find the probability he will choose a female student 8 times.
-
22M.2.SL.TZ2.1a.ii:
interquartile range of the reaction times.
-
SPM.1.SL.TZ0.6a:
State the null hypothesis.
-
SPM.1.SL.TZ0.12a:
Find the exact value of .
-
SPM.1.AHL.TZ0.16b:
Find the probability of a Type I error.
-
SPM.2.SL.TZ0.3d:
Copy and complete the information in the following table.
-
SPM.2.SL.TZ0.3c.i:
Use your regression equation from part (b) to estimate Minsun’s score when Stan awards a perfect 10.
-
SPM.3.AHL.TZ0.1e.ii:
State why it was important to test that both sets of points were normally distributed.
-
SPM.3.AHL.TZ0.1f.i:
Perform a test on the data from school A to show it is reasonable to assume a linear relationship between effort scores and improvements in IB points. You may assume effort scores follow a normal distribution.
-
22M.2.AHL.TZ2.5a.ii:
What does represent in this context?
-
EXM.2.AHL.TZ0.12a:
Use the trapezoidal rule to find an estimate for the area.
-
EXM.1.AHL.TZ0.17b:
We know that she went out for lunch on a particular Sunday, find the probability that she went out for lunch on the following Tuesday.
-
EXM.2.SL.TZ0.5e:
State the hypotheses for this t-test.
-
EXM.1.AHL.TZ0.19b:
State a possible disadvantage of using this test for reliability.
-
EXM.2.SL.TZ0.5c.i:
The new drug.
-
EXM.3.AHL.TZ0.8c:
Perform a suitable test, at the 5% significance level, to determine if the scores follow a normal distribution, with the mean and variance found in part (a). You should clearly state your hypotheses, the degrees of freedom, the p-value and your conclusion.
-
EXM.3.AHL.TZ0.9d.i:
Explain why the number of degrees of freedom is 2.
-
22M.3.AHL.TZ1.1a.i:
Find the equation of the regression line of on .
-
EXM.1.SL.TZ0.11b:
Explain what is meant by the 5% level of significance.
-
EXM.2.AHL.TZ0.29c:
A different horse breeder collected data on the time and outcome of births. The data are summarized in the following table:
Carry out an appropriate test at the 5% significance level to decide whether there is an association between time and outcome.
-
18M.2.SL.TZ2.S_10c:
Find the probability that a bag of apples selected at random contains at most one small apple.
-
18N.1.AHL.TZ0.H_1b:
Show that the events and are not independent.
-
18M.1.SL.TZ1.T_10a:
Complete the Venn diagram using the given information.
-
18M.1.SL.TZ2.T_7b:
Find the probability that the boy answered questions in Hindi.
-
18M.2.SL.TZ1.T_5e:
120 contestants attempted this game.
Find the expected number of contestants who fell into a trap while attempting to pass through a door in the third wall.
-
18M.2.SL.TZ2.T_1a.i:
Write down the value of a.
-
17N.1.SL.TZ0.S_1a:
Complete the following tree diagram.
-
17M.1.SL.TZ2.T_2a:
Write down an expression, in terms of , for the number of children who play only basketball.
-
19M.2.SL.TZ1.T_1d.ii:
Hence describe the correlation between temperature difference from 37 °C and heartbeat.
-
19M.2.SL.TZ2.T_1e.iii:
Find the probability that at least one of the two students is female.
-
18N.2.SL.TZ0.T_1b.i:
Find the exact value of m and of c for these data.
-
18N.2.SL.TZ0.T_1c.ii:
Justify whether it is valid to use the regression line y on x to estimate Jerome’s examination score.
-
16N.2.SL.TZ0.T_2c:
Write down the value of .
-
18M.1.SL.TZ1.T_2b.i:
Find the standard deviation
-
18M.1.SL.TZ1.T_2b.ii:
Find the interquartile range.
-
18M.2.SL.TZ1.T_2e:
State, with a reason, whether you would reject the null hypothesis.
-
18M.2.SL.TZ2.S_1b:
Use your equation to estimate the mean weight of a child that is 1.95 years old.
-
19M.1.SL.TZ1.T_11a:
In the following table, write down the letter of the corresponding graph next to the given mean and standard deviation.
-
19M.1.SL.TZ1.T_11b:
Find the probability that a suitcase weighs less than 15 kg.
-
18M.2.SL.TZ2.T_3d.i:
For this test state the null hypothesis.
-
17N.1.SL.TZ0.T_1b:
Draw a box-and-whisker diagram, for these students’ ages, on the following grid.

-
17N.1.SL.TZ0.T_5c:
By placing a tick (✔) in the correct box, determine which of the following statements is true:

-
17N.1.SL.TZ0.T_5d:
Diogo is 18 years old. Give a reason why the regression line should not be used to estimate the number of hours Diogo watches television per week.
-
17M.2.SL.TZ2.T_1e:
Write down the number of degrees of freedom for this test.
-
19M.1.AHL.TZ1.H_6b:
.
-
18M.1.SL.TZ1.S_2b:
One student sent k text messages, where k > 11 . Given that k is an outlier, find the least value of k.
-
17N.2.SL.TZ0.S_8d.ii:
Find the number of hives that have a high production.
-
17M.2.SL.TZ1.T_1d:
Use your regression line to estimate the average weight of the brain of grey wolves.
-
17M.2.SL.TZ1.T_5a.ii:
Calculate the standard deviation.
-
17M.2.SL.TZ2.S_2a.i:
Find the value of and of .
-
17M.2.AHL.TZ2.H_10d:
Find the median of .
-
16N.2.SL.TZ0.S_10b:
(i) Write down the value of .
(ii) Find .
-
16N.1.AHL.TZ0.H_2a:
Complete the probability distribution table for .

-
18N.1.SL.TZ0.S_9c:
Hayley plays the game when = 5. She pays $20 to play and can earn money back depending on the number of draws it takes to obtain a blue marble. She earns no money back if she obtains a blue marble on her first draw. Let M be the amount of money that she earns back playing the game. This information is shown in the following table.
Find the value of so that this is a fair game.
-
17M.1.SL.TZ2.S_3a:
Write down .
-
18M.2.AHL.TZ2.H_9a.i:
Find the probability that exactly 4 taxis arrive during T.
-
17M.2.SL.TZ1.S_4a:
Find .
-
17M.1.SL.TZ1.S_1b:
A girl is selected at random. Find the probability that she takes economics but not history.
-
16N.2.SL.TZ0.T_4a:
Draw a diagram that shows this information.
-
18N.2.AHL.TZ0.H_10b.ii:
an estimate for the standard deviation of the number of emails received per working day.
-
18N.2.AHL.TZ0.H_10c:
Give one piece of evidence that suggests Willow’s Poisson distribution model is not a good fit.
-
18N.2.AHL.TZ0.H_10d:
Suppose that the probability of Archie receiving more than 10 emails in total on any one day is 0.99. Find the value of λ.
-
18M.2.AHL.TZ2.H_9b:
During quiet periods of the day, taxis arrive at a mean rate of 1.3 taxis every 10 minutes.
Find the probability that during a period of 15 minutes, of which the first 10 minutes is busy and the next 5 minutes is quiet, that exactly 2 taxis arrive.
-
17N.2.AHL.TZ0.H_6a:
Find the probability that Lucca eats at least one banana in a particular day.
-
19M.3.AHL.TZ0.Hsp_3a:
State the distribution of giving its mean and variance.
-
16N.2.AHL.TZ0.H_8a:
Find and .
-
18M.1.SL.TZ2.S_8d:
Given that Pablo is late for work, find the probability that he left home before 07:00.
-
18M.2.SL.TZ2.S_10a.ii:
Show that μ = 106.
-
17M.1.SL.TZ1.T_4c.i:
Write down the elements of the set ;
-
19M.3.AHL.TZ0.Hsp_3d:
Find a 90 % confidence interval for .
-
18N.3.AHL.TZ0.Hsp_2c:
Before seeing these results the managing director believed that the mean time was 26 minutes.
Explain whether your answers to part (b) support her belief.
-
17N.1.SL.TZ0.S_5b:
Given that , find the value of .
-
16N.3.AHL.TZ0.Hsp_2c:
Use this model to find the values of and such that , where and are symmetrical about the mean of .
-
17M.1.SL.TZ1.S_9a:
Find the value of .
-
17M.2.SL.TZ2.S_8b.i:
Write down the coordinates of A.
-
19N.2.AHL.TZ0.H_2b:
Find the probability that she will run at least two marathons in exactly four out of the following five years.
-
19N.1.SL.TZ0.T_13c:
Use your graphic display calculator to find an estimate for the standard deviation of the weight of the eggs.
-
19N.1.SL.TZ0.T_4b:
Complete the following Venn diagram using all elements of .
-
19N.2.SL.TZ0.T_1a:
State , the null hypothesis for this test.
-
19N.2.SL.TZ0.T_1f.ii:
Calculate the probability that the customer is an adult or that the customer chose shrimp.
Sub sections and their related questions
SL 4.1—Concepts, reliability and sampling techniques
-
SPM.1.SL.TZ0.3a:
State whether the data is discrete or continuous.
-
SPM.1.SL.TZ0.3b:
Find the value of .
-
SPM.1.SL.TZ0.3c:
It was not possible to ask every person in the school, so the Headmaster arranged the student names in alphabetical order and then asked every 10th person on the list.
Identify the sampling technique used in the survey.
-
EXM.1.SL.TZ0.5a:
Recalling definitions, such as the Lower Quartile is the piece of data with the data placed in order, find an expression for the Interquartile Range.
-
EXM.1.SL.TZ0.5b:
Hence, show that a data set with only 5 numbers in it cannot have any outliers.
-
EXM.1.SL.TZ0.5c:
Give an example of a set of data with 7 numbers in it that does have an outlier, justify this fact by stating the Interquartile Range.
-
EXM.2.SL.TZ0.5a:
State the name for this type of sampling technique.
-
EXM.2.SL.TZ0.5b:
Calculate the number of volunteers in the sample under the age of 30.
-
EXM.2.SL.TZ0.5c.i:
The new drug.
-
EXM.2.SL.TZ0.5c.ii:
The current drug.
-
EXM.2.SL.TZ0.5d:
State an assumption that the company is making, in order to use a t-test.
-
EXM.2.SL.TZ0.5e:
State the hypotheses for this t-test.
-
EXM.2.SL.TZ0.5f:
Find the p-value for this t-test.
-
EXM.2.SL.TZ0.5g:
State the conclusion of this test, in context, giving a reason.
-
18M.1.AHL.TZ2.H_3a:
Find the value of p.
-
18M.1.AHL.TZ2.H_3b.i:
Find μ, the expected value of X.
-
18M.1.AHL.TZ2.H_3b.ii:
Find P(X > μ).
-
18N.2.AHL.TZ0.H_10a.i:
Using this distribution model, find .
-
18N.2.AHL.TZ0.H_10a.ii:
Using this distribution model, find the standard deviation of .
-
18N.2.AHL.TZ0.H_10b.i:
an estimate for the mean number of emails received per working day.
-
18N.2.AHL.TZ0.H_10b.ii:
an estimate for the standard deviation of the number of emails received per working day.
-
18N.2.AHL.TZ0.H_10c:
Give one piece of evidence that suggests Willow’s Poisson distribution model is not a good fit.
-
18N.2.AHL.TZ0.H_10d:
Suppose that the probability of Archie receiving more than 10 emails in total on any one day is 0.99. Find the value of λ.
-
18N.2.AHL.TZ0.H_10e:
Now suppose that Archie received exactly 20 emails in total in a consecutive two day period. Show that the probability that he received exactly 10 of them on the first day is independent of λ.
-
16N.2.AHL.TZ0.H_11a:
Show that and .
-
16N.2.AHL.TZ0.H_11b:
Find the values of the constants and .
-
16N.2.AHL.TZ0.H_11c:
Deduce that for .
-
16N.2.AHL.TZ0.H_11d:
(i) Hence show that has two modes and .
(ii) State the values of and .
-
16N.2.AHL.TZ0.H_11e:
Determine the minimum value of such that the probability Kati receives at least one free gift is greater than 0.5.
-
19M.2.AHL.TZ1.H_3b.ii:
the standard deviation.
-
17N.1.SL.TZ0.S_5a:
Find .
-
17N.1.SL.TZ0.S_5b:
Given that , find the value of .
-
19M.2.SL.TZ1.S_9a:
Find the gradient of .
-
19M.2.SL.TZ1.S_9b:
Find u.
-
19M.2.SL.TZ1.S_9c:
Find the acute angle between and .
-
19M.2.SL.TZ1.S_9d.i:
Find .
-
19M.2.SL.TZ1.S_9d.ii:
Hence, write down .
-
19M.2.SL.TZ1.S_9d.iii:
Hence or otherwise, find the obtuse angle formed by the tangent line to at and the tangent line to at .
-
19M.1.SL.TZ2.S_9a:
Find the value of .
-
19M.1.SL.TZ2.S_9b:
Line passes through the origin and has a gradient of . Find the equation of .
-
19M.1.SL.TZ2.S_9c:
Find the derivative of .
-
19M.1.SL.TZ2.S_9d:
The following diagram shows the graph of for 0 ≤ ≤ 3. Line is a tangent to the graph of at point P.
Given that is parallel to , find the -coordinate of P.
-
16N.2.SL.TZ0.S_10a:
(i) Find the value of .
(ii) Show that .
(iii) Find the value of .
-
16N.2.SL.TZ0.S_10b:
(i) Write down the value of .
(ii) Find .
-
16N.2.SL.TZ0.S_10c:
(i) Find .
(ii) Hence or otherwise, find the maximum positive rate of change of .
-
18M.1.SL.TZ1.S_2a:
Find the value of the interquartile range.
-
18M.1.SL.TZ1.S_2b:
One student sent k text messages, where k > 11 . Given that k is an outlier, find the least value of k.
-
17M.1.SL.TZ1.S_6a.i:
Write down the gradient of the curve of at P.
-
17M.1.SL.TZ1.S_6a.ii:
Find the equation of the normal to the curve of at P.
-
17M.1.SL.TZ1.S_6b:
Determine the concavity of the graph of when and justify your answer.
-
17M.1.SL.TZ1.S_9a:
Find the value of .
-
17M.1.SL.TZ1.S_9b:
Find the value of .
-
17M.1.SL.TZ1.S_9c:
The line is a tangent to the curve of . Find the values of .
-
17M.2.SL.TZ2.S_8a:
Find the value of .
-
17M.2.SL.TZ2.S_8b.i:
Write down the coordinates of A.
-
17M.2.SL.TZ2.S_8b.ii:
Write down the rate of change of at A.
-
17M.2.SL.TZ2.S_8c.i:
Find the coordinates of B.
-
17M.2.SL.TZ2.S_8c.ii:
Find the the rate of change of at B.
-
17M.2.SL.TZ2.S_8d:
Let be the region enclosed by the graph of , the -axis, the line and the line . The region is rotated 360° about the -axis. Find the volume of the solid formed.
-
18M.1.SL.TZ1.S_7:
Consider f(x), g(x) and h(x), for x∈ where h(x) = (x).
Given that g(3) = 7 , g′ (3) = 4 and f ′ (7) = −5 , find the gradient of the normal to the curve of h at x = 3.
-
18N.1.SL.TZ0.S_10a:
Find the coordinates of P.
-
18N.1.SL.TZ0.S_10b.i:
Find .
-
18N.1.SL.TZ0.S_10b.ii:
Hence, find the equation of L in terms of .
-
18N.1.SL.TZ0.S_10c:
The graph of has a local minimum at the point Q. The line L passes through Q.
Find the value of .
-
18N.2.SL.TZ0.S_10a:
Find the volume of the container.
-
18N.2.SL.TZ0.S_10b.i:
Find the value of and of .
-
18N.2.SL.TZ0.S_10b.ii:
During the interval < < , he volume of water in the container increases by m3. Find the value of .
-
18N.2.SL.TZ0.S_10c:
When = 0, the volume of water in the container is 2.3 m3. It is known that the container is never completely full of water during the 4 hour period.
Find the minimum volume of empty space in the container during the 4 hour period.
-
19M.2.SL.TZ1.S_3a:
Find .
-
19M.2.SL.TZ1.S_3b:
The graph of has a horizontal tangent line at and at . Find .
-
EXN.1.SL.TZ0.1a:
Calculate the number of grade students who should be in the sample.
-
EXN.1.SL.TZ0.1b:
The Principal selects the students for the sample by asking those who took part in a previous survey if they would like to take part in another. She takes the first of those who reply positively, up to the maximum needed for the sample.
State which two of the sampling methods listed below best describe the method used.
Stratified Quota Convenience Systematic Simple random
-
21M.1.AHL.TZ1.11a:
Identify the type of sampling used by the restaurant manager.
-
21M.2.SL.TZ1.1a:
State which of the two sampling methods, systematic or quota, Jason has used.
-
21M.2.SL.TZ1.1d:
Determine whether Jason is correct. Support your reasoning.
-
21M.3.AHL.TZ1.2b:
Show that employees are selected for the sample from the national department.
-
21M.2.SL.TZ2.1a:
State the sampling method being used.
-
21M.3.AHL.TZ2.1b:
Juliet classifies response as an outlier and removes it from the data. Suggest one possible justification for her decision to remove it.
-
22M.1.SL.TZ2.7a:
Show that the test score of would not be considered an outlier.
-
22M.2.SL.TZ1.3b:
Determine if the Netherlands’ score is an outlier for this data. Justify your answer.
-
22M.2.AHL.TZ1.3a:
Name the type of sampling that best describes the method used by the Principal.
SL 4.2—Histograms, CF graphs, box plots
-
17M.1.SL.TZ2.T_6a:
Write down the median length of these leaves.
-
17M.1.SL.TZ2.T_6b:
Write down the number of leaves with a length less than or equal to 8 cm.
-
16N.2.SL.TZ0.S_8a:
Find the mean number of hours spent browsing the Internet.
-
16N.2.SL.TZ0.S_8b:
During week 2, the students worked on a major project and they each spent an additional five hours browsing the Internet. For week 2, write down
(i) the mean;
(ii) the standard deviation.
-
16N.2.SL.TZ0.S_8c:
During week 3 each student spent 5% less time browsing the Internet than during week 1. For week 3, find
(i) the median;
(ii) the variance.
-
16N.2.SL.TZ0.S_8d:
(i) Find the number of students who spent between 25 and 30 hours browsing the Internet.
(ii) Given that 10% of the students spent more than k hours browsing the Internet, find the maximum value of .
-
17M.1.SL.TZ2.S_8a.ii:
Write down the number of employees who worked 50 hours or less.
-
17M.1.SL.TZ2.S_8b.i:
Find the amount of money an employee earned for working 40 hours;
-
17N.2.SL.TZ0.S_8a:
Write down the value of and of .
-
17N.2.SL.TZ0.S_8b:
Use this regression line to estimate the monthly honey production from a hive that has 270 bees.
-
17N.2.SL.TZ0.S_8c:
Write down the number of low production hives.
-
17N.2.SL.TZ0.S_8d.i:
Find the value of ;
-
17N.2.SL.TZ0.S_8d.ii:
Find the number of hives that have a high production.
-
17N.2.SL.TZ0.S_8e:
Adam decides to increase the number of bees in each low production hive. Research suggests that there is a probability of 0.75 that a low production hive becomes a regular production hive. Calculate the probability that 30 low production hives become regular production hives.
-
17M.1.SL.TZ1.T_1a:
Complete the following table.

-
18M.2.SL.TZ1.T_2a:
State the alternative hypothesis.
-
18M.2.SL.TZ1.T_2b:
Calculate the expected frequency of flights travelling at most 500 km and arriving slightly delayed.
-
18M.2.SL.TZ1.T_2c:
Write down the number of degrees of freedom.
-
18M.2.SL.TZ1.T_2d.i:
Write down the χ2 statistic.
-
18M.2.SL.TZ1.T_2d.ii:
Write down the associated p-value.
-
18M.2.SL.TZ1.T_2e:
State, with a reason, whether you would reject the null hypothesis.
-
18M.2.SL.TZ1.T_2f:
Write down the probability that this flight arrived on time.
-
18M.2.SL.TZ1.T_2g:
Given that this flight was not heavily delayed, find the probability that it travelled between 500 km and 5000 km.
-
18M.2.SL.TZ1.T_2h:
Two flights are chosen at random from those which were slightly delayed.
Find the probability that each of these flights travelled at least 5000 km.
-
18M.1.SL.TZ2.T_7a:
State the number of boys who answered questions in Portuguese.
-
18M.1.SL.TZ2.T_7b:
Find the probability that the boy answered questions in Hindi.
-
18M.1.SL.TZ2.T_7c:
Two girls are selected at random.
Calculate the probability that one girl answered questions in Mandarin and the other answered questions in Hindi.
-
17M.1.SL.TZ2.T_7a:
Write down an equation, in terms of and , for the total number of times the die was rolled.
-
17M.1.SL.TZ2.T_7b:
Using the mean score, write down a second equation in terms of and .
-
17M.1.SL.TZ2.T_7c:
Find the value of and of .
-
19M.1.SL.TZ1.T_5a:
Complete the cumulative frequency table.
-
19M.1.SL.TZ1.T_5b:
Write down the probability that a bouquet of roses sold is not small.
-
19M.1.SL.TZ1.T_5c:
A customer buys a large bouquet.
Find the probability that there are 12 roses in this bouquet.
-
18M.1.SL.TZ1.T_6c.i:
Write down the mid-interval value for the 100 < x ≤ 150 group.
-
18M.1.SL.TZ2.T_12a:
Write down the mid-interval value for 10 ≤ t < 15.
-
18M.1.SL.TZ2.T_12b.i:
Write down the total number of customers in terms of k.
-
17N.2.SL.TZ0.T_1a:
State whether is a discrete or a continuous variable.
-
17N.2.SL.TZ0.T_1b.i:
Write down, for , the modal class;
-
17N.2.SL.TZ0.T_1b.ii:
Write down, for , the mid-interval value of the modal class.
-
17N.2.SL.TZ0.T_1c.i:
Use your graphic display calculator to estimate the mean of ;
-
17N.2.SL.TZ0.T_1c.ii:
Use your graphic display calculator to estimate the standard deviation of .
-
17N.2.SL.TZ0.T_1d:
Find the expected frequency of students choosing the Science category and obtaining 31 to 40 correct answers.
-
17N.2.SL.TZ0.T_1e.i:
Write down the null hypothesis for this test;
-
17N.2.SL.TZ0.T_1e.ii:
Write down the number of degrees of freedom.
-
17N.2.SL.TZ0.T_1f.i:
Write down the -value for the test;
-
17N.2.SL.TZ0.T_1f.ii:
Write down the statistic.
-
17N.2.SL.TZ0.T_1g:
State the result of the test. Give a reason for your answer.
-
19M.1.SL.TZ2.T_12a.i:
.
-
19M.1.SL.TZ2.T_12a.ii:
.
-
19M.1.SL.TZ2.T_12b:
On the same grid, complete the cumulative frequency curve for these data.
-
19M.1.SL.TZ2.T_12c:
Use the cumulative frequency curve to find an estimate for the number of students who worked at most 35 hours per month.
-
17N.1.SL.TZ0.T_1a.ii:
For the students in this group write down the median age.
-
17N.1.SL.TZ0.T_1b:
Draw a box-and-whisker diagram, for these students’ ages, on the following grid.

-
18M.2.SL.TZ2.T_2a:
Find the number of buses that travelled a distance between 15000 and 20000 kilometres.
-
18M.2.SL.TZ2.T_2b.i:
Use the cumulative frequency curve to find the median distance.
-
18M.2.SL.TZ2.T_2b.ii:
Use the cumulative frequency curve to find the lower quartile.
-
18M.2.SL.TZ2.T_2b.iii:
Use the cumulative frequency curve to find the upper quartile.
-
18M.2.SL.TZ2.T_2c:
Hence write down the interquartile range.
-
18M.2.SL.TZ2.T_2d:
Write down the percentage of buses that travelled a distance greater than the upper quartile.
-
18M.2.SL.TZ2.T_2e:
Find the number of buses that travelled a distance less than or equal to 12 000 km.
-
18M.2.SL.TZ2.T_2f:
Find the value of m.
-
18M.2.SL.TZ2.T_2g:
The smallest distance travelled by one of the buses was 2500 km.
The longest distance travelled by one of the buses was 23 000 km.On graph paper, draw a box-and-whisker diagram for these data. Use a scale of 2 cm to represent 5000 km.
-
19M.1.SL.TZ2.T_6c:
The health inspector visits two school cafeterias. She inspects the same number of meals at each cafeteria. The data is shown in the following box-and-whisker diagrams.
Meals prepared in the school cafeterias are required to have less than 10 grams of sugar.
State, giving a reason, which school cafeteria has more meals that do not meet the requirement.
-
16N.1.SL.TZ0.T_2b:
Draw a box-and-whisker diagram on the grid below to represent the Vitamin C content, in milligrams, for this sample.

-
19N.1.SL.TZ0.T_13a:
State whether the weight of the eggs is a continuous or discrete variable.
-
19N.1.SL.TZ0.T_13b:
Write down the modal grade of the eggs.
-
19N.1.SL.TZ0.T_13c:
Use your graphic display calculator to find an estimate for the standard deviation of the weight of the eggs.
-
19N.1.SL.TZ0.T_13d:
The mean weight of these eggs is 64.9 grams, correct to three significant figures.
Use the table and your answer to part (c) to find the smallest possible number of eggs that could be within one standard deviation of the mean.
-
19N.1.SL.TZ0.T_15a:
Write down the median rehearsal time.
-
19N.1.SL.TZ0.T_15b:
State whether Stephen is correct. Give a reason for your answer.
-
19N.1.SL.TZ0.T_15c:
On days, Stephen practiced exactly minutes.
Find the possible values of .
-
19N.2.SL.TZ0.T_1a:
State , the null hypothesis for this test.
-
19N.2.SL.TZ0.T_1b:
Write down the number of degrees of freedom.
-
19N.2.SL.TZ0.T_1c:
Show that the expected number of children who chose shrimp is , correct to two significant figures.
-
19N.2.SL.TZ0.T_1d.i:
the statistic.
-
19N.2.SL.TZ0.T_1d.ii:
the -value.
-
19N.2.SL.TZ0.T_1e:
State the conclusion for this test. Give a reason for your answer.
-
19N.2.SL.TZ0.T_1f.i:
Calculate the probability that the customer is an adult.
-
19N.2.SL.TZ0.T_1f.ii:
Calculate the probability that the customer is an adult or that the customer chose shrimp.
-
19N.2.SL.TZ0.T_1f.iii:
Given that the customer is a child, calculate the probability that they chose pasta or fish.
-
20N.1.SL.TZ0.S_8a:
Find the value of .
-
20N.1.SL.TZ0.S_8b:
Write down the value of the median distance in kilometres (km).
-
20N.1.SL.TZ0.S_8c:
Find the value of .
-
20N.1.SL.TZ0.S_8d:
Find .
-
20N.1.SL.TZ0.S_8e:
The first athletes that completed the race won a prize.
Given that an athlete took between and minutes to complete the race, calculate the probability that they won a prize.
-
20N.1.SL.TZ0.T_3a:
Write down the modal group for these data.
-
20N.1.SL.TZ0.T_3b:
Use your graphic display calculator to find an estimate of the standard deviation of the weights of mangoes from this harvest.
-
20N.1.SL.TZ0.T_3c:
On the grid below, draw a histogram for the data in the table.
-
EXN.1.SL.TZ0.4a:
Find the number of potatoes in the sample with a weight of more than grams.
-
EXN.1.SL.TZ0.4b.i:
Find the median weight.
-
EXN.1.SL.TZ0.4b.ii:
Find the lower quartile.
-
EXN.1.SL.TZ0.4b.iii:
Find the upper quartile.
-
EXN.1.SL.TZ0.4c:
The weight of the smallest potato in the sample is grams and the weight of the largest is grams.
Use the scale shown below to draw a box and whisker diagram showing the distribution of the weights of the potatoes. You may assume there are no outliers.
-
21M.2.SL.TZ1.1b:
Write down the median time to read the book.
-
21M.2.SL.TZ1.1c:
Calculate the interquartile range.
-
21M.1.SL.TZ2.5a.i:
the minimum number of sick days taken during the year.
-
21M.1.SL.TZ2.5a.ii:
the lower quartile.
-
21M.1.SL.TZ2.5a.iii:
the median.
-
21M.1.SL.TZ2.5b:
Paul claims that this box and whisker diagram can be used to infer that the percentage of employees who took fewer than six sick days is smaller than the percentage of employees who took more than eleven sick days.
State whether Paul is correct. Justify your answer.
-
21N.1.SL.TZ0.9a:
Find the median of the scores obtained.
-
21N.1.SL.TZ0.9b:
Find an expression for in terms of .
-
21N.1.SL.TZ0.9c.i:
Find the number of students who obtained a grade .
-
21N.1.SL.TZ0.9c.ii:
Find the minimum score needed to obtain a grade .
-
22M.1.SL.TZ2.7b:
With reference to the box and whisker diagrams, state one aspect that may support the researcher’s opinion and one aspect that may counter it.
-
22M.2.SL.TZ2.1a.i:
median reaction time.
-
22M.2.SL.TZ2.1a.ii:
interquartile range of the reaction times.
-
22M.2.SL.TZ2.1b:
Find the estimated number of teenagers who have a reaction time greater than seconds.
-
22M.2.SL.TZ2.1c:
Determine the percentile of the reaction times from the cumulative frequency graph.
-
22M.2.SL.TZ2.1d.i:
Write down the value of .
-
22M.2.SL.TZ2.1d.ii:
Write down the value of .
SL 4.3—Mean, median, mode. Mean of grouped data, standard deviation. Quartiles, IQR
-
SPM.1.SL.TZ0.3a:
State whether the data is discrete or continuous.
-
SPM.1.SL.TZ0.3b:
Find the value of .
-
SPM.1.SL.TZ0.3c:
It was not possible to ask every person in the school, so the Headmaster arranged the student names in alphabetical order and then asked every 10th person on the list.
Identify the sampling technique used in the survey.
-
18M.1.AHL.TZ2.H_3a:
Find the value of p.
-
18M.1.AHL.TZ2.H_3b.i:
Find μ, the expected value of X.
-
18M.1.AHL.TZ2.H_3b.ii:
Find P(X > μ).
-
18N.2.AHL.TZ0.H_10a.i:
Using this distribution model, find .
-
18N.2.AHL.TZ0.H_10a.ii:
Using this distribution model, find the standard deviation of .
-
18N.2.AHL.TZ0.H_10b.i:
an estimate for the mean number of emails received per working day.
-
18N.2.AHL.TZ0.H_10b.ii:
an estimate for the standard deviation of the number of emails received per working day.
-
18N.2.AHL.TZ0.H_10c:
Give one piece of evidence that suggests Willow’s Poisson distribution model is not a good fit.
-
18N.2.AHL.TZ0.H_10d:
Suppose that the probability of Archie receiving more than 10 emails in total on any one day is 0.99. Find the value of λ.
-
18N.2.AHL.TZ0.H_10e:
Now suppose that Archie received exactly 20 emails in total in a consecutive two day period. Show that the probability that he received exactly 10 of them on the first day is independent of λ.
-
16N.2.AHL.TZ0.H_11a:
Show that and .
-
16N.2.AHL.TZ0.H_11b:
Find the values of the constants and .
-
16N.2.AHL.TZ0.H_11c:
Deduce that for .
-
16N.2.AHL.TZ0.H_11d:
(i) Hence show that has two modes and .
(ii) State the values of and .
-
16N.2.AHL.TZ0.H_11e:
Determine the minimum value of such that the probability Kati receives at least one free gift is greater than 0.5.
-
19M.2.AHL.TZ1.H_3b.ii:
the standard deviation.
-
16N.2.SL.TZ0.S_8a:
Find the mean number of hours spent browsing the Internet.
-
16N.2.SL.TZ0.S_8b:
During week 2, the students worked on a major project and they each spent an additional five hours browsing the Internet. For week 2, write down
(i) the mean;
(ii) the standard deviation.
-
16N.2.SL.TZ0.S_8c:
During week 3 each student spent 5% less time browsing the Internet than during week 1. For week 3, find
(i) the median;
(ii) the variance.
-
16N.2.SL.TZ0.S_8d:
(i) Find the number of students who spent between 25 and 30 hours browsing the Internet.
(ii) Given that 10% of the students spent more than k hours browsing the Internet, find the maximum value of .
-
18M.1.SL.TZ1.S_2a:
Find the value of the interquartile range.
-
18M.1.SL.TZ1.S_2b:
One student sent k text messages, where k > 11 . Given that k is an outlier, find the least value of k.
-
17M.1.SL.TZ2.S_8a.ii:
Write down the number of employees who worked 50 hours or less.
-
17M.1.SL.TZ2.S_8b.i:
Find the amount of money an employee earned for working 40 hours;
-
18M.2.SL.TZ1.T_2a:
State the alternative hypothesis.
-
18M.2.SL.TZ1.T_2b:
Calculate the expected frequency of flights travelling at most 500 km and arriving slightly delayed.
-
18M.2.SL.TZ1.T_2c:
Write down the number of degrees of freedom.
-
18M.2.SL.TZ1.T_2d.i:
Write down the χ2 statistic.
-
18M.2.SL.TZ1.T_2d.ii:
Write down the associated p-value.
-
18M.2.SL.TZ1.T_2e:
State, with a reason, whether you would reject the null hypothesis.
-
18M.2.SL.TZ1.T_2f:
Write down the probability that this flight arrived on time.
-
18M.2.SL.TZ1.T_2g:
Given that this flight was not heavily delayed, find the probability that it travelled between 500 km and 5000 km.
-
18M.2.SL.TZ1.T_2h:
Two flights are chosen at random from those which were slightly delayed.
Find the probability that each of these flights travelled at least 5000 km.
-
17M.1.SL.TZ2.T_7a:
Write down an equation, in terms of and , for the total number of times the die was rolled.
-
17M.1.SL.TZ2.T_7b:
Using the mean score, write down a second equation in terms of and .
-
17M.1.SL.TZ2.T_7c:
Find the value of and of .
-
18M.1.SL.TZ1.T_6c.i:
Write down the mid-interval value for the 100 < x ≤ 150 group.
-
18M.1.SL.TZ2.T_12a:
Write down the mid-interval value for 10 ≤ t < 15.
-
18M.1.SL.TZ2.T_12b.i:
Write down the total number of customers in terms of k.
-
17N.2.SL.TZ0.T_1a:
State whether is a discrete or a continuous variable.
-
17N.2.SL.TZ0.T_1b.i:
Write down, for , the modal class;
-
17N.2.SL.TZ0.T_1b.ii:
Write down, for , the mid-interval value of the modal class.
-
17N.2.SL.TZ0.T_1c.i:
Use your graphic display calculator to estimate the mean of ;
-
17N.2.SL.TZ0.T_1c.ii:
Use your graphic display calculator to estimate the standard deviation of .
-
17N.2.SL.TZ0.T_1d:
Find the expected frequency of students choosing the Science category and obtaining 31 to 40 correct answers.
-
17N.2.SL.TZ0.T_1e.i:
Write down the null hypothesis for this test;
-
17N.2.SL.TZ0.T_1e.ii:
Write down the number of degrees of freedom.
-
17N.2.SL.TZ0.T_1f.i:
Write down the -value for the test;
-
17N.2.SL.TZ0.T_1f.ii:
Write down the statistic.
-
17N.2.SL.TZ0.T_1g:
State the result of the test. Give a reason for your answer.
-
17N.1.SL.TZ0.T_1a.ii:
For the students in this group write down the median age.
-
17N.1.SL.TZ0.T_1b:
Draw a box-and-whisker diagram, for these students’ ages, on the following grid.

-
18M.2.SL.TZ2.T_2a:
Find the number of buses that travelled a distance between 15000 and 20000 kilometres.
-
18M.2.SL.TZ2.T_2b.i:
Use the cumulative frequency curve to find the median distance.
-
18M.2.SL.TZ2.T_2b.ii:
Use the cumulative frequency curve to find the lower quartile.
-
18M.2.SL.TZ2.T_2b.iii:
Use the cumulative frequency curve to find the upper quartile.
-
18M.2.SL.TZ2.T_2c:
Hence write down the interquartile range.
-
18M.2.SL.TZ2.T_2d:
Write down the percentage of buses that travelled a distance greater than the upper quartile.
-
18M.2.SL.TZ2.T_2e:
Find the number of buses that travelled a distance less than or equal to 12 000 km.
-
18M.2.SL.TZ2.T_2f:
Find the value of m.
-
18M.2.SL.TZ2.T_2g:
The smallest distance travelled by one of the buses was 2500 km.
The longest distance travelled by one of the buses was 23 000 km.On graph paper, draw a box-and-whisker diagram for these data. Use a scale of 2 cm to represent 5000 km.
-
19M.1.SL.TZ2.T_6c:
The health inspector visits two school cafeterias. She inspects the same number of meals at each cafeteria. The data is shown in the following box-and-whisker diagrams.
Meals prepared in the school cafeterias are required to have less than 10 grams of sugar.
State, giving a reason, which school cafeteria has more meals that do not meet the requirement.
-
16N.1.SL.TZ0.T_2b:
Draw a box-and-whisker diagram on the grid below to represent the Vitamin C content, in milligrams, for this sample.

-
18M.1.SL.TZ1.T_2a:
Find the value of x.
-
18M.1.SL.TZ1.T_2b.i:
Find the standard deviation
-
18M.1.SL.TZ1.T_2b.ii:
Find the interquartile range.
-
16N.2.SL.TZ0.T_1a:
On graph paper, draw a scatter diagram for these data. Use a scale of 2 cm to represent 5 hours on the -axis and 2 cm to represent 10 points on the -axis.
-
16N.2.SL.TZ0.T_1b:
(i) , the mean number of hours spent on social media;
(ii) , the mean number of IB Diploma points.
-
16N.2.SL.TZ0.T_1c:
Plot the point on your scatter diagram and label this point M.
-
16N.2.SL.TZ0.T_1e:
Write down the equation of the regression line on for these eight male students.
-
16N.2.SL.TZ0.T_1f:
Draw the regression line, from part (e), on your scatter diagram.
-
16N.2.SL.TZ0.T_1g:
Use the given equation of the regression line to estimate the number of IB Diploma points that this girl obtained.
-
16N.2.SL.TZ0.T_1h:
Write down a reason why this estimate is not reliable.
-
17M.2.SL.TZ1.T_5a.i:
Calculate the mean test grade of the students;
-
17M.2.SL.TZ1.T_5a.ii:
Calculate the standard deviation.
-
17M.2.SL.TZ1.T_5b:
Find the median test grade of the students.
-
17M.2.SL.TZ1.T_5c:
Find the interquartile range.
-
17M.2.SL.TZ1.T_5d:
Find the probability that this student scored a grade 5 or higher.
-
17M.2.SL.TZ1.T_5e:
Given that the first student chosen at random scored a grade 5 or higher, find the probability that both students scored a grade 6.
-
17M.2.SL.TZ1.T_5f.i:
Calculate the probability that a student chosen at random spent at least 90 minutes preparing for the test.
-
17M.2.SL.TZ1.T_5f.ii:
Calculate the expected number of students that spent at least 90 minutes preparing for the test.
-
19M.2.SL.TZ1.T_3a:
Write down the total number of people, from this group, who are pet owners.
-
19M.2.SL.TZ1.T_3b:
Write down the modal number of pets.
-
19M.2.SL.TZ1.T_3c.i:
For these data, write down the median number of pets.
-
19M.2.SL.TZ1.T_3c.ii:
For these data, write down the lower quartile.
-
19M.2.SL.TZ1.T_3c.iii:
For these data, write down the upper quartile.
-
19M.2.SL.TZ1.T_3d:
Write down the ratio of teenagers to non-teenagers in its simplest form.
-
19M.2.SL.TZ1.T_3e.i:
State the null hypothesis.
-
19M.2.SL.TZ1.T_3e.ii:
State the alternative hypothesis.
-
19M.2.SL.TZ1.T_3f:
Write down the number of degrees of freedom for this test.
-
19M.2.SL.TZ1.T_3g:
Calculate the expected number of teenagers that prefer cats.
-
19M.2.SL.TZ1.T_3i:
State the conclusion for this test. Give a reason for your answer.
-
19M.1.SL.TZ2.T_14a.i:
On the following diagram, shade the region representing the probability that the price of a kilogram of tomatoes, chosen at random, will be higher than 3.22 euro.
-
19M.1.SL.TZ2.T_14a.ii:
Find the price that is two standard deviations above the mean price.
-
19M.1.SL.TZ2.T_14b:
Find the probability that the price of a kilogram of tomatoes, chosen at random, will be between 2.00 and 3.00 euro.
-
19M.1.SL.TZ2.T_14c:
To stimulate reasonable pricing, the city offers a free permit to the sellers whose price of a kilogram of tomatoes is in the lowest 20 %.
Find the highest price that a seller can charge and still receive a free permit.
-
19N.1.SL.TZ0.T_13a:
State whether the weight of the eggs is a continuous or discrete variable.
-
19N.1.SL.TZ0.T_13b:
Write down the modal grade of the eggs.
-
19N.1.SL.TZ0.T_13c:
Use your graphic display calculator to find an estimate for the standard deviation of the weight of the eggs.
-
19N.1.SL.TZ0.T_13d:
The mean weight of these eggs is 64.9 grams, correct to three significant figures.
Use the table and your answer to part (c) to find the smallest possible number of eggs that could be within one standard deviation of the mean.
-
20N.1.SL.TZ0.S_8a:
Find the value of .
-
20N.1.SL.TZ0.S_8b:
Write down the value of the median distance in kilometres (km).
-
20N.1.SL.TZ0.S_8c:
Find the value of .
-
20N.1.SL.TZ0.S_8d:
Find .
-
20N.1.SL.TZ0.S_8e:
The first athletes that completed the race won a prize.
Given that an athlete took between and minutes to complete the race, calculate the probability that they won a prize.
-
20N.2.SL.TZ0.S_9a:
Find the probability that it will take Fiona between minutes and minutes to walk to the bus stop.
-
20N.2.SL.TZ0.S_9b:
Find .
-
20N.2.SL.TZ0.S_9c:
Find the probability that the bus journey takes less than minutes.
-
20N.2.SL.TZ0.S_9d:
Find the probability that Fiona will arrive on time.
-
20N.2.SL.TZ0.S_9e:
This year, Fiona will go to school on days.
Calculate the number of days Fiona is expected to arrive on time.
-
20N.1.SL.TZ0.T_3a:
Write down the modal group for these data.
-
20N.1.SL.TZ0.T_3b:
Use your graphic display calculator to find an estimate of the standard deviation of the weights of mangoes from this harvest.
-
20N.1.SL.TZ0.T_3c:
On the grid below, draw a histogram for the data in the table.
-
20N.1.SL.TZ0.T_7a:
Find the height of Flower .
-
20N.1.SL.TZ0.T_7b:
Using this information, write down an equation in and .
-
20N.1.SL.TZ0.T_7c:
Write down a second equation in and .
-
20N.1.SL.TZ0.T_7d.i:
Using your answers to parts (b) and (c), find the height of Flower .
-
20N.1.SL.TZ0.T_7d.ii:
Using your answers to parts (b) and (c), find the height of Flower .
-
21M.1.SL.TZ1.2a:
the mode.
-
21M.1.SL.TZ1.2b:
the mean.
-
21M.1.SL.TZ1.2c:
the standard deviation.
-
21N.1.SL.TZ0.9a:
Find the median of the scores obtained.
-
21N.1.SL.TZ0.9b:
Find an expression for in terms of .
-
21N.1.SL.TZ0.9c.i:
Find the number of students who obtained a grade .
-
21N.1.SL.TZ0.9c.ii:
Find the minimum score needed to obtain a grade .
-
21N.1.AHL.TZ0.14a:
Find the probability that a sack is under its labelled weight.
-
21N.1.AHL.TZ0.14b:
Find the lower quartile of the weights of the sacks of potatoes.
-
21N.1.AHL.TZ0.14c:
The sacks of potatoes are transported in crates. There are sacks in each crate and the weights of the sacks of potatoes are independent of each other.
Find the probability that the total weight of the sacks of potatoes in a crate exceeds .
-
22M.1.SL.TZ2.7b:
With reference to the box and whisker diagrams, state one aspect that may support the researcher’s opinion and one aspect that may counter it.
-
22M.2.SL.TZ1.3a.i:
the upper quartile.
-
22M.2.SL.TZ1.3a.ii:
the interquartile range.
-
22M.2.AHL.TZ1.3b.i:
the mean mark.
-
22M.2.AHL.TZ1.3b.ii:
the standard deviation of the marks.
-
22M.2.SL.TZ2.1a.ii:
interquartile range of the reaction times.
-
22M.2.SL.TZ2.1b:
Find the estimated number of teenagers who have a reaction time greater than seconds.
-
22M.2.SL.TZ2.1c:
Determine the percentile of the reaction times from the cumulative frequency graph.
-
22M.2.SL.TZ2.1d.i:
Write down the value of .
-
22M.2.SL.TZ2.1d.ii:
Write down the value of .
-
22M.2.SL.TZ2.1e:
Write down the modal class from the table.
-
22M.2.SL.TZ2.1f:
Use your graphic display calculator to find an estimate of the mean reaction time.
-
22M.2.SL.TZ2.1g:
Suggest how, if at all, the estimated mean and estimated median reaction times will change if the errors are corrected. Justify your response.
SL 4.4—Pearsons, scatter diagrams, eqn of y on x
-
SPM.2.SL.TZ0.3a.i:
Write down the value of the Pearson’s product–moment correlation coefficient, .
-
SPM.2.SL.TZ0.3a.ii:
Using the value of , interpret the relationship between Stan’s score and Minsun’s score.
-
SPM.2.SL.TZ0.3b:
Write down the equation of the regression line on .
-
SPM.2.SL.TZ0.3c.i:
Use your regression equation from part (b) to estimate Minsun’s score when Stan awards a perfect 10.
-
SPM.2.SL.TZ0.3c.ii:
State whether this estimate is reliable. Justify your answer.
-
SPM.2.SL.TZ0.3d:
Copy and complete the information in the following table.
-
SPM.2.SL.TZ0.3e.i:
Find the value of the Spearman’s rank correlation coefficient, .
-
SPM.2.SL.TZ0.3e.ii:
Comment on the result obtained for .
-
SPM.2.SL.TZ0.3f:
The Commissioner believes Minsun’s score for competitor G is too high and so decreases the score from 9.5 to 9.1.
Explain why the value of the Spearman’s rank correlation coefficient does not change.
-
EXM.1.AHL.TZ0.15a:
Show that .
-
EXM.1.AHL.TZ0.15b:
Find the equation of the regression line of on .
-
EXM.1.AHL.TZ0.15c.i:
find the value of and of .
-
EXM.1.AHL.TZ0.15c.ii:
predict the temperature of the metal rod after 3 minutes.
-
17M.2.SL.TZ1.T_1a:
Find the range of the average body weights for these seven species of mammal.
-
17M.2.SL.TZ1.T_1b.i:
For the data from these seven species calculate , the Pearson’s product–moment correlation coefficient;
-
17M.2.SL.TZ1.T_1b.ii:
For the data from these seven species describe the correlation between the average body weight and the average weight of the brain.
-
17M.2.SL.TZ1.T_1c:
Write down the equation of the regression line on , in the form .
-
17M.2.SL.TZ1.T_1d:
Use your regression line to estimate the average weight of the brain of grey wolves.
-
17M.2.SL.TZ1.T_1e:
Find the percentage error in your estimate in part (d).
-
18N.2.SL.TZ0.T_1a.ii:
Use your graphic display calculator to write down , the mean examination score.
-
18N.2.SL.TZ0.T_1a.iii:
Use your graphic display calculator to write down r , Pearson’s product–moment correlation coefficient.
-
18N.2.SL.TZ0.T_1b.i:
Find the exact value of m and of c for these data.
-
18N.2.SL.TZ0.T_1c.i:
Use the regression line y on x to estimate Jerome’s examination score.
-
18N.2.SL.TZ0.T_1c.ii:
Justify whether it is valid to use the regression line y on x to estimate Jerome’s examination score.
-
18N.3.AHL.TZ0.Hsp_3a.i:
Find the probability that a fish from this lake will have a weight of more than 560 grams.
-
18N.3.AHL.TZ0.Hsp_3a.ii:
The maximum weight a hand net can hold is 6 kg. Find the probability that a catch of 11 fish can be carried in the hand net.
-
18N.3.AHL.TZ0.Hsp_3b.i:
State the distribution of your test statistic, including the parameter.
-
18N.3.AHL.TZ0.Hsp_3b.ii:
Find the p-value for the test.
-
18N.3.AHL.TZ0.Hsp_3b.iii:
State the conclusion of the test, justifying your answer.
-
18N.3.AHL.TZ0.Hsp_3c.i:
State suitable hypotheses for the test.
-
18N.3.AHL.TZ0.Hsp_3c.ii:
Find the product-moment correlation coefficient .
-
18N.3.AHL.TZ0.Hsp_3c.iii:
State the p-value and interpret it in this context.
-
18N.3.AHL.TZ0.Hsp_3d:
Use an appropriate regression line to estimate the weight of a fish with length 360 mm.
-
18M.2.SL.TZ1.S_8a:
Find the value of a and of b.
-
18M.2.SL.TZ1.S_8b:
Use the regression equation to estimate the value of y when x = 3.57.
-
18M.2.SL.TZ1.S_8c:
The relationship between x and y can be modelled using the formula y = kxn, where k ≠ 0 , n ≠ 0 , n ≠ 1.
By expressing ln y in terms of ln x, find the value of n and of k.
-
17N.2.SL.TZ0.S_8a:
Write down the value of and of .
-
17N.2.SL.TZ0.S_8b:
Use this regression line to estimate the monthly honey production from a hive that has 270 bees.
-
17N.2.SL.TZ0.S_8c:
Write down the number of low production hives.
-
17N.2.SL.TZ0.S_8d.i:
Find the value of ;
-
17N.2.SL.TZ0.S_8d.ii:
Find the number of hives that have a high production.
-
17N.2.SL.TZ0.S_8e:
Adam decides to increase the number of bees in each low production hive. Research suggests that there is a probability of 0.75 that a low production hive becomes a regular production hive. Calculate the probability that 30 low production hives become regular production hives.
-
18M.2.SL.TZ2.S_1a.i:
Find the value of a and of b.
-
18M.2.SL.TZ2.S_1a.ii:
Write down the correlation coefficient.
-
18M.2.SL.TZ2.S_1b:
Use your equation to estimate the mean weight of a child that is 1.95 years old.
-
17M.2.SL.TZ2.S_2a.i:
Find the value of and of .
-
17M.2.SL.TZ2.S_2a.ii:
Write down the value of .
-
17M.2.SL.TZ2.S_2b:
Use the regression equation to estimate the number of visitors on a day when the maximum temperature is 15 °C.
-
17M.1.SL.TZ1.S_4a.i:
Write down the independent variable.
-
17M.1.SL.TZ1.S_4a.ii:
Write down the boiling temperature of the liquid.
-
17M.1.SL.TZ1.S_4b:
Jim describes the correlation as very strong. Circle the value below which best represents the correlation coefficient.
-
17M.1.SL.TZ1.S_4c:
Jim’s model is , for . Use his model to predict the decrease in temperature for any 2 minute interval.
-
18N.2.SL.TZ0.S_2b:
Another athlete on this sports team has a hand length of 21.5 cm. Use the regression equation to estimate the height of this athlete.
-
19M.2.SL.TZ2.S_1a.i:
Write down the value of and of .
-
19M.2.SL.TZ2.S_1a.ii:
Find the correlation coefficient.
-
19M.2.SL.TZ2.S_1b:
Use the regression equation to estimate the BMI of an adult man whose waist size is 95 cm.
-
16N.2.SL.TZ0.T_1a:
On graph paper, draw a scatter diagram for these data. Use a scale of 2 cm to represent 5 hours on the -axis and 2 cm to represent 10 points on the -axis.
-
16N.2.SL.TZ0.T_1b:
(i) , the mean number of hours spent on social media;
(ii) , the mean number of IB Diploma points.
-
16N.2.SL.TZ0.T_1c:
Plot the point on your scatter diagram and label this point M.
-
16N.2.SL.TZ0.T_1e:
Write down the equation of the regression line on for these eight male students.
-
16N.2.SL.TZ0.T_1f:
Draw the regression line, from part (e), on your scatter diagram.
-
16N.2.SL.TZ0.T_1g:
Use the given equation of the regression line to estimate the number of IB Diploma points that this girl obtained.
-
16N.2.SL.TZ0.T_1h:
Write down a reason why this estimate is not reliable.
-
18M.1.SL.TZ1.T_4a.i:
For these data, find Pearson’s product-moment correlation coefficient, r.
-
18M.1.SL.TZ1.T_4a.ii:
For these data, find the equation of the regression line y on x.
-
18M.1.SL.TZ1.T_4b:
Using the equation of the regression line, estimate the concentration of dissolved oxygen in the river when the temperature is 18 °C.
-
17N.1.SL.TZ0.T_5a:
Find the mean number of hours that the people surveyed watch television per week.
-
17N.1.SL.TZ0.T_5b:
Draw the regression line on the scatter diagram.
-
17N.1.SL.TZ0.T_5c:
By placing a tick (✔) in the correct box, determine which of the following statements is true:

-
17N.1.SL.TZ0.T_5d:
Diogo is 18 years old. Give a reason why the regression line should not be used to estimate the number of hours Diogo watches television per week.
-
17M.2.SL.TZ2.T_3a:
Draw a scatter diagram for this data. Use a scale of 2 cm for 5000 folders on the horizontal axis and 2 cm for 10 000 Euros on the vertical axis.
-
17M.2.SL.TZ2.T_3b.i:
Write down, for this set of data the mean number of folders produced, ;
-
17M.2.SL.TZ2.T_3b.ii:
Write down, for this set of data the mean production cost, .
-
17M.2.SL.TZ2.T_3c:
Label the point on the scatter diagram.
-
17M.2.SL.TZ2.T_3e:
State a reason why the regression line on is appropriate to model the relationship between these variables.
-
17M.2.SL.TZ2.T_3f:
Use your graphic display calculator to find the equation of the regression line on .
-
17M.2.SL.TZ2.T_3g:
Draw the regression line on on the scatter diagram.
-
17M.2.SL.TZ2.T_3h:
Use the equation of the regression line to estimate the least number of folders that the factory needs to sell in a month to exceed its production cost for that month.
-
18M.1.SL.TZ2.T_1a:
Plot and label the point M on the scatter diagram.
-
18M.1.SL.TZ2.T_1b:
Draw the line of best fit, by eye, on the scatter diagram.
-
18M.1.SL.TZ2.T_1c:
Using your line of best fit, estimate the physics test score for a student with a score of 20 in their mathematics test.
-
19M.2.SL.TZ1.T_1b.i:
Write down, for this set of data the mean temperature difference from 37 °C, .
-
19M.2.SL.TZ1.T_1b.ii:
Write down, for this set of data the mean number of heartbeats per minute, .
-
19M.2.SL.TZ1.T_1c:
Plot and label the point M(, ) on the scatter diagram.
-
19M.2.SL.TZ1.T_1d.i:
Use your graphic display calculator to find the Pearson’s product–moment correlation coefficient, .
-
19M.2.SL.TZ1.T_1d.ii:
Hence describe the correlation between temperature difference from 37 °C and heartbeat.
-
19M.2.SL.TZ1.T_1f:
Draw the regression line on on the scatter diagram.
-
19N.3.AHL.TZ0.Hsp_1a.i:
State suitable hypotheses and to test Peter’s claim, using a two-tailed test.
-
19N.3.AHL.TZ0.Hsp_1a.ii:
Carry out a suitable test at the 5 % significance level. With reference to the -value, state your conclusion in the context of Peter’s claim.
-
19N.3.AHL.TZ0.Hsp_1b:
Peter uses the regression line of on as and calculates that a student with a Mathematics test score of 73 will have a running time of 101 seconds. Comment on the validity of his calculation.
-
19N.1.SL.TZ0.T_6a:
Use your graphic display calculator to find the equation of the regression line on .
-
19N.1.SL.TZ0.T_6b:
Use your regression equation to estimate the cost of a flight from Hong Kong to Tokyo with Galois Airways.
-
19N.1.SL.TZ0.T_6c:
Explain why it is valid to use the regression equation to estimate the airfare between Hong Kong and Tokyo.
-
20N.2.SL.TZ0.S_2a.i:
Find the value of and of .
-
20N.2.SL.TZ0.S_2a.ii:
Write down the correlation coefficient.
-
20N.2.SL.TZ0.S_2b:
Using the regression equation, estimate the number of hot chocolates that Lucy will sell on a day when the maximum temperature is .
-
20N.2.SL.TZ0.T_1a:
On graph paper, draw a scatter diagram to show the results of Don’s investigation. Use a scale of to represent units on the -axis, and to represent units on the -axis.
-
20N.2.SL.TZ0.T_1b.i:
Calculate , the mean wind speed.
-
20N.2.SL.TZ0.T_1b.ii:
Calculate , the mean time to fully charge the robot.
-
20N.2.SL.TZ0.T_1c:
Plot and label the point on your scatter diagram.
-
20N.2.SL.TZ0.T_1d.i:
Calculate , Pearson’s product–moment correlation coefficient.
-
20N.2.SL.TZ0.T_1d.ii:
Describe the correlation between the wind speed and the time to fully charge the robot.
-
20N.2.SL.TZ0.T_1e.i:
Write down the equation of the regression line on , in the form .
-
20N.2.SL.TZ0.T_1e.ii:
Draw this regression line on your scatter diagram.
-
20N.2.SL.TZ0.T_1e.iii:
Hence or otherwise estimate the charging time when the wind speed is .
-
20N.2.SL.TZ0.T_1f:
Don concluded from his investigation: “There is no causation between wind speed and the time to fully charge the robot”.
In the context of the question, briefly explain the meaning of “no causation”.
-
EXN.1.SL.TZ0.8a:
Assuming the data follows a linear model for this period, find the regression line of on for the remaining data.
-
EXN.1.SL.TZ0.8b:
Use your line to find an estimate for the water temperature on the first day of May.
-
EXN.1.SL.TZ0.8c.i:
Explain why your line should not be used to estimate the value of at which the temperature is .
-
EXN.1.SL.TZ0.8c.ii:
Explain in context why your line should not be used to predict the value for December (month ).
-
EXN.1.SL.TZ0.8d:
State a more appropriate model for the water temperature in the lake over an extended period of time. You are not expected to calculate any parameters.
-
EXN.1.AHL.TZ0.12a:
Explain why this graph indicates that is inversely proportional to .
-
EXN.1.AHL.TZ0.12b:
Find the equation of the least squares regression line of against .
-
EXN.1.AHL.TZ0.12c.i:
Use your answer to part (b) to write down the value of to the nearest integer.
-
EXN.1.AHL.TZ0.12c.ii:
Find an expression for in terms of .
-
EXN.2.SL.TZ0.1c:
Which of the correlation coefficients would you recommend is used to assess whether or not there is an association between total number of minutes late and distance from school? Fully justify your answer.
-
21M.2.SL.TZ1.1e:
Describe the correlation.
-
21M.2.SL.TZ1.1f:
Find the percentage score calculated by Jason.
-
21M.2.SL.TZ1.1g:
State whether it is valid to use the regression line on for Jason’s estimate. Give a reason for your answer.
-
21M.3.AHL.TZ2.1c.i:
Calculate the mean annual income for these remaining responses.
-
21M.3.AHL.TZ2.1c.ii:
Determine the value of , Pearson’s product-moment correlation coefficient, for these remaining responses.
-
21M.3.AHL.TZ2.1e.i:
Use Juliet’s data to find the value of and of .
-
21M.3.AHL.TZ2.1e.ii:
Interpret, referring to income and happiness, what the value of represents.
-
21N.1.SL.TZ0.1a:
For this data, find the value of the Pearson’s product-moment correlation coefficient, .
-
21N.1.SL.TZ0.1b:
Comment on your answer to part (a), using the information that Eduardo found.
-
21N.1.SL.TZ0.1c:
Write down the equation of the regression line of on , in the form .
-
21N.1.SL.TZ0.1d:
A 57-year-old male also ran in the race.
Use the equation of the regression line to estimate the time he took to complete the race.
-
21N.1.AHL.TZ0.12a:
Use the data in the second table to find the value of and the value of for the regression line, .
-
21N.1.AHL.TZ0.12b:
Assuming that the model found in part (a) remains valid, estimate the percentage of trees in stock when .
-
21N.3.AHL.TZ0.2a.i:
Find the equation of the regression line of on .
-
21N.3.AHL.TZ0.2a.ii:
Interpret the meaning of parameter in the context of the model.
-
21N.3.AHL.TZ0.2a.iii:
Suggest why Eva’s use of the linear regression equation in this way could be unreliable.
-
21N.3.AHL.TZ0.2b.i:
Find the equation of the least squares quadratic regression curve.
-
21N.3.AHL.TZ0.2b.ii:
Find the value of .
-
21N.3.AHL.TZ0.2b.iii:
Hence, write down a suitable domain for Eva’s function .
-
21N.3.AHL.TZ0.2c:
Show that .
-
21N.3.AHL.TZ0.2d:
By solving the differential equation , show that the general solution is given by , where .
-
21N.3.AHL.TZ0.2e:
Use the general solution from part (d) and the initial condition to predict the value of .
-
21N.3.AHL.TZ0.2f:
Find this new height.
-
21N.3.AHL.TZ0.2g.i:
Show that , where .
-
21N.3.AHL.TZ0.2g.ii:
Use Euler’s method with a step length of minutes to estimate the maximum value of .
-
22M.3.AHL.TZ1.1a.i:
Find the equation of the regression line of on .
-
22M.3.AHL.TZ1.1a.ii:
Write down the value of , Pearson’s product-moment correlation coefficient.
-
22M.3.AHL.TZ1.1f.i:
Use linear regression to estimate the value of and of .
-
22M.2.SL.TZ1.3c:
State whether it would be appropriate for Chester to use the equation of a regression line for on to predict a country’s Eurovision score. Justify your answer.
-
22M.2.AHL.TZ2.4e.i:
.
It is not required to state units for this value.
-
22M.2.AHL.TZ2.4e.ii:
.
It is not required to state units for this value.
SL 4.5—Probability concepts, expected numbers
-
SPM.1.SL.TZ0.13a:
Find the probability that on any given day Mr Burke chooses a female student to answer a question.
-
SPM.1.SL.TZ0.13b:
Find the probability he will choose a female student 8 times.
-
SPM.1.SL.TZ0.13c:
Find the probability he will choose a male student at most 9 times.
-
19M.1.SL.TZ1.T_7a:
Place the numbers and in the correct position on the Venn diagram.
-
19M.1.SL.TZ1.T_7b:
In the table indicate which two of the given statements are true by placing a tick (✔) in the right hand column.
-
19M.1.SL.TZ2.T_11a:
Write down the elements that belong to .
-
19M.1.SL.TZ2.T_11b.i:
Write down the elements that belong to .
-
19M.1.SL.TZ2.T_11b.ii:
Write down .
-
16N.2.SL.TZ0.T_6a:
Write down a formula for , the surface area to be coated.
-
16N.2.SL.TZ0.T_6b:
Express this volume in .
-
16N.2.SL.TZ0.T_6c:
Write down, in terms of and , an equation for the volume of this water container.
-
16N.2.SL.TZ0.T_6d:
Show that .
-
16N.2.SL.TZ0.T_6e:
Find .
-
16N.2.SL.TZ0.T_6f:
Using your answer to part (e), find the value of which minimizes .
-
16N.2.SL.TZ0.T_6g:
Find the value of this minimum area.
-
16N.2.SL.TZ0.T_6h:
Find the least number of cans of water-resistant material that will coat the area in part (g).
-
19M.2.AHL.TZ2.H_3a:
Complete the given probability tree diagram for Iqbal’s three attempts, labelling each branch with the correct probability.
-
19M.2.AHL.TZ2.H_3b:
Calculate the probability that Iqbal passes at least two of the papers he attempts.
-
19M.2.AHL.TZ2.H_3c:
Find the probability that Iqbal passes his third paper, given that he passed only one previous paper.
-
17M.2.AHL.TZ2.H_5a:
Draw a tree diagram to represent this information for the first three days of July.
-
17M.2.AHL.TZ2.H_5b:
Find the probability that the 3rd July is calm.
-
17M.2.AHL.TZ2.H_5c:
Find the probability that the 1st July was calm given that the 3rd July is windy.
-
17M.2.AHL.TZ2.H_1a:
One of the players is chosen at random. Find the probability that this player’s score was 5 or more.
-
17M.2.AHL.TZ2.H_1b:
Calculate the mean score.
-
17N.1.SL.TZ0.S_1a:
Complete the following tree diagram.

-
17N.1.SL.TZ0.S_1b:
Find the probability that exactly one of the selected balls is green.
-
18M.2.SL.TZ1.S_5a:
Find P(A ∩ B′ ).
-
18M.2.SL.TZ1.S_5b:
Given that P((A ∪ B)′ ) = 0.19, find P(A | B′ ).
-
18M.1.SL.TZ2.S_8a:
Copy and complete the following tree diagram.
-
18M.1.SL.TZ2.S_8b:
Find the probability that Pablo leaves home before 07:00 and is late for work.
-
18M.1.SL.TZ2.S_8c:
Find the probability that Pablo is late for work.
-
18M.1.SL.TZ2.S_8d:
Given that Pablo is late for work, find the probability that he left home before 07:00.
-
18M.1.SL.TZ2.S_8e:
Two days next week Pablo will drive to work. Find the probability that he will be late at least once.
-
19M.2.SL.TZ1.S_10a.i:
Find the probability of rolling exactly one red face.
-
19M.2.SL.TZ1.S_10a.ii:
Find the probability of rolling two or more red faces.
-
19M.2.SL.TZ1.S_10b:
Show that, after a turn, the probability that Ted adds exactly $10 to his winnings is .
-
19M.2.SL.TZ1.S_10c.i:
Write down the value of .
-
19M.2.SL.TZ1.S_10c.ii:
Hence, find the value of .
-
19M.2.SL.TZ1.S_10d:
Ted will always have another turn if he expects an increase to his winnings.
Find the least value of for which Ted should end the game instead of having another turn.
-
17M.1.SL.TZ1.S_1a.i:
Find the value of ;
-
17M.1.SL.TZ1.S_1a.ii:
Find the value of .
-
17M.1.SL.TZ1.S_1b:
A girl is selected at random. Find the probability that she takes economics but not history.
-
18N.1.SL.TZ0.S_9a.i:
Find the probability, in terms of , that the game will end on her first draw.
-
18N.1.SL.TZ0.S_9a.ii:
Find the probability, in terms of , that the game will end on her second draw.
-
18N.1.SL.TZ0.S_9b.i:
third draw.
-
18N.1.SL.TZ0.S_9b.ii:
fourth draw.
-
18N.1.SL.TZ0.S_9c:
Hayley plays the game when = 5. She pays $20 to play and can earn money back depending on the number of draws it takes to obtain a blue marble. She earns no money back if she obtains a blue marble on her first draw. Let M be the amount of money that she earns back playing the game. This information is shown in the following table.
Find the value of so that this is a fair game.
-
19M.1.SL.TZ1.S_1a:
Find the value of .
-
19M.1.SL.TZ1.S_1b:
Find the value of .
-
19M.1.SL.TZ1.S_1c:
Find .
-
16N.1.SL.TZ0.S_5a:
Find .
-
16N.1.SL.TZ0.S_5b:
Find .
-
17M.2.SL.TZ2.S_10a.i:
Find .
-
17M.2.SL.TZ2.S_10a.ii:
Find .
-
17M.2.SL.TZ2.S_10b.i:
Write down the probability of drawing three blue marbles.
-
17M.2.SL.TZ2.S_10b.ii:
Explain why the probability of drawing three white marbles is .
-
17M.2.SL.TZ2.S_10b.iii:
The bag contains a total of ten marbles of which are white. Find .
-
17M.2.SL.TZ2.S_10d:
Grant plays the game until he wins two prizes. Find the probability that he wins his second prize on his eighth attempt.
-
17M.2.SL.TZ2.S_B10c:
Jill plays the game nine times. Find the probability that she wins exactly two prizes.
-
17N.1.SL.TZ0.T_7a:
Complete the Venn diagram for these students.

-
17N.1.SL.TZ0.T_7b:
One of the students who joined the sports club is chosen at random. Find the probability that this student joined both clubs.
-
17N.1.SL.TZ0.T_7c:
Determine whether the events and are independent.
-
18M.1.SL.TZ1.T_10a:
Complete the Venn diagram using the given information.
-
18M.1.SL.TZ1.T_10b:
Find the value of x.
-
18M.1.SL.TZ1.T_10c:
Write down the value of .
-
18M.1.SL.TZ2.T_9a.i:
Write down an expression, in set notation, for the shaded region represented by Diagram 1.
-
18M.1.SL.TZ2.T_9a.ii:
Write down an expression, in set notation, for the shaded region represented by Diagram 2.
-
18M.1.SL.TZ2.T_9a.iii:
Write down an expression, in set notation, for the shaded region represented by Diagram 3.
-
18M.1.SL.TZ2.T_9b.i:
Shade, on the Venn diagram, the region represented by the set .
-
18M.1.SL.TZ2.T_9b.ii:
Shade, on the Venn diagram, the region represented by the set .
-
18M.2.SL.TZ2.T_1a.i:
Write down the value of a.
-
18M.2.SL.TZ2.T_1a.ii:
Write down the value of b.
-
18M.2.SL.TZ2.T_1b.i:
Use the tree diagram to find the probability that an employee encountered traffic and was late for work.
-
18M.2.SL.TZ2.T_1b.ii:
Use the tree diagram to find the probability that an employee was late for work.
-
18M.2.SL.TZ2.T_1b.iii:
Use the tree diagram to find the probability that an employee encountered traffic given that they were late for work.
-
18M.2.SL.TZ2.T_1c.i:
Find the value of x.
-
18M.2.SL.TZ2.T_1c.ii:
Find the value of y.
-
18M.2.SL.TZ2.T_1d:
Find the number of employees who, in the last year, did not travel to work by car, bicycle or public transportation.
-
18M.2.SL.TZ2.T_1e:
Find .
-
17M.1.SL.TZ2.T_2a:
Write down an expression, in terms of , for the number of children who play only basketball.
-
17M.1.SL.TZ2.T_2b:
Complete the Venn diagram using the above information.
-
17M.1.SL.TZ2.T_2c:
Find the number of children who play only football.
-
17M.1.SL.TZ2.T_2d:
Write down the value of .
-
16N.1.SL.TZ0.T_3a:
In the table indicate whether the given statements are True or False.

-
16N.1.SL.TZ0.T_3b:
On the Venn diagram, shade the region .
-
17M.1.SL.TZ1.T_4a:
Write down the number of sporting activities offered by the school during its school year.
-
17M.1.SL.TZ1.T_4b:
Determine whether rock-climbing is offered by the school in the fall/autumn trimester.
-
17M.1.SL.TZ1.T_4c.i:
Write down the elements of the set ;
-
17M.1.SL.TZ1.T_4c.ii:
Write down .
-
17M.1.SL.TZ1.T_4d:
Write down, in terms of , and , an expression for the set which contains only archery, baseball, kayaking and surfing.
-
16N.2.SL.TZ0.T_2a:
Draw a Venn diagram to represent the given information, using sets labelled , and .
-
16N.2.SL.TZ0.T_2b:
Show that .
-
16N.2.SL.TZ0.T_2c:
Write down the value of .
-
16N.2.SL.TZ0.T_2d:
Find the probability that this person
(i) went on at most one trip;
(ii) went on the coach trip, given that this person also went on both the helicopter trip and the boat trip.
-
18N.2.SL.TZ0.T_2a.i:
Find the number of students in the school that are taught in Spanish.
-
18N.2.SL.TZ0.T_2a.ii:
Find the number of students in the school that study Mathematics in English.
-
18N.2.SL.TZ0.T_2a.iii:
Find the number of students in the school that study both Biology and Mathematics.
-
18N.2.SL.TZ0.T_2b.i:
Write down .
-
18N.2.SL.TZ0.T_2b.ii:
Write down .
-
18N.2.SL.TZ0.T_2c.i:
Find the probability that this student studies Mathematics.
-
18N.2.SL.TZ0.T_2c.ii:
Find the probability that this student studies neither Biology nor Mathematics.
-
18N.2.SL.TZ0.T_2c.iii:
Find the probability that this student is taught in Spanish, given that the student studies Biology.
-
19N.1.SL.TZ0.T_9a:
Find the probability that Sungwon’s score on her first turn is greater than .
-
19N.1.SL.TZ0.T_9b:
Find the probability that Sungwon scores greater than on both of her first two turns.
-
19N.1.SL.TZ0.T_9c:
Sungwon will play the game for turns.
Find the expected number of times the score on a turn is greater than .
-
19N.2.SL.TZ0.T_1a:
State , the null hypothesis for this test.
-
19N.2.SL.TZ0.T_1b:
Write down the number of degrees of freedom.
-
19N.2.SL.TZ0.T_1c:
Show that the expected number of children who chose shrimp is , correct to two significant figures.
-
19N.2.SL.TZ0.T_1d.i:
the statistic.
-
19N.2.SL.TZ0.T_1d.ii:
the -value.
-
19N.2.SL.TZ0.T_1e:
State the conclusion for this test. Give a reason for your answer.
-
19N.2.SL.TZ0.T_1f.i:
Calculate the probability that the customer is an adult.
-
19N.2.SL.TZ0.T_1f.ii:
Calculate the probability that the customer is an adult or that the customer chose shrimp.
-
19N.2.SL.TZ0.T_1f.iii:
Given that the customer is a child, calculate the probability that they chose pasta or fish.
-
20N.1.SL.TZ0.S_8a:
Find the value of .
-
20N.1.SL.TZ0.S_8b:
Write down the value of the median distance in kilometres (km).
-
20N.1.SL.TZ0.S_8c:
Find the value of .
-
20N.1.SL.TZ0.S_8d:
Find .
-
20N.1.SL.TZ0.S_8e:
The first athletes that completed the race won a prize.
Given that an athlete took between and minutes to complete the race, calculate the probability that they won a prize.
-
20N.2.SL.TZ0.S_9a:
Find the probability that it will take Fiona between minutes and minutes to walk to the bus stop.
-
20N.2.SL.TZ0.S_9b:
Find .
-
20N.2.SL.TZ0.S_9c:
Find the probability that the bus journey takes less than minutes.
-
20N.2.SL.TZ0.S_9d:
Find the probability that Fiona will arrive on time.
-
20N.2.SL.TZ0.S_9e:
This year, Fiona will go to school on days.
Calculate the number of days Fiona is expected to arrive on time.
-
20N.1.SL.TZ0.T_6a.i:
Find the probability that the first ball chosen is labelled .
-
20N.1.SL.TZ0.T_6a.ii:
Find the probability that the first ball chosen is labelled or labelled .
-
20N.1.SL.TZ0.T_6b:
Find the probability that the second ball chosen is labelled , given that the first ball chosen was labelled .
-
20N.1.SL.TZ0.T_6c:
Find the probability that both balls chosen are labelled .
-
21N.1.SL.TZ0.11a:
Write down the value of .
-
21N.1.SL.TZ0.11b:
Find an expression, in terms of , for the probability of a person not having blue eyes and having fair hair.
-
21N.1.SL.TZ0.11c.i:
.
-
21N.1.SL.TZ0.11c.ii:
.
-
21N.2.SL.TZ0.1a.i:
prefers a tablet.
-
21N.2.SL.TZ0.1a.ii:
is years old and prefers a mobile phone.
-
21N.2.SL.TZ0.1a.iii:
prefers a laptop given that they are years old.
-
21N.2.SL.TZ0.1a.iv:
prefers a tablet or is years old.
-
21N.2.SL.TZ0.1b:
State the null and alternative hypotheses.
-
21N.2.SL.TZ0.1c:
Write down the number of degrees of freedom.
-
21N.2.SL.TZ0.1d.i:
Write down the test statistic.
-
21N.2.SL.TZ0.1d.ii:
Write down the -value.
-
21N.2.SL.TZ0.1d.iii:
State the conclusion for the test in context. Give a reason for your answer.
-
21N.2.SL.TZ0.3a.i:
maximum value of .
-
21N.2.SL.TZ0.3a.ii:
minimum value of .
-
21N.2.SL.TZ0.3b.i:
Find the time, in seconds, it takes for the blade to make one complete rotation under these conditions.
-
21N.2.SL.TZ0.3b.ii:
Calculate the angle, in degrees, that the blade turns through in one second.
-
21N.2.SL.TZ0.3c.i:
Write down the amplitude of the function.
-
21N.2.SL.TZ0.3c.ii:
Find the period of the function.
-
21N.2.SL.TZ0.3d:
Sketch the function for , clearly labelling the coordinates of the maximum and minimum points.
-
21N.2.SL.TZ0.3e.i:
Find the height of above the ground when .
-
21N.2.SL.TZ0.3e.ii:
Find the time, in seconds, that point is above a height of , during each complete rotation.
-
21N.2.SL.TZ0.3f.i:
At any given instant, find the probability that point is visible from Tim’s window.
-
21N.2.SL.TZ0.3f.ii:
The wind speed increases. The blades rotate at twice the speed, but still at a constant rate.
At any given instant, find the probability that Tim can see point from his window. Justify your answer.
-
22M.1.SL.TZ2.2a:
Find the probability that a randomly chosen applicant from this group was accepted by the university.
-
22M.1.SL.TZ2.2b:
Find the probability that the applicant applied for the Arts programme.
-
22M.1.SL.TZ2.2c:
Two different applicants are chosen at random from the original group.
Find the probability that both applicants applied to the Arts programme.
-
22M.1.SL.TZ2.5a:
Calculate the expected number of people who will pass this polygraph test.
-
22M.1.SL.TZ2.5b:
Calculate the probability that exactly people will fail this polygraph test.
-
22M.1.SL.TZ2.5c:
Determine the probability that fewer than people will pass this polygraph test.
-
22M.2.SL.TZ1.5c:
Find, to the nearest integer, the expected increase or decrease in the money made by the airline if they decide to sell tickets rather than .
SL 4.6—Combined, mutually exclusive, conditional, independence, prob diagrams
-
SPM.1.SL.TZ0.12a:
Find the exact value of .
-
SPM.1.SL.TZ0.12b:
Calculate the expected score.
-
SPM.1.SL.TZ0.12c:
Jae Hee plays the game twice and adds the two scores together.
Find the probability Jae Hee has a total score of −3.
-
19M.1.SL.TZ1.T_7a:
Place the numbers and in the correct position on the Venn diagram.
-
19M.1.SL.TZ1.T_7b:
In the table indicate which two of the given statements are true by placing a tick (✔) in the right hand column.
-
19M.1.SL.TZ2.T_11a:
Write down the elements that belong to .
-
19M.1.SL.TZ2.T_11b.i:
Write down the elements that belong to .
-
19M.1.SL.TZ2.T_11b.ii:
Write down .
-
16N.2.SL.TZ0.T_6a:
Write down a formula for , the surface area to be coated.
-
16N.2.SL.TZ0.T_6b:
Express this volume in .
-
16N.2.SL.TZ0.T_6c:
Write down, in terms of and , an equation for the volume of this water container.
-
16N.2.SL.TZ0.T_6d:
Show that .
-
16N.2.SL.TZ0.T_6e:
Find .
-
16N.2.SL.TZ0.T_6f:
Using your answer to part (e), find the value of which minimizes .
-
16N.2.SL.TZ0.T_6g:
Find the value of this minimum area.
-
16N.2.SL.TZ0.T_6h:
Find the least number of cans of water-resistant material that will coat the area in part (g).
-
18N.2.AHL.TZ0.H_10a.i:
Using this distribution model, find .
-
18N.2.AHL.TZ0.H_10a.ii:
Using this distribution model, find the standard deviation of .
-
18N.2.AHL.TZ0.H_10b.i:
an estimate for the mean number of emails received per working day.
-
18N.2.AHL.TZ0.H_10b.ii:
an estimate for the standard deviation of the number of emails received per working day.
-
18N.2.AHL.TZ0.H_10c:
Give one piece of evidence that suggests Willow’s Poisson distribution model is not a good fit.
-
18N.2.AHL.TZ0.H_10d:
Suppose that the probability of Archie receiving more than 10 emails in total on any one day is 0.99. Find the value of λ.
-
18N.2.AHL.TZ0.H_10e:
Now suppose that Archie received exactly 20 emails in total in a consecutive two day period. Show that the probability that he received exactly 10 of them on the first day is independent of λ.
-
16N.2.AHL.TZ0.H_11a:
Show that and .
-
16N.2.AHL.TZ0.H_11b:
Find the values of the constants and .
-
16N.2.AHL.TZ0.H_11c:
Deduce that for .
-
16N.2.AHL.TZ0.H_11d:
(i) Hence show that has two modes and .
(ii) State the values of and .
-
16N.2.AHL.TZ0.H_11e:
Determine the minimum value of such that the probability Kati receives at least one free gift is greater than 0.5.
-
19M.2.AHL.TZ2.H_3a:
Complete the given probability tree diagram for Iqbal’s three attempts, labelling each branch with the correct probability.
-
19M.2.AHL.TZ2.H_3b:
Calculate the probability that Iqbal passes at least two of the papers he attempts.
-
19M.2.AHL.TZ2.H_3c:
Find the probability that Iqbal passes his third paper, given that he passed only one previous paper.
-
17M.2.AHL.TZ2.H_5a:
Draw a tree diagram to represent this information for the first three days of July.
-
17M.2.AHL.TZ2.H_5b:
Find the probability that the 3rd July is calm.
-
17M.2.AHL.TZ2.H_5c:
Find the probability that the 1st July was calm given that the 3rd July is windy.
-
17M.2.AHL.TZ2.H_1a:
One of the players is chosen at random. Find the probability that this player’s score was 5 or more.
-
17M.2.AHL.TZ2.H_1b:
Calculate the mean score.
-
17N.2.AHL.TZ0.H_2a:
Find .
-
17N.2.AHL.TZ0.H_2b:
Find .
-
17N.2.AHL.TZ0.H_2c:
Hence show that events and are independent.
-
17M.2.AHL.TZ1.H_1a:
Calculate ;
-
17M.2.AHL.TZ1.H_1b:
Find .
-
18M.2.AHL.TZ1.H_8:
Each of the 25 students in a class are asked how many pets they own. Two students own three pets and no students own more than three pets. The mean and standard deviation of the number of pets owned by students in the class are and respectively.
Find the number of students in the class who do not own a pet.
-
18N.1.AHL.TZ0.H_1a:
By drawing a Venn diagram, or otherwise, find .
-
18N.1.AHL.TZ0.H_1b:
Show that the events and are not independent.
-
16N.1.AHL.TZ0.H_10a:
Show that .
-
16N.1.AHL.TZ0.H_10b:
(i) show that ;
(ii) hence find .
-
19M.2.AHL.TZ1.H_9a.i:
both a sandwich and a cake.
-
19M.2.AHL.TZ1.H_9a.ii:
only a sandwich.
-
19M.2.AHL.TZ1.H_9b.i:
Find the expected number of cakes sold on a typical day.
-
19M.2.AHL.TZ1.H_9b.ii:
Find the probability that more than 100 cakes will be sold on a typical day.
-
19M.2.AHL.TZ1.H_9c.i:
A customer is selected at random. Find the probability that the customer is male and buys a sandwich.
-
19M.2.AHL.TZ1.H_9c.ii:
A female customer is selected at random. Find the probability that she buys a sandwich.
-
18M.2.AHL.TZ1.H_6:
The mean number of squirrels in a certain area is known to be 3.2 squirrels per hectare of woodland. Within this area, there is a 56 hectare woodland nature reserve. It is known that there are currently at least 168 squirrels in this reserve.
Assuming the population of squirrels follow a Poisson distribution, calculate the probability that there are more than 190 squirrels in the reserve.
-
17M.1.AHL.TZ1.H_10a:
Find the value of .
-
17M.1.AHL.TZ1.H_10b.i:
By considering the graph of f write down the mean of ;
-
17M.1.AHL.TZ1.H_10b.ii:
By considering the graph of f write down the median of ;
-
17M.1.AHL.TZ1.H_10b.iii:
By considering the graph of f write down the mode of .
-
17M.1.AHL.TZ1.H_10c.i:
Show that .
-
17M.1.AHL.TZ1.H_10c.ii:
Hence state the interquartile range of .
-
17M.1.AHL.TZ1.H_10d:
Calculate .
-
17N.1.SL.TZ0.S_1a:
Complete the following tree diagram.

-
17N.1.SL.TZ0.S_1b:
Find the probability that exactly one of the selected balls is green.
-
18M.2.SL.TZ1.S_5a:
Find P(A ∩ B′ ).
-
18M.2.SL.TZ1.S_5b:
Given that P((A ∪ B)′ ) = 0.19, find P(A | B′ ).
-
18M.1.SL.TZ2.S_8a:
Copy and complete the following tree diagram.
-
18M.1.SL.TZ2.S_8b:
Find the probability that Pablo leaves home before 07:00 and is late for work.
-
18M.1.SL.TZ2.S_8c:
Find the probability that Pablo is late for work.
-
18M.1.SL.TZ2.S_8d:
Given that Pablo is late for work, find the probability that he left home before 07:00.
-
18M.1.SL.TZ2.S_8e:
Two days next week Pablo will drive to work. Find the probability that he will be late at least once.
-
19M.2.SL.TZ1.S_10a.i:
Find the probability of rolling exactly one red face.
-
19M.2.SL.TZ1.S_10a.ii:
Find the probability of rolling two or more red faces.
-
19M.2.SL.TZ1.S_10b:
Show that, after a turn, the probability that Ted adds exactly $10 to his winnings is .
-
19M.2.SL.TZ1.S_10c.i:
Write down the value of .
-
19M.2.SL.TZ1.S_10c.ii:
Hence, find the value of .
-
19M.2.SL.TZ1.S_10d:
Ted will always have another turn if he expects an increase to his winnings.
Find the least value of for which Ted should end the game instead of having another turn.
-
17M.1.SL.TZ1.S_1a.i:
Find the value of ;
-
17M.1.SL.TZ1.S_1a.ii:
Find the value of .
-
17M.1.SL.TZ1.S_1b:
A girl is selected at random. Find the probability that she takes economics but not history.
-
18N.1.SL.TZ0.S_9a.i:
Find the probability, in terms of , that the game will end on her first draw.
-
18N.1.SL.TZ0.S_9a.ii:
Find the probability, in terms of , that the game will end on her second draw.
-
18N.1.SL.TZ0.S_9b.i:
third draw.
-
18N.1.SL.TZ0.S_9b.ii:
fourth draw.
-
18N.1.SL.TZ0.S_9c:
Hayley plays the game when = 5. She pays $20 to play and can earn money back depending on the number of draws it takes to obtain a blue marble. She earns no money back if she obtains a blue marble on her first draw. Let M be the amount of money that she earns back playing the game. This information is shown in the following table.
Find the value of so that this is a fair game.
-
19M.1.SL.TZ1.S_1a:
Find the value of .
-
19M.1.SL.TZ1.S_1b:
Find the value of .
-
19M.1.SL.TZ1.S_1c:
Find .
-
16N.1.SL.TZ0.S_5a:
Find .
-
16N.1.SL.TZ0.S_5b:
Find .
-
17M.2.SL.TZ2.S_10a.i:
Find .
-
17M.2.SL.TZ2.S_10a.ii:
Find .
-
17M.2.SL.TZ2.S_10b.i:
Write down the probability of drawing three blue marbles.
-
17M.2.SL.TZ2.S_10b.ii:
Explain why the probability of drawing three white marbles is .
-
17M.2.SL.TZ2.S_10b.iii:
The bag contains a total of ten marbles of which are white. Find .
-
17M.2.SL.TZ2.S_10d:
Grant plays the game until he wins two prizes. Find the probability that he wins his second prize on his eighth attempt.
-
17M.2.SL.TZ2.S_B10c:
Jill plays the game nine times. Find the probability that she wins exactly two prizes.
-
17N.2.SL.TZ0.S_4a:
Find the value of .
-
17N.2.SL.TZ0.S_4b:
Write down .
-
17N.2.SL.TZ0.S_4c:
Find .
-
18M.2.SL.TZ1.S_9a:
Find the probability that an orange weighs between 289 g and 310 g.
-
18M.2.SL.TZ1.S_9b.i:
Find the standardized value for 289 g.
-
18M.2.SL.TZ1.S_9b.ii:
Hence, find the value of σ.
-
18M.2.SL.TZ1.S_9c:
To the nearest gram, find the minimum weight of an orange that the grocer will buy.
-
18M.2.SL.TZ1.S_9d:
Find the probability that the grocer buys more than half the oranges in a box selected at random.
-
18M.2.SL.TZ1.S_9e:
The grocer selects two boxes at random.
Find the probability that the grocer buys more than half the oranges in each box.
-
17M.2.SL.TZ1.S_9a:
Find .
-
17M.2.SL.TZ1.S_9b:
Find the value of .
-
17M.2.SL.TZ1.S_9c:
Find .
-
17M.2.SL.TZ1.S_9d:
Given that , find .
-
19M.1.SL.TZ1.S_9a:
Find P(−1.6 < < 2.4). Write your answer in terms of and .
-
19M.1.SL.TZ1.S_9b:
Given that > −1.6, find the probability that z < 2.4 . Write your answer in terms of and .
-
19M.1.SL.TZ1.S_9c:
Write down the standardized value for .
-
19M.1.SL.TZ1.S_9d:
It is also known that P( > 2) = .
Find .
-
19M.2.SL.TZ2.S_9a:
Show that event A and event D are not independent.
-
19M.2.SL.TZ2.S_9b.i:
Find .
-
19M.2.SL.TZ2.S_9b.ii:
Given that all passengers for a flight arrive on time, find the probability that the flight does not depart on time.
-
19M.2.SL.TZ2.S_9c:
Find the value of .
-
19M.2.SL.TZ2.S_9d:
All flights have two pilots. Find the percentage of flights where both pilots flew more than 30 hours last week.
-
18N.2.SL.TZ0.S_9c:
A randomly selected participant has a reaction time greater than 0.65 seconds. Find the probability that the participant is in Group X.
-
18N.2.SL.TZ0.S_9d:
Ten of the participants with reaction times greater than 0.65 are selected at random. Find the probability that at least two of them are in Group X.
-
18M.2.SL.TZ1.T_2a:
State the alternative hypothesis.
-
18M.2.SL.TZ1.T_2b:
Calculate the expected frequency of flights travelling at most 500 km and arriving slightly delayed.
-
18M.2.SL.TZ1.T_2c:
Write down the number of degrees of freedom.
-
18M.2.SL.TZ1.T_2d.i:
Write down the χ2 statistic.
-
18M.2.SL.TZ1.T_2d.ii:
Write down the associated p-value.
-
18M.2.SL.TZ1.T_2e:
State, with a reason, whether you would reject the null hypothesis.
-
18M.2.SL.TZ1.T_2f:
Write down the probability that this flight arrived on time.
-
18M.2.SL.TZ1.T_2g:
Given that this flight was not heavily delayed, find the probability that it travelled between 500 km and 5000 km.
-
18M.2.SL.TZ1.T_2h:
Two flights are chosen at random from those which were slightly delayed.
Find the probability that each of these flights travelled at least 5000 km.
-
18M.1.SL.TZ2.T_7a:
State the number of boys who answered questions in Portuguese.
-
18M.1.SL.TZ2.T_7b:
Find the probability that the boy answered questions in Hindi.
-
19M.1.SL.TZ1.T_5a:
Complete the cumulative frequency table.
-
19M.1.SL.TZ1.T_5b:
Write down the probability that a bouquet of roses sold is not small.
-
19M.1.SL.TZ1.T_5c:
A customer buys a large bouquet.
Find the probability that there are 12 roses in this bouquet.
-
17N.1.SL.TZ0.T_7a:
Complete the Venn diagram for these students.

-
17N.1.SL.TZ0.T_7b:
One of the students who joined the sports club is chosen at random. Find the probability that this student joined both clubs.
-
17N.1.SL.TZ0.T_7c:
Determine whether the events and are independent.
-
18M.1.SL.TZ1.T_10a:
Complete the Venn diagram using the given information.
-
18M.1.SL.TZ1.T_10b:
Find the value of x.
-
18M.1.SL.TZ1.T_10c:
Write down the value of .
-
18M.1.SL.TZ2.T_9a.i:
Write down an expression, in set notation, for the shaded region represented by Diagram 1.
-
18M.1.SL.TZ2.T_9a.ii:
Write down an expression, in set notation, for the shaded region represented by Diagram 2.
-
18M.1.SL.TZ2.T_9a.iii:
Write down an expression, in set notation, for the shaded region represented by Diagram 3.
-
18M.1.SL.TZ2.T_9b.i:
Shade, on the Venn diagram, the region represented by the set .
-
18M.1.SL.TZ2.T_9b.ii:
Shade, on the Venn diagram, the region represented by the set .
-
18M.2.SL.TZ2.T_1a.i:
Write down the value of a.
-
18M.2.SL.TZ2.T_1a.ii:
Write down the value of b.
-
18M.2.SL.TZ2.T_1b.i:
Use the tree diagram to find the probability that an employee encountered traffic and was late for work.
-
18M.2.SL.TZ2.T_1b.ii:
Use the tree diagram to find the probability that an employee was late for work.
-
18M.2.SL.TZ2.T_1b.iii:
Use the tree diagram to find the probability that an employee encountered traffic given that they were late for work.
-
18M.2.SL.TZ2.T_1c.i:
Find the value of x.
-
18M.2.SL.TZ2.T_1c.ii:
Find the value of y.
-
18M.2.SL.TZ2.T_1d:
Find the number of employees who, in the last year, did not travel to work by car, bicycle or public transportation.
-
18M.2.SL.TZ2.T_1e:
Find .
-
17M.1.SL.TZ2.T_2a:
Write down an expression, in terms of , for the number of children who play only basketball.
-
17M.1.SL.TZ2.T_2b:
Complete the Venn diagram using the above information.
-
17M.1.SL.TZ2.T_2c:
Find the number of children who play only football.
-
17M.1.SL.TZ2.T_2d:
Write down the value of .
-
16N.1.SL.TZ0.T_3a:
In the table indicate whether the given statements are True or False.

-
16N.1.SL.TZ0.T_3b:
On the Venn diagram, shade the region .
-
17M.1.SL.TZ1.T_4a:
Write down the number of sporting activities offered by the school during its school year.
-
17M.1.SL.TZ1.T_4b:
Determine whether rock-climbing is offered by the school in the fall/autumn trimester.
-
17M.1.SL.TZ1.T_4c.i:
Write down the elements of the set ;
-
17M.1.SL.TZ1.T_4c.ii:
Write down .
-
17M.1.SL.TZ1.T_4d:
Write down, in terms of , and , an expression for the set which contains only archery, baseball, kayaking and surfing.
-
16N.2.SL.TZ0.T_2a:
Draw a Venn diagram to represent the given information, using sets labelled , and .
-
16N.2.SL.TZ0.T_2b:
Show that .
-
16N.2.SL.TZ0.T_2c:
Write down the value of .
-
16N.2.SL.TZ0.T_2d:
Find the probability that this person
(i) went on at most one trip;
(ii) went on the coach trip, given that this person also went on both the helicopter trip and the boat trip.
-
18N.2.SL.TZ0.T_2a.i:
Find the number of students in the school that are taught in Spanish.
-
18N.2.SL.TZ0.T_2a.ii:
Find the number of students in the school that study Mathematics in English.
-
18N.2.SL.TZ0.T_2a.iii:
Find the number of students in the school that study both Biology and Mathematics.
-
18N.2.SL.TZ0.T_2b.i:
Write down .
-
18N.2.SL.TZ0.T_2b.ii:
Write down .
-
18N.2.SL.TZ0.T_2c.i:
Find the probability that this student studies Mathematics.
-
18N.2.SL.TZ0.T_2c.ii:
Find the probability that this student studies neither Biology nor Mathematics.
-
18N.2.SL.TZ0.T_2c.iii:
Find the probability that this student is taught in Spanish, given that the student studies Biology.
-
19M.1.SL.TZ2.T_5a:
Using the given information, complete the following Venn diagram.
-
19M.1.SL.TZ2.T_5b:
Find the number of surveyed students who did not like any of the three flavours.
-
19M.1.SL.TZ2.T_5c:
A student is chosen at random from the surveyed students.
Find the probability that this student likes kiwi fruit smoothies given that they like mango smoothies.
-
17N.2.SL.TZ0.T_4a:
Find the probability that this person is not allergic to nuts.
-
17N.2.SL.TZ0.T_4b:
Find the probability that both people chosen are not allergic to nuts.
-
17N.2.SL.TZ0.T_4c:
Copy and complete the tree diagram.
-
17N.2.SL.TZ0.T_4d:
Find the probability that this adult is allergic to nuts and the liquid turns blue.
-
17N.2.SL.TZ0.T_4e:
Find the probability that the liquid turns blue.
-
17N.2.SL.TZ0.T_4f:
Find the probability that the tested adult is allergic to nuts given that the liquid turned blue.
-
17N.2.SL.TZ0.T_4g:
Estimate the number of employees, from this 38, who are allergic to nuts.
-
18M.2.SL.TZ1.T_5a:
Write down the probability that Ayako avoids the trap in this wall.
-
18M.2.SL.TZ1.T_5b:
Find the probability that only one of Ayako and Natsuko falls into a trap while attempting to pass through a door in the first wall.
-
18M.2.SL.TZ1.T_5c:
Copy the probability tree diagram and write down the relevant probabilities along the branches.
-
18M.2.SL.TZ1.T_5d.i:
A contestant is chosen at random. Find the probability that this contestant fell into a trap while attempting to pass through a door in the second wall.
-
18M.2.SL.TZ1.T_5d.ii:
A contestant is chosen at random. Find the probability that this contestant fell into a trap.
-
18M.2.SL.TZ1.T_5e:
120 contestants attempted this game.
Find the expected number of contestants who fell into a trap while attempting to pass through a door in the third wall.
-
19M.2.SL.TZ2.T_1a:
Write down the null hypothesis, H0 , for this test.
-
19M.2.SL.TZ2.T_1b:
State the number of degrees of freedom.
-
19M.2.SL.TZ2.T_1c.i:
the expected frequency of female students who chose to take the Chinese class.
-
19M.2.SL.TZ2.T_1d:
State whether or not H0 should be rejected. Justify your statement.
-
19M.2.SL.TZ2.T_1e.i:
Find the probability that the student does not take the Spanish class.
-
19M.2.SL.TZ2.T_1e.ii:
Find the probability that neither of the two students take the Spanish class.
-
19M.2.SL.TZ2.T_1e.iii:
Find the probability that at least one of the two students is female.
-
19M.1.SL.TZ1.T_12a:
Find the probability that both spins are yellow.
-
19M.1.SL.TZ1.T_12b:
Find the probability that at least one of the spins is yellow.
-
19M.1.SL.TZ1.T_12c:
Write down the probability that the second spin is yellow, given that the first spin is blue.
-
17M.1.SL.TZ2.T_10a:
Write down the probability that a light bulb produced by Home Shine is not defective.
-
17M.1.SL.TZ2.T_10b.i:
Find the probability that both light bulbs are not defective.
-
17M.1.SL.TZ2.T_10b.ii:
Find the probability that at least one of Francesco’s light bulbs is defective.
-
17M.1.SL.TZ2.T_10c:
Write down an expression, in terms of , for the probability that at least one of Deborah’s three light bulbs is defective.
-
17M.1.SL.TZ1.T_7a:
Complete the tree diagram.
-
17M.1.SL.TZ1.T_7b:
Find the probability that Sara’s baggage arrives in London.
-
18N.1.SL.TZ0.T_8a:
Complete the tree diagram.
-
18N.1.SL.TZ0.T_8b:
Find the probability that Jorgé chooses a red disc.
-
18N.1.SL.TZ0.T_14a:
Find the value of k.
-
18N.1.SL.TZ0.T_14b:
Find the probability that a randomly chosen student will be accepted by Marron College.
-
18N.1.SL.TZ0.T_14c:
Given that Naomi attends Marron College, find the probability that she achieved a mark of at least 500 on the test.
-
16N.1.SL.TZ0.T_12a:
Complete the tree diagram below.

-
16N.1.SL.TZ0.T_12b:
Find the value of .
-
19N.1.SL.TZ0.T_4a:
Write down .
-
19N.1.SL.TZ0.T_4b:
Complete the following Venn diagram using all elements of .
-
19N.1.SL.TZ0.T_4c:
Write down an element that belongs to .
-
19N.1.SL.TZ0.T_9a:
Find the probability that Sungwon’s score on her first turn is greater than .
-
19N.1.SL.TZ0.T_9b:
Find the probability that Sungwon scores greater than on both of her first two turns.
-
19N.1.SL.TZ0.T_9c:
Sungwon will play the game for turns.
Find the expected number of times the score on a turn is greater than .
-
19N.2.SL.TZ0.T_1a:
State , the null hypothesis for this test.
-
19N.2.SL.TZ0.T_1b:
Write down the number of degrees of freedom.
-
19N.2.SL.TZ0.T_1c:
Show that the expected number of children who chose shrimp is , correct to two significant figures.
-
19N.2.SL.TZ0.T_1d.i:
the statistic.
-
19N.2.SL.TZ0.T_1d.ii:
the -value.
-
19N.2.SL.TZ0.T_1e:
State the conclusion for this test. Give a reason for your answer.
-
19N.2.SL.TZ0.T_1f.i:
Calculate the probability that the customer is an adult.
-
19N.2.SL.TZ0.T_1f.ii:
Calculate the probability that the customer is an adult or that the customer chose shrimp.
-
19N.2.SL.TZ0.T_1f.iii:
Given that the customer is a child, calculate the probability that they chose pasta or fish.
-
20N.1.SL.TZ0.S_1a.i:
Find the value of .
-
20N.1.SL.TZ0.S_1a.ii:
Find the value of .
-
20N.1.SL.TZ0.S_1b:
Find the probability that a randomly selected student from the class plays tennis or volleyball, but not both.
-
20N.2.SL.TZ0.S_9a:
Find the probability that it will take Fiona between minutes and minutes to walk to the bus stop.
-
20N.2.SL.TZ0.S_9b:
Find .
-
20N.2.SL.TZ0.S_9c:
Find the probability that the bus journey takes less than minutes.
-
20N.2.SL.TZ0.S_9d:
Find the probability that Fiona will arrive on time.
-
20N.2.SL.TZ0.S_9e:
This year, Fiona will go to school on days.
Calculate the number of days Fiona is expected to arrive on time.
-
20N.1.SL.TZ0.T_14a:
Complete the values in the tree diagram.
-
20N.1.SL.TZ0.T_14b:
Find the value of .
-
20N.1.SL.TZ0.T_14c:
Given that Andre did not become the champion, find the probability that he lost in the semi-final.
-
20N.1.SL.TZ0.T_6a.i:
Find the probability that the first ball chosen is labelled .
-
20N.1.SL.TZ0.T_6a.ii:
Find the probability that the first ball chosen is labelled or labelled .
-
20N.1.SL.TZ0.T_6b:
Find the probability that the second ball chosen is labelled , given that the first ball chosen was labelled .
-
20N.1.SL.TZ0.T_6c:
Find the probability that both balls chosen are labelled .
-
20N.2.SL.TZ0.T_2a.i:
Find the value of .
-
20N.2.SL.TZ0.T_2a.ii:
Find the value of .
-
20N.2.SL.TZ0.T_2b:
Find the number of students who visited at least two types of main attraction.
-
20N.2.SL.TZ0.T_2c:
Write down the value of .
-
20N.2.SL.TZ0.T_2d.i:
Find the probability that a randomly selected student visited the rollercoasters.
-
20N.2.SL.TZ0.T_2d.ii:
Find the probability that a randomly selected student visited the virtual reality rides.
-
20N.2.SL.TZ0.T_2e:
Hence determine whether the events in parts (d)(i) and (d)(ii) are independent. Justify your reasoning.
-
EXN.1.SL.TZ0.10a:
Write down the probability it rains during Paula’s lunch break.
-
EXN.1.SL.TZ0.10b:
Find the probability it will rain in each of the three hours Paula is working outside.
-
EXN.1.SL.TZ0.10c:
Find the probability it will not rain while Paula is outside.
-
EXN.1.SL.TZ0.10d:
Find the probability it will rain at least once while Paula is outside.
-
EXN.1.AHL.TZ0.6a:
Write down the probability it rains during Paula’s lunch break.
-
EXN.1.AHL.TZ0.6b:
Find the probability it will not rain while Paula is outside.
-
EXN.1.AHL.TZ0.6d:
Given it rains at least once while Paula is outside find the probability that it rains during her lunch hour.
-
EXN.1.AHL.TZ0.6c:
Find the probability it will rain at least once while Paula is outside.
-
21M.2.SL.TZ2.1b.i:
.
-
21M.2.SL.TZ2.1b.ii:
.
-
21M.2.SL.TZ2.1b.iii:
.
-
21M.2.SL.TZ2.1b.iv:
.
-
21M.2.SL.TZ2.1c.i:
will not have the disease and will test positive.
-
21M.2.SL.TZ2.1c.ii:
will test negative.
-
21M.2.SL.TZ2.1c.iii:
has the disease given that they tested negative.
-
21M.2.SL.TZ2.1d:
The medical centre finds the actual number of positive results in their sample is different than predicted by the tree diagram. Explain why this might be the case.
-
21M.2.SL.TZ2.1e:
Draw a Venn diagram to illustrate this information, placing all relevant information on the diagram.
-
21M.2.SL.TZ2.1f:
Find the total number of patients who visited the centre during this day.
-
21M.2.AHL.TZ2.2e:
A cat is selected at random from all cats.
Find the probability that the cat was female, given that its weight was over .
-
21N.1.SL.TZ0.11a:
Write down the value of .
-
21N.1.SL.TZ0.11b:
Find an expression, in terms of , for the probability of a person not having blue eyes and having fair hair.
-
21N.1.SL.TZ0.11c.i:
.
-
21N.1.SL.TZ0.11c.ii:
.
-
21N.2.SL.TZ0.1a.i:
prefers a tablet.
-
21N.2.SL.TZ0.1a.ii:
is years old and prefers a mobile phone.
-
21N.2.SL.TZ0.1a.iii:
prefers a laptop given that they are years old.
-
21N.2.SL.TZ0.1a.iv:
prefers a tablet or is years old.
-
21N.2.SL.TZ0.1b:
State the null and alternative hypotheses.
-
21N.2.SL.TZ0.1c:
Write down the number of degrees of freedom.
-
21N.2.SL.TZ0.1d.i:
Write down the test statistic.
-
21N.2.SL.TZ0.1d.ii:
Write down the -value.
-
21N.2.SL.TZ0.1d.iii:
State the conclusion for the test in context. Give a reason for your answer.
-
21N.2.SL.TZ0.5a.i:
a dart lands less than from .
-
21N.2.SL.TZ0.5a.ii:
a dart lands more than from .
-
21N.2.SL.TZ0.5b:
Find the probability that Arianne throws two consecutive darts that land more than from .
-
21N.2.SL.TZ0.5c:
Find the probability that Arianne does not score a point on a turn of three darts.
-
21N.2.SL.TZ0.5d.i:
Find the probability that Arianne scores at least points in the competition.
-
21N.2.SL.TZ0.5d.ii:
Find the probability that Arianne scores at least points and less than points.
-
21N.2.SL.TZ0.5d.iii:
Given that Arianne scores at least points, find the probability that Arianne scores less than points.
-
21N.2.AHL.TZ0.3a.i:
a dart lands less than from .
-
21N.2.AHL.TZ0.3a.ii:
a dart lands more than from .
-
21N.2.AHL.TZ0.3b:
Find the probability that Arianne throws two consecutive darts that land more than from .
-
21N.2.AHL.TZ0.3c:
Find the probability that Arianne does not score a point on a turn of three darts.
-
21N.2.AHL.TZ0.3d.i:
Find Arianne’s expected score in the competition.
-
21N.2.AHL.TZ0.3d.ii:
Find the probability that Arianne scores at least points in the competition.
-
21N.2.AHL.TZ0.3d.iii:
Find the probability that Arianne scores at least points and less than points.
-
21N.2.AHL.TZ0.3d.iv:
Given that Arianne scores at least points, find the probability that Arianne scores less than points.
-
22M.3.AHL.TZ2.2h:
Show that Jonas’s network satisfies the requirement of there being less than a probability of the network failing after a power surge.
-
22M.1.SL.TZ1.10a:
Complete the tree diagram.
-
22M.1.SL.TZ1.10b:
Find the probability that Karl takes two socks of the same colour.
-
22M.1.SL.TZ1.10c:
Given that Karl has two socks of the same colour find the probability that he has two brown socks.
-
22M.1.SL.TZ2.2a:
Find the probability that a randomly chosen applicant from this group was accepted by the university.
-
22M.1.SL.TZ2.2b:
Find the probability that the applicant applied for the Arts programme.
-
22M.1.SL.TZ2.2c:
Two different applicants are chosen at random from the original group.
Find the probability that both applicants applied to the Arts programme.
-
22M.1.AHL.TZ2.12c:
Find the probability of making an error using the zoologist’s method.
SL 4.7—Discrete random variables
-
SPM.1.SL.TZ0.12a:
Find the exact value of .
-
SPM.1.SL.TZ0.12b:
Calculate the expected score.
-
SPM.1.SL.TZ0.12c:
Jae Hee plays the game twice and adds the two scores together.
Find the probability Jae Hee has a total score of −3.
-
17M.2.AHL.TZ2.H_1a:
One of the players is chosen at random. Find the probability that this player’s score was 5 or more.
-
17M.2.AHL.TZ2.H_1b:
Calculate the mean score.
-
17M.1.AHL.TZ1.H_10a:
Find the value of .
-
17M.1.AHL.TZ1.H_10b.i:
By considering the graph of f write down the mean of ;
-
17M.1.AHL.TZ1.H_10b.ii:
By considering the graph of f write down the median of ;
-
17M.1.AHL.TZ1.H_10b.iii:
By considering the graph of f write down the mode of .
-
17M.1.AHL.TZ1.H_10c.i:
Show that .
-
17M.1.AHL.TZ1.H_10c.ii:
Hence state the interquartile range of .
-
17M.1.AHL.TZ1.H_10d:
Calculate .
-
18M.1.AHL.TZ1.H_3a:
Find the value of a and the value of b.
-
18M.1.AHL.TZ1.H_3b:
Find the expected value of T.
-
18M.2.AHL.TZ1.H_10a:
Show that .
-
18M.2.AHL.TZ1.H_10b:
Find .
-
18M.2.AHL.TZ1.H_10c:
Given that , and that 0.25 < s < 0.4 , find the value of s.
-
17M.2.AHL.TZ2.H_10a:
Show that and .
-
17M.2.AHL.TZ2.H_10b:
Find .
-
17M.2.AHL.TZ2.H_10c:
Find .
-
17M.2.AHL.TZ2.H_10d:
Find the median of .
-
17M.2.AHL.TZ2.H_10e:
Find .
-
17M.2.AHL.TZ2.H_10f:
Find .
-
19M.2.AHL.TZ2.H_10a:
Find the probability that on a randomly selected day, Steffi does not visit Will’s house.
-
19M.2.AHL.TZ2.H_10b:
Copy and complete the probability distribution table for Y.
-
19M.2.AHL.TZ2.H_10c:
Hence find the expected number of times per day that Steffi is fed at Will’s house.
-
19M.2.AHL.TZ2.H_10d:
In any given year of 365 days, the probability that Steffi does not visit Will for at most days in total is 0.5 (to one decimal place). Find the value of .
-
19M.2.AHL.TZ2.H_10e:
Show that the expected number of occasions per year on which Steffi visits Will’s house and is not fed is at least 30.
-
16N.1.AHL.TZ0.H_1:
Find the coordinates of the point of intersection of the planes defined by the equations and .
-
16N.1.AHL.TZ0.H_2a:
Complete the probability distribution table for .

-
16N.1.AHL.TZ0.H_2b:
Find the expected value of .
-
16N.2.AHL.TZ0.H_1a:
Determine the value of .
-
16N.2.AHL.TZ0.H_1b:
Find the value of .
-
19M.2.SL.TZ1.S_10a.i:
Find the probability of rolling exactly one red face.
-
19M.2.SL.TZ1.S_10a.ii:
Find the probability of rolling two or more red faces.
-
19M.2.SL.TZ1.S_10b:
Show that, after a turn, the probability that Ted adds exactly $10 to his winnings is .
-
19M.2.SL.TZ1.S_10c.i:
Write down the value of .
-
19M.2.SL.TZ1.S_10c.ii:
Hence, find the value of .
-
19M.2.SL.TZ1.S_10d:
Ted will always have another turn if he expects an increase to his winnings.
Find the least value of for which Ted should end the game instead of having another turn.
-
18N.1.SL.TZ0.S_9a.i:
Find the probability, in terms of , that the game will end on her first draw.
-
18N.1.SL.TZ0.S_9a.ii:
Find the probability, in terms of , that the game will end on her second draw.
-
18N.1.SL.TZ0.S_9b.i:
third draw.
-
18N.1.SL.TZ0.S_9b.ii:
fourth draw.
-
18N.1.SL.TZ0.S_9c:
Hayley plays the game when = 5. She pays $20 to play and can earn money back depending on the number of draws it takes to obtain a blue marble. She earns no money back if she obtains a blue marble on her first draw. Let M be the amount of money that she earns back playing the game. This information is shown in the following table.
Find the value of so that this is a fair game.
-
17M.2.SL.TZ2.S_10a.i:
Find .
-
17M.2.SL.TZ2.S_10a.ii:
Find .
-
17M.2.SL.TZ2.S_10b.i:
Write down the probability of drawing three blue marbles.
-
17M.2.SL.TZ2.S_10b.ii:
Explain why the probability of drawing three white marbles is .
-
17M.2.SL.TZ2.S_10b.iii:
The bag contains a total of ten marbles of which are white. Find .
-
17M.2.SL.TZ2.S_10d:
Grant plays the game until he wins two prizes. Find the probability that he wins his second prize on his eighth attempt.
-
17M.2.SL.TZ2.S_B10c:
Jill plays the game nine times. Find the probability that she wins exactly two prizes.
-
17N.2.SL.TZ0.S_4a:
Find the value of .
-
17N.2.SL.TZ0.S_4b:
Write down .
-
17N.2.SL.TZ0.S_4c:
Find .
-
18M.2.SL.TZ1.S_2a:
Find the value of k.
-
18M.2.SL.TZ1.S_2b:
Calculate the expected value of the score.
-
18M.2.SL.TZ1.S_2c:
The die is rolled 80 times. On how many rolls would you expect to obtain a three?
-
18M.2.SL.TZ2.S_10a.i:
Write down the value of k.
-
18M.2.SL.TZ2.S_10a.ii:
Show that μ = 106.
-
18M.2.SL.TZ2.S_10b:
Find P(M < 95) .
-
18M.2.SL.TZ2.S_10c:
Find the probability that a bag of apples selected at random contains at most one small apple.
-
18M.2.SL.TZ2.S_10d.i:
Find the expected number of bags in this crate that contain at most one small apple.
-
18M.2.SL.TZ2.S_10d.ii:
Find the probability that at least 48 bags in this crate contain at most one small apple.
-
17M.2.SL.TZ1.S_4a:
Find .
-
17M.2.SL.TZ1.S_4b.i:
Hence, find the probability that exactly students are left handed;
-
17M.2.SL.TZ1.S_4b.ii:
Hence, find the probability that fewer than students are left handed.
-
16N.2.SL.TZ0.S_7a:
Write down the probability that the first disc selected is red.
-
16N.2.SL.TZ0.S_7b:
Let be the number of red discs selected. Find the smallest value of for which .
-
20N.2.SL.TZ0.S_3a:
Find an expression for in terms of .
-
20N.2.SL.TZ0.S_3b.i:
Find the value of which gives the largest value of .
-
20N.2.SL.TZ0.S_3b.ii:
Hence, find the largest value of .
-
EXN.1.SL.TZ0.12a:
Write down the value of .
-
EXN.1.SL.TZ0.12b:
Find the value of .
-
EXN.1.SL.TZ0.12c:
A game is played in which the arrow attached to the centre of the disc is spun and the sector in which the arrow stops is noted. If the arrow stops in sector the player wins points, otherwise they lose points.
Let be the number of points won
Find .
-
EXN.2.SL.TZ0.4a:
Find the probability of scoring a six when rolling the novelty die.
-
21M.1.SL.TZ1.10a:
Complete the table to show the probability distribution of .
-
21M.1.SL.TZ1.10b.i:
a player scores at least in a game.
-
21M.1.SL.TZ1.10b.ii:
a player scores , given that they scored at least .
-
21M.1.SL.TZ1.10c:
Find the expected score of a game.
SL 4.8—Binomial distribution
-
SPM.1.SL.TZ0.13a:
Find the probability that on any given day Mr Burke chooses a female student to answer a question.
-
SPM.1.SL.TZ0.13b:
Find the probability he will choose a female student 8 times.
-
SPM.1.SL.TZ0.13c:
Find the probability he will choose a male student at most 9 times.
-
SPM.1.AHL.TZ0.17a:
Find the probability he will choose a female student 8 times.
-
SPM.1.AHL.TZ0.17b:
The Head of Year, Mrs Smith, decides to select a student at random from the year group to read the notices in assembly. There are 80 students in total in the year group. Mrs Smith calculates the probability of picking a male student 8 times in the first 20 assemblies is 0.153357 correct to 6 decimal places.
Find the number of male students in the year group.
-
EXM.1.SL.TZ0.9:
Six coins are tossed simultaneously 320 times, with the following results.
At the 5% level of significance, test the hypothesis that all the coins are fair.
-
EXM.1.AHL.TZ0.55a.i:
Calculate the mean number of brown eggs in a box.
-
EXM.1.AHL.TZ0.55a.ii:
Hence estimate , the probability that a randomly chosen egg is brown.
-
EXM.1.AHL.TZ0.55b:
By calculating an appropriate statistic, test, at the 5% significance level, whether or not the binomial distribution gives a good fit to these data.
-
EXM.2.AHL.TZ0.28a.i:
State suitable hypotheses for testing this belief.
-
EXM.2.AHL.TZ0.28a.ii:
Calculate the mean of these data and hence estimate the value of .
-
EXM.2.AHL.TZ0.28a.iii:
Calculate an appropriate value of and state your conclusion, using a 1% significance level.
-
EXM.2.AHL.TZ0.28b.i:
Find the significance level of this procedure.
-
EXM.2.AHL.TZ0.28b.ii:
Some time later, the actual value of is 503. Find the probability of a Type II error.
-
19M.1.AHL.TZ1.H_10a.i:
Find the remainder when is divided by .
-
19M.1.AHL.TZ1.H_10a.ii:
Find the remainder when is divided by .
-
19M.1.AHL.TZ1.H_10b:
Prove that has only one real zero.
-
19M.1.AHL.TZ1.H_10c:
Write down the transformation that will transform the graph of onto the graph of .
-
19M.1.AHL.TZ1.H_10d:
The random variable follows a Poisson distribution with a mean of and .
Find the value of .
-
18N.2.AHL.TZ0.H_10a.i:
Using this distribution model, find .
-
18N.2.AHL.TZ0.H_10a.ii:
Using this distribution model, find the standard deviation of .
-
18N.2.AHL.TZ0.H_10b.i:
an estimate for the mean number of emails received per working day.
-
18N.2.AHL.TZ0.H_10b.ii:
an estimate for the standard deviation of the number of emails received per working day.
-
18N.2.AHL.TZ0.H_10c:
Give one piece of evidence that suggests Willow’s Poisson distribution model is not a good fit.
-
18N.2.AHL.TZ0.H_10d:
Suppose that the probability of Archie receiving more than 10 emails in total on any one day is 0.99. Find the value of λ.
-
18N.2.AHL.TZ0.H_10e:
Now suppose that Archie received exactly 20 emails in total in a consecutive two day period. Show that the probability that he received exactly 10 of them on the first day is independent of λ.
-
19M.2.AHL.TZ1.H_9a.i:
both a sandwich and a cake.
-
19M.2.AHL.TZ1.H_9a.ii:
only a sandwich.
-
19M.2.AHL.TZ1.H_9b.i:
Find the expected number of cakes sold on a typical day.
-
19M.2.AHL.TZ1.H_9b.ii:
Find the probability that more than 100 cakes will be sold on a typical day.
-
19M.2.AHL.TZ1.H_9c.i:
A customer is selected at random. Find the probability that the customer is male and buys a sandwich.
-
19M.2.AHL.TZ1.H_9c.ii:
A female customer is selected at random. Find the probability that she buys a sandwich.
-
18M.2.AHL.TZ1.H_6:
The mean number of squirrels in a certain area is known to be 3.2 squirrels per hectare of woodland. Within this area, there is a 56 hectare woodland nature reserve. It is known that there are currently at least 168 squirrels in this reserve.
Assuming the population of squirrels follow a Poisson distribution, calculate the probability that there are more than 190 squirrels in the reserve.
-
17M.2.AHL.TZ2.H_10a:
Show that and .
-
17M.2.AHL.TZ2.H_10b:
Find .
-
17M.2.AHL.TZ2.H_10c:
Find .
-
17M.2.AHL.TZ2.H_10d:
Find the median of .
-
17M.2.AHL.TZ2.H_10e:
Find .
-
17M.2.AHL.TZ2.H_10f:
Find .
-
19M.2.AHL.TZ2.H_10a:
Find the probability that on a randomly selected day, Steffi does not visit Will’s house.
-
19M.2.AHL.TZ2.H_10b:
Copy and complete the probability distribution table for Y.
-
19M.2.AHL.TZ2.H_10c:
Hence find the expected number of times per day that Steffi is fed at Will’s house.
-
19M.2.AHL.TZ2.H_10d:
In any given year of 365 days, the probability that Steffi does not visit Will for at most days in total is 0.5 (to one decimal place). Find the value of .
-
19M.2.AHL.TZ2.H_10e:
Show that the expected number of occasions per year on which Steffi visits Will’s house and is not fed is at least 30.
-
17N.2.AHL.TZ0.H_6a:
Find the probability that Lucca eats at least one banana in a particular day.
-
17N.2.AHL.TZ0.H_6b:
Find the expected number of weeks in the year in which Lucca eats no bananas.
-
17N.1.AHL.TZ0.H_10a:
Show that the probability that Chloe wins the game is .
-
17N.1.AHL.TZ0.H_10b.i:
Determine the mean of X.
-
17N.1.AHL.TZ0.H_10b.ii:
Determine the variance of X.
-
19M.2.AHL.TZ2.H_2a:
Calculate the probability that, on a randomly selected day, Timmy makes a profit.
-
19M.2.AHL.TZ2.H_2b:
The shop is open for 24 days every month.
Calculate the probability that, in a randomly selected month, Timmy makes a profit on between 5 and 10 days (inclusive).
-
18M.2.AHL.TZ2.H_8a:
Find the least possible value of n.
-
18M.2.AHL.TZ2.H_8b:
It is further given that P(X ≤ 1) = 0.09478 correct to 4 significant figures.
Determine the value of n and the value of p.
-
18M.2.AHL.TZ2.H_9a.i:
Find the probability that exactly 4 taxis arrive during T.
-
18M.2.AHL.TZ2.H_9a.ii:
Find the most likely number of taxis that would arrive during T.
-
18M.2.AHL.TZ2.H_9a.iii:
Given that more than 5 taxis arrive during T, find the probability that exactly 7 taxis arrive during T.
-
18M.2.AHL.TZ2.H_9b:
During quiet periods of the day, taxis arrive at a mean rate of 1.3 taxis every 10 minutes.
Find the probability that during a period of 15 minutes, of which the first 10 minutes is busy and the next 5 minutes is quiet, that exactly 2 taxis arrive.
-
16N.2.AHL.TZ0.H_3a:
Show that .
-
16N.2.AHL.TZ0.H_3b:
Given that and , use part (a) to find the value of .
-
17N.2.SL.TZ0.S_8a:
Write down the value of and of .
-
17N.2.SL.TZ0.S_8b:
Use this regression line to estimate the monthly honey production from a hive that has 270 bees.
-
17N.2.SL.TZ0.S_8c:
Write down the number of low production hives.
-
17N.2.SL.TZ0.S_8d.i:
Find the value of ;
-
17N.2.SL.TZ0.S_8d.ii:
Find the number of hives that have a high production.
-
17N.2.SL.TZ0.S_8e:
Adam decides to increase the number of bees in each low production hive. Research suggests that there is a probability of 0.75 that a low production hive becomes a regular production hive. Calculate the probability that 30 low production hives become regular production hives.
-
19M.2.SL.TZ1.S_10a.i:
Find the probability of rolling exactly one red face.
-
19M.2.SL.TZ1.S_10a.ii:
Find the probability of rolling two or more red faces.
-
19M.2.SL.TZ1.S_10b:
Show that, after a turn, the probability that Ted adds exactly $10 to his winnings is .
-
19M.2.SL.TZ1.S_10c.i:
Write down the value of .
-
19M.2.SL.TZ1.S_10c.ii:
Hence, find the value of .
-
19M.2.SL.TZ1.S_10d:
Ted will always have another turn if he expects an increase to his winnings.
Find the least value of for which Ted should end the game instead of having another turn.
-
17M.2.SL.TZ2.S_10a.i:
Find .
-
17M.2.SL.TZ2.S_10a.ii:
Find .
-
17M.2.SL.TZ2.S_10b.i:
Write down the probability of drawing three blue marbles.
-
17M.2.SL.TZ2.S_10b.ii:
Explain why the probability of drawing three white marbles is .
-
17M.2.SL.TZ2.S_10b.iii:
The bag contains a total of ten marbles of which are white. Find .
-
17M.2.SL.TZ2.S_10d:
Grant plays the game until he wins two prizes. Find the probability that he wins his second prize on his eighth attempt.
-
17M.2.SL.TZ2.S_B10c:
Jill plays the game nine times. Find the probability that she wins exactly two prizes.
-
18M.2.SL.TZ1.S_9a:
Find the probability that an orange weighs between 289 g and 310 g.
-
18M.2.SL.TZ1.S_9b.i:
Find the standardized value for 289 g.
-
18M.2.SL.TZ1.S_9b.ii:
Hence, find the value of σ.
-
18M.2.SL.TZ1.S_9c:
To the nearest gram, find the minimum weight of an orange that the grocer will buy.
-
18M.2.SL.TZ1.S_9d:
Find the probability that the grocer buys more than half the oranges in a box selected at random.
-
18M.2.SL.TZ1.S_9e:
The grocer selects two boxes at random.
Find the probability that the grocer buys more than half the oranges in each box.
-
18N.2.SL.TZ0.S_9c:
A randomly selected participant has a reaction time greater than 0.65 seconds. Find the probability that the participant is in Group X.
-
18N.2.SL.TZ0.S_9d:
Ten of the participants with reaction times greater than 0.65 are selected at random. Find the probability that at least two of them are in Group X.
-
18M.2.SL.TZ2.S_10a.i:
Write down the value of k.
-
18M.2.SL.TZ2.S_10a.ii:
Show that μ = 106.
-
18M.2.SL.TZ2.S_10b:
Find P(M < 95) .
-
18M.2.SL.TZ2.S_10c:
Find the probability that a bag of apples selected at random contains at most one small apple.
-
18M.2.SL.TZ2.S_10d.i:
Find the expected number of bags in this crate that contain at most one small apple.
-
18M.2.SL.TZ2.S_10d.ii:
Find the probability that at least 48 bags in this crate contain at most one small apple.
-
17M.2.SL.TZ1.S_4a:
Find .
-
17M.2.SL.TZ1.S_4b.i:
Hence, find the probability that exactly students are left handed;
-
17M.2.SL.TZ1.S_4b.ii:
Hence, find the probability that fewer than students are left handed.
-
16N.2.SL.TZ0.S_7a:
Write down the probability that the first disc selected is red.
-
16N.2.SL.TZ0.S_7b:
Let be the number of red discs selected. Find the smallest value of for which .
-
20N.2.SL.TZ0.T_6a:
Sketch a diagram to represent this information.
-
20N.2.SL.TZ0.T_6b:
Show that .
-
20N.2.SL.TZ0.T_6c.i:
Find the probability that Emlyn plays between and in a game.
-
20N.2.SL.TZ0.T_6c.ii:
Find the probability that Emlyn plays more than in a game.
-
20N.2.SL.TZ0.T_6d:
Find the value of .
-
20N.2.SL.TZ0.T_6e:
Find the probability he plays between and in one game and more than in the other game.
-
20N.2.SL.TZ0.T_6f:
Find the expected number of successful shots Emlyn will make on Monday, based on the results from Saturday and Sunday.
-
20N.2.SL.TZ0.T_6g:
Emlyn claims the results from Saturday and Sunday show that his expected number of successful shots will be more than Johan’s.
Determine if Emlyn’s claim is correct. Justify your reasoning.
-
EXN.2.SL.TZ0.4b:
Find the probability of scoring more than sixes when this die is rolled times.
-
EXN.3.AHL.TZ0.1c.i:
Write down the value of and the value of .
-
EXN.3.AHL.TZ0.1c.ii:
State an assumption that is being made for to be considered as following a binomial distribution.
-
21M.3.AHL.TZ1.2e.ii:
The tests are performed at the significance level.
Assuming that:- there is no correlation between the marks in any of the sections and scores in any of the attributes,
- the outcome of each hypothesis test is independent of the outcome of the other hypothesis tests,
find the probability that at least one of the tests will be significant.
-
21M.3.AHL.TZ1.2e.iii:
The firm obtains a significant result when comparing section of the written assessment and attribute . Interpret this result.
-
21M.2.SL.TZ2.4e:
Ten of the cats are chosen at random. Find the probability that exactly one of them weighs over .
-
21M.2.AHL.TZ2.2d.i:
Find the probability that exactly one of them weighs over .
-
21M.2.AHL.TZ2.2d.ii:
Let be the number of cats weighing over .
Find the variance of .
-
21N.2.SL.TZ0.5a.i:
a dart lands less than from .
-
21N.2.SL.TZ0.5a.ii:
a dart lands more than from .
-
21N.2.SL.TZ0.5b:
Find the probability that Arianne throws two consecutive darts that land more than from .
-
21N.2.SL.TZ0.5c:
Find the probability that Arianne does not score a point on a turn of three darts.
-
21N.2.SL.TZ0.5d.i:
Find the probability that Arianne scores at least points in the competition.
-
21N.2.SL.TZ0.5d.ii:
Find the probability that Arianne scores at least points and less than points.
-
21N.2.SL.TZ0.5d.iii:
Given that Arianne scores at least points, find the probability that Arianne scores less than points.
-
21N.2.AHL.TZ0.3a.i:
a dart lands less than from .
-
21N.2.AHL.TZ0.3a.ii:
a dart lands more than from .
-
21N.2.AHL.TZ0.3b:
Find the probability that Arianne throws two consecutive darts that land more than from .
-
21N.2.AHL.TZ0.3c:
Find the probability that Arianne does not score a point on a turn of three darts.
-
21N.2.AHL.TZ0.3d.i:
Find Arianne’s expected score in the competition.
-
21N.2.AHL.TZ0.3d.ii:
Find the probability that Arianne scores at least points in the competition.
-
21N.2.AHL.TZ0.3d.iii:
Find the probability that Arianne scores at least points and less than points.
-
21N.2.AHL.TZ0.3d.iv:
Given that Arianne scores at least points, find the probability that Arianne scores less than points.
-
21N.2.AHL.TZ0.7a.i:
Write down null and alternative hypotheses for Loreto’s test.
-
21N.2.AHL.TZ0.7a.ii:
Using the data from Loreto’s sample, perform the hypothesis test at a significance level to determine if Loreto should employ extra staff.
-
21N.2.AHL.TZ0.7b.i:
Write down null and alternative hypotheses for this test.
-
21N.2.AHL.TZ0.7b.ii:
Perform the test, clearly stating the conclusion in context.
-
22M.3.AHL.TZ2.2h:
Show that Jonas’s network satisfies the requirement of there being less than a probability of the network failing after a power surge.
-
22M.1.SL.TZ2.5a:
Calculate the expected number of people who will pass this polygraph test.
-
22M.1.SL.TZ2.5b:
Calculate the probability that exactly people will fail this polygraph test.
-
22M.1.SL.TZ2.5c:
Determine the probability that fewer than people will pass this polygraph test.
-
22M.2.SL.TZ1.5a:
The airline sells tickets for this flight. Find the probability that more than passengers arrive to board the flight.
-
22M.2.SL.TZ1.5b.i:
Write down the expected number of passengers who will arrive to board the flight if tickets are sold.
-
22M.2.SL.TZ1.5b.ii:
Find the maximum number of tickets that could be sold if the expected number of passengers who arrive to board the flight must be less than or equal to .
-
22M.2.SL.TZ2.1a.i:
median reaction time.
SL 4.9—Normal distribution and calculations
-
EXM.3.AHL.TZ0.8a.i:
Find unbiased estimates for the population mean.
-
EXM.3.AHL.TZ0.8a.ii:
Find unbiased estimates for the population Variance.
-
EXM.3.AHL.TZ0.8b:
Show that the expected frequency for 20 < ≤ 4 is 31.5 correct to 1 decimal place.
-
EXM.3.AHL.TZ0.8c:
Perform a suitable test, at the 5% significance level, to determine if the scores follow a normal distribution, with the mean and variance found in part (a). You should clearly state your hypotheses, the degrees of freedom, the p-value and your conclusion.
-
EXM.3.AHL.TZ0.8d:
Use the normal distribution model to find the score required to pass.
-
EXM.3.AHL.TZ0.8e:
Perform a suitable test, at the 5% significance level, to determine if there is a difference between the mean scores of males and females. You should clearly state your hypotheses, the p-value and your conclusion.
-
EXM.3.AHL.TZ0.8f:
Perform a suitable test, at the 5% significance level, to determine if it is easier to achieve a distinction on the new exam. You should clearly state your hypotheses, the critical region and your conclusion.
-
EXM.3.AHL.TZ0.8g.i:
Find the probability of making a Type I error.
-
EXM.3.AHL.TZ0.8g.ii:
Given that find the probability of making a Type II error.
-
EXM.1.SL.TZ0.8a:
Copy and complete the table, showing how you arrived at your answers.
-
EXM.1.SL.TZ0.8b:
Test the hypothesis at the 5% level of significance.
-
EXM.1.AHL.TZ0.59a:
Calculate unbiased estimates of the population mean and the population variance.
-
EXM.1.AHL.TZ0.59b.i:
State suitable hypotheses.
-
EXM.1.AHL.TZ0.59b.ii:
Calculate the value of the statistic and state your conclusion using a 10% level of significance.
-
EXM.2.AHL.TZ0.26a:
The scientists wish to investigate the claim that Group B gain weight faster than Group A. Test this claim at the 5% level of significance, noting which hypothesis test you are using. You may assume that the weight gain for each group is normally distributed, with the same variance, and independent from each other.
-
EXM.2.AHL.TZ0.26b:
The data from the two samples above are combined to form a single set of data. The following frequency table gives the observed frequencies for the combined sample. The data has been divided into five intervals.
Test, at the 5% level, whether the combined data can be considered to be a sample from a normal population with a mean of 380.
-
EXM.2.AHL.TZ0.27a.i:
a goodness of fit test (a complete explanation required);
-
EXM.2.AHL.TZ0.27a.ii:
the level of significance of a hypothesis test.
-
EXM.2.AHL.TZ0.27b:
Find the mean and standard deviation of the sample data in the table above. Show how you arrived at your answers.
-
EXM.2.AHL.TZ0.27c:
Most of the expected frequencies have been calculated in the third column. (Frequencies have been rounded to the nearest integer, and frequencies in the first and last classes have been extended to include the rest of the data beyond 15 and 225. Find the values of , and and show how you arrived at your answers.
-
EXM.2.AHL.TZ0.27d:
In order to test for the goodness of fit, the test statistic was calculated to be 1.0847. Show how this was done.
-
EXM.2.AHL.TZ0.27e:
State your hypotheses, critical number, decision rule and conclusion (using a 5% level of significance).
-
19M.2.AHL.TZ2.H_2a:
Calculate the probability that, on a randomly selected day, Timmy makes a profit.
-
19M.2.AHL.TZ2.H_2b:
The shop is open for 24 days every month.
Calculate the probability that, in a randomly selected month, Timmy makes a profit on between 5 and 10 days (inclusive).
-
17M.2.AHL.TZ2.H_3a:
Given that and find the probability that a randomly chosen packet of biscuits is underweight.
-
17M.2.AHL.TZ2.H_3b:
Calculate the new value of giving your answer correct to two decimal places.
-
17M.2.AHL.TZ2.H_3c:
Calculate the new value of .
-
18M.2.AHL.TZ1.H_4a:
Find the probability that a wolf selected at random is at least 5 years old.
-
18M.2.AHL.TZ1.H_4b:
Eight wolves are independently selected at random and their ages recorded.
Find the probability that more than six of these wolves are at least 5 years old.
-
18M.2.AHL.TZ2.H_3a:
Sketch the probability density function for X, and shade the region representing P(μ − 2σ < X < μ + σ).
-
18M.2.AHL.TZ2.H_3b:
Find the value of P(μ − 2σ < X < μ + σ).
-
18M.2.AHL.TZ2.H_3c:
Find the value of k for which P(μ − kσ < X < μ + kσ) = 0.5.
-
17M.2.AHL.TZ1.H_9a:
Find the probability that a runner selected at random will complete the marathon in less than 3 hours.
-
17M.2.AHL.TZ1.H_9b:
Calculate .
-
17M.2.AHL.TZ1.H_9c:
Find the standard deviation of the times taken by female runners.
-
18N.2.AHL.TZ0.H_3a:
Find the value of and the value of .
-
18N.2.AHL.TZ0.H_3b:
Find the probability that a randomly selected Infiglow battery will have a life of at least 15 hours.
-
18N.3.AHL.TZ0.Hsp_3a.i:
Find the probability that a fish from this lake will have a weight of more than 560 grams.
-
18N.3.AHL.TZ0.Hsp_3a.ii:
The maximum weight a hand net can hold is 6 kg. Find the probability that a catch of 11 fish can be carried in the hand net.
-
18N.3.AHL.TZ0.Hsp_3b.i:
State the distribution of your test statistic, including the parameter.
-
18N.3.AHL.TZ0.Hsp_3b.ii:
Find the p-value for the test.
-
18N.3.AHL.TZ0.Hsp_3b.iii:
State the conclusion of the test, justifying your answer.
-
18N.3.AHL.TZ0.Hsp_3c.i:
State suitable hypotheses for the test.
-
18N.3.AHL.TZ0.Hsp_3c.ii:
Find the product-moment correlation coefficient .
-
18N.3.AHL.TZ0.Hsp_3c.iii:
State the p-value and interpret it in this context.
-
18N.3.AHL.TZ0.Hsp_3d:
Use an appropriate regression line to estimate the weight of a fish with length 360 mm.
-
19M.1.AHL.TZ1.H_6a:
.
-
19M.1.AHL.TZ1.H_6b:
.
-
16N.2.AHL.TZ0.H_8a:
Find and .
-
16N.2.AHL.TZ0.H_8b:
Find .
-
17N.2.AHL.TZ0.H_4:
It is given that one in five cups of coffee contain more than 120 mg of caffeine.
It is also known that three in five cups contain more than 110 mg of caffeine.Assume that the caffeine content of coffee is modelled by a normal distribution.
Find the mean and standard deviation of the caffeine content of coffee. -
18M.2.SL.TZ1.S_9a:
Find the probability that an orange weighs between 289 g and 310 g.
-
18M.2.SL.TZ1.S_9b.i:
Find the standardized value for 289 g.
-
18M.2.SL.TZ1.S_9b.ii:
Hence, find the value of σ.
-
18M.2.SL.TZ1.S_9c:
To the nearest gram, find the minimum weight of an orange that the grocer will buy.
-
18M.2.SL.TZ1.S_9d:
Find the probability that the grocer buys more than half the oranges in a box selected at random.
-
18M.2.SL.TZ1.S_9e:
The grocer selects two boxes at random.
Find the probability that the grocer buys more than half the oranges in each box.
-
17M.2.SL.TZ1.S_9a:
Find .
-
17M.2.SL.TZ1.S_9b:
Find the value of .
-
17M.2.SL.TZ1.S_9c:
Find .
-
17M.2.SL.TZ1.S_9d:
Given that , find .
-
19M.1.SL.TZ1.S_9a:
Find P(−1.6 < < 2.4). Write your answer in terms of and .
-
19M.1.SL.TZ1.S_9b:
Given that > −1.6, find the probability that z < 2.4 . Write your answer in terms of and .
-
19M.1.SL.TZ1.S_9c:
Write down the standardized value for .
-
19M.1.SL.TZ1.S_9d:
It is also known that P( > 2) = .
Find .
-
19M.2.SL.TZ2.S_9a:
Show that event A and event D are not independent.
-
19M.2.SL.TZ2.S_9b.i:
Find .
-
19M.2.SL.TZ2.S_9b.ii:
Given that all passengers for a flight arrive on time, find the probability that the flight does not depart on time.
-
19M.2.SL.TZ2.S_9c:
Find the value of .
-
19M.2.SL.TZ2.S_9d:
All flights have two pilots. Find the percentage of flights where both pilots flew more than 30 hours last week.
-
18N.2.SL.TZ0.S_9c:
A randomly selected participant has a reaction time greater than 0.65 seconds. Find the probability that the participant is in Group X.
-
18N.2.SL.TZ0.S_9d:
Ten of the participants with reaction times greater than 0.65 are selected at random. Find the probability that at least two of them are in Group X.
-
18M.2.SL.TZ2.S_10a.i:
Write down the value of k.
-
18M.2.SL.TZ2.S_10a.ii:
Show that μ = 106.
-
18M.2.SL.TZ2.S_10b:
Find P(M < 95) .
-
18M.2.SL.TZ2.S_10c:
Find the probability that a bag of apples selected at random contains at most one small apple.
-
18M.2.SL.TZ2.S_10d.i:
Find the expected number of bags in this crate that contain at most one small apple.
-
18M.2.SL.TZ2.S_10d.ii:
Find the probability that at least 48 bags in this crate contain at most one small apple.
-
17M.1.SL.TZ2.S_3a:
Write down .
-
17M.1.SL.TZ2.S_3b:
Find .
-
17M.1.SL.TZ2.S_3c:
Find .
-
17N.2.SL.TZ0.S_7:
The heights of adult males in a country are normally distributed with a mean of 180 cm and a standard deviation of . 17% of these men are shorter than 168 cm. 80% of them have heights between and 192 cm.
Find the value of .
-
16N.2.SL.TZ0.S_5a:
Given that 5.3% of newborn babies have a low birth weight, find .
-
16N.2.SL.TZ0.S_5b:
A newborn baby has a low birth weight.
Find the probability that the baby weighs at least 2.15 kg.
-
17M.2.SL.TZ1.T_5a.i:
Calculate the mean test grade of the students;
-
17M.2.SL.TZ1.T_5a.ii:
Calculate the standard deviation.
-
17M.2.SL.TZ1.T_5b:
Find the median test grade of the students.
-
17M.2.SL.TZ1.T_5c:
Find the interquartile range.
-
17M.2.SL.TZ1.T_5d:
Find the probability that this student scored a grade 5 or higher.
-
17M.2.SL.TZ1.T_5e:
Given that the first student chosen at random scored a grade 5 or higher, find the probability that both students scored a grade 6.
-
17M.2.SL.TZ1.T_5f.i:
Calculate the probability that a student chosen at random spent at least 90 minutes preparing for the test.
-
17M.2.SL.TZ1.T_5f.ii:
Calculate the expected number of students that spent at least 90 minutes preparing for the test.
-
19M.1.SL.TZ2.T_14a.i:
On the following diagram, shade the region representing the probability that the price of a kilogram of tomatoes, chosen at random, will be higher than 3.22 euro.
-
19M.1.SL.TZ2.T_14a.ii:
Find the price that is two standard deviations above the mean price.
-
19M.1.SL.TZ2.T_14b:
Find the probability that the price of a kilogram of tomatoes, chosen at random, will be between 2.00 and 3.00 euro.
-
19M.1.SL.TZ2.T_14c:
To stimulate reasonable pricing, the city offers a free permit to the sellers whose price of a kilogram of tomatoes is in the lowest 20 %.
Find the highest price that a seller can charge and still receive a free permit.
-
18N.1.SL.TZ0.T_14a:
Find the value of k.
-
18N.1.SL.TZ0.T_14b:
Find the probability that a randomly chosen student will be accepted by Marron College.
-
18N.1.SL.TZ0.T_14c:
Given that Naomi attends Marron College, find the probability that she achieved a mark of at least 500 on the test.
-
18M.1.SL.TZ1.T_13a.i:
Find the probability that a student, chosen at random arrives at least 60 minutes after the school opens.
-
18M.1.SL.TZ1.T_13a.ii:
Find the probability that a student, chosen at random arrives between 45 minutes and 55 minutes after the school opens.
-
18M.1.SL.TZ1.T_13b:
A second school, Mulberry Park, also opens at 08:00 every morning. The arrival times of the students at this school follows exactly the same distribution as Malthouse school.
Given that, on one morning, 15 students arrive at least 60 minutes after the school opens, estimate the number of students at Mulberry Park school.
-
17N.1.SL.TZ0.T_13a:
Find the probability that this applicant took at least 40 minutes to complete the test.
-
17N.1.SL.TZ0.T_13b:
Find the value of .
-
17N.1.SL.TZ0.T_13c:
Estimate the number of applicants who completed the test in less than 25 minutes.
-
17M.1.SL.TZ2.T_11a:
Write down the probability that the mass of one of these corncobs is greater than 400 grams.
-
17M.1.SL.TZ2.T_11b:
Find the value of .
-
17M.1.SL.TZ2.T_11c:
Estimate the interquartile range of the distribution.
-
16N.2.SL.TZ0.T_4a:
Draw a diagram that shows this information.
-
16N.2.SL.TZ0.T_4b:
(i) Find the probability that a box of cereal, chosen at random, is sold.
(ii) Calculate the manufacturer’s expected daily income from these sales.
-
16N.2.SL.TZ0.T_4c:
Calculate the manufacturer’s expected daily recycling cost.
-
16N.2.SL.TZ0.T_4d:
Calculate the value of .
-
18M.2.SL.TZ2.T_3a.i:
Find the probability that a basketball player has a weight that is less than 61 kg.
-
18M.2.SL.TZ2.T_3a.ii:
In a training session there are 40 basketball players.
Find the expected number of players with a weight less than 61 kg in this training session.
-
18M.2.SL.TZ2.T_3b.i:
Sketch a normal curve to represent this probability.
-
18M.2.SL.TZ2.T_3b.ii:
Find the value of q.
-
18M.2.SL.TZ2.T_3c:
Given that P(W > k) = 0.225 , find the value of k.
-
18M.2.SL.TZ2.T_3d.i:
For this test state the null hypothesis.
-
18M.2.SL.TZ2.T_3d.ii:
For this test find the p-value.
-
18M.2.SL.TZ2.T_3e:
State a conclusion for this test. Justify your answer.
-
19M.1.SL.TZ1.T_11a:
In the following table, write down the letter of the corresponding graph next to the given mean and standard deviation.
-
19M.1.SL.TZ1.T_11b:
Find the probability that a suitcase weighs less than 15 kg.
-
19M.1.SL.TZ1.T_11c:
Any suitcase that weighs more than kg is identified as excess baggage.
19.6 % of the suitcases at this airport are identified as excess baggage.Find the value of .
-
19N.1.SL.TZ0.T_12a:
Write down the probability that the runner completed the race in more than minutes.
-
19N.1.SL.TZ0.T_12b:
Calculate the probability that the runner completed the race in less than minutes.
-
19N.1.SL.TZ0.T_12c:
It is known that of the runners took more than minutes and less than minutes to complete the race.
Find the value of .
-
20N.2.SL.TZ0.T_6a:
Sketch a diagram to represent this information.
-
20N.2.SL.TZ0.T_6b:
Show that .
-
20N.2.SL.TZ0.T_6c.i:
Find the probability that Emlyn plays between and in a game.
-
20N.2.SL.TZ0.T_6c.ii:
Find the probability that Emlyn plays more than in a game.
-
20N.2.SL.TZ0.T_6d:
Find the value of .
-
20N.2.SL.TZ0.T_6e:
Find the probability he plays between and in one game and more than in the other game.
-
20N.2.SL.TZ0.T_6f:
Find the expected number of successful shots Emlyn will make on Monday, based on the results from Saturday and Sunday.
-
20N.2.SL.TZ0.T_6g:
Emlyn claims the results from Saturday and Sunday show that his expected number of successful shots will be more than Johan’s.
Determine if Emlyn’s claim is correct. Justify your reasoning.
-
EXN.1.SL.TZ0.3a:
Find the probability that an apple from the tree has a weight greater than grams.
-
EXN.2.AHL.TZ0.3b:
Find the value of and the value of , giving your answers correct to one decimal place.
-
EXN.2.AHL.TZ0.6a:
Find the probability that a melon selected at random will have a mass greater than .
-
21M.1.AHL.TZ1.14a:
Find the mean weight of a bag of apples.
-
21M.1.AHL.TZ1.14b:
Find the standard deviation of the weights of these bags of apples.
-
21M.1.AHL.TZ1.14c:
Find the probability that a bag selected at random weighs more than .
-
21M.2.SL.TZ1.4a.i:
in less than .
-
21M.2.SL.TZ1.4a.ii:
in more than .
-
21M.2.SL.TZ1.4b.i:
and .
-
21M.2.SL.TZ1.4b.ii:
and .
-
21M.2.SL.TZ2.4a:
Sketch a diagram showing the above information.
-
21M.2.SL.TZ2.4b:
Find the proportion of male Persian cats weighing between and .
-
21M.2.SL.TZ2.4c:
Determine the expected number of cats in this group that have a weight of less than .
-
21M.2.SL.TZ2.4d:
It is found that of the cats weigh more than . Estimate the value of .
-
21M.2.AHL.TZ2.2a:
Sketch a diagram showing the above information.
-
21M.2.AHL.TZ2.2b:
Find the proportion of male Persian cats weighing between and .
-
21M.2.AHL.TZ2.2c:
Determine the expected number of cats in this group that have a weight of less than .
-
21M.2.AHL.TZ2.4a:
Write down a transition matrix indicating the annual population movement between clinics.
-
21M.2.AHL.TZ2.4b:
Find a prediction for the ratio of the number of patients Doctor Black will have, compared to Doctor Green, after two years.
-
21M.2.AHL.TZ2.4c:
Find a matrix , with integer elements, such that , where is a diagonal matrix.
-
21M.2.AHL.TZ2.4d:
Hence, show that the long-term transition matrix is given by .
-
21M.2.AHL.TZ2.4e:
Hence, or otherwise, determine the expected ratio of the number of patients Doctor Black would have compared to Doctor Green in the long term.
-
21N.1.AHL.TZ0.14a:
Find the probability that a sack is under its labelled weight.
-
21N.1.AHL.TZ0.14b:
Find the lower quartile of the weights of the sacks of potatoes.
-
21N.1.AHL.TZ0.14c:
The sacks of potatoes are transported in crates. There are sacks in each crate and the weights of the sacks of potatoes are independent of each other.
Find the probability that the total weight of the sacks of potatoes in a crate exceeds .
-
21N.2.SL.TZ0.5a.i:
a dart lands less than from .
-
21N.2.SL.TZ0.5a.ii:
a dart lands more than from .
-
21N.2.SL.TZ0.5b:
Find the probability that Arianne throws two consecutive darts that land more than from .
-
21N.2.SL.TZ0.5c:
Find the probability that Arianne does not score a point on a turn of three darts.
-
21N.2.SL.TZ0.5d.i:
Find the probability that Arianne scores at least points in the competition.
-
21N.2.SL.TZ0.5d.ii:
Find the probability that Arianne scores at least points and less than points.
-
21N.2.SL.TZ0.5d.iii:
Given that Arianne scores at least points, find the probability that Arianne scores less than points.
-
21N.2.AHL.TZ0.3a.i:
a dart lands less than from .
-
21N.2.AHL.TZ0.3a.ii:
a dart lands more than from .
-
21N.2.AHL.TZ0.3b:
Find the probability that Arianne throws two consecutive darts that land more than from .
-
21N.2.AHL.TZ0.3c:
Find the probability that Arianne does not score a point on a turn of three darts.
-
21N.2.AHL.TZ0.3d.i:
Find Arianne’s expected score in the competition.
-
21N.2.AHL.TZ0.3d.ii:
Find the probability that Arianne scores at least points in the competition.
-
21N.2.AHL.TZ0.3d.iii:
Find the probability that Arianne scores at least points and less than points.
-
21N.2.AHL.TZ0.3d.iv:
Given that Arianne scores at least points, find the probability that Arianne scores less than points.
-
22M.1.SL.TZ1.8a:
Write down the percentage of bags that weigh more than .
-
22M.1.SL.TZ1.8b:
Find the probability that a randomly chosen bag is rejected for being underweight.
-
22M.1.SL.TZ1.8c:
A bag that weighs more than grams is rejected by the factory for being overweight. The factory rejects of bags for being overweight.
Find the value of .
-
22M.1.AHL.TZ1.9a:
Find the probability that the company fails the inspection.
-
22M.1.SL.TZ2.10a:
Determine the probability that a Fuji apple selected at random will be a large apple.
-
22M.1.SL.TZ2.10b:
Find the value of .
-
22M.1.AHL.TZ2.12a:
Find the probability of making a Type I error when weighing a male cuttlefish.
-
22M.1.AHL.TZ2.12b:
Find the probability of making a Type II error when weighing a female cuttlefish.
SL 4.10—Spearman’s rank correlation coefficient
-
SPM.2.SL.TZ0.3a.i:
Write down the value of the Pearson’s product–moment correlation coefficient, .
-
SPM.2.SL.TZ0.3a.ii:
Using the value of , interpret the relationship between Stan’s score and Minsun’s score.
-
SPM.2.SL.TZ0.3b:
Write down the equation of the regression line on .
-
SPM.2.SL.TZ0.3c.i:
Use your regression equation from part (b) to estimate Minsun’s score when Stan awards a perfect 10.
-
SPM.2.SL.TZ0.3c.ii:
State whether this estimate is reliable. Justify your answer.
-
SPM.2.SL.TZ0.3d:
Copy and complete the information in the following table.
-
SPM.2.SL.TZ0.3e.i:
Find the value of the Spearman’s rank correlation coefficient, .
-
SPM.2.SL.TZ0.3e.ii:
Comment on the result obtained for .
-
SPM.2.SL.TZ0.3f:
The Commissioner believes Minsun’s score for competitor G is too high and so decreases the score from 9.5 to 9.1.
Explain why the value of the Spearman’s rank correlation coefficient does not change.
-
EXM.1.SL.TZ0.2a:
Calculate Spearman’s rank correlation coefficient for this data.
-
EXM.1.SL.TZ0.2b:
State what conclusion Kayla can make from the answer in part (a).
-
EXM.1.SL.TZ0.3a:
Explain why it is not appropriate to use Pearson’s product moment correlation coefficient to measure the strength of the relationship between and .
-
EXM.1.SL.TZ0.3b:
Explain why it is appropriate to use Spearman’s rank correlation coefficient to measure the strength of the relationship between and .
-
EXM.1.SL.TZ0.3c:
Calculate Spearman’s rank correlation coefficient for this data.
-
EXM.1.SL.TZ0.3d:
State what conclusion Charles can make from the answer in part (c).
-
EXN.2.SL.TZ0.1c:
Which of the correlation coefficients would you recommend is used to assess whether or not there is an association between total number of minutes late and distance from school? Fully justify your answer.
-
EXN.2.AHL.TZ0.3c:
Find the value of the test statistic for this test.
-
EXN.2.AHL.TZ0.3d:
Determine the degrees of freedom for Dana’s test.
-
EXN.2.AHL.TZ0.3e:
It is given that the critical value for this test is .
State the conclusion of the test in context. Use your answer to part (c) to justify your conclusion.
-
21M.2.SL.TZ1.1h:
Copy and complete the information in the following table.
-
21M.2.SL.TZ1.1i.i:
Calculate the value of .
-
21M.2.SL.TZ1.1i.ii:
Interpret your result.
-
21M.3.AHL.TZ1.2c.i:
Without calculation, explain why it might not be appropriate to calculate a correlation coefficient for the whole sample of employees.
-
21M.3.AHL.TZ1.2c.ii:
Find for the seven employees working in the international department.
-
21M.3.AHL.TZ1.2c.iii:
Hence comment on the validity of the written assessment as a measure of the level of performance of employees in this department. Justify your answer.
-
22M.2.SL.TZ1.3d.i:
.
-
22M.2.SL.TZ1.3d.ii:
.
-
22M.2.SL.TZ1.3d.iii:
.
-
22M.2.SL.TZ1.3e.i:
Find the value of the Spearman’s rank correlation coefficient .
-
22M.2.SL.TZ1.3e.ii:
Interpret the value obtained for .
-
22M.2.SL.TZ1.3f:
When calculating the ranks, Chester incorrectly read the Netherlands’ score as . Explain why the value of the Spearman’s rank correlation does not change despite this error.
SL 4.11—Expected, observed, hypotheses, chi squared, gof, t-test
-
SPM.1.SL.TZ0.6a:
State the null hypothesis.
-
SPM.1.SL.TZ0.6b:
Calculate the -value for this test.
-
SPM.1.SL.TZ0.6c:
State, giving a reason, whether the null hypothesis should be accepted.
-
SPM.1.SL.TZ0.9a.i:
State the null hypothesis.
-
SPM.1.SL.TZ0.9a.ii:
State the alternative hypothesis.
-
SPM.1.SL.TZ0.9b:
Calculate the -value for this test.
-
SPM.1.SL.TZ0.9c:
State, giving a reason, whether Ms Calhoun should accept the null hypothesis.
-
SPM.3.AHL.TZ0.1a:
Identify a test that might have been used to verify the null hypothesis that the predictions from the standardized test can be modelled by a normal distribution.
-
SPM.3.AHL.TZ0.1b:
State why comparing only the final IB points of the students from the two schools would not be a valid test for the effectiveness of the two different teaching methods.
-
SPM.3.AHL.TZ0.1c.i:
Find the mean change.
-
SPM.3.AHL.TZ0.1c.ii:
Find the standard deviation of the changes.
-
SPM.3.AHL.TZ0.1d:
Use a paired -test to determine whether there is significant evidence that the students in school A have improved their IB points since the start of the course.
-
SPM.3.AHL.TZ0.1e.i:
Use an appropriate test to determine whether there is evidence, at the 5 % significance level, that the students in school B have improved more than those in school A.
-
SPM.3.AHL.TZ0.1e.ii:
State why it was important to test that both sets of points were normally distributed.
-
SPM.3.AHL.TZ0.1f.i:
Perform a test on the data from school A to show it is reasonable to assume a linear relationship between effort scores and improvements in IB points. You may assume effort scores follow a normal distribution.
-
SPM.3.AHL.TZ0.1f.ii:
Hence, find the expected improvement between predicted and final points for an increase of one unit in effort grades, giving your answer to one decimal place.
-
SPM.3.AHL.TZ0.1g:
Use an appropriate test to determine whether showing an improvement is independent of gender.
-
SPM.3.AHL.TZ0.1h:
If you were to repeat the test performed in part (e) intending to compare the quality of the teaching between the two schools, suggest two ways in which you might choose your sample to improve the validity of the test.
-
EXM.1.SL.TZ0.7a:
State an assumption that Anita is making, in order to use a t-test.
-
EXM.1.SL.TZ0.7b:
State the hypotheses for this t-test.
-
EXM.1.SL.TZ0.7c:
Find the p-value for this t-test.
-
EXM.1.SL.TZ0.7d:
State the conclusion of this test, in context, giving a reason.
-
EXM.2.SL.TZ0.5a:
State the name for this type of sampling technique.
-
EXM.2.SL.TZ0.5b:
Calculate the number of volunteers in the sample under the age of 30.
-
EXM.2.SL.TZ0.5c.i:
The new drug.
-
EXM.2.SL.TZ0.5c.ii:
The current drug.
-
EXM.2.SL.TZ0.5d:
State an assumption that the company is making, in order to use a t-test.
-
EXM.2.SL.TZ0.5e:
State the hypotheses for this t-test.
-
EXM.2.SL.TZ0.5f:
Find the p-value for this t-test.
-
EXM.2.SL.TZ0.5g:
State the conclusion of this test, in context, giving a reason.
-
EXM.3.AHL.TZ0.8a.i:
Find unbiased estimates for the population mean.
-
EXM.3.AHL.TZ0.8a.ii:
Find unbiased estimates for the population Variance.
-
EXM.3.AHL.TZ0.8b:
Show that the expected frequency for 20 < ≤ 4 is 31.5 correct to 1 decimal place.
-
EXM.3.AHL.TZ0.8c:
Perform a suitable test, at the 5% significance level, to determine if the scores follow a normal distribution, with the mean and variance found in part (a). You should clearly state your hypotheses, the degrees of freedom, the p-value and your conclusion.
-
EXM.3.AHL.TZ0.8d:
Use the normal distribution model to find the score required to pass.
-
EXM.3.AHL.TZ0.8e:
Perform a suitable test, at the 5% significance level, to determine if there is a difference between the mean scores of males and females. You should clearly state your hypotheses, the p-value and your conclusion.
-
EXM.3.AHL.TZ0.8f:
Perform a suitable test, at the 5% significance level, to determine if it is easier to achieve a distinction on the new exam. You should clearly state your hypotheses, the critical region and your conclusion.
-
EXM.3.AHL.TZ0.8g.i:
Find the probability of making a Type I error.
-
EXM.3.AHL.TZ0.8g.ii:
Given that find the probability of making a Type II error.
-
EXM.3.AHL.TZ0.9a:
Use an exponential regression to find the value of and of , correct to 4 decimal places.
-
EXM.3.AHL.TZ0.9b.i:
the number of new people infected on day 6.
-
EXM.3.AHL.TZ0.9b.ii:
the day when the total number of people infected will be greater than 1000.
-
EXM.3.AHL.TZ0.9c:
Use your answer to part (a) to show that the model predicts 16.7 people will be infected on the first day.
-
EXM.3.AHL.TZ0.9d.i:
Explain why the number of degrees of freedom is 2.
-
EXM.3.AHL.TZ0.9d.ii:
Perform a goodness of fit test at the 5% significance level. You should clearly state your hypotheses, the p-value, and your conclusion.
-
EXM.3.AHL.TZ0.9e:
Give two reasons why the prediction in part (b)(ii) might be lower than 14.
-
EXM.3.AHL.TZ0.9f.i:
.
-
EXM.3.AHL.TZ0.9f.ii:
.
-
EXM.3.AHL.TZ0.9f.iii:
.
-
EXM.3.AHL.TZ0.9g:
Hence predict the total number of people infected by this disease after several months.
-
EXM.3.AHL.TZ0.9h:
Use the logistic model to find the day when the rate of increase of people infected is greatest.
-
EXM.2.AHL.TZ0.24a:
Show that this data leads to an estimated value of .
-
EXM.2.AHL.TZ0.24b:
Stating null and alternative hypotheses, carry out an appropriate test at the 5 % level to decide whether the farmer’s claim can be justified.
-
EXM.2.SL.TZ0.6a:
Use a chi-squared goodness of fit test to investigate whether or not, at the 5 % level of significance, the N(0, 1) distribution can be used to model these results.
-
EXM.2.SL.TZ0.6b.i:
a Type I error.
-
EXM.2.SL.TZ0.6b.ii:
a Type II error.
-
EXM.1.SL.TZ0.8a:
Copy and complete the table, showing how you arrived at your answers.
-
EXM.1.SL.TZ0.8b:
Test the hypothesis at the 5% level of significance.
-
EXM.1.SL.TZ0.9:
Six coins are tossed simultaneously 320 times, with the following results.
At the 5% level of significance, test the hypothesis that all the coins are fair.
-
EXM.1.SL.TZ0.10a:
Showing all steps clearly, test whether the die is fair
(i) at the 5% level of significance;
(ii) at the 1% level of significance.
-
EXM.1.SL.TZ0.10b:
Explain what is meant by “level of significance” in part (a).
-
EXM.1.SL.TZ0.11a:
Test this claim at the 5% level of significance.
-
EXM.1.SL.TZ0.11b:
Explain what is meant by the 5% level of significance.
-
EXM.1.AHL.TZ0.55a.i:
Calculate the mean number of brown eggs in a box.
-
EXM.1.AHL.TZ0.55a.ii:
Hence estimate , the probability that a randomly chosen egg is brown.
-
EXM.1.AHL.TZ0.55b:
By calculating an appropriate statistic, test, at the 5% significance level, whether or not the binomial distribution gives a good fit to these data.
-
EXM.1.AHL.TZ0.56a:
Calculate the mean number of eggs laid by these birds.
-
EXM.1.AHL.TZ0.56b.i:
Write down appropriate hypotheses.
-
EXM.1.AHL.TZ0.56b.ii:
Carry out a test at the 1% significance level, and state your conclusion.
-
EXM.1.AHL.TZ0.57b:
The number of cars passing a certain point in a road was recorded during 80 equal time intervals and summarized in the table below.
Carry out a goodness of fit test at the 5% significance level to decide if the above data can be modelled by a Poisson distribution.
-
EXM.1.AHL.TZ0.58a:
Find the exact value of the mean of this distribution.
-
EXM.1.AHL.TZ0.58b:
Test, at the 5% level of significance, whether or not the data can be modelled by a Poisson distribution.
-
EXM.1.AHL.TZ0.59a:
Calculate unbiased estimates of the population mean and the population variance.
-
EXM.1.AHL.TZ0.59b.i:
State suitable hypotheses.
-
EXM.1.AHL.TZ0.59b.ii:
Calculate the value of the statistic and state your conclusion using a 10% level of significance.
-
EXM.2.AHL.TZ0.26a:
The scientists wish to investigate the claim that Group B gain weight faster than Group A. Test this claim at the 5% level of significance, noting which hypothesis test you are using. You may assume that the weight gain for each group is normally distributed, with the same variance, and independent from each other.
-
EXM.2.AHL.TZ0.26b:
The data from the two samples above are combined to form a single set of data. The following frequency table gives the observed frequencies for the combined sample. The data has been divided into five intervals.
Test, at the 5% level, whether the combined data can be considered to be a sample from a normal population with a mean of 380.
-
EXM.2.AHL.TZ0.27a.i:
a goodness of fit test (a complete explanation required);
-
EXM.2.AHL.TZ0.27a.ii:
the level of significance of a hypothesis test.
-
EXM.2.AHL.TZ0.27b:
Find the mean and standard deviation of the sample data in the table above. Show how you arrived at your answers.
-
EXM.2.AHL.TZ0.27c:
Most of the expected frequencies have been calculated in the third column. (Frequencies have been rounded to the nearest integer, and frequencies in the first and last classes have been extended to include the rest of the data beyond 15 and 225. Find the values of , and and show how you arrived at your answers.
-
EXM.2.AHL.TZ0.27d:
In order to test for the goodness of fit, the test statistic was calculated to be 1.0847. Show how this was done.
-
EXM.2.AHL.TZ0.27e:
State your hypotheses, critical number, decision rule and conclusion (using a 5% level of significance).
-
EXM.2.AHL.TZ0.28a.i:
State suitable hypotheses for testing this belief.
-
EXM.2.AHL.TZ0.28a.ii:
Calculate the mean of these data and hence estimate the value of .
-
EXM.2.AHL.TZ0.28a.iii:
Calculate an appropriate value of and state your conclusion, using a 1% significance level.
-
EXM.2.AHL.TZ0.28b.i:
Find the significance level of this procedure.
-
EXM.2.AHL.TZ0.28b.ii:
Some time later, the actual value of is 503. Find the probability of a Type II error.
-
EXM.2.AHL.TZ0.29a:
A horse breeder records the number of births for each of 100 horses during the past eight years. The results are summarized in the following table:
Stating null and alternative hypotheses carry out an appropriate test at the 5% significance level to decide whether the results can be modelled by B (6, 0.5).
-
EXM.2.AHL.TZ0.29b:
Without doing any further calculations, explain briefly how you would carry out a test, at the 5% significance level, to decide if the data can be modelled by B(6, ), where is unspecified.
-
EXM.2.AHL.TZ0.29c:
A different horse breeder collected data on the time and outcome of births. The data are summarized in the following table:
Carry out an appropriate test at the 5% significance level to decide whether there is an association between time and outcome.
-
18M.2.SL.TZ1.T_2a:
State the alternative hypothesis.
-
18M.2.SL.TZ1.T_2b:
Calculate the expected frequency of flights travelling at most 500 km and arriving slightly delayed.
-
18M.2.SL.TZ1.T_2c:
Write down the number of degrees of freedom.
-
18M.2.SL.TZ1.T_2d.i:
Write down the χ2 statistic.
-
18M.2.SL.TZ1.T_2d.ii:
Write down the associated p-value.
-
18M.2.SL.TZ1.T_2e:
State, with a reason, whether you would reject the null hypothesis.
-
18M.2.SL.TZ1.T_2f:
Write down the probability that this flight arrived on time.
-
18M.2.SL.TZ1.T_2g:
Given that this flight was not heavily delayed, find the probability that it travelled between 500 km and 5000 km.
-
18M.2.SL.TZ1.T_2h:
Two flights are chosen at random from those which were slightly delayed.
Find the probability that each of these flights travelled at least 5000 km.
-
17N.2.SL.TZ0.T_1a:
State whether is a discrete or a continuous variable.
-
17N.2.SL.TZ0.T_1b.i:
Write down, for , the modal class;
-
17N.2.SL.TZ0.T_1b.ii:
Write down, for , the mid-interval value of the modal class.
-
17N.2.SL.TZ0.T_1c.i:
Use your graphic display calculator to estimate the mean of ;
-
17N.2.SL.TZ0.T_1c.ii:
Use your graphic display calculator to estimate the standard deviation of .
-
17N.2.SL.TZ0.T_1d:
Find the expected frequency of students choosing the Science category and obtaining 31 to 40 correct answers.
-
17N.2.SL.TZ0.T_1e.i:
Write down the null hypothesis for this test;
-
17N.2.SL.TZ0.T_1e.ii:
Write down the number of degrees of freedom.
-
17N.2.SL.TZ0.T_1f.i:
Write down the -value for the test;
-
17N.2.SL.TZ0.T_1f.ii:
Write down the statistic.
-
17N.2.SL.TZ0.T_1g:
State the result of the test. Give a reason for your answer.
-
19M.2.SL.TZ1.T_3a:
Write down the total number of people, from this group, who are pet owners.
-
19M.2.SL.TZ1.T_3b:
Write down the modal number of pets.
-
19M.2.SL.TZ1.T_3c.i:
For these data, write down the median number of pets.
-
19M.2.SL.TZ1.T_3c.ii:
For these data, write down the lower quartile.
-
19M.2.SL.TZ1.T_3c.iii:
For these data, write down the upper quartile.
-
19M.2.SL.TZ1.T_3d:
Write down the ratio of teenagers to non-teenagers in its simplest form.
-
19M.2.SL.TZ1.T_3e.i:
State the null hypothesis.
-
19M.2.SL.TZ1.T_3e.ii:
State the alternative hypothesis.
-
19M.2.SL.TZ1.T_3f:
Write down the number of degrees of freedom for this test.
-
19M.2.SL.TZ1.T_3g:
Calculate the expected number of teenagers that prefer cats.
-
19M.2.SL.TZ1.T_3i:
State the conclusion for this test. Give a reason for your answer.
-
19M.2.SL.TZ2.T_1a:
Write down the null hypothesis, H0 , for this test.
-
19M.2.SL.TZ2.T_1b:
State the number of degrees of freedom.
-
19M.2.SL.TZ2.T_1c.i:
the expected frequency of female students who chose to take the Chinese class.
-
19M.2.SL.TZ2.T_1d:
State whether or not H0 should be rejected. Justify your statement.
-
19M.2.SL.TZ2.T_1e.i:
Find the probability that the student does not take the Spanish class.
-
19M.2.SL.TZ2.T_1e.ii:
Find the probability that neither of the two students take the Spanish class.
-
19M.2.SL.TZ2.T_1e.iii:
Find the probability that at least one of the two students is female.
-
18M.2.SL.TZ2.T_3a.i:
Find the probability that a basketball player has a weight that is less than 61 kg.
-
18M.2.SL.TZ2.T_3a.ii:
In a training session there are 40 basketball players.
Find the expected number of players with a weight less than 61 kg in this training session.
-
18M.2.SL.TZ2.T_3b.i:
Sketch a normal curve to represent this probability.
-
18M.2.SL.TZ2.T_3b.ii:
Find the value of q.
-
18M.2.SL.TZ2.T_3c:
Given that P(W > k) = 0.225 , find the value of k.
-
18M.2.SL.TZ2.T_3d.i:
For this test state the null hypothesis.
-
18M.2.SL.TZ2.T_3d.ii:
For this test find the p-value.
-
18M.2.SL.TZ2.T_3e:
State a conclusion for this test. Justify your answer.
-
17M.2.SL.TZ2.T_1a.i:
If the teacher chooses a response at random, find the probability that it is a response to the Calculus question;
-
17M.2.SL.TZ2.T_1a.ii:
If the teacher chooses a response at random, find the probability that it is a satisfactory response to the Calculus question;
-
17M.2.SL.TZ2.T_1a.iii:
If the teacher chooses a response at random, find the probability that it is a satisfactory response, given that it is a response to the Calculus question.
-
17M.2.SL.TZ2.T_1b:
The teacher groups the responses by topic, and chooses two responses to the Logic question. Find the probability that both are not satisfactory.
-
17M.2.SL.TZ2.T_1c:
State the null hypothesis for this test.
-
17M.2.SL.TZ2.T_1d:
Show that the expected frequency of satisfactory Calculus responses is 12.
-
17M.2.SL.TZ2.T_1e:
Write down the number of degrees of freedom for this test.
-
17M.2.SL.TZ2.T_1f:
Use your graphic display calculator to find the statistic for this data.
-
17M.2.SL.TZ2.T_1g:
State the conclusion of this test. Give a reason for your answer.
-
16N.1.SL.TZ0.T_6a:
Find the expected frequency of the patients who became infected whilst in Nightingale ward.
-
16N.1.SL.TZ0.T_6b:
For this test, write down the statistic.
-
16N.1.SL.TZ0.T_6c:
State, giving a reason, whether the null hypothesis should be rejected.
-
17M.1.SL.TZ1.T_6a:
Write down the value of .
-
17M.1.SL.TZ1.T_6b:
Write down the number of degrees of freedom for this test.
-
17M.1.SL.TZ1.T_6c:
Use your graphic display calculator to find the statistic for this test.
-
17M.1.SL.TZ1.T_6d:
State the conclusion for this test. Give a reason for your answer.
-
18N.1.SL.TZ0.T_4a:
State the null hypothesis, H0, for this test.
-
18N.1.SL.TZ0.T_4b:
Calculate the expected number of male engineers.
-
18N.1.SL.TZ0.T_4c:
Find the p-value for this test.
-
18N.1.SL.TZ0.T_4d:
Abhinav rejects H0.
State a reason why Abhinav is incorrect in doing so.
-
19N.2.SL.TZ0.T_1a:
State , the null hypothesis for this test.
-
19N.2.SL.TZ0.T_1b:
Write down the number of degrees of freedom.
-
19N.2.SL.TZ0.T_1c:
Show that the expected number of children who chose shrimp is , correct to two significant figures.
-
19N.2.SL.TZ0.T_1d.i:
the statistic.
-
19N.2.SL.TZ0.T_1d.ii:
the -value.
-
19N.2.SL.TZ0.T_1e:
State the conclusion for this test. Give a reason for your answer.
-
19N.2.SL.TZ0.T_1f.i:
Calculate the probability that the customer is an adult.
-
19N.2.SL.TZ0.T_1f.ii:
Calculate the probability that the customer is an adult or that the customer chose shrimp.
-
19N.2.SL.TZ0.T_1f.iii:
Given that the customer is a child, calculate the probability that they chose pasta or fish.
-
20N.1.SL.TZ0.T_10a:
Write down , the null hypothesis for this test.
-
20N.1.SL.TZ0.T_10b:
Find the expected number of short trips when it rained.
-
20N.1.SL.TZ0.T_10c:
The -value for this test is .
State the conclusion to Isaac’s test. Justify your reasoning.
-
EXN.1.SL.TZ0.3b.i:
Find the -value for the owner’s test.
-
EXN.1.SL.TZ0.3b.ii:
The test is performed at the significance level.
State the conclusion of the test, giving a reason for your answer.
-
EXN.2.SL.TZ0.4c.i:
Find the expected frequency for each of the numbers if the manufacturer’s claim is true.
-
EXN.2.SL.TZ0.4c.ii:
Write down the null and alternative hypotheses.
-
EXN.2.SL.TZ0.4c.iii:
State the degrees of freedom for the test.
-
EXN.2.SL.TZ0.4c.iv:
Determine the conclusion of the test, clearly justifying your answer.
-
21M.1.SL.TZ1.6a:
State, in words, the null hypothesis.
-
21M.1.SL.TZ1.6b:
Calculate the -value for this test.
-
21M.1.SL.TZ1.6c:
State whether the result of the test supports Arriane’s claim. Justify your reasoning.
-
21M.2.SL.TZ1.4c:
State the null and alternative hypotheses.
-
21M.2.SL.TZ1.4d:
Find the -value for the test.
-
21M.2.SL.TZ1.4e:
State the conclusion of the test. Give a reason for your answer.
-
21M.3.AHL.TZ1.2a:
Use an appropriate test, at the significance level, to determine whether a new employee staying with the firm is independent of their interview rating. State the null and alternative hypotheses, the -value and the conclusion of the test.
-
21M.1.SL.TZ2.8a:
Write down the null and alternative hypotheses.
-
21M.1.SL.TZ2.8b:
Find the -value for this test.
-
21M.1.SL.TZ2.8c:
Write down the conclusion to the test. Give a reason for your answer.
-
21M.1.SL.TZ2.11a:
Find an estimate for how many copies the vendor expects to sell each day.
-
21M.1.SL.TZ2.11b.i:
Write down the degrees of freedom for this test.
-
21M.1.SL.TZ2.11b.ii:
Write down the conclusion to the test. Give a reason for your answer.
-
21M.1.AHL.TZ2.9a:
Find an estimate for how many copies the vendor expects to sell each day.
-
21M.1.AHL.TZ2.9b.ii:
Write down the degrees of freedom for this test.
-
21M.1.AHL.TZ2.9b.iii:
Write down the conclusion to the test. Give a reason for your answer.
-
21M.1.AHL.TZ2.9b.i:
State the null and alternative hypotheses for this test.
-
21N.2.SL.TZ0.1a.i:
prefers a tablet.
-
21N.2.SL.TZ0.1a.ii:
is years old and prefers a mobile phone.
-
21N.2.SL.TZ0.1a.iii:
prefers a laptop given that they are years old.
-
21N.2.SL.TZ0.1a.iv:
prefers a tablet or is years old.
-
21N.2.SL.TZ0.1b:
State the null and alternative hypotheses.
-
21N.2.SL.TZ0.1c:
Write down the number of degrees of freedom.
-
21N.2.SL.TZ0.1d.i:
Write down the test statistic.
-
21N.2.SL.TZ0.1d.ii:
Write down the -value.
-
21N.2.SL.TZ0.1d.iii:
State the conclusion for the test in context. Give a reason for your answer.
-
21N.2.AHL.TZ0.7a.i:
Write down null and alternative hypotheses for Loreto’s test.
-
21N.2.AHL.TZ0.7a.ii:
Using the data from Loreto’s sample, perform the hypothesis test at a significance level to determine if Loreto should employ extra staff.
-
21N.2.AHL.TZ0.7b.i:
Write down null and alternative hypotheses for this test.
-
21N.2.AHL.TZ0.7b.ii:
Perform the test, clearly stating the conclusion in context.
-
22M.3.AHL.TZ2.1d.i:
Write down the number of degrees of freedom for her test.
-
22M.3.AHL.TZ2.1d.ii:
Perform the goodness of fit test and state, with reason, a conclusion.
-
22M.3.AHL.TZ2.1e.i:
By finding a critical value, perform this test at a significance level.
-
22M.1.SL.TZ1.7a:
Write down the null and alternative hypotheses.
-
22M.1.SL.TZ1.7b:
Write down the degrees of freedom.
-
22M.1.SL.TZ1.7c:
Write down the expected frequency of rolling a .
-
22M.1.SL.TZ1.7d:
Find the -value for the test.
-
22M.1.SL.TZ1.7e:
State the conclusion of the test. Give a reason for your answer.
-
22M.1.SL.TZ2.8a:
State the alternative hypothesis.
-
22M.1.SL.TZ2.8b:
Calculate the -value for this test.
-
22M.1.SL.TZ2.8c.i:
State the conclusion of the test. Justify your answer.
-
22M.1.SL.TZ2.8c.ii:
State what your conclusion means in context.
-
22M.1.AHL.TZ2.9c.i:
State the alternative hypothesis.
-
22M.1.AHL.TZ2.9c.ii:
Given that all assumptions for this test are satisfied, carry out an appropriate hypothesis test. State and justify your conclusion. Use a significance level.
-
22M.2.AHL.TZ1.3c:
Perform an appropriate test at the significance level to see if the mean marks achieved by the students in the school are higher than the national standard. It can be assumed that the marks come from a normal population.
-
22M.2.AHL.TZ1.3d:
State one reason why the test might not be valid.
AHL 4.12—Data collection, reliability and validity tests
-
SPM.3.AHL.TZ0.1a:
Identify a test that might have been used to verify the null hypothesis that the predictions from the standardized test can be modelled by a normal distribution.
-
SPM.3.AHL.TZ0.1b:
State why comparing only the final IB points of the students from the two schools would not be a valid test for the effectiveness of the two different teaching methods.
-
SPM.3.AHL.TZ0.1c.i:
Find the mean change.
-
SPM.3.AHL.TZ0.1c.ii:
Find the standard deviation of the changes.
-
SPM.3.AHL.TZ0.1d:
Use a paired -test to determine whether there is significant evidence that the students in school A have improved their IB points since the start of the course.
-
SPM.3.AHL.TZ0.1e.i:
Use an appropriate test to determine whether there is evidence, at the 5 % significance level, that the students in school B have improved more than those in school A.
-
SPM.3.AHL.TZ0.1e.ii:
State why it was important to test that both sets of points were normally distributed.
-
SPM.3.AHL.TZ0.1f.i:
Perform a test on the data from school A to show it is reasonable to assume a linear relationship between effort scores and improvements in IB points. You may assume effort scores follow a normal distribution.
-
SPM.3.AHL.TZ0.1f.ii:
Hence, find the expected improvement between predicted and final points for an increase of one unit in effort grades, giving your answer to one decimal place.
-
SPM.3.AHL.TZ0.1g:
Use an appropriate test to determine whether showing an improvement is independent of gender.
-
SPM.3.AHL.TZ0.1h:
If you were to repeat the test performed in part (e) intending to compare the quality of the teaching between the two schools, suggest two ways in which you might choose your sample to improve the validity of the test.
-
EXM.1.AHL.TZ0.19a:
State the name of this test for reliability.
-
EXM.1.AHL.TZ0.19b:
State a possible disadvantage of using this test for reliability.
-
EXM.1.AHL.TZ0.19c:
Calculate Pearson’s product moment correlation coefficient for this data.
-
EXM.1.AHL.TZ0.19d:
Hence determine, with a reason, if the survey is reliable.
-
EXM.1.AHL.TZ0.20a:
State the name of this test for validity.
-
EXM.1.AHL.TZ0.20b:
Calculate Pearson’s product moment correlation coefficient for this data.
-
EXM.1.AHL.TZ0.20c:
Hence determine, with a reason, if the new exam is a valid indicator of future performance.
-
EXM.2.AHL.TZ0.24a:
Show that this data leads to an estimated value of .
-
EXM.2.AHL.TZ0.24b:
Stating null and alternative hypotheses, carry out an appropriate test at the 5 % level to decide whether the farmer’s claim can be justified.
-
EXM.1.AHL.TZ0.55a.i:
Calculate the mean number of brown eggs in a box.
-
EXM.1.AHL.TZ0.55a.ii:
Hence estimate , the probability that a randomly chosen egg is brown.
-
EXM.1.AHL.TZ0.55b:
By calculating an appropriate statistic, test, at the 5% significance level, whether or not the binomial distribution gives a good fit to these data.
-
EXM.1.AHL.TZ0.56a:
Calculate the mean number of eggs laid by these birds.
-
EXM.1.AHL.TZ0.56b.i:
Write down appropriate hypotheses.
-
EXM.1.AHL.TZ0.56b.ii:
Carry out a test at the 1% significance level, and state your conclusion.
-
EXM.1.AHL.TZ0.57b:
The number of cars passing a certain point in a road was recorded during 80 equal time intervals and summarized in the table below.
Carry out a goodness of fit test at the 5% significance level to decide if the above data can be modelled by a Poisson distribution.
-
EXM.1.AHL.TZ0.58a:
Find the exact value of the mean of this distribution.
-
EXM.1.AHL.TZ0.58b:
Test, at the 5% level of significance, whether or not the data can be modelled by a Poisson distribution.
-
EXM.1.AHL.TZ0.59a:
Calculate unbiased estimates of the population mean and the population variance.
-
EXM.1.AHL.TZ0.59b.i:
State suitable hypotheses.
-
EXM.1.AHL.TZ0.59b.ii:
Calculate the value of the statistic and state your conclusion using a 10% level of significance.
-
EXM.2.AHL.TZ0.26a:
The scientists wish to investigate the claim that Group B gain weight faster than Group A. Test this claim at the 5% level of significance, noting which hypothesis test you are using. You may assume that the weight gain for each group is normally distributed, with the same variance, and independent from each other.
-
EXM.2.AHL.TZ0.26b:
The data from the two samples above are combined to form a single set of data. The following frequency table gives the observed frequencies for the combined sample. The data has been divided into five intervals.
Test, at the 5% level, whether the combined data can be considered to be a sample from a normal population with a mean of 380.
-
EXM.2.AHL.TZ0.27a.i:
a goodness of fit test (a complete explanation required);
-
EXM.2.AHL.TZ0.27a.ii:
the level of significance of a hypothesis test.
-
EXM.2.AHL.TZ0.27b:
Find the mean and standard deviation of the sample data in the table above. Show how you arrived at your answers.
-
EXM.2.AHL.TZ0.27c:
Most of the expected frequencies have been calculated in the third column. (Frequencies have been rounded to the nearest integer, and frequencies in the first and last classes have been extended to include the rest of the data beyond 15 and 225. Find the values of , and and show how you arrived at your answers.
-
EXM.2.AHL.TZ0.27d:
In order to test for the goodness of fit, the test statistic was calculated to be 1.0847. Show how this was done.
-
EXM.2.AHL.TZ0.27e:
State your hypotheses, critical number, decision rule and conclusion (using a 5% level of significance).
-
EXM.2.AHL.TZ0.28a.i:
State suitable hypotheses for testing this belief.
-
EXM.2.AHL.TZ0.28a.ii:
Calculate the mean of these data and hence estimate the value of .
-
EXM.2.AHL.TZ0.28a.iii:
Calculate an appropriate value of and state your conclusion, using a 1% significance level.
-
EXM.2.AHL.TZ0.28b.i:
Find the significance level of this procedure.
-
EXM.2.AHL.TZ0.28b.ii:
Some time later, the actual value of is 503. Find the probability of a Type II error.
-
EXM.2.AHL.TZ0.29a:
A horse breeder records the number of births for each of 100 horses during the past eight years. The results are summarized in the following table:
Stating null and alternative hypotheses carry out an appropriate test at the 5% significance level to decide whether the results can be modelled by B (6, 0.5).
-
EXM.2.AHL.TZ0.29b:
Without doing any further calculations, explain briefly how you would carry out a test, at the 5% significance level, to decide if the data can be modelled by B(6, ), where is unspecified.
-
EXM.2.AHL.TZ0.29c:
A different horse breeder collected data on the time and outcome of births. The data are summarized in the following table:
Carry out an appropriate test at the 5% significance level to decide whether there is an association between time and outcome.
-
21M.3.AHL.TZ1.2d.i:
State the name of this type of test for reliability.
-
21M.3.AHL.TZ2.1a.i:
Describe one way in which Juliet could improve the reliability of her investigation.
-
21M.3.AHL.TZ2.1a.ii:
Describe one criticism that can be made about the validity of Juliet’s investigation.
AHL 4.13—Non-linear regression
-
SPM.1.AHL.TZ0.12:
Product research leads a company to believe that the revenue () made by selling its goods at a price () can be modelled by the equation.
, ,
There are two competing models, A and B with different values for the parameters and .
Model A has = 3, = −0.5 and model B has = 2.5, = −0.6.
The company experiments by selling the goods at three different prices in three similar areas and the results are shown in the following table.
The company will choose the model with the smallest value for the sum of square residuals.
Determine which model the company chose.
-
EXM.2.AHL.TZ0.12a:
Use the trapezoidal rule to find an estimate for the area.
-
EXM.2.AHL.TZ0.12b.i:
Use all the coordinates in the table to find the equation of the least squares cubic regression curve.
-
EXM.2.AHL.TZ0.12b.ii:
Write down the coefficient of determination.
-
EXM.2.AHL.TZ0.12c.i:
Write down an expression for the area enclosed by the cubic regression curve, the -axis, the -axis and the line .
-
EXM.2.AHL.TZ0.12c.ii:
Find the value of this area.
-
EXM.1.AHL.TZ0.21a:
Explain why can be modeled by an exponential function.
-
EXM.1.AHL.TZ0.21b:
Find the equation of the least squares exponential regression curve for .
-
EXM.1.AHL.TZ0.21c.i:
Write down the coefficient of determination, .
-
EXM.1.AHL.TZ0.21c.ii:
Interpret what the value of tells you about the model.
-
EXM.1.AHL.TZ0.21d:
Hence predict the temperature of the water after 3 minutes.
-
EXM.3.AHL.TZ0.7a.i:
Use the trapezoidal rule to find an estimate for the area.
-
EXM.3.AHL.TZ0.7a.ii:
With reference to the shape of the graph, explain whether your answer to part (a)(i) will be an over-estimate or an underestimate of the area.
-
EXM.3.AHL.TZ0.7b.i:
Use all the coordinates in the table to find the equation of the least squares cubic regression curve.
-
EXM.3.AHL.TZ0.7b.ii:
Write down the coefficient of determination.
-
EXM.3.AHL.TZ0.7c.i:
Write down an expression for the area enclosed by the cubic function, the -axis, the -axis and the line .
-
EXM.3.AHL.TZ0.7c.ii:
Find the value of this area.
-
EXM.3.AHL.TZ0.7d.i:
Show that .
-
EXM.3.AHL.TZ0.7d.ii:
Hence explain how a straight line graph could be drawn using the coordinates in the table.
-
EXM.3.AHL.TZ0.7d.iii:
By finding the equation of a suitable regression line, show that and .
-
EXM.3.AHL.TZ0.7d.iv:
Hence find the area enclosed by the exponential function, the -axis, the -axis and the line .
-
EXM.3.AHL.TZ0.9a:
Use an exponential regression to find the value of and of , correct to 4 decimal places.
-
EXM.3.AHL.TZ0.9b.i:
the number of new people infected on day 6.
-
EXM.3.AHL.TZ0.9b.ii:
the day when the total number of people infected will be greater than 1000.
-
EXM.3.AHL.TZ0.9c:
Use your answer to part (a) to show that the model predicts 16.7 people will be infected on the first day.
-
EXM.3.AHL.TZ0.9d.i:
Explain why the number of degrees of freedom is 2.
-
EXM.3.AHL.TZ0.9d.ii:
Perform a goodness of fit test at the 5% significance level. You should clearly state your hypotheses, the p-value, and your conclusion.
-
EXM.3.AHL.TZ0.9e:
Give two reasons why the prediction in part (b)(ii) might be lower than 14.
-
EXM.3.AHL.TZ0.9f.i:
.
-
EXM.3.AHL.TZ0.9f.ii:
.
-
EXM.3.AHL.TZ0.9f.iii:
.
-
EXM.3.AHL.TZ0.9g:
Hence predict the total number of people infected by this disease after several months.
-
EXM.3.AHL.TZ0.9h:
Use the logistic model to find the day when the rate of increase of people infected is greatest.
-
EXN.2.AHL.TZ0.4e:
Find the sum of the square residuals for Jorge’s model using the values .
-
21M.2.AHL.TZ1.4b.i:
Find the equation of the least squares regression quadratic curve for these four points.
-
21M.2.AHL.TZ1.4b.ii:
By considering the gradient of this curve when , explain why it may not be a good model.
-
21M.2.AHL.TZ1.4c:
Find the equation of the new model.
-
21M.3.AHL.TZ2.1e.iii:
Find the value of , of and of .
-
21M.3.AHL.TZ2.1e.iv:
Find the coefficient of determination for each of the two models she considers.
-
21M.3.AHL.TZ2.1e.v:
Hence compare the two models.
-
21M.3.AHL.TZ2.1e.vi:
Juliet decides to use the coefficient of determination to choose between these two models.
Comment on the validity of her decision.
-
21N.1.AHL.TZ0.12a:
Use the data in the second table to find the value of and the value of for the regression line, .
-
21N.1.AHL.TZ0.12b:
Assuming that the model found in part (a) remains valid, estimate the percentage of trees in stock when .
-
21N.3.AHL.TZ0.2a.i:
Find the equation of the regression line of on .
-
21N.3.AHL.TZ0.2a.ii:
Interpret the meaning of parameter in the context of the model.
-
21N.3.AHL.TZ0.2a.iii:
Suggest why Eva’s use of the linear regression equation in this way could be unreliable.
-
21N.3.AHL.TZ0.2b.i:
Find the equation of the least squares quadratic regression curve.
-
21N.3.AHL.TZ0.2b.ii:
Find the value of .
-
21N.3.AHL.TZ0.2b.iii:
Hence, write down a suitable domain for Eva’s function .
-
21N.3.AHL.TZ0.2c:
Show that .
-
21N.3.AHL.TZ0.2d:
By solving the differential equation , show that the general solution is given by , where .
-
21N.3.AHL.TZ0.2e:
Use the general solution from part (d) and the initial condition to predict the value of .
-
21N.3.AHL.TZ0.2f:
Find this new height.
-
21N.3.AHL.TZ0.2g.i:
Show that , where .
-
21N.3.AHL.TZ0.2g.ii:
Use Euler’s method with a step length of minutes to estimate the maximum value of .
-
22M.3.AHL.TZ1.1b.iii:
Write down the value of for this model.
-
22M.3.AHL.TZ1.1b.iv:
Hence comment on the suitability of the model from (b)(ii) in comparison with the linear model found in part (a).
AHL 4.14—Linear transformation of a single RV, E(X) and VAR(X), unbiased estimators
-
SPM.1.AHL.TZ0.9a:
Find for this sample.
-
SPM.1.AHL.TZ0.9b:
Find a 95 % confidence interval for the population mean, giving your answer to 4 significant figures.
-
SPM.1.AHL.TZ0.9c:
The bags are labelled as being 1.5 kg mass. Comment on this claim with reference to your answer in part (b).
-
EXM.3.AHL.TZ0.8a.i:
Find unbiased estimates for the population mean.
-
EXM.3.AHL.TZ0.8a.ii:
Find unbiased estimates for the population Variance.
-
EXM.3.AHL.TZ0.8b:
Show that the expected frequency for 20 < ≤ 4 is 31.5 correct to 1 decimal place.
-
EXM.3.AHL.TZ0.8c:
Perform a suitable test, at the 5% significance level, to determine if the scores follow a normal distribution, with the mean and variance found in part (a). You should clearly state your hypotheses, the degrees of freedom, the p-value and your conclusion.
-
EXM.3.AHL.TZ0.8d:
Use the normal distribution model to find the score required to pass.
-
EXM.3.AHL.TZ0.8e:
Perform a suitable test, at the 5% significance level, to determine if there is a difference between the mean scores of males and females. You should clearly state your hypotheses, the p-value and your conclusion.
-
EXM.3.AHL.TZ0.8f:
Perform a suitable test, at the 5% significance level, to determine if it is easier to achieve a distinction on the new exam. You should clearly state your hypotheses, the critical region and your conclusion.
-
EXM.3.AHL.TZ0.8g.i:
Find the probability of making a Type I error.
-
EXM.3.AHL.TZ0.8g.ii:
Given that find the probability of making a Type II error.
-
EXM.1.AHL.TZ0.59a:
Calculate unbiased estimates of the population mean and the population variance.
-
EXM.1.AHL.TZ0.59b.i:
State suitable hypotheses.
-
EXM.1.AHL.TZ0.59b.ii:
Calculate the value of the statistic and state your conclusion using a 10% level of significance.
-
19M.3.AHL.TZ0.Hsp_3a:
State the distribution of giving its mean and variance.
-
19M.3.AHL.TZ0.Hsp_3b:
Find an unbiased estimate for .
-
19M.3.AHL.TZ0.Hsp_3c:
Find an unbiased estimate for .
-
19M.3.AHL.TZ0.Hsp_3d:
Find a 90 % confidence interval for .
-
19M.3.AHL.TZ0.Hsp_3e.i:
Find the -value for the test.
-
19M.3.AHL.TZ0.Hsp_3e.ii:
Write down the conclusion reached.
-
18N.3.AHL.TZ0.Hsp_3a.i:
Find the probability that a fish from this lake will have a weight of more than 560 grams.
-
18N.3.AHL.TZ0.Hsp_3a.ii:
The maximum weight a hand net can hold is 6 kg. Find the probability that a catch of 11 fish can be carried in the hand net.
-
18N.3.AHL.TZ0.Hsp_3b.i:
State the distribution of your test statistic, including the parameter.
-
18N.3.AHL.TZ0.Hsp_3b.ii:
Find the p-value for the test.
-
18N.3.AHL.TZ0.Hsp_3b.iii:
State the conclusion of the test, justifying your answer.
-
18N.3.AHL.TZ0.Hsp_3c.i:
State suitable hypotheses for the test.
-
18N.3.AHL.TZ0.Hsp_3c.ii:
Find the product-moment correlation coefficient .
-
18N.3.AHL.TZ0.Hsp_3c.iii:
State the p-value and interpret it in this context.
-
18N.3.AHL.TZ0.Hsp_3d:
Use an appropriate regression line to estimate the weight of a fish with length 360 mm.
-
18M.3.AHL.TZ0.Hsp_1a:
Find the probability that a randomly chosen male bird weighs between 4.75 kg and 4.85 kg.
-
18M.3.AHL.TZ0.Hsp_1b:
Find the probability that the weight of a randomly chosen male bird is more than twice the weight of a randomly chosen female bird.
-
18M.3.AHL.TZ0.Hsp_1c:
Two randomly chosen male birds and three randomly chosen female birds are placed on a weighing machine that has a weight limit of 18 kg. Find the probability that the total weight of these five birds is greater than the weight limit.
-
19M.3.AHL.TZ0.Hsp_2a:
Find the probability that the time taken for a randomly chosen customer to be dealt with by an employee is greater than 180 seconds.
-
19M.3.AHL.TZ0.Hsp_2b:
Find the probability that the time taken by an employee to deal with a queue of three customers is less than nine minutes.
-
19M.3.AHL.TZ0.Hsp_2c:
At the start of the day, one employee, Amanda, has a queue of four customers. A second employee, Brian, has a queue of three customers. You may assume they work independently.
Find the probability that Amanda’s queue will be dealt with before Brian’s queue.
-
18N.3.AHL.TZ0.Hsp_1a:
.
-
18N.3.AHL.TZ0.Hsp_1b:
Var.
-
18N.3.AHL.TZ0.Hsp_1c:
.
-
20N.3.AHL.TZ0.Hsp_3a:
Assuming that the shopkeeper’s claim is correct, find the probability that the weight of six randomly chosen carrots is more than two times the weight of one randomly chosen broccoli.
-
20N.3.AHL.TZ0.Hsp_3b:
Find the least value of required to ensure that the width of the confidence interval is less than .
-
20N.3.AHL.TZ0.Hsp_3c:
Find the significance level for this test.
-
20N.3.AHL.TZ0.Hsp_3d:
Given that the weights of the broccoli actually follow a normal distribution with mean and variance , find the probability of Anjali making a Type II error.
-
EXN.2.AHL.TZ0.3a:
Use the given value of to find the value of .
-
EXN.2.AHL.TZ0.6b.i:
both have a mass greater than .
-
EXN.2.AHL.TZ0.6b.ii:
have a total mass greater than .
-
EXN.3.AHL.TZ0.1d.i:
Show that an estimate for is .
-
EXN.3.AHL.TZ0.1d.ii:
Hence show that the variance of the proportion of marked fish in the sample, , is .
-
EXN.3.AHL.TZ0.1e.i:
Taking the value for the variance given in (d) (ii) as a good approximation for the true variance, find the upper and lower bounds for the proportion of marked fish in the lake.
-
EXN.3.AHL.TZ0.1e.ii:
Hence find upper and lower bounds for the number of fish in the lake when .
-
EXN.3.AHL.TZ0.1f:
Given this result, comment on the validity of the linear model used in part (a).
-
21M.1.AHL.TZ1.14a:
Find the mean weight of a bag of apples.
-
21M.1.AHL.TZ1.14b:
Find the standard deviation of the weights of these bags of apples.
-
21M.1.AHL.TZ1.14c:
Find the probability that a bag selected at random weighs more than .
-
21M.1.AHL.TZ2.10a:
Find an unbiased estimate for the mean number of chocolates per packet.
-
21M.1.AHL.TZ2.10b:
Use the formula to determine an unbiased estimate for the variance of the number of chocolates per packet.
-
21M.1.AHL.TZ2.10c:
Find a confidence interval for . You may assume that all conditions for a confidence interval have been met.
-
21M.1.AHL.TZ2.10d:
Suggest, with justification, a valid conclusion that Talha could make.
-
22M.1.AHL.TZ1.9b:
A statistician in the company suggests it would be fairer if the company passes the inspection when the mean weight of five randomly chosen bags is greater than .
Find the probability of passing the inspection if the statistician’s suggestion is followed.
-
22M.1.AHL.TZ2.9a:
Find an unbiased estimate of the population mean of .
-
22M.1.AHL.TZ2.9b:
Find an unbiased estimate of the population variance of .
-
22M.2.AHL.TZ1.3e.i:
their mean standardized score.
-
22M.2.AHL.TZ1.3e.ii:
the standard deviation of their standardized score.
AHL 4.15—Central limit theorem
-
SPM.1.AHL.TZ0.10:
In a coffee shop, the time it takes to serve a customer can be modelled by a normal distribution with a mean of 1.5 minutes and a standard deviation of 0.4 minutes.
Two customers enter the shop together. They are served one at a time.
Find the probability that the total time taken to serve both customers will be less than 4 minutes.
Clearly state any assumptions you have made.
-
16N.3.AHL.TZ0.Hsp_2a:
Find
(i) ;
(ii) .
-
16N.3.AHL.TZ0.Hsp_2b:
Explain why a normal distribution can be used to give an approximate model for .
-
16N.3.AHL.TZ0.Hsp_2c:
Use this model to find the values of and such that , where and are symmetrical about the mean of .
-
16N.3.AHL.TZ0.Hsp_2d:
Calculate the probability that he makes a Type II error.
-
19M.3.AHL.TZ0.Hsp_3a:
State the distribution of giving its mean and variance.
-
19M.3.AHL.TZ0.Hsp_3b:
Find an unbiased estimate for .
-
19M.3.AHL.TZ0.Hsp_3c:
Find an unbiased estimate for .
-
19M.3.AHL.TZ0.Hsp_3d:
Find a 90 % confidence interval for .
-
19M.3.AHL.TZ0.Hsp_3e.i:
Find the -value for the test.
-
19M.3.AHL.TZ0.Hsp_3e.ii:
Write down the conclusion reached.
-
19N.3.AHL.TZ0.Hsp_3a:
State the central limit theorem as applied to a random sample of size , taken from a distribution with mean and variance .
-
21M.1.AHL.TZ1.14a:
Find the mean weight of a bag of apples.
-
21M.1.AHL.TZ1.14b:
Find the standard deviation of the weights of these bags of apples.
-
21M.1.AHL.TZ1.14c:
Find the probability that a bag selected at random weighs more than .
-
21N.1.AHL.TZ0.14a:
Find the probability that a sack is under its labelled weight.
-
21N.1.AHL.TZ0.14b:
Find the lower quartile of the weights of the sacks of potatoes.
-
21N.1.AHL.TZ0.14c:
The sacks of potatoes are transported in crates. There are sacks in each crate and the weights of the sacks of potatoes are independent of each other.
Find the probability that the total weight of the sacks of potatoes in a crate exceeds .
-
22M.1.AHL.TZ1.9b:
A statistician in the company suggests it would be fairer if the company passes the inspection when the mean weight of five randomly chosen bags is greater than .
Find the probability of passing the inspection if the statistician’s suggestion is followed.
AHL 4.16—Confidence intervals
-
SPM.1.AHL.TZ0.9a:
Find for this sample.
-
SPM.1.AHL.TZ0.9b:
Find a 95 % confidence interval for the population mean, giving your answer to 4 significant figures.
-
SPM.1.AHL.TZ0.9c:
The bags are labelled as being 1.5 kg mass. Comment on this claim with reference to your answer in part (b).
-
19M.3.AHL.TZ0.Hsp_3a:
State the distribution of giving its mean and variance.
-
19M.3.AHL.TZ0.Hsp_3b:
Find an unbiased estimate for .
-
19M.3.AHL.TZ0.Hsp_3c:
Find an unbiased estimate for .
-
19M.3.AHL.TZ0.Hsp_3d:
Find a 90 % confidence interval for .
-
19M.3.AHL.TZ0.Hsp_3e.i:
Find the -value for the test.
-
19M.3.AHL.TZ0.Hsp_3e.ii:
Write down the conclusion reached.
-
18M.3.AHL.TZ0.Hsp_3a:
State suitable hypotheses for a two-tailed test.
-
18M.3.AHL.TZ0.Hsp_3b:
Find the critical region for testing at the 5 % significance level.
-
18M.3.AHL.TZ0.Hsp_3c:
Find the probability of making a Type II error.
-
18M.3.AHL.TZ0.Hsp_3d:
Another model of smartphone whose battery life may be assumed to be normally distributed with mean μ hours and standard deviation 1.2 hours is tested. A researcher measures the battery life of six of these smartphones and calculates a confidence interval of [10.2, 11.4] for μ.
Calculate the confidence level of this interval.
-
18N.3.AHL.TZ0.Hsp_2a.i:
Find unbiased estimates of the mean of .
-
18N.3.AHL.TZ0.Hsp_2a.ii:
Find unbiased estimates of the variance of .
-
18N.3.AHL.TZ0.Hsp_2b:
Assuming that is normally distributed, find
(i) the 90% confidence interval for the mean time taken to travel to work by the workers of this company,
(ii) the 95% confidence interval for the mean time taken to travel to work by the workers of this company.
-
18N.3.AHL.TZ0.Hsp_2c:
Before seeing these results the managing director believed that the mean time was 26 minutes.
Explain whether your answers to part (b) support her belief.
-
19N.3.AHL.TZ0.Hsp_3b:
Jack takes a random sample of size 100 and calculates that . Find an approximate 90 % confidence interval for .
-
21M.1.AHL.TZ2.10a:
Find an unbiased estimate for the mean number of chocolates per packet.
-
21M.1.AHL.TZ2.10b:
Use the formula to determine an unbiased estimate for the variance of the number of chocolates per packet.
-
21M.1.AHL.TZ2.10c:
Find a confidence interval for . You may assume that all conditions for a confidence interval have been met.
-
21M.1.AHL.TZ2.10d:
Suggest, with justification, a valid conclusion that Talha could make.
AHL 4.17—Poisson distribution
-
EXM.1.AHL.TZ0.56a:
Calculate the mean number of eggs laid by these birds.
-
EXM.1.AHL.TZ0.56b.i:
Write down appropriate hypotheses.
-
EXM.1.AHL.TZ0.56b.ii:
Carry out a test at the 1% significance level, and state your conclusion.
-
EXM.1.AHL.TZ0.57b:
The number of cars passing a certain point in a road was recorded during 80 equal time intervals and summarized in the table below.
Carry out a goodness of fit test at the 5% significance level to decide if the above data can be modelled by a Poisson distribution.
-
EXM.1.AHL.TZ0.58a:
Find the exact value of the mean of this distribution.
-
EXM.1.AHL.TZ0.58b:
Test, at the 5% level of significance, whether or not the data can be modelled by a Poisson distribution.
-
17M.1.AHL.TZ2.H_7a:
The random variable has the Poisson distribution . Given that , find the value of in the form where is an integer.
-
17M.1.AHL.TZ2.H_7b:
The random variable has the Poisson distribution . Find in the form where and are integers.
-
17M.2.AHL.TZ1.H_5a:
Find the probability that the carpet laid in the first room has fewer than three faults.
-
17M.2.AHL.TZ1.H_5b:
Find the probability that exactly seven rooms will have fewer than three faults in the carpet.
-
19M.1.AHL.TZ1.H_10a.i:
Find the remainder when is divided by .
-
19M.1.AHL.TZ1.H_10a.ii:
Find the remainder when is divided by .
-
19M.1.AHL.TZ1.H_10b:
Prove that has only one real zero.
-
19M.1.AHL.TZ1.H_10c:
Write down the transformation that will transform the graph of onto the graph of .
-
19M.1.AHL.TZ1.H_10d:
The random variable follows a Poisson distribution with a mean of and .
Find the value of .
-
18N.2.AHL.TZ0.H_10a.i:
Using this distribution model, find .
-
18N.2.AHL.TZ0.H_10a.ii:
Using this distribution model, find the standard deviation of .
-
18N.2.AHL.TZ0.H_10b.i:
an estimate for the mean number of emails received per working day.
-
18N.2.AHL.TZ0.H_10b.ii:
an estimate for the standard deviation of the number of emails received per working day.
-
18N.2.AHL.TZ0.H_10c:
Give one piece of evidence that suggests Willow’s Poisson distribution model is not a good fit.
-
18N.2.AHL.TZ0.H_10d:
Suppose that the probability of Archie receiving more than 10 emails in total on any one day is 0.99. Find the value of λ.
-
18N.2.AHL.TZ0.H_10e:
Now suppose that Archie received exactly 20 emails in total in a consecutive two day period. Show that the probability that he received exactly 10 of them on the first day is independent of λ.
-
18M.2.AHL.TZ1.H_6:
The mean number of squirrels in a certain area is known to be 3.2 squirrels per hectare of woodland. Within this area, there is a 56 hectare woodland nature reserve. It is known that there are currently at least 168 squirrels in this reserve.
Assuming the population of squirrels follow a Poisson distribution, calculate the probability that there are more than 190 squirrels in the reserve.
-
19M.2.AHL.TZ2.H_10a:
Find the probability that on a randomly selected day, Steffi does not visit Will’s house.
-
19M.2.AHL.TZ2.H_10b:
Copy and complete the probability distribution table for Y.
-
19M.2.AHL.TZ2.H_10c:
Hence find the expected number of times per day that Steffi is fed at Will’s house.
-
19M.2.AHL.TZ2.H_10d:
In any given year of 365 days, the probability that Steffi does not visit Will for at most days in total is 0.5 (to one decimal place). Find the value of .
-
19M.2.AHL.TZ2.H_10e:
Show that the expected number of occasions per year on which Steffi visits Will’s house and is not fed is at least 30.
-
17N.2.AHL.TZ0.H_6a:
Find the probability that Lucca eats at least one banana in a particular day.
-
17N.2.AHL.TZ0.H_6b:
Find the expected number of weeks in the year in which Lucca eats no bananas.
-
18M.2.AHL.TZ2.H_9a.i:
Find the probability that exactly 4 taxis arrive during T.
-
18M.2.AHL.TZ2.H_9a.ii:
Find the most likely number of taxis that would arrive during T.
-
18M.2.AHL.TZ2.H_9a.iii:
Given that more than 5 taxis arrive during T, find the probability that exactly 7 taxis arrive during T.
-
18M.2.AHL.TZ2.H_9b:
During quiet periods of the day, taxis arrive at a mean rate of 1.3 taxis every 10 minutes.
Find the probability that during a period of 15 minutes, of which the first 10 minutes is busy and the next 5 minutes is quiet, that exactly 2 taxis arrive.
-
16N.2.AHL.TZ0.H_3a:
Show that .
-
16N.2.AHL.TZ0.H_3b:
Given that and , use part (a) to find the value of .
-
19N.2.AHL.TZ0.H_2a:
Calculate the probability that Audrey will run at least two marathons in a particular year.
-
19N.2.AHL.TZ0.H_2b:
Find the probability that she will run at least two marathons in exactly four out of the following five years.
-
20N.1.AHL.TZ0.F_13a.i:
Calculate the value of , the product moment correlation coefficient of the sample.
-
20N.1.AHL.TZ0.F_13a.ii:
Assuming that the distribution of is bivariate normal with product moment correlation coefficient , calculate the -value of your result when testing the hypotheses .
-
20N.1.AHL.TZ0.F_13a.iii:
State whether your -value suggests that and are independent.
-
20N.1.AHL.TZ0.F_13b:
Given a further value from the distribution of , , predict the corresponding value of . Give your answer to one decimal place.
-
20N.2.AHL.TZ0.H_2:
Jenna is a keen book reader. The number of books she reads during one week can be modelled by a Poisson distribution with mean .
Determine the expected number of weeks in one year, of weeks, during which Jenna reads at least four books.
-
20N.2.AHL.TZ0.H_5a.i:
State two assumptions made in order for this model to be valid.
-
20N.2.AHL.TZ0.H_5a.ii:
Find the probability that at least three people from the sample took a holiday in the Lake District in .
-
20N.2.AHL.TZ0.H_5b:
From a random sample of holidaymakers, the probability that at least one of them took a holiday in the Lake District in is greater than .
Determine the least possible value of .
-
20N.2.AHL.TZ0.H_9a:
Find the probability that a randomly selected packet has a weight less than .
-
20N.2.AHL.TZ0.H_9b:
The probability that a randomly selected packet has a weight greater than grams is . Find the value of .
-
20N.2.AHL.TZ0.H_9c:
A packet is randomly selected. Given that the packet has a weight greater than , find the probability that it has a weight greater than .
-
20N.2.AHL.TZ0.H_9d:
From a random sample of packets, determine the number of packets that would be expected to have a weight lying within standard deviations of the mean.
-
20N.2.AHL.TZ0.H_9e:
Packets are delivered to supermarkets in batches of . Determine the probability that at least packets from a randomly selected batch have a weight less than .
-
EXN.1.AHL.TZ0.16a:
Find the probability that more than people arrive at the ride before Shunsuke.
-
EXN.1.AHL.TZ0.16b:
Find the probability there will be space for him on the car.
-
21M.1.AHL.TZ1.1:
George goes fishing. From experience he knows that the mean number of fish he catches per hour is . It is assumed that the number of fish he catches can be modelled by a Poisson distribution.
On a day in which George spends hours fishing, find the probability that he will catch more than fish.
-
21M.1.AHL.TZ2.15a:
State the null and alternative hypotheses for the test.
-
21M.1.AHL.TZ2.15b:
Find the probability that Sheila will make a type I error in her test conclusion.
-
21M.1.AHL.TZ2.15c:
Sheila finds coffees were sold during the -hour period.
State Sheila’s conclusion to the test. Justify your answer.
-
21M.2.AHL.TZ2.5b.i:
on exactly occasions.
-
21M.2.AHL.TZ2.5b.ii:
during the first and third month only.
-
21M.2.AHL.TZ2.5c:
Find the probability that over a -month period, there will be exactly months when Bill does not visit the garden.
-
21M.2.AHL.TZ2.5d:
After the first year, a number of baby magpies start to visit Hank’s garden. It may be assumed that each of these baby magpies visits the garden randomly and independently, and that the number of times each baby magpie visits the garden per month is modelled by a Poisson distribution with mean .
Determine the least number of magpies required, including Bill, in order that the probability of Hank’s garden having at least magpie visits per month is greater than .
-
21N.2.AHL.TZ0.7a.i:
Write down null and alternative hypotheses for Loreto’s test.
-
21N.2.AHL.TZ0.7a.ii:
Using the data from Loreto’s sample, perform the hypothesis test at a significance level to determine if Loreto should employ extra staff.
-
21N.2.AHL.TZ0.7b.i:
Write down null and alternative hypotheses for this test.
-
21N.2.AHL.TZ0.7b.ii:
Perform the test, clearly stating the conclusion in context.
-
22M.3.AHL.TZ2.1a.i:
Find the mean and variance for the sample data given in the table.
-
22M.3.AHL.TZ2.1a.ii:
Hence state why Aimmika believes her data follows a Poisson distribution.
-
22M.3.AHL.TZ2.1b:
State one assumption that Aimmika needs to make about the sales of bags of rice to support her belief that it follows a Poisson distribution.
-
22M.3.AHL.TZ2.1c:
Find the value of , of , and of . Give your answers to decimal places.
-
22M.1.AHL.TZ1.12d:
Find .
-
22M.1.AHL.TZ1.15a:
Assuming the null hypothesis to be true, state the distribution of .
-
22M.1.AHL.TZ1.15b:
Find the probability of a Type I error.
AHL 4.18—T and Z test, type I and II errors
-
SPM.1.AHL.TZ0.16a:
State a suitable null and alternative hypotheses for Tom’s test.
-
SPM.1.AHL.TZ0.16b:
Find the probability of a Type I error.
-
SPM.1.AHL.TZ0.16c:
The average number of fish caught in an hour is actually 2.5.
Find the probability of a Type II error.
-
SPM.3.AHL.TZ0.1a:
Identify a test that might have been used to verify the null hypothesis that the predictions from the standardized test can be modelled by a normal distribution.
-
SPM.3.AHL.TZ0.1b:
State why comparing only the final IB points of the students from the two schools would not be a valid test for the effectiveness of the two different teaching methods.
-
SPM.3.AHL.TZ0.1c.i:
Find the mean change.
-
SPM.3.AHL.TZ0.1c.ii:
Find the standard deviation of the changes.
-
SPM.3.AHL.TZ0.1d:
Use a paired -test to determine whether there is significant evidence that the students in school A have improved their IB points since the start of the course.
-
SPM.3.AHL.TZ0.1e.i:
Use an appropriate test to determine whether there is evidence, at the 5 % significance level, that the students in school B have improved more than those in school A.
-
SPM.3.AHL.TZ0.1e.ii:
State why it was important to test that both sets of points were normally distributed.
-
SPM.3.AHL.TZ0.1f.i:
Perform a test on the data from school A to show it is reasonable to assume a linear relationship between effort scores and improvements in IB points. You may assume effort scores follow a normal distribution.
-
SPM.3.AHL.TZ0.1f.ii:
Hence, find the expected improvement between predicted and final points for an increase of one unit in effort grades, giving your answer to one decimal place.
-
SPM.3.AHL.TZ0.1g:
Use an appropriate test to determine whether showing an improvement is independent of gender.
-
SPM.3.AHL.TZ0.1h:
If you were to repeat the test performed in part (e) intending to compare the quality of the teaching between the two schools, suggest two ways in which you might choose your sample to improve the validity of the test.
-
EXM.3.AHL.TZ0.8a.i:
Find unbiased estimates for the population mean.
-
EXM.3.AHL.TZ0.8a.ii:
Find unbiased estimates for the population Variance.
-
EXM.3.AHL.TZ0.8b:
Show that the expected frequency for 20 < ≤ 4 is 31.5 correct to 1 decimal place.
-
EXM.3.AHL.TZ0.8c:
Perform a suitable test, at the 5% significance level, to determine if the scores follow a normal distribution, with the mean and variance found in part (a). You should clearly state your hypotheses, the degrees of freedom, the p-value and your conclusion.
-
EXM.3.AHL.TZ0.8d:
Use the normal distribution model to find the score required to pass.
-
EXM.3.AHL.TZ0.8e:
Perform a suitable test, at the 5% significance level, to determine if there is a difference between the mean scores of males and females. You should clearly state your hypotheses, the p-value and your conclusion.
-
EXM.3.AHL.TZ0.8f:
Perform a suitable test, at the 5% significance level, to determine if it is easier to achieve a distinction on the new exam. You should clearly state your hypotheses, the critical region and your conclusion.
-
EXM.3.AHL.TZ0.8g.i:
Find the probability of making a Type I error.
-
EXM.3.AHL.TZ0.8g.ii:
Given that find the probability of making a Type II error.
-
EXM.2.AHL.TZ0.26a:
The scientists wish to investigate the claim that Group B gain weight faster than Group A. Test this claim at the 5% level of significance, noting which hypothesis test you are using. You may assume that the weight gain for each group is normally distributed, with the same variance, and independent from each other.
-
EXM.2.AHL.TZ0.26b:
The data from the two samples above are combined to form a single set of data. The following frequency table gives the observed frequencies for the combined sample. The data has been divided into five intervals.
Test, at the 5% level, whether the combined data can be considered to be a sample from a normal population with a mean of 380.
-
EXM.2.AHL.TZ0.28a.i:
State suitable hypotheses for testing this belief.
-
EXM.2.AHL.TZ0.28a.ii:
Calculate the mean of these data and hence estimate the value of .
-
EXM.2.AHL.TZ0.28a.iii:
Calculate an appropriate value of and state your conclusion, using a 1% significance level.
-
EXM.2.AHL.TZ0.28b.i:
Find the significance level of this procedure.
-
EXM.2.AHL.TZ0.28b.ii:
Some time later, the actual value of is 503. Find the probability of a Type II error.
-
16N.3.AHL.TZ0.Hsp_2a:
Find
(i) ;
(ii) .
-
16N.3.AHL.TZ0.Hsp_2b:
Explain why a normal distribution can be used to give an approximate model for .
-
16N.3.AHL.TZ0.Hsp_2c:
Use this model to find the values of and such that , where and are symmetrical about the mean of .
-
16N.3.AHL.TZ0.Hsp_2d:
Calculate the probability that he makes a Type II error.
-
19M.3.AHL.TZ0.Hsp_3a:
State the distribution of giving its mean and variance.
-
19M.3.AHL.TZ0.Hsp_3b:
Find an unbiased estimate for .
-
19M.3.AHL.TZ0.Hsp_3c:
Find an unbiased estimate for .
-
19M.3.AHL.TZ0.Hsp_3d:
Find a 90 % confidence interval for .
-
19M.3.AHL.TZ0.Hsp_3e.i:
Find the -value for the test.
-
19M.3.AHL.TZ0.Hsp_3e.ii:
Write down the conclusion reached.
-
18N.3.AHL.TZ0.Hsp_3a.i:
Find the probability that a fish from this lake will have a weight of more than 560 grams.
-
18N.3.AHL.TZ0.Hsp_3a.ii:
The maximum weight a hand net can hold is 6 kg. Find the probability that a catch of 11 fish can be carried in the hand net.
-
18N.3.AHL.TZ0.Hsp_3b.i:
State the distribution of your test statistic, including the parameter.
-
18N.3.AHL.TZ0.Hsp_3b.ii:
Find the p-value for the test.
-
18N.3.AHL.TZ0.Hsp_3b.iii:
State the conclusion of the test, justifying your answer.
-
18N.3.AHL.TZ0.Hsp_3c.i:
State suitable hypotheses for the test.
-
18N.3.AHL.TZ0.Hsp_3c.ii:
Find the product-moment correlation coefficient .
-
18N.3.AHL.TZ0.Hsp_3c.iii:
State the p-value and interpret it in this context.
-
18N.3.AHL.TZ0.Hsp_3d:
Use an appropriate regression line to estimate the weight of a fish with length 360 mm.
-
18M.3.AHL.TZ0.Hsp_3a:
State suitable hypotheses for a two-tailed test.
-
18M.3.AHL.TZ0.Hsp_3b:
Find the critical region for testing at the 5 % significance level.
-
18M.3.AHL.TZ0.Hsp_3c:
Find the probability of making a Type II error.
-
18M.3.AHL.TZ0.Hsp_3d:
Another model of smartphone whose battery life may be assumed to be normally distributed with mean μ hours and standard deviation 1.2 hours is tested. A researcher measures the battery life of six of these smartphones and calculates a confidence interval of [10.2, 11.4] for μ.
Calculate the confidence level of this interval.
-
17N.3.AHL.TZ0.Hsp_2a:
Determine unbiased estimates for and .
-
17N.3.AHL.TZ0.Hsp_2b.i:
Use a two-tailed test to determine the -value for the above results.
-
17N.3.AHL.TZ0.Hsp_2b.ii:
Interpret your -value at the 5% level of significance, justifying your conclusion.
-
17M.3.AHL.TZ0.Hsp_1a:
State suitable hypotheses to test the inspector’s claim.
-
17M.3.AHL.TZ0.Hsp_1b:
Find unbiased estimates of and .
-
17M.3.AHL.TZ0.Hsp_1c.i:
Carry out an appropriate test and state the -value obtained.
-
17M.3.AHL.TZ0.Hsp_1c.ii:
Using a 10% significance level and justifying your answer, state your conclusion in context.
-
17N.3.AHL.TZ0.Hsp_4a:
State suitable hypotheses to investigate whether or not , are independent.
-
17N.3.AHL.TZ0.Hsp_4b:
Find the least value of for which the test concludes that .
-
19N.3.AHL.TZ0.Hsp_1a.i:
State suitable hypotheses and to test Peter’s claim, using a two-tailed test.
-
19N.3.AHL.TZ0.Hsp_1a.ii:
Carry out a suitable test at the 5 % significance level. With reference to the -value, state your conclusion in the context of Peter’s claim.
-
19N.3.AHL.TZ0.Hsp_1b:
Peter uses the regression line of on as and calculates that a student with a Mathematics test score of 73 will have a running time of 101 seconds. Comment on the validity of his calculation.
-
19N.3.AHL.TZ0.Hsp_3c.i:
Find the critical region for Josie’s test, giving your answer correct to two decimal places.
-
19N.3.AHL.TZ0.Hsp_3c.ii:
Write down the probability that Josie makes a Type I error.
-
19N.3.AHL.TZ0.Hsp_3c.iii:
Given that the probability that Josie makes a Type II error is 0.25, find the value of , giving your answer correct to three significant figures.
-
20N.3.AHL.TZ0.Hsp_3a:
Assuming that the shopkeeper’s claim is correct, find the probability that the weight of six randomly chosen carrots is more than two times the weight of one randomly chosen broccoli.
-
20N.3.AHL.TZ0.Hsp_3b:
Find the least value of required to ensure that the width of the confidence interval is less than .
-
20N.3.AHL.TZ0.Hsp_3c:
Find the significance level for this test.
-
20N.3.AHL.TZ0.Hsp_3d:
Given that the weights of the broccoli actually follow a normal distribution with mean and variance , find the probability of Anjali making a Type II error.
-
EXN.2.AHL.TZ0.6c:
Write down the null and alternative hypotheses for the test.
-
EXN.2.AHL.TZ0.6d:
Find the critical region for this test.
-
EXN.2.AHL.TZ0.6e:
Find the mean and standard deviation of the mass of the melons for this year.
-
EXN.2.AHL.TZ0.6f:
Find the probability of a Type II error in the owner’s test.
-
21M.1.AHL.TZ1.11b:
Write down the null and alternative hypotheses.
-
21M.1.AHL.TZ1.11c:
Find the -value for the test.
-
21M.1.AHL.TZ1.11d:
State the conclusion of the test. Give a reason for your answer.
-
21M.3.AHL.TZ1.2d.ii:
For the data in this table, test the null hypothesis, , against the alternative hypothesis, , at the significance level. You may assume that all the requirements for carrying out the test have been met.
-
21M.3.AHL.TZ1.2d.iii:
Hence comment on the reliability of the written assessment.
-
21M.3.AHL.TZ1.2e.i:
Write down the number of tests they carry out.
-
21M.3.AHL.TZ1.2e.ii:
The tests are performed at the significance level.
Assuming that:- there is no correlation between the marks in any of the sections and scores in any of the attributes,
- the outcome of each hypothesis test is independent of the outcome of the other hypothesis tests,
find the probability that at least one of the tests will be significant.
-
21M.3.AHL.TZ1.2e.iii:
The firm obtains a significant result when comparing section of the written assessment and attribute . Interpret this result.
-
21M.1.AHL.TZ2.15a:
State the null and alternative hypotheses for the test.
-
21M.1.AHL.TZ2.15b:
Find the probability that Sheila will make a type I error in her test conclusion.
-
21M.1.AHL.TZ2.15c:
Sheila finds coffees were sold during the -hour period.
State Sheila’s conclusion to the test. Justify your answer.
-
21M.3.AHL.TZ2.1d.i:
State why the hypothesis test should be one-tailed.
-
21M.3.AHL.TZ2.1d.ii:
State the null and alternative hypotheses for this test.
-
21M.3.AHL.TZ2.1d.iii:
The critical value for this test, at the significance level, is . Juliet assumes that the population is bivariate normal.
Determine whether there is significant evidence of a positive correlation between annual income and happiness. Justify your answer.
-
21M.3.AHL.TZ2.1f.i:
State the name of the test which Juliet should use.
-
21M.3.AHL.TZ2.1f.ii:
State the null and alternative hypotheses for this test.
-
21M.3.AHL.TZ2.1f.iii:
Perform the test, using a significance level, and state your conclusion in context.
-
21N.2.AHL.TZ0.7a.i:
Write down null and alternative hypotheses for Loreto’s test.
-
21N.2.AHL.TZ0.7a.ii:
Using the data from Loreto’s sample, perform the hypothesis test at a significance level to determine if Loreto should employ extra staff.
-
21N.2.AHL.TZ0.7b.i:
Write down null and alternative hypotheses for this test.
-
21N.2.AHL.TZ0.7b.ii:
Perform the test, clearly stating the conclusion in context.
-
22M.3.AHL.TZ1.1a.iii:
Explain why it would not be appropriate to conduct a hypothesis test on the value of found in (a)(ii).
-
22M.3.AHL.TZ2.1e.ii:
Hence state the probability of a Type I error for this test.
-
22M.3.AHL.TZ2.1f:
By considering the claims of both Aimmika and Nichakarn, explain whether the advertising was beneficial to the store.
-
22M.1.AHL.TZ1.15c:
Find the probability of a Type II error, if the number of cars now follows a Poisson distribution with a mean of cars per minute.
-
22M.1.AHL.TZ2.12a:
Find the probability of making a Type I error when weighing a male cuttlefish.
-
22M.1.AHL.TZ2.12b:
Find the probability of making a Type II error when weighing a female cuttlefish.
AHL 4.19—Transition matrices – Markov chains
-
SPM.2.AHL.TZ0.6a:
Write down a transition matrix T representing the movements between the two companies in a particular year.
-
SPM.2.AHL.TZ0.6b:
Find the eigenvalues and corresponding eigenvectors of T.
-
SPM.2.AHL.TZ0.6c:
Hence write down matrices P and D such that T = PDP−1.
-
SPM.2.AHL.TZ0.6d:
Find an expression for the number of customers company X has after years, where .
-
SPM.2.AHL.TZ0.6e:
Hence write down the number of customers that company X can expect to have in the long term.
-
EXM.1.AHL.TZ0.17a:
Write down the transition matrix for this Markov chain.
-
EXM.1.AHL.TZ0.17b:
We know that she went out for lunch on a particular Sunday, find the probability that she went out for lunch on the following Tuesday.
-
EXM.1.AHL.TZ0.17c:
Find the steady state probability vector for this Markov chain.
-
EXM.1.AHL.TZ0.18a:
Show that is always an eigenvalue for M and find the other eigenvalue in terms of and .
-
EXM.1.AHL.TZ0.18b:
Find the steady state probability vector for M in terms of and .
-
EXM.3.AHL.TZ0.4a.i:
Draw a transition state diagram for this Markov chain problem.
-
EXM.3.AHL.TZ0.4a.ii:
Explain why for any transition state diagram the sum of the out degrees of the directed edges from a vertex (state) must add up to +1.
-
EXM.3.AHL.TZ0.4b:
Write down the transition matrix M, for this Markov chain problem.
-
EXM.3.AHL.TZ0.4c.i:
Find the steady state probability vector for this Markov chain problem.
-
EXM.3.AHL.TZ0.4c.ii:
Explain which part of the transition state diagram confirms this.
-
EXM.3.AHL.TZ0.4d:
Explain why having a steady state probability vector means that the matrix M must have an eigenvalue of .
-
EXM.3.AHL.TZ0.4e:
Find .
-
EXM.3.AHL.TZ0.4f:
Hence, deduce the form of .
-
EXM.3.AHL.TZ0.4g:
Explain how your answer to part (f) fits with your answer to part (c).
-
EXM.3.AHL.TZ0.4h:
Find the minimum number of tosses of the coin that Abi will have to make to be at least 95% certain of having finished the game by reaching state C.
-
EXN.1.AHL.TZ0.5a:
Find the transition matrix for the maze.
-
EXN.1.AHL.TZ0.5b:
A scientist sets up the robot and then leaves it moving around the maze for a long period of time.
Find the probability that the robot is in room when the scientist returns.
-
21M.2.AHL.TZ1.5a:
It is sunny today. Find the probability that it will be sunny in three days’ time.
-
21M.2.AHL.TZ1.5b:
Find the eigenvalues and eigenvectors of .
-
21M.2.AHL.TZ1.5c.i:
Write down the matrix .
-
21M.2.AHL.TZ1.5c.ii:
Write down the matrix .
-
21M.2.AHL.TZ1.5d:
Hence find the long-term percentage of sunny days in Vokram.
-
21M.1.AHL.TZ2.13a:
Determine the transition matrix for this graph.
-
21M.1.AHL.TZ2.13b:
If the mouse was left to wander indefinitely, use your graphic display calculator to estimate the percentage of time that the mouse would spend at point .
-
21M.1.AHL.TZ2.13c:
Comment on your answer to part (b), referring to at least one limitation of the model.
-
21N.1.AHL.TZ0.9a:
Complete the following transition diagram to represent this information.
-
21N.1.AHL.TZ0.9b:
Katie works for days in a year.
Find the probability that Katie cycles to work on her final working day of the year.
-
22M.1.AHL.TZ1.11b:
Using your answer to (a), or otherwise, find the long-term probability of the switch being in state . Give your answer in the form , where .
-
22M.2.AHL.TZ2.5a.i:
Write down the value of .
-
22M.2.AHL.TZ2.5a.ii:
What does represent in this context?
-
22M.2.AHL.TZ2.5d.i:
when .
-
22M.2.AHL.TZ2.5d.ii:
in the long term.
