| Date | May 2019 | Marks available | 2 | Reference code | 19M.2.AHL.TZ2.H_3 |
| Level | Additional Higher Level | Paper | Paper 2 | Time zone | Time zone 2 |
| Command term | Calculate | Question number | H_3 | Adapted from | N/A |
Question
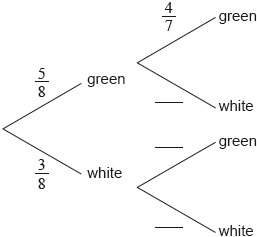
Iqbal attempts three practice papers in mathematics. The probability that he passes the first paper is 0.6. Whenever he gains a pass in a paper, his confidence increases so that the probability of him passing the next paper increases by 0.1. Whenever he fails a paper the probability of him passing the next paper is 0.6.
Complete the given probability tree diagram for Iqbal’s three attempts, labelling each branch with the correct probability.
Calculate the probability that Iqbal passes at least two of the papers he attempts.
Find the probability that Iqbal passes his third paper, given that he passed only one previous paper.
Markscheme
* This question is from an exam for a previous syllabus, and may contain minor differences in marking or structure.
A1A1A1
Note: Award A1 for each correct column of probabilities.
[3 marks]
probability (at least twice) =
EITHER
(0.6×0.7×0.8)+(0.6×0.7×0.2)+(0.6×0.3×0.6)+(0.4×0.6×0.7)(0.6×0.7×0.8)+(0.6×0.7×0.2)+(0.6×0.3×0.6)+(0.4×0.6×0.7) (M1)
OR
(0.6×0.7)+(0.6×0.3×0.6)+(0.4×0.6×0.7)(0.6×0.7)+(0.6×0.3×0.6)+(0.4×0.6×0.7) (M1)
Note: Award M1 for summing all required probabilities.
THEN
= 0.696 A1
[2 marks]
P(passes third paper given only one paper passed before)
=P(passes third AND only one paper passed before)P(passes once in first two papers)=P(passes third AND only one paper passed before)P(passes once in first two papers) (M1)
=(0.6×0.3×0.6)+(0.4×0.6×0.7)(0.6×0.3)+(0.4×0.6)=(0.6×0.3×0.6)+(0.4×0.6×0.7)(0.6×0.3)+(0.4×0.6) A1
= 0.657 A1
[3 marks]