IB Questionbank

User interface language: English | Español

Date	November 2018	Marks available	7	Reference code	18N.1.SL.TZ0.S_9
Level	Standard Level	Paper	Paper 1	Time zone	Time zone 0
Command term	Find	Question number	S_9	Adapted from	N/A

Question

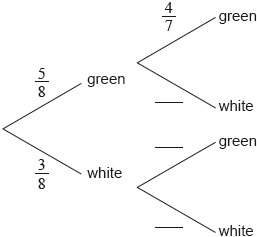
A bag contains $n$ $n$ marbles, two of which are blue. Hayley plays a game in which she randomly draws marbles out of the bag, one after another, without replacement. The game ends when Hayley draws a blue marble.

Let $n$ $n$ = 5. Find the probability that the game will end on her

Find the probability, in terms of $n$ $n$ , that the game will end on her first draw.

[1]

a.i.

Find the probability, in terms of $n$ $n$ , that the game will end on her second draw.

[3]

a.ii.

third draw.

[2]

b.i.

fourth draw.

[2]

b.ii.

Hayley plays the game when $n$ $n$ = 5. She pays $20 to play and can earn money back depending on the number of draws it takes to obtain a blue marble. She earns no money back if she obtains a blue marble on her first draw. Let M be the amount of money that she earns back playing the game. This information is shown in the following table.

Find the value of $k$ $k$ so that this is a fair game.

[7]

Markscheme

$\frac{2}{n}$ $\frac{2}{n}$ A1 N1

[1 mark]

a.i.

correct probability for one of the draws A1

eg P(not blue first) = $\frac{n - 2}{n}$ $\frac{{n - 2}}{n}$ , blue second = $\frac{2}{n - 1}$ $\frac{2}{{n - 1}}$

valid approach (M1)

eg recognizing loss on first in order to win on second, P(B' then B), P(B') × P(B | B'), tree diagram

correct expression in terms of $n$ $n$ A1 N3

eg $\frac{n - 2}{n} \times \frac{2}{n - 1}$ $\frac{{n - 2}}{n} \times \frac{2}{{n - 1}}$ , $\frac{2 n - 4}{n^{2} - n}$ $\frac{{2n - 4}}{{{n^2} - n}}$ , $\frac{2 (n - 2)}{n (n - 1)}$ $\frac{{2\left( {n - 2} \right)}}{{n\left( {n - 1} \right)}}$

[3 marks]

a.ii.

correct working (A1)

eg $\frac{3}{5} \times \frac{2}{4} \times \frac{2}{3}$ $\frac{3}{5} \times \frac{2}{4} \times \frac{2}{3}$

$\frac{12}{60} (= \frac{1}{5})$ $\frac{{12}}{{60}}\,\,\,\left( { = \frac{1}{5}} \right)$ A1 N2

[2 marks]

b.i.

correct working (A1)

eg $\frac{3}{5} \times \frac{2}{4} \times \frac{1}{3} \times \frac{2}{2}$ $\frac{3}{5} \times \frac{2}{4} \times \frac{1}{3} \times \frac{2}{2}$

$\frac{6}{60} (= \frac{1}{10})$ $\frac{6}{{60}}\,\,\,\left( { = \frac{1}{{10}}} \right)$ A1 N2

[2 marks]

b.ii.

correct probabilities (seen anywhere) (A1)(A1)

eg $P (1) = \frac{2}{5}$ ${\text{P}}\left( {\text{1}} \right) = \frac{2}{5}$ , $P (2) = \frac{6}{20}$ ${\text{P}}\left( {\text{2}} \right) = \frac{6}{{20}}$ (may be seen on tree diagram)

valid approach to find E (M) or expected winnings using their probabilities (M1)

eg $P (1) \times (0) + P (2) \times (20) + P (3) \times (8 k) + P (4) \times (12 k)$ ${\text{P}}\left( {\text{1}} \right) \times \left( 0 \right) + {\text{P}}\left( {\text{2}} \right) \times \left( {20} \right) + {\text{P}}\left( {\text{3}} \right) \times \left( {8k} \right) + {\text{P}}\left( {\text{4}} \right) \times \left( {12k} \right)$ ,

$P (1) \times (- 20) + P (2) \times (0) + P (3) \times (8 k - 20) + P (4) \times (12 k - 20)$ ${\text{P}}\left( {\text{1}} \right) \times \left( { - 20} \right) + {\text{P}}\left( {\text{2}} \right) \times \left( 0 \right) + {\text{P}}\left( {\text{3}} \right) \times \left( {8k - 20} \right) + {\text{P}}\left( {\text{4}} \right) \times \left( {12k - 20} \right)$

correct working to find E (M) or expected winnings (A1)

eg $\frac{2}{5} (0) + \frac{3}{10} (20) + \frac{1}{5} (8 k) + \frac{1}{10} (12 k)$ $\frac{2}{5}\left( 0 \right) + \frac{3}{{10}}\left( {20} \right) + \frac{1}{5}\left( {8k} \right) + \frac{1}{{10}}\left( {12k} \right)$ ,

$\frac{2}{5} (- 20) + \frac{3}{10} (0) + \frac{1}{5} (8 k - 20) + \frac{1}{10} (12 k - 20)$ $\frac{2}{5}\left( { - 20} \right) + \frac{3}{{10}}\left( 0 \right) + \frac{1}{5}\left( {8k - 20} \right) + \frac{1}{{10}}\left( {12k - 20} \right)$

correct equation for fair game A1

eg $\frac{3}{{10}}\left( {20} \right) + \frac{1}{5}\left( {8k} \right) + \frac{1}{{10}}\left( {12k} \right) = 20$ , $\frac{2}{5}\left( { - 20} \right) + \frac{1}{5}\left( {8k - 20} \right) + \frac{1}{{10}}\left( {12k - 20} \right) = 0$