IB Questionbank

User interface language: English | Español

Date	November 2016	Marks available	2	Reference code	16N.1.AHL.TZ0.H_2
Level	Additional Higher Level	Paper	Paper 1	Time zone	Time zone 0
Command term	Complete	Question number	H_2	Adapted from	N/A

Question

The faces of a fair six-sided die are numbered 1, 2, 2, 4, 4, 6. Let $X$ $X$ be the discrete random variable that models the score obtained when this die is rolled.

Complete the probability distribution table for $X$ $X$ .

N16/5/MATHL/HP1/ENG/TZ0/02.a

[2]

Find the expected value of $X$ $X$ .

[2]

Markscheme

* This question is from an exam for a previous syllabus, and may contain minor differences in marking or structure.

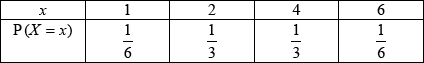N16/5/MATHL/HP1/ENG/TZ0/02.a/M A1A1

Note: Award A1 for each correct row.

[2 marks]

$E (X) = 1 \times \frac{1}{6} + 2 \times \frac{1}{3} + 4 \times \frac{1}{3} + 6 \times \frac{1}{6}$ ${\text{E}}(X) = 1 \times \frac{1}{6} + 2 \times \frac{1}{3} + 4 \times \frac{1}{3} + 6 \times \frac{1}{6}$ (M1)

$= \frac{19}{6} (= 3 \frac{1}{6})$ $= \frac{{19}}{6}{\text{ }}\left( { = 3\frac{1}{6}} \right)$ A1

Note: If the probabilities in (a) are not values between 0 and 1 or lead to $E (X) > 6$ ${\text{E}}(X) > 6$ award M1A0 to correct method using the incorrect probabilities; otherwise allow FT marks.

[2 marks]

Examiners report

[N/A]

Syllabus sections

Topic 4—Statistics and probability » SL 4.7—Discrete random variables

Show 78 related questions

19M.2.AHL.TZ2.H_10b:
Copy and complete the probability distribution table for Y.
18M.2.SL.TZ2.S_10d.i:
Find the expected number of bags in this crate that contain at most one small apple.
20N.2.SL.TZ0.S_3b.ii:
Hence, find the largest value of $E (X)$ $E (X)$ .
17M.1.AHL.TZ1.H_10a:
Find the value of $k$ $k$ .
17M.1.AHL.TZ1.H_10b.i:
By considering the graph of f write down the mean of $X$ $X$ ;
18M.2.AHL.TZ1.H_10b:
Find $P (X < 1)$ ${\text{P}}\left( {X < 1} \right)$ .
17M.2.SL.TZ2.S_10b.i:
Write down the probability of drawing three blue marbles.
18M.2.SL.TZ2.S_10d.ii:
Find the probability that at least 48 bags in this crate contain at most one small apple.
SPM.1.SL.TZ0.12a:
Find the exact value of $p$ $p$ .
19M.2.SL.TZ1.S_10c.i:
Write down the value of $x$ $x$ .
18M.2.SL.TZ1.S_2c:
The die is rolled 80 times. On how many rolls would you expect to obtain a three?
19M.2.SL.TZ1.S_10c.ii:
Hence, find the value of $y$ $y$ .
17N.2.SL.TZ0.S_4b:
Write down $P (X = 2)$ ${\text{P}}(X = 2)$ .
17M.2.AHL.TZ2.H_10c:
Find $Var (X)$ ${\text{Var}}(X)$ .
17M.2.AHL.TZ2.H_10f:
Find ${\text{P}}(Y \geqslant 3)$ .
16N.2.AHL.TZ0.H_1a:
Determine the value of ${\text{E}}({X^2})$ .
17M.2.SL.TZ1.S_4b.ii:
Hence, find the probability that fewer than $k$ students are left handed.
18N.1.SL.TZ0.S_9c:
Hayley plays the game when $n$ = 5. She pays $20 to play and can earn money back depending on the number of draws it takes to obtain a blue marble. She earns no money back if she obtains a blue marble on her first draw. Let M be the amount of money that she earns back playing the game. This information is shown in the following table.

Find the value of $k$ so that this is a fair game.
17M.2.AHL.TZ2.H_10e:
Find ${\text{E}}(Y)$ .
19M.2.SL.TZ1.S_10a.ii:
Find the probability of rolling two or more red faces.
19M.2.SL.TZ1.S_10a.i:
Find the probability of rolling exactly one red face.
17M.1.AHL.TZ1.H_10b.ii:
By considering the graph of f write down the median of $X$ ;
17M.2.SL.TZ1.S_4b.i:
Hence, find the probability that exactly $k$ students are left handed;
16N.1.AHL.TZ0.H_1:
Find the coordinates of the point of intersection of the planes defined by the equations $x + y + z = 3,{\text{ }}x - y + z = 5$ and $x + y + 2z = 6$ .
17M.2.AHL.TZ2.H_10b:
Find ${\text{E}}(X)$ .
17N.2.SL.TZ0.S_4a:
Find the value of $k$ .
16N.1.AHL.TZ0.H_2b:
Find the expected value of $X$ .
18N.1.SL.TZ0.S_9a.i:
Find the probability, in terms of $n$ , that the game will end on her first draw.
18M.2.SL.TZ1.S_2a:
Find the value of k.
16N.2.AHL.TZ0.H_1b:
Find the value of ${\text{Var}}(X)$ .
19M.2.AHL.TZ2.H_10a:
Find the probability that on a randomly selected day, Steffi does not visit Will’s house.
17M.2.AHL.TZ2.H_1a:
One of the players is chosen at random. Find the probability that this player’s score was 5 or more.
18N.1.SL.TZ0.S_9b.ii:
fourth draw.
18M.1.AHL.TZ1.H_3b:
Find the expected value of T.
SPM.1.SL.TZ0.12b:
Calculate the expected score.
18M.2.AHL.TZ1.H_10a:
Show that $a = \frac{2}{3}$ .
16N.2.SL.TZ0.S_7a:
Write down the probability that the first disc selected is red.
17M.2.SL.TZ1.S_4a:
Find $k$ .
18M.1.AHL.TZ1.H_3a:
Find the value of a and the value of b.
20N.2.SL.TZ0.S_3a:
Find an expression for $q$ in terms of $p$ .
20N.2.SL.TZ0.S_3b.i:
Find the value of $p$ which gives the largest value of $E (X)$ .
17M.2.SL.TZ2.S_10d:
Grant plays the game until he wins two prizes. Find the probability that he wins his second prize on his eighth attempt.
EXN.1.SL.TZ0.12a:
Write down the value of $Σ_{i = 1}^{9} u_{i}$ .
EXN.1.SL.TZ0.12b:
Find the value of $u_{1}$ .
18M.2.SL.TZ2.S_10a.ii:
Show that μ = 106.
19M.2.SL.TZ1.S_10b:
Show that, after a turn, the probability that Ted adds exactly $10 to his winnings is ${\frac{1}{3}}$ .
17N.2.SL.TZ0.S_4c:
Find ${\text{P}}(X = 2|X > 0)$ .
17M.2.SL.TZ2.S_10a.i:
Find $q$ .
19M.2.AHL.TZ2.H_10d:
In any given year of 365 days, the probability that Steffi does not visit Will for at most $n$ days in total is 0.5 (to one decimal place). Find the value of $n$ .
18M.2.SL.TZ2.S_10b:
Find P(M < 95) .
17M.2.SL.TZ2.S_B10c:
Jill plays the game nine times. Find the probability that she wins exactly two prizes.
18N.1.SL.TZ0.S_9b.i:
third draw.
17M.2.SL.TZ2.S_10b.ii:
Explain why the probability of drawing three white marbles is $\frac{1}{6}$ .
21M.1.SL.TZ1.10a:
Complete the table to show the probability distribution of $T$ .
21M.1.SL.TZ1.10b.i:
a player scores at least $3$ in a game.
21M.1.SL.TZ1.10b.ii:
a player scores $6$ , given that they scored at least $3$ .
18M.2.AHL.TZ1.H_10c:
Given that ${\text{P}}\left( {s < X < 0.8} \right) = 2 \times {\text{P}}\left( {2s < X < 0.8} \right)$ , and that 0.25 < s < 0.4 , find the value of s.
21M.1.SL.TZ1.10c:
Find the expected score of a game.
18M.2.SL.TZ2.S_10c:
Find the probability that a bag of apples selected at random contains at most one small apple.
17M.2.SL.TZ2.S_10a.ii:
Find $p$ .
18M.2.SL.TZ1.S_2b:
Calculate the expected value of the score.
18M.2.SL.TZ2.S_10a.i:
Write down the value of k.
17M.2.AHL.TZ2.H_10a:
Show that $a = 32$ and $b = \frac{1}{{12}}$ .
17M.2.AHL.TZ2.H_1b:
Calculate the mean score.
19M.2.AHL.TZ2.H_10e:
Show that the expected number of occasions per year on which Steffi visits Will’s house and is not fed is at least 30.
16N.2.SL.TZ0.S_7b:
Let $X$ be the number of red discs selected. Find the smallest value of $m$ for which ${\text{Var}}(X{\text{ }}) < 0.6$ .
19M.2.AHL.TZ2.H_10c:
Hence find the expected number of times per day that Steffi is fed at Will’s house.
SPM.1.SL.TZ0.12c:
Jae Hee plays the game twice and adds the two scores together.

Find the probability Jae Hee has a total score of −3.
17M.2.SL.TZ2.S_10b.iii:
The bag contains a total of ten marbles of which $w$ are white. Find $w$ .
17M.2.AHL.TZ2.H_10d:
Find the median of $X$ .
18N.1.SL.TZ0.S_9a.ii:
Find the probability, in terms of $n$ , that the game will end on her second draw.
17M.1.AHL.TZ1.H_10b.iii:
By considering the graph of f write down the mode of $X$ .
17M.1.AHL.TZ1.H_10d:
Calculate $P(X \leqslant 4|X \geqslant 3)$ .
EXN.2.SL.TZ0.4a:
Find the probability of scoring a six when rolling the novelty die.
19M.2.SL.TZ1.S_10d:
Ted will always have another turn if he expects an increase to his winnings.

Find the least value of $w$ for which Ted should end the game instead of having another turn.
17M.1.AHL.TZ1.H_10c.i:
Show that $P(0 \leqslant X \leqslant 2) = \frac{1}{4}$ .
EXN.1.SL.TZ0.12c:
A game is played in which the arrow attached to the centre of the disc is spun and the sector in which the arrow stops is noted. If the arrow stops in sector $1$ the player wins $10$ points, otherwise they lose $2$ points.

Let $X$ be the number of points won

Find $E (X)$ .
17M.1.AHL.TZ1.H_10c.ii:
Hence state the interquartile range of $X$ .

Hide related questions

Topic 4—Statistics and probability

Question

Markscheme

Examiners report

Syllabus sections

View options