5.9.1 Integrating Further Functions

As with other problems in integration the results in this revision note may have further uses such as

evaluating a definite integral
finding the constant of integration
finding areas under a curve, between a line and a curve or between two curves

Integrating with Reciprocal Trigonometric Functions

cosec (cosecant, csc), sec (secant) and cot (cotangent) are the reciprocal functions of sine, cosine and tangent respectively.

What are the antiderivatives involving reciprocal trigonometric functions?

$\int_{}^{} \sec^{2} x d x = \tan x + c$
$\int_{}^{} \sec x \tan x d x = \sec x + c$
$\int_{}^{} cosec x \cot x d x = - cosec x + c$
$\int_{}^{} {cosec}^{2} x d x = - \cot x + c$
These are not given in the formula booklet directly

they are listed the other way round as ‘standard derivatives’
be careful with the negatives in the last two results
and remember “+c” !

**How do I integrate these if a linear function of x is involved?**

All integration rules could apply alongside the results above
The use of reverse chain rule is particularly common
- For linear functions the following results can be useful
  - $\int_{}^{} \sec (a x + b) \tan (a x + b) d x = \frac{1}{a} \sec (a x + b) + c$
  - $\int_{}^{} cosec (a x + b) \cot (a x + b) d x = - \frac{1}{a} cosec (a x + b) + c$
  - $\int_{}^{} \cot (a x + b) d x = - \frac{1}{a} {cosec}^{2} (a x + b) + c$
- These are not in the formula booklet
  - they can be deduced by spotting reverse chain rule
  - they are not essential to remember but can make problems easier

Exam Tip

Even if you think you have remembered these antiderivatives, always use the formula booklet to double check
- those squares, negatives and "1 over"'s are easy to get muddled up!
Remember to use 'adjust' and 'compensate' for reverse chain rule when coefficients are involved

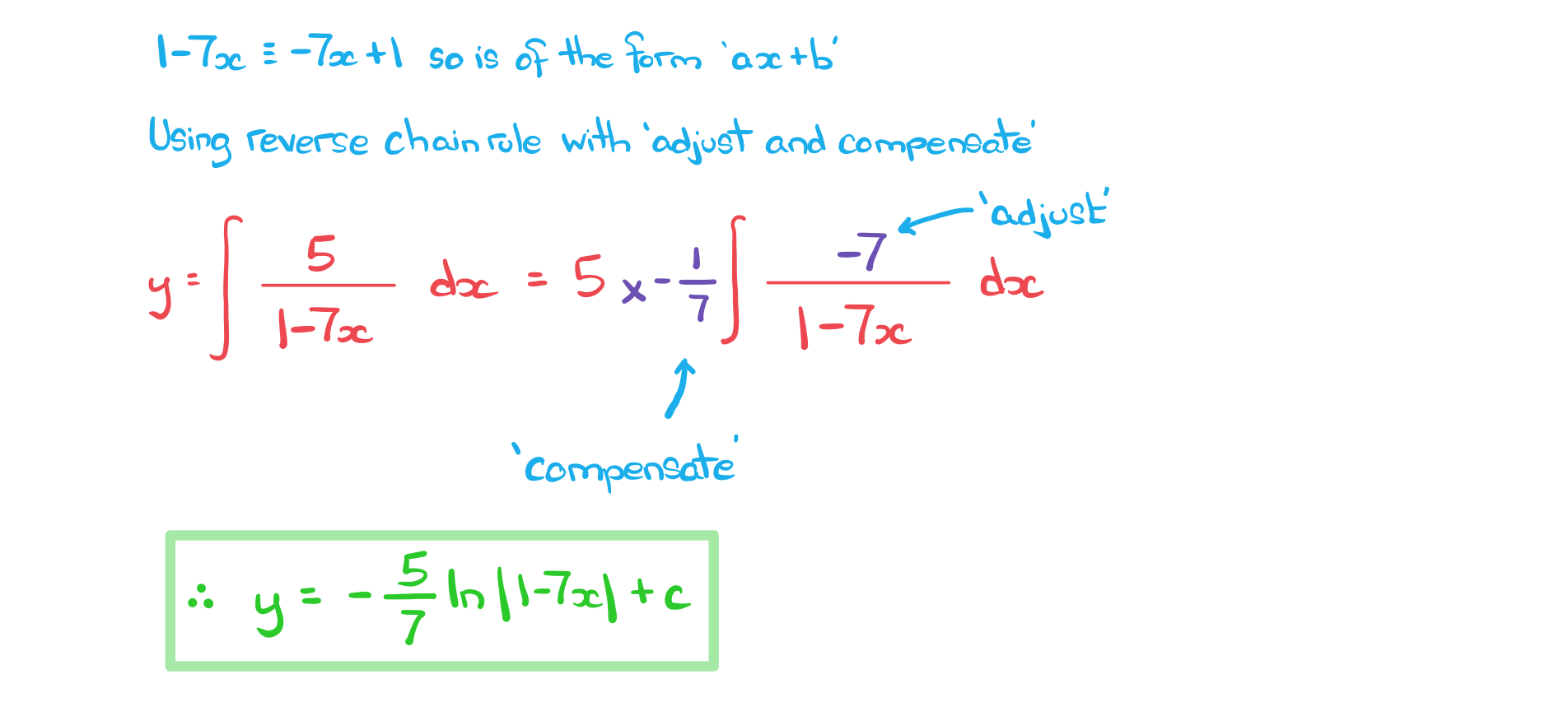
Worked Example

The graph of $y = f (x)$ where $f (x) = \int_{}^{} 2 \sec^{2} 5 x d x$ passes through the point $(\frac{π}{3}, 0)$ .

Show that $5 y = 2 (\sqrt{3} + \tan 5 x)$ .

5-9-1-ib-hl-aa-only-we1-soltn

Integrating with Inverse Trigonometric Functions

arcsin, arccos and arctan are (one-to-one) functions defined as the inverse functions of sine, cosine and tangent respectively.

What are the antiderivatives involving the inverse trigonometric functions?

$\int_{}^{} \frac{1}{\sqrt{1 - x^{2}}} d x = \arcsin x + c$
$\int_{}^{} \frac{1}{1 + x^{2}} d x = \arctan x + c$
Note that the antiderivative involving $\arccos x$ would arise from

$\int_{}^{} - \frac{1}{\sqrt{1 - x^{2}}} d x = \arccos x + c$

- However, the negative can be treated as a coefficient of -1 and so

$\int_{}^{} - \frac{1}{\sqrt{1 - x^{2}}} d x = - \int_{}^{} \frac{1}{\sqrt{1 - x^{2}}} d x = - \arcsin x + c$

- Similarly,

$\int_{}^{} \frac{1}{\sqrt{1 - x^{2}}} d x = - \int_{}^{} - \frac{1}{\sqrt{1 - x^{2}}} d x = - \arccos x + c$

Unless a question requires otherwise, stick to the first two results
These are listed in the formula booklet the other way round as ‘standard derivatives’
For the antiderivative involving $\arctan x$ , note that $(1 + x^{2})$ is the same as $(x^{2} + 1)$

How do I integrate these expressions if the denominator is not in the correct form?

Some problems involve integrands that look very similar to the above but the denominators start with a number other than one
- denominators of the form $a^{2} \pm {(b x)}^{2}$ (with or without the square root!)
- the integrand can be rewritten by taking a factor of $a^{2}$
  - this means the denominator will start with 1
  - e.g. $9 + 4 x^{2} = 9 (1 + \frac{4}{9} x^{2}) = 9 (1 + {(\frac{2}{3} x)}^{2})$
  - $\frac{2}{3} x$ is a linear function of $x$ , so use ‘adjust and compensate’
- Another type of problem has a quadratic denominator
  - denominators of the form $a x^{2} + b x + c$
    - a rearrangement of this is more likely but it is still quadratic
  - the integrand can be rewritten by completing the square
  - e.g. $5 - x^{2} + 4 x = 5 - (x^{2} + 4 x) = 5 - [{(x + 2)}^{2} - 4] = 9 - {(x + 2)}^{2}$
  - This can then be dealt with like the first type of problem above

Exam Tip

Always start integrals involving the inverse trig functions by rewrtiting the denominator into the correct form
- With or without the square root, the denominator should be of the form $1 \pm {[g (x)]}^{2}$
- The numerator can be dealt with afterwards using 'adjust' and 'compensate' if necessary

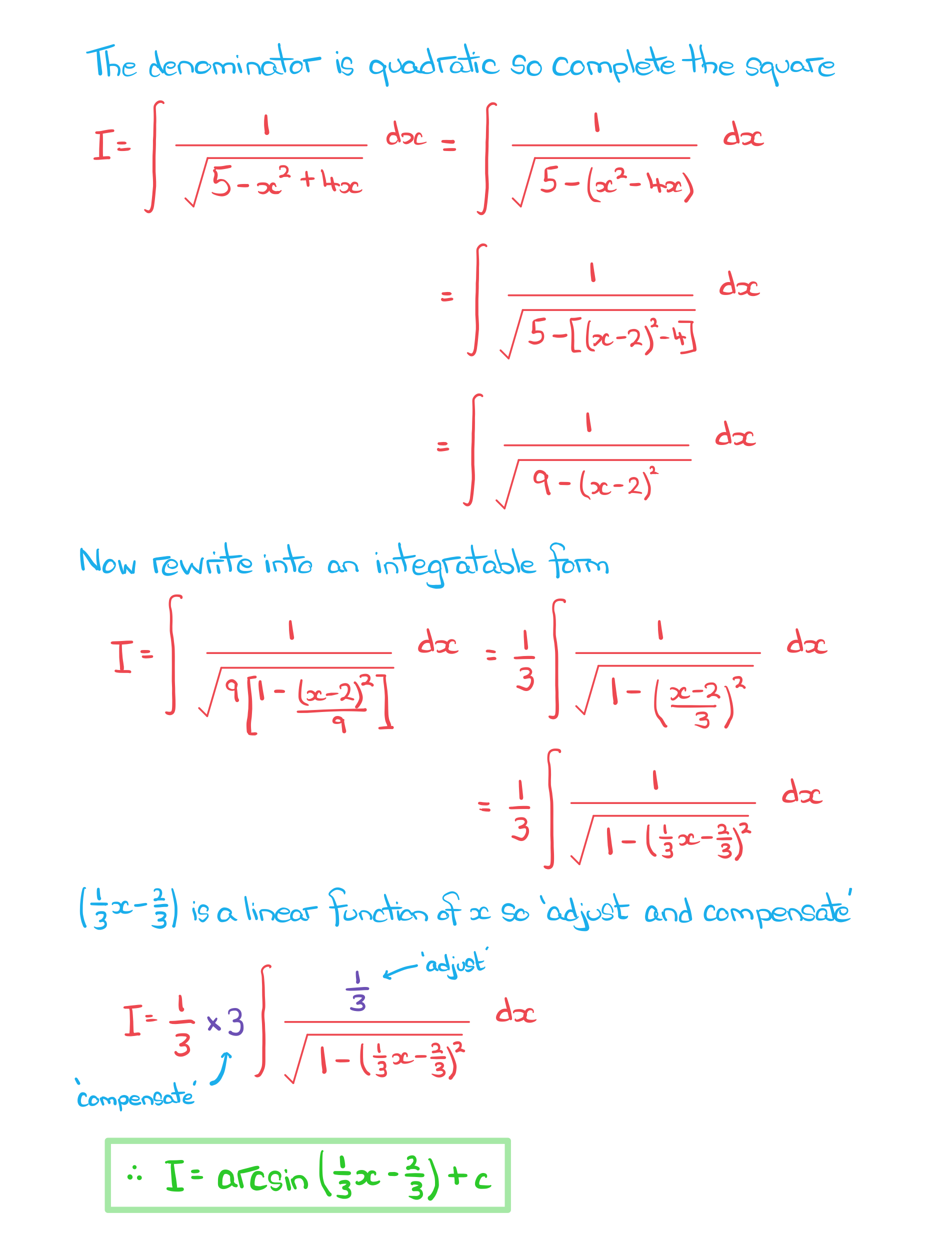
Worked Example

a) Find

\int_{}^{} \frac{1}{9 + 4 x^{2}} d x

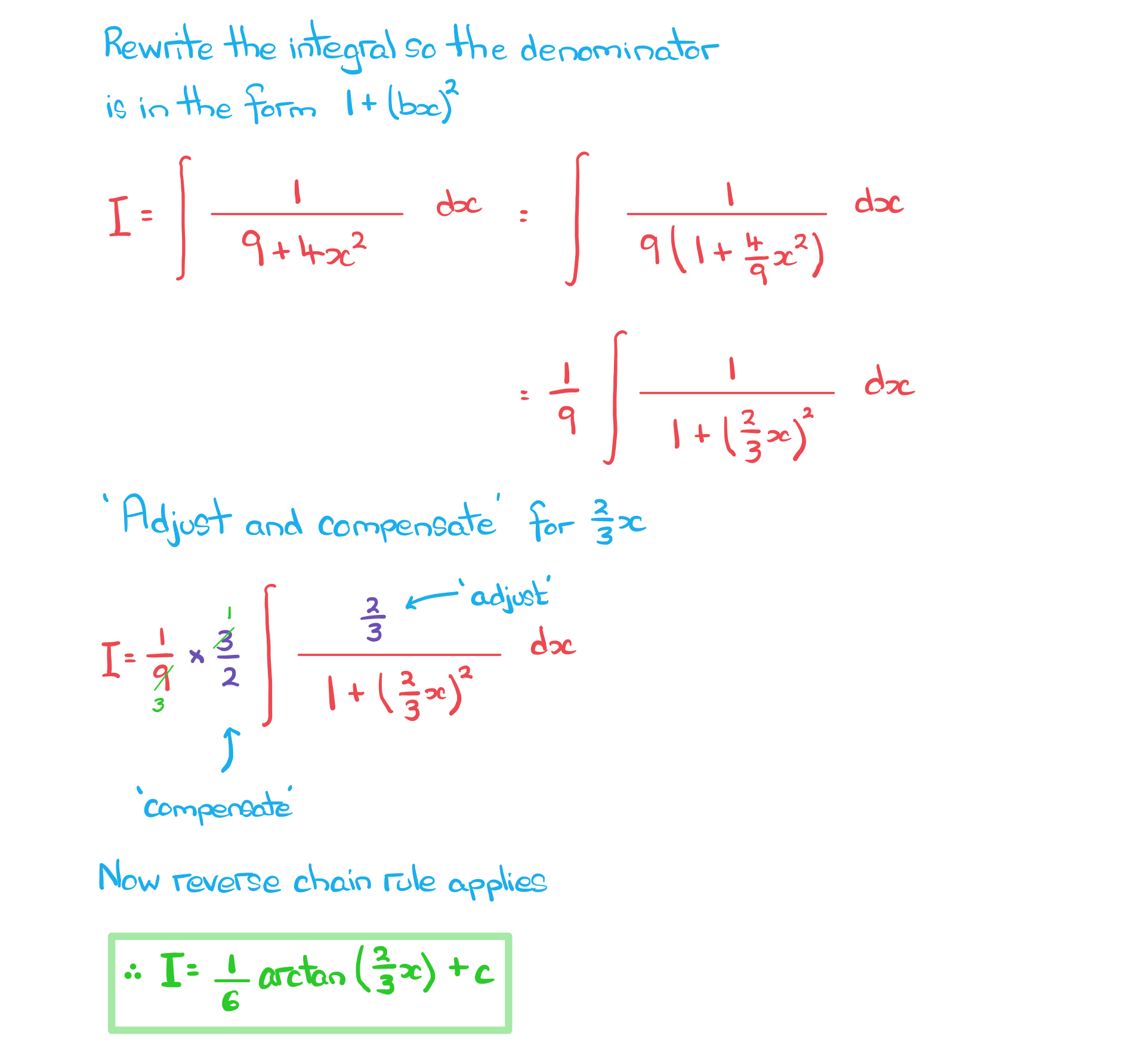
5-9-1-ib-hl-aa-only-we2a-soltn

b) Find $\int_{}^{} \frac{1}{\sqrt{5 - x^{2} + 4 x}} d x$ .

5-9-1-ib-hl-aa-only-we2b-soltn

Integrating Exponential & Logarithmic Functions

Exponential functions have the general form $y = a^{x}$ . Special case: $y = e^{x}$ .

Logarithmic functions have the general form $y = \log_{a} x$ . Special case: $y = \log_{e} x = \ln x$ .

What are the antiderivatives of exponential and logarithmic functions?

Those involving the special cases have been met before
- $\int_{}^{} e^{x} d x = e^{x} + c$
- $\int_{}^{} \frac{1}{x} d x = \ln | x | + c$
- These are given in the formula booklet
Also
- $\int_{}^{} a^{x} d x = \frac{1}{\ln a} a^{x} + c$
- This is also given in the formula booklet
By reverse chain rule
- $\int_{}^{} \frac{1}{x \ln a} d x = \log_{a} | x | + c$
- This is not in the formula booklet
There is also the reverse chain rule to look out for
- this occurs when the numerator is (almost) the derivative of the denominator
- $\int_{}^{} \frac{f' (x)}{f (x)} d x = \ln |f (x)| + c$

**How do I integrate exponentials and logarithms with a linear function of x involved?**

In the case of linear functions of the form $a x + b$

$\int_{}^{} e^{a x + b} d x = \frac{1}{a} e^{a x + b} + c$
$\int_{}^{} \frac{1}{a x + b} d x = \frac{1}{a} \ln |a x + b| + c$
These are not in the formula booklet but can be derived using ‘adjust and compensate’ from reverse chain rule

Exam Tip

Remember to always use the modulus signs for logarithmic terms in the antiderivative
- Once it is deduced that $g (x)$ in $\ln | g (x) |$ (say) is guaranteed to be positive, the modulus signs can be replaced with brackets

Worked Example

a) Find $\int_{}^{} 3^{2 x + 4} d x$ .

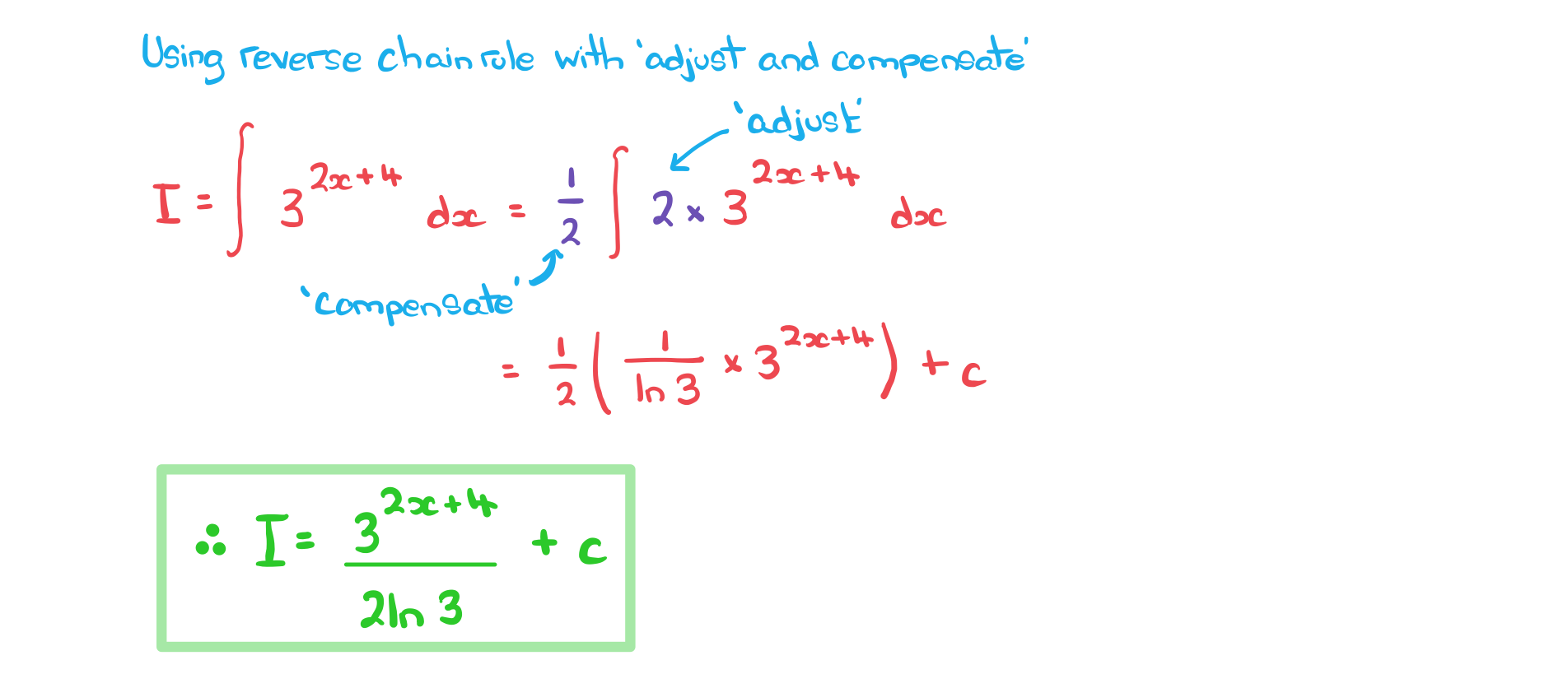
5-9-1-ib-hl-aa-only-we3a-soltn

b) Given $\frac{d y}{d x} = \frac{5}{1 - 7 x}$ show that $y = - \frac{5}{7} \ln |1 - 7 x| + c$ , where $c$ is a constant.

5-9-1-ib-hl-aa-only-we3b-soltn

DP IB Maths: AA HL

Revision Notes

5.9.1 Integrating Further Functions

Integrating with Reciprocal Trigonometric Functions

What are the antiderivatives involving reciprocal trigonometric functions?

**How do I integrate these if a linear function of x is involved?**

Exam Tip

Worked Example

Integrating with Inverse Trigonometric Functions

What are the antiderivatives involving the inverse trigonometric functions?

How do I integrate these expressions if the denominator is not in the correct form?

Exam Tip

Worked Example

Integrating Exponential & Logarithmic Functions

What are the antiderivatives of exponential and logarithmic functions?

**How do I integrate exponentials and logarithms with a linear function of x involved?**

Exam Tip

Worked Example

Author: Paul

Resources

Members

Company

Quick Links