5.8.3 Implicit Differentiation

Implicit Differentiation

What is implicit differentiation?

An equation connecting x and y is not always easy to write explicitly in the form $y = f (x)$ or $x = f (y)$
- In such cases the equation is written implicitly
  - as a function of $x$ and $y$
  - in the form $f (x, y) = 0$
Such equations can be differentiated implicitly using the chain rule

$\frac{d}{d x} [f (y)] = f' (y) \frac{d y}{d x}$

A shortcut way of thinking about this is that ‘ $y$ is a function of a $x$ ’
- when differentiating a function of $y$ chain rule says “differentiate with respect to $y$ , then multiply by the derivative of $y$ ” (which is $\frac{d y}{d x}$ )

Applications of Implicit Differentiation

What type of problems could involve implicit differentiation?

Broadly speaking there are three types of problem that could involve implicit differentiation
- algebraic problems involving graphs, derivatives, tangents, normals, etc
  - where it is not practical to write $y$ explicitly in terms of $x$
  - usually in such cases, $\frac{d y}{d x}$ will be in terms of $x$ and $y$
- optimisation problems that involve time derivatives
  - more than one variable may be involved too

e.g. Volume of a cylinder, $V = π r^{2} h$

e.g. The side length and (so) area of a square increase over time

- any problem that involves differentiating with respect to an extraneous variable
  - e.g. $y = f (x)$ but the derivative $\frac{d y}{d θ}$ is required (rather than $\frac{d y}{d x}$ )

How do I apply implicit differentiation to algebraic problems?

Algebraic problems revolve around values of the derivative (gradient) $(\frac{d y}{d x})$
- if not required to find this value it will either be given or implied
Particular problems focus on special case tangent values
- horizontal tangents
  - also referred to as tangents parallel to the $x$ -axis
  - this is when $\frac{d y}{d x} = 0$
- vertical tangents
  - also referred to as tangents parallel to the $y$ -axis
  - this is when $\frac{d x}{d y} = 0$
  - In such cases it may appear that $\frac{1}{\frac{d y}{d x}} = 0$ but this has no solutions; this occurs when for nearby values of $x$ , $\frac{d y}{d x} \to \pm \infty$

(i.e. very steep gradients, near vertical)

Other problems may involve finding equations of (other) tangents and/or normals
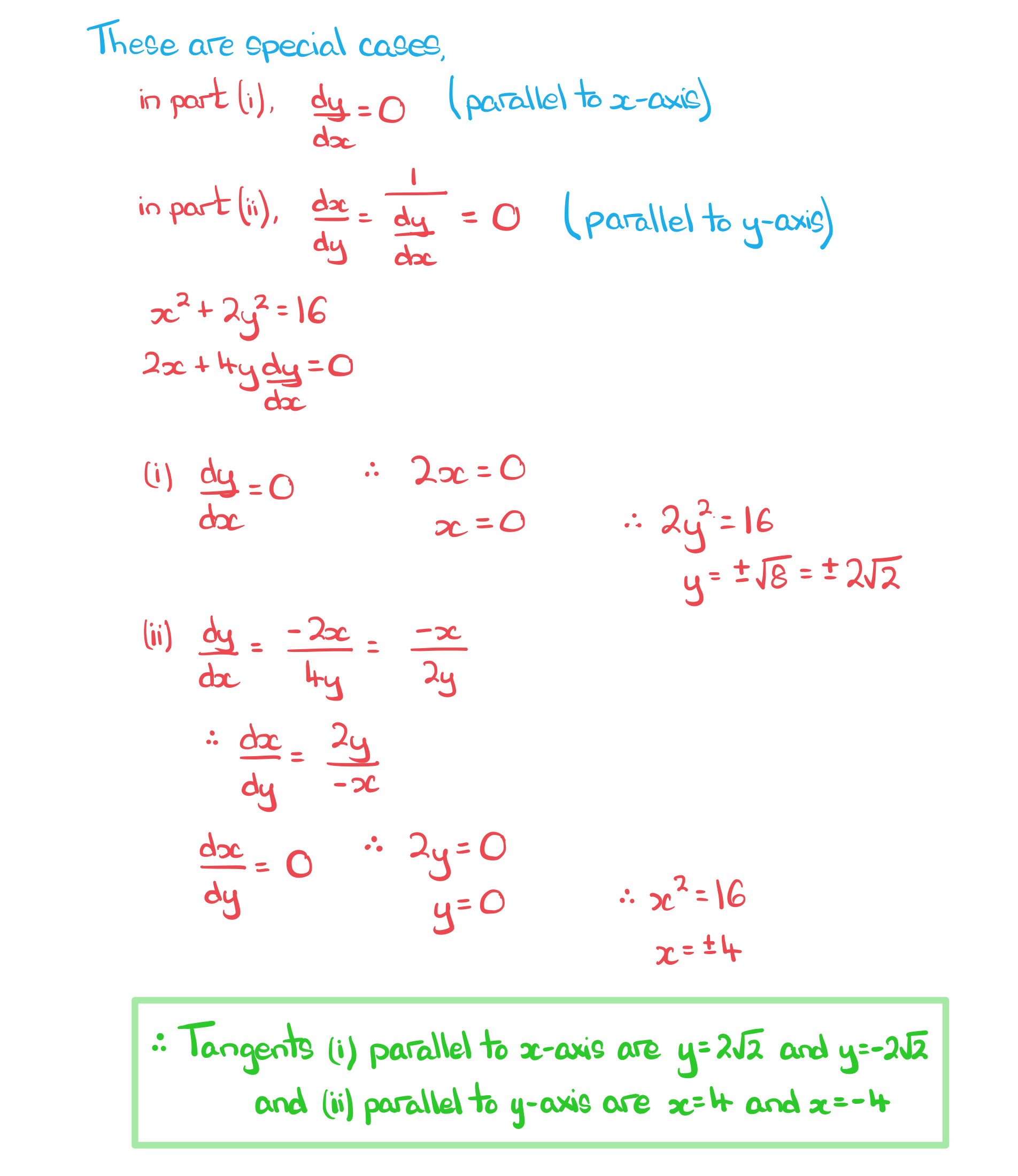
For problems that involve finding the coordinates of points on a curve with a specified gradient the method below can be used

STEP 1
Differentiate the equation of the curve implicitly

STEP 2
Substitute the given or implied value of $\frac{d y}{d x}$ to create an equation linking $x$ and $y$

STEP 3
There are now two equations

- the original equation
- the linking equation

Solve them simultaneously to find the $x$ and $y$ coordinates as required

Exam Tip

After some rearranging, $\frac{d y}{d x}$ will be in terms of both $x$ and $y$
- There is usually no need (unless asked to by the question) to write $\frac{d y}{d x}$ in terms of $x$ (or $y$ ) only
If evalutaing derivatives, you'll need both $x$ and $y$ coordinates, so one may have to be found from the other using the original function

Worked Example

The curve C has equation $x^{2} + 2 y^{2} = 16$ .

Find the exact coordinates of the points where the normal to curve C has gradient 2.

5-8-2-ib-hl-aa-only-we3a-soltn

Find the equations of the tangents to the curve that are

(i) parallel to the x-axis

(ii) parallel to the y-axis.

5-8-2-ib-hl-aa-only-we3b-soltn

How do I apply implicit differentiation to optimisation problems?

For a single variable use chain rule to differentiate implicitly
- e.g. A square with side length changing over time, $A = x^{2}$

$\frac{d A}{d t} = 2 x \frac{d x}{d t}$

For more than one variable use product rule (and chain rule) to differentiate implicitly
- e.g. A square-based pyramid with base length and height changing over time, $V = \frac{1}{3} x^{2} h$

$\frac{d V}{d t} = \frac{1}{3} [x^{2} \frac{d h}{d t} + 2 x \frac{d x}{d t} h] = \frac{1}{3} x (x \frac{d h}{d t} + 2 h \frac{d x}{d t})$

After differentiating implicitly the rest of the question should be similar to any other optimisation problem
- be aware of phrasing
  - “the rate of change of the height of the pyramid” (over time) is $\frac{d h}{d t}$
- when finding the location of minimum and maximum problems
  - there is not necessarily a turning point
  - the minimum or maximum could be at the start or end of a given or appropriate interval

Exam Tip

If you are struggling to tell which derivative is needed for a question, writing all possibilities down may help you
- You don't need to work them out at this stage but if you conisder them it may nudge you to the next stage of the solution
- e.g. For $V = π r^{2} h$ , possible derivatives are $\frac{d V}{d r}, \frac{d V}{d h}$ and $\frac{d V}{d t}$

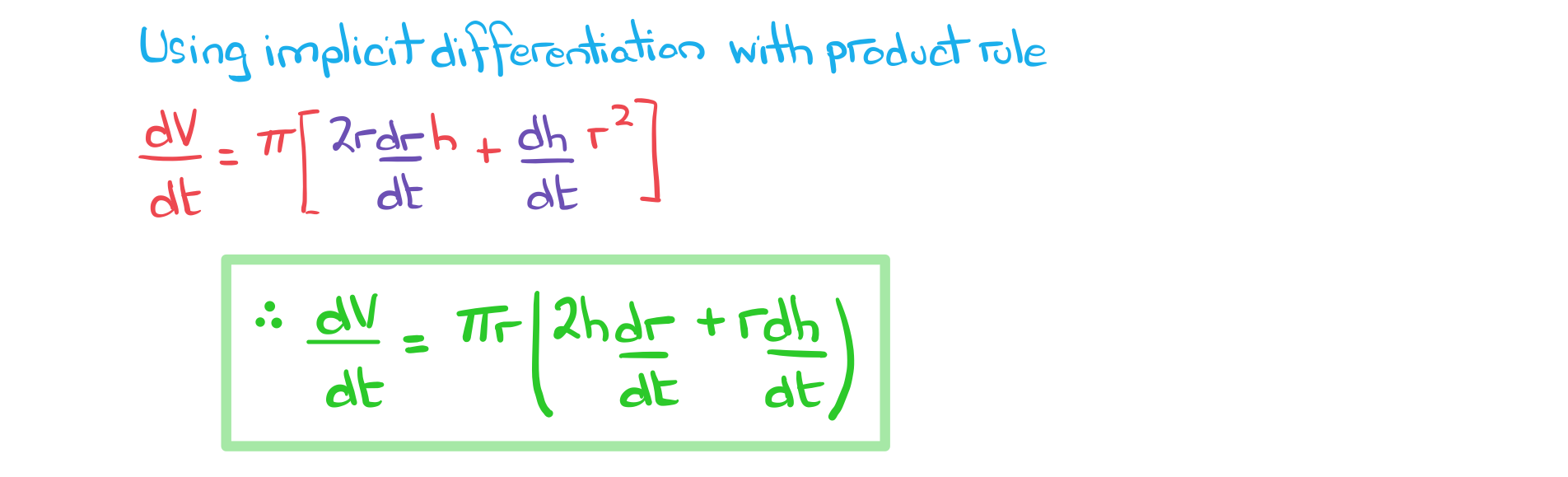
Worked Example

The radius, $r$ cm, and height, $h$ cm, of a cylinder are increasing with time. The volume, $V$ cm³, of the cylinder at time $t$ seconds is given by $V = π r^{2} h$ .

a) Find an expression for $\frac{d V}{d t}$ .

5-8-2-ib-hl-aa-only-we2a-soltn

At time $T$ seconds, the radius of the cylinder is 4 cm, expanding at a rate of 2 cm s^-1. At the same time, the height of the cylinder is 10 cm, expanding at a rate of 3 cm s^-1.

Find the rate at which the volume is expanding at time $T$ seconds.

5-8-2-ib-hl-aa-only-we2b-soltn

DP IB Maths: AA HL

Revision Notes

5.8.3 Implicit Differentiation

Implicit Differentiation

What is implicit differentiation?

Applications of Implicit Differentiation

What type of problems could involve implicit differentiation?

How do I apply implicit differentiation to algebraic problems?

Exam Tip

Worked Example

How do I apply implicit differentiation to optimisation problems?

Exam Tip

Worked Example

Author: Paul

Resources

Members

Company

Quick Links