5.11.2 Maclaurin Series from Differential Equations

If you have a differential equation of the form $\frac{d y}{d x} = g (x, y)$ along with the value of $y (0)$ it is possible to build up the Maclaurin series of the solution $y = f (x)$ term by term

This does not necessarily tell you the explicit function of $x$ that corresponds to the Maclaurin series you are finding
But the Maclaurin series you find is the exact Maclaurin series for the solution to the differential equation

The Maclaurin series can be used to approximate the value of the solution y = f(x) for different values of $x$

You can increase the accuracy of this approximation by calculating additional terms of the Maclaurin series for higher powers of $x$

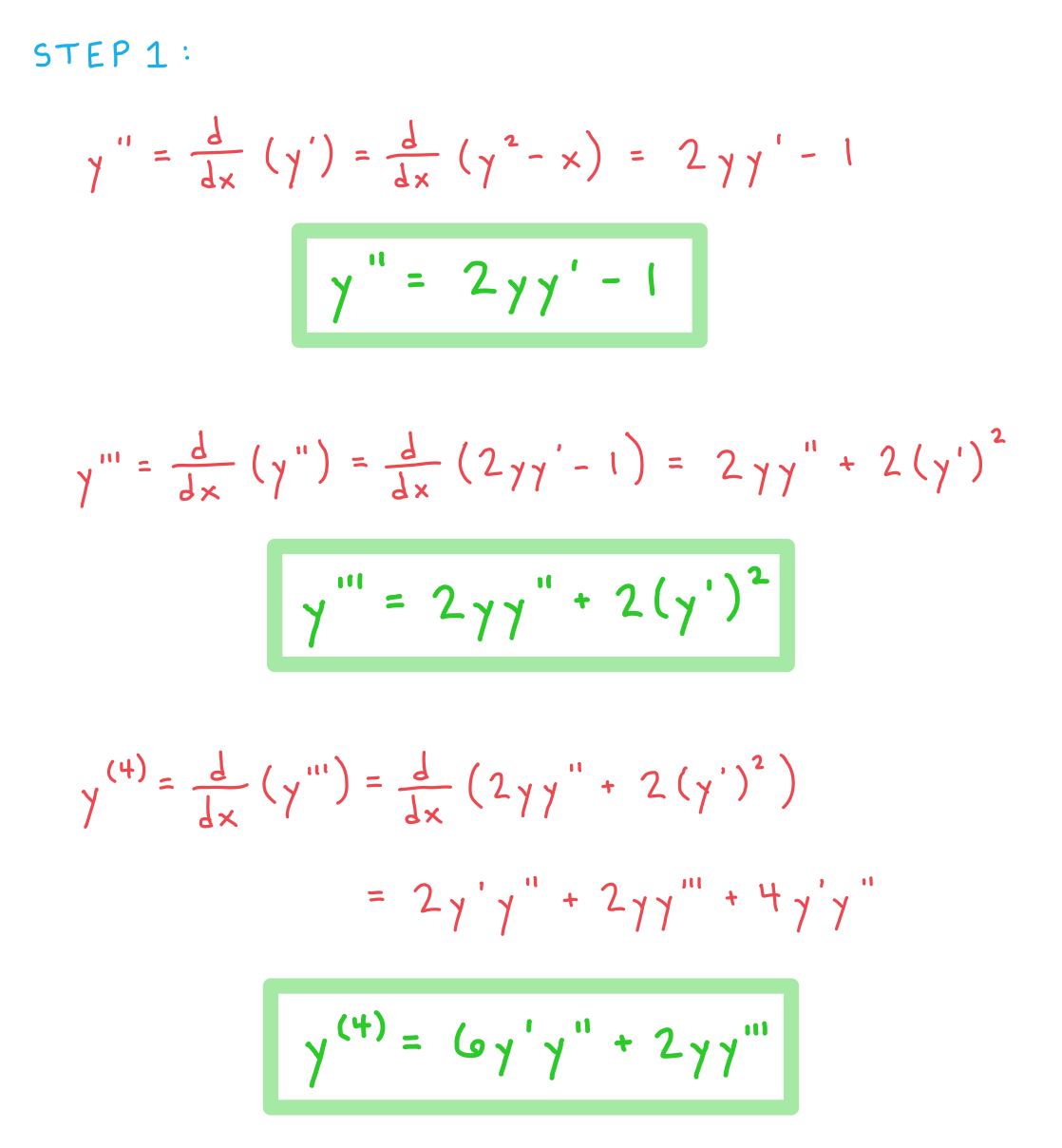
STEP 1: Use implicit differentiation to find expressions for $y'', y'''$ etc., in terms of $x, y$ and lower-order derivatives of $y$
- The number of derivatives you need to find depends on how many terms of the Maclaurin series you want to find
- For example, if you want the Maclaurin series up to the term, then you will need to find derivatives up to $y^{(4)}$ (the fourth derivative of $y$ )
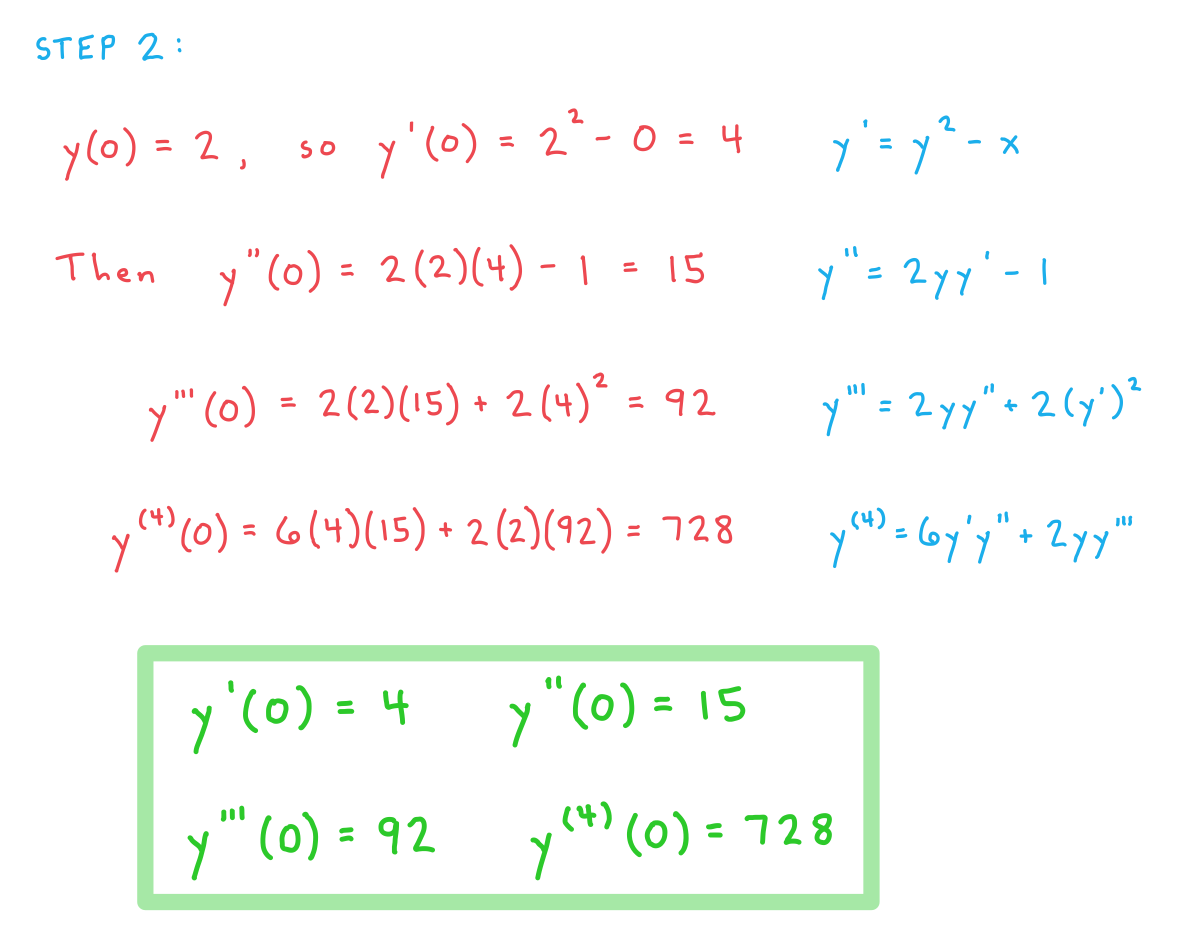
STEP 2: Using the given initial value for $y (0)$ , find the values of $y' (0), y'' (0), y''' (0),$ etc., one by one
- Each value you find will then allow you to find the value for the next higher derivative
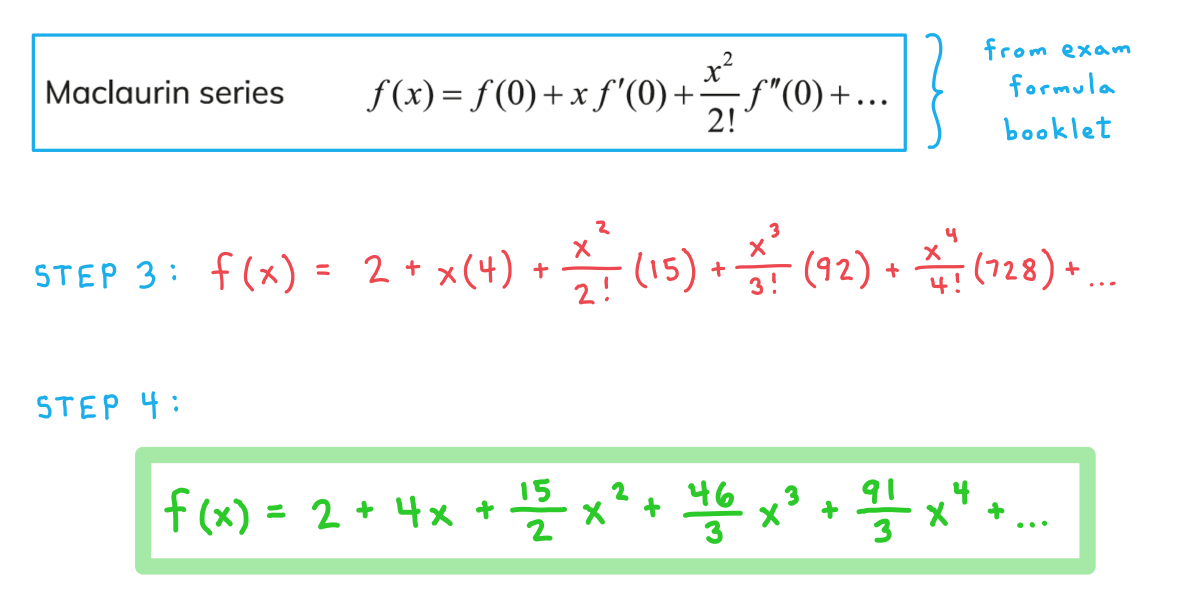
STEP 3: Put the values found in STEP 2 into the general Maclaurin series formula

$f (x) = f (0) + x f' (0) + \frac{x^{2}}{2!} f'' (0) + . . .$

- This formula is in your exam formula booklet
- $y = f (x)$ is the solution to the differential equation, so $y (0)$ corresponds to $f (0)$ in the formula, $y' (0)$ corresponds to $f' (0)$ , and so on
STEP 4: Simplify the coefficients for each of the powers of $x$ in the resultant Maclaurin series

Consider the differential equation $y' = y^{2} - x$ with the initial condition $y (0) = 2$ .

Use implicit differentiation to find expressions for

y''

y'''

and

y^{(4)}

5-11-2-ib-aa-hl-maclaurin-series-from-diff-eqns-a-we-solution

Use the given initial condition to find the values of

y' (0), y'' (0), y''' (0)

and

y^{(4)} = 0

5-11-2-ib-aa-hl-maclaurin-series-from-diff-eqns-b-we-solution

Let $y = f (x)$ be the solution to the differential equation with the given initial condition.

Find the first five terms of the Maclaurin series for

f (x)

5-11-2-ib-aa-hl-maclaurin-series-from-diff-eqns-c-we-solution

DP IB Maths: AA HL

5.11.2 Maclaurin Series from Differential Equations