| Date | May 2013 | Marks available | 3 | Reference code | 13M.2.sl.TZ2.3 |
| Level | SL only | Paper | 2 | Time zone | TZ2 |
| Command term | Find | Question number | 3 | Adapted from | N/A |
Question
The following diagram shows a triangle ABC.
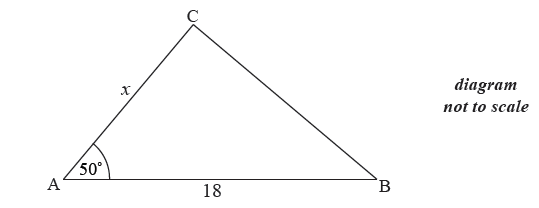
The area of triangle ABC is 80 cm2 , AB =18 cm , AC =x cm and BˆAC=50∘ .
Find x .
Find BC.
Markscheme
correct substitution into area formula (A1)
eg 12(18x)sin50
setting their area expression equal to 80 (M1)
eg 9xsin50=80
x=11.6 A1 N2
[3 marks]
evidence of choosing cosine rule (M1)
eg c2=a2+b2+2absinC
correct substitution into right hand side (may be in terms of x) (A1)
eg 11.62+182−2(11.6)(18)cos50
BC =13.8 A1 N2
[3 marks]
Examiners report
The vast majority of candidates were very successful with this question. A small minority drew an altitude from C and used right triangle trigonometry. Errors included working in radian mode, assuming that the angle at C was 90∘, and incorrectly applying the order of operations when evaluating the cosine rule.
The vast majority of candidates were very successful with this question. A small minority drew an altitude from C and used right triangle trigonometry. Errors included working in radian mode, assuming that the angle at C was 90∘, and incorrectly applying the order of operations when evaluating the cosine rule.

