| Date | May 2008 | Marks available | 3 | Reference code | 08M.2.sl.TZ1.2 |
| Level | SL only | Paper | 2 | Time zone | TZ1 |
| Command term | Find | Question number | 2 | Adapted from | N/A |
Question
The diagram below shows triangle PQR. The length of [PQ] is 7 cm , the length of [PR] is 10 cm , and PˆQR is 75∘ .
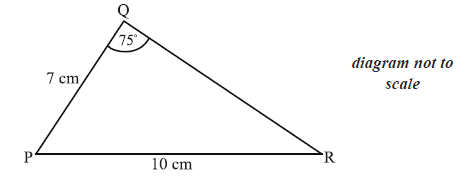
Find PˆRQ .
Find the area of triangle PQR.
Markscheme
choosing sine rule (M1)
correct substitution sinR7=sin75∘10 A1
sinR=0.676148…
PˆRQ=42.5∘ A1 N2
[3 marks]
P=180−75−R
P=62.5 (A1)
substitution into any correct formula A1
e.g. area ΔPQR=12×7×10×sin(their P)
=31.0 (cm2) A1 N2
[3 marks]
Examiners report
This question was well done with most students using the law of sines to find the angle.
In part (b), the most common error occurred when angle R or 75 degrees was used to find the area. This particular question was the most common place to incur an accuracy penalty.

