| Date | May 2013 | Marks available | 2 | Reference code | 13M.2.sl.TZ1.8 |
| Level | SL only | Paper | 2 | Time zone | TZ1 |
| Command term | Find | Question number | 8 | Adapted from | N/A |
Question
The diagram shows a circle of radius 8 metres. The points ABCD lie on the circumference of the circle.
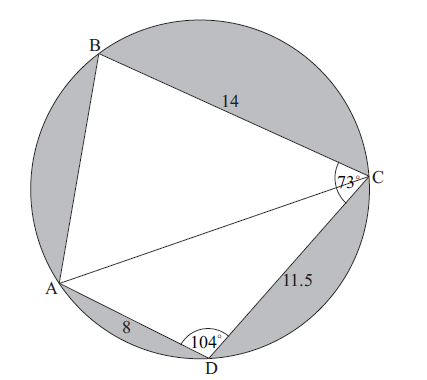
BC = 14 m, CD = 11.5 m, AD = 8 m, AˆDC=104∘ , and BˆCD=73∘ .
Find AC.
(i) Find AˆCD .
(ii) Hence, find AˆCB .
Find the area of triangle ADC.
(c) Find the area of triangle ADC.
(d) Hence or otherwise, find the total area of the shaded regions.
Hence or otherwise, find the total area of the shaded regions.
Markscheme
evidence of choosing cosine rule (M1)
eg c2=a2+b2−2abcosC , CD2+AD2−2×CD×ADcosD
correct substitution A1
eg 11.52+82−2×11.5×8cos104 , 196.25−184cos104
AC =15.5 (m) A1 N2
[3 marks]
(i) METHOD 1
evidence of choosing sine rule (M1)
eg sinAa=sinBb , sinAˆCDAD=sinDAC
correct substitution A1
eg sinAˆCD8=sin10415.516…
AˆCD=30.0∘ A1 N2
METHOD 2
evidence of choosing cosine rule (M1)
eg c2=a2+b2−2abcosC
correct substitution A1
e.g. 82=11.52+15.516…2−2(11.5)(15.516…)cosC
AˆCD=30.0∘ A1 N2
(ii) subtracting their AˆCD from 73 (M1)
eg 73−AˆCD , 70−30.017…
AˆCB=43.0∘ A1 N2
[5 marks]
correct substitution (A1)
eg area ΔADC=12(8)(11.5)sin104
area =44.6 (m2) A1 N2
[2 marks]
(c) correct substitution (A1)
eg area ΔADC=12(8)(11.5)sin104
area =44.6 (m2) A1 N2
[2 marks]
(d) attempt to subtract (M1)
eg circle−ABCD , πr2−ΔADC−ΔACB
area ΔACB=12(15.516…)(14)sin42.98 (A1)
correct working A1
eg π(8)2−44.6336…−12(15.516…)(14)sin42.98 , 64π−44.6−74.1
shaded area is 82.4 (m2) A1 N3
[4 marks]
Total [6 marks]
attempt to subtract (M1)
eg circle−ABCD , πr2−ΔADC−ΔACB
area ΔACB=12(15.516…)(14)sin42.98 (A1)
correct working A1
eg π(8)2−44.6336…−12(15.516…)(14)sin42.98 , 64π−44.6−74.1
shaded area is 82.4 (m2) A1 N3
[4 marks]
Total [6 marks]
Examiners report
There was an error on this question, where the measurements were inconsistent. Whichever method a candidate used to answer the question, the inconsistencies did not cause a problem. The markscheme included a variety of solutions based on possible combinations of solutions, and examiners were instructed to notify the IB assessment centre of any candidates adversely affected. Candidate scripts did not indicate any adverse effect.
Despite this unfortunate error, the question posed few difficulties for candidates and most approached the problem as intended. Although there were other ways to approach the problem (using properties of cyclic quadrilaterals) few considered this, likely due to the fact that cyclic quadrilaterals is not part of the syllabus.
There was an error on this question, where the measurements were inconsistent. Whichever method a candidate used to answer the question, the inconsistencies did not cause a problem. The markscheme included a variety of solutions based on possible combinations of solutions, and examiners were instructed to notify the IB assessment centre of any candidates adversely affected. Candidate scripts did not indicate any adverse effect.
Despite this unfortunate error, the question posed few difficulties for candidates and most approached the problem as intended. Although there were other ways to approach the problem (using properties of cyclic quadrilaterals) few considered this, likely due to the fact that cyclic quadrilaterals is not part of the syllabus.
There was an error on this question, where the measurements were inconsistent. Whichever method a candidate used to answer the question, the inconsistencies did not cause a problem. The markscheme included a variety of solutions based on possible combinations of solutions, and examiners were instructed to notify the IB assessment centre of any candidates adversely affected. Candidate scripts did not indicate any adverse effect.
Despite this unfortunate error, the question posed few difficulties for candidates and most approached the problem as intended. Although there were other ways to approach the problem (using properties of cyclic quadrilaterals) few considered this, likely due to the fact that cyclic quadrilaterals is not part of the syllabus. Candidates were proficient in their use of sine and cosine rules and most could find the area of the required triangle in part (c). Those who made errors in this question either had their GDC in the wrong mode or were rounding values prematurely while some misinformed candidates treated ADC as a right-angled triangle.
There was an error on this question, where the measurements were inconsistent. Whichever method a candidate used to answer the question, the inconsistencies did not cause a problem. The markscheme included a variety of solutions based on possible combinations of solutions, and examiners were instructed to notify the IB assessment centre of any candidates adversely affected. Candidate scripts did not indicate any adverse effect.
Despite this unfortunate error, the question posed few difficulties for candidates and most approached the problem as intended. Although there were other ways to approach the problem (using properties of cyclic quadrilaterals) few considered this, likely due to the fact that cyclic quadrilaterals is not part of the syllabus. Candidates were proficient in their use of sine and cosine rules and most could find the area of the required triangle in part (c). Those who made errors in this question either had their GDC in the wrong mode or were rounding values prematurely while some misinformed candidates treated ADC as a right-angled triangle. In part (d), most candidates recognized what to do and often obtained follow through marks from errors made in previous parts.
There was an error on this question, where the measurements were inconsistent. Whichever method a candidate used to answer the question, the inconsistencies did not cause a problem. The markscheme included a variety of solutions based on possible combinations of solutions, and examiners were instructed to notify the IB assessment centre of any candidates adversely affected. Candidate scripts did not indicate any adverse effect.
Despite this unfortunate error, the question posed few difficulties for candidates and most approached the problem as intended. Although there were other ways to approach the problem (using properties of cyclic quadrilaterals) few considered this, likely due to the fact that cyclic quadrilaterals is not part of the syllabus.
In part (d), most candidates recognized what to do and often obtained follow through marks from errors made in previous parts.

