| Date | May 2012 | Marks available | 2 | Reference code | 12M.2.sl.TZ2.1 |
| Level | SL only | Paper | 2 | Time zone | TZ2 |
| Command term | Find | Question number | 1 | Adapted from | N/A |
Question
The following diagram shows ΔPQR , where RQ = 9 cm, PˆRQ=70∘ and PˆQR=45∘ .
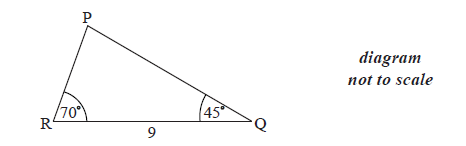
Find RˆPQ .
Find PR .
Find the area of ΔPQR .
Markscheme
RˆPQ=65∘ A1 N1
[1 mark]
evidence of choosing sine rule (M1)
correct substitution A1
e.g. PRsin45∘=9sin65∘
7.021854078
PR=7.02 A1 N2
[3 marks]
correct substitution (A1)
e.g. area=12×9×7.02…×sin70∘
29.69273008
area=29.7 A1 N2
[2 marks]
Examiners report
This question was attempted in a satisfactory manner.
The sine rule was applied satisfactory in part (b) but some obtained an incorrect answer due to having their calculators in radian mode. Some incorrect substitutions were seen, either by choosing an incorrect side or substituting 70 instead of sin70∘ . Approaches using a combination of the cosine rule and/or right-angled triangle trigonometry were seen.
Approaches using a combination of the cosine rule and/or right-angled triangle trigonometry were seen, especially in part (c) to calculate the area of the triangle.
A few candidates set about finding the height, then used the formula for the area of a right-angled triangle.

