| Date | November 2015 | Marks available | 5 | Reference code | 15N.2.sl.TZ0.8 |
| Level | SL only | Paper | 2 | Time zone | TZ0 |
| Command term | Find | Question number | 8 | Adapted from | N/A |
Question
The following diagram shows the quadrilateral ABCDABCD.
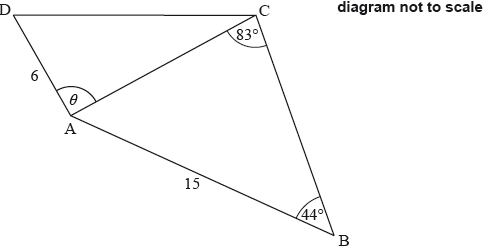
AD=6 cm, AB=15 cm,AˆBC=44∘,AˆCB=83∘andDˆAC=θAD=6 cm, AB=15 cm,A^BC=44∘,A^CB=83∘andD^AC=θ
Find ACAC.
Find the area of triangle ABCABC.
The area of triangle ACDACD is half the area of triangle ABCABC.
Find the possible values of θθ.
Given that θθ is obtuse, find CDCD.
Markscheme
evidence of choosing sine rule (M1)
egACsinCˆBA=ABsinAˆCBACsinC^BA=ABsinA^CB
correct substitution (A1)
egACsin44∘=15sin83∘ACsin44∘=15sin83∘
10.498110.4981
AC=10.5 (cm)AC=10.5 (cm) A1 N2
[3 marks]
finding CˆABC^AB (seen anywhere) (A1)
eg180∘−44∘−83∘,CˆAB=53∘180∘−44∘−83∘,C^AB=53∘
correct substitution for area of triangle ABC A1
eg12×15×10.4981×sin53∘
62.8813
area=62.9 (cm2) A1 N2
[3 marks]
correct substitution for area of triangle DAC (A1)
eg12×6×10.4981×sinθ
attempt to equate area of triangle ACD to half the area of triangle ABC (M1)
egarea ACD=12× area ABC; 2ACD=ABC
correct equation A1
eg12×6×10.4981×sinθ=12(62.9), 62.9887sinθ=62.8813, sinθ=0.998294
86.6531, 93.3468
θ=86.7∘ , θ=93.3∘ A1A1 N2
[5 marks]
Note: Note: If candidates use an acute angle from part (c) in the cosine rule, award M1A0A0 in part (d).
evidence of choosing cosine rule (M1)
egCD2=AD2+AC2−2×AD×AC×cosθ
correct substitution into rhs (A1)
egCD2=62+10.4982−2(6)(10.498)cos93.336∘
12.3921
12.4 (cm) A1 N2
[3 marks]
Total [14 marks]

