| Date | November 2016 | Marks available | 2 | Reference code | 16N.1.sl.TZ0.8 |
| Level | SL only | Paper | 1 | Time zone | TZ0 |
| Command term | Write down | Question number | 8 | Adapted from | N/A |
Question
Let →OA=(−104) and →OB=(413).
The point C is such that →AC=(−11−1).
The following diagram shows triangle ABC. Let D be a point on [BC], with acute angle ADC=θ.
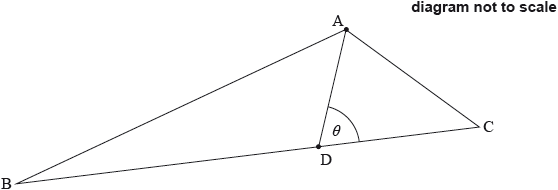
(i) Find →AB.
(ii) Find |→AB|.
Show that the coordinates of C are (−2, 1, 3).
Write down an expression in terms of θ for
(i) angle ADB;
(ii) area of triangle ABD.
Given that area ΔABDarea ΔACD=3, show that BDBC=34.
Hence or otherwise, find the coordinates of point D.
Markscheme
(i) valid approach to find →AB
eg→OB−→OA, (4−(−1)1−03−4)
→AB=(51−1) A1 N2
(ii) valid approach to find |→AB| (M1)
eg√(5)2+(1)2+(−1)2
|→AB|=√27 A1 N2
[4 marks]
correct approach A1
eg→OC=(−11−1)+(−104)
C has coordinates (−2, 1, 3) AG N0
[1 mark]
(i) AˆDB=π−θ,ˆD=180−θ A1 N1
(ii) any correct expression for the area involving θ A1 N1
egarea=12×AD×BD×sin(180−θ), 12absinθ, 12|→DA||→DB|sin(π−θ)
[2 marks]
METHOD 1 (using sine formula for area)
correct expression for the area of triangle ACD (seen anywhere) (A1)
eg12AD×DC×sinθ
correct equation involving areas A1
eg12AD×BD×sin(π−θ)12AD×DC×sinθ=3
recognizing that sin(π−θ)=sinθ (seen anywhere) (A1)
BDDC=3 (seen anywhere) (A1)
correct approach using ratio A1
eg3→DC+→DC=→BC, →BC=4→DC
correct ratio BDBC=34 AG N0
METHOD 2 (Geometric approach)
recognising ΔABD and ΔACD have same height (A1)
eguse of h for both triangles, 12BD×h12CD×h=3
correct approach A2
egBD=3x and DC=x, BDDC=3
correct working A2
egBC=4x, BD+DC=4DC, BDBC=3x4x, BDBC=3DC4DC
BDBC=34 AG N0
[5 marks]
correct working (seen anywhere) (A1)
eg→BD=34→BC, →OD=→OB+34(−600), →CD=14→CB
valid approach (seen anywhere) (M1)
eg→OD=→OB+→BD, →BC=(−600)
correct working to find x-coordinate (A1)
eg(413)+34(−600), x=4+34(−6), −2+14(6)
D is (−12, 1, 3) A1 N3
[4 marks]

