Let $f(x) = \cos x$, for $0$ $\le$ $x$ $\le$ $2\pi$. The following diagram shows the graph of $f$.

There are $x$-intercepts at $x = \frac{\pi }{2},{\text{ }}\frac{{3\pi }}{2}$.

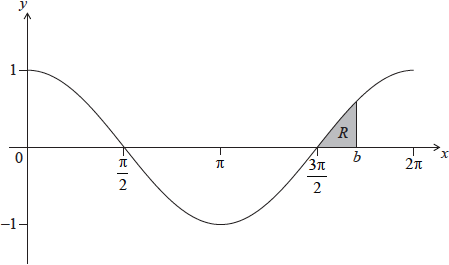
The shaded region $R$ is enclosed by the graph of $f$, the line $x = b$, where $b > \frac{{3\pi }}{2}$, and the $x$-axis. The area of $R$ is $\left( {1 - \frac{{\sqrt 3 }}{2}} \right)$. Find the value of $b$.

attempt to set up integral (accept missing or incorrect limits and missing ${\text{d}}x$)     M1

eg$\;\;\;\int_{\frac{{3\pi }}{2}}^b {\cos x{\text{d}}x,{\text{ }}\int_a^b {\cos x{\text{d}}x,{\text{ }}\int_{\frac{{3\pi }}{2}}^b {f{\text{d}}x,{\text{ }}\int {\cos x} } } }$

correct integration (accept missing or incorrect limits)     (A1)

eg$\;\;\;[\sin x]_{\frac{{3\pi }}{2}}^b,{\text{ }}\sin x$

substituting correct limits into their integrated function and subtracting (in any order)     (M1)

eg$\;\;\;\sin b - \sin \left( {\frac{{3\pi }}{2}} \right),{\text{ }}\sin \left( {\frac{{3\pi }}{2}} \right) - \sin b$

$\sin \left( {\frac{{3\pi }}{2}} \right) =  - 1\;\;\;$(seen anywhere)     (A1)

setting their result from an integrated function equal to $\left( {1 - \frac{{\sqrt 3 }}{2}} \right)$     M1

eg$\;\;\;\sin b =  - \frac{{\sqrt 3 }}{2}$

evaluating ${\sin ^{ - 1}}\left( {\frac{{\sqrt 3 }}{2}} \right) = \frac{\pi }{3}$ or ${\sin ^{ - 1}}\left( { - \frac{{\sqrt 3 }}{2}} \right) =  - \frac{\pi }{3}$     (A1)

eg$\;\;\;b = \frac{\pi }{3},{\text{ }} - 60^\circ$

identifying correct value     (A1)

eg$\;\;\;2\pi  - \frac{\pi }{3},{\text{ }}360 - 60$

$b = \frac{{5\pi }}{3}$     A1     N3

[8 marks]

Most candidates recognised that a definite integral was required and many were able to set up a correct equation. Incorrect integration leading to $- \sin x$ was quite common and poor notation was frequently seen. Some candidates appeared to guess their value from the graph, showing little supporting work.