| Date | May 2009 | Marks available | 7 | Reference code | 09M.1.sl.TZ1.10 |
| Level | SL only | Paper | 1 | Time zone | TZ1 |
| Command term | Find | Question number | 10 | Adapted from | N/A |
Question
Let f(x)=axx2+1f(x)=axx2+1 , −8≤x≤8−8≤x≤8 , a∈R .The graph of f is shown below.
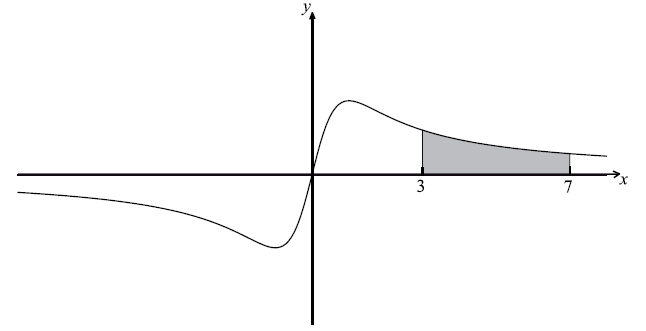
The region between x=3 and x=7 is shaded.
Show that f(−x)=−f(x) .
Given that f″(x)=2ax(x2−3)(x2+1)3 , find the coordinates of all points of inflexion.
It is given that ∫f(x)dx=a2ln(x2+1)+C .
(i) Find the area of the shaded region, giving your answer in the form plnq .
(ii) Find the value of ∫842f(x−1)dx .
Markscheme
METHOD 1
evidence of substituting −x for x (M1)
f(−x)=a(−x)(−x)2+1 A1
f(−x)=−axx2+1 (=−f(x)) AG N0
METHOD 2
y=−f(x) is reflection of y=f(x) in x axis
and y=f(−x) is reflection of y=f(x) in y axis (M1)
sketch showing these are the same A1
f(−x)=−axx2+1 (=−f(x)) AG N0
[2 marks]
evidence of appropriate approach (M1)
e.g. f″(x)=0
to set the numerator equal to 0 (A1)
e.g. 2ax(x2−3)=0 ; (x2−3)=0
(0, 0) , (√3,a√34) , (−√3,−a√34) (accept x=0 , y=0 etc) A1A1A1A1A1 N5
[7 marks]
(i) correct expression A2
e.g. [a2ln(x2+1)]73 , a2ln50−a2ln10 , a2(ln50−ln10)
area = a2ln5 A1A1 N2
(ii) METHOD 1
recognizing the shift that does not change the area (M1)
e.g. ∫84f(x−1)dx=∫73f(x)dx , a2ln5
recognizing that the factor of 2 doubles the area (M1)
e.g. ∫842f(x−1)dx=2∫84f(x−1)dx (=2∫73f(x)dx)
∫842f(x−1)dx=aln5 (i.e. 2× their answer to (c)(i)) A1 N3
METHOD 2
changing variable
let w=x−1 , so dwdx=1
2∫f(w)dw=2a2ln(w2+1)+c (M1)
substituting correct limits
e.g. [aln[(x−1)2+1]]84 , [aln(w2+1)]73 , aln50−aln10 (M1)
∫842f(x−1)dx=aln5 A1 N3
[7 marks]
Examiners report
Part (a) was achieved by some candidates, although brackets around the −x were commonly neglected. Some attempted to show the relationship by substituting a specific value for x . This earned no marks as a general argument is required.
Although many recognized the requirement to set the second derivative to zero in (b), a majority neglected to give their answers as ordered pairs, only writing the x-coordinates. Some did not consider the negative root.
For those who found a correct expression in (c)(i), many finished by calculating ln50−ln10=ln40 . Few recognized that the translation did not change the area, although some factored the 2 from the integrand, appreciating that the area is double that in (c)(i).

