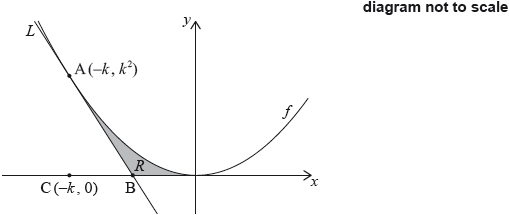
Write down $f'(x)$.

Find the gradient of $L$.

Show that the $x$-coordinate of B is $- \frac{k}{2}$.

Find the area of triangle ABC, giving your answer in terms of $k$.

Given that the area of triangle ABC is $p$ times the area of $R$, find the value of $p$.

$f'(x) = 2x$     A1     N1

[1 mark]

attempt to substitute $x =  - k$ into their derivative     (M1)

gradient of $L$ is $- 2k$     A1     N2

[2 marks]

METHOD 1 

attempt to substitute coordinates of A and their gradient into equation of a line     (M1)

eg$\,\,\,\,\,$${k^2} =  - 2k( - k) + b$

correct equation of $L$ in any form     (A1)

eg$\,\,\,\,\,$$y - {k^2} =  - 2k(x + k),{\text{ }}y =  - 2kx - {k^2}$

valid approach     (M1)

eg$\,\,\,\,\,$$y = 0$

correct substitution into $L$ equation     A1

eg$\,\,\,\,\,$$- {k^2} =  - 2kx - 2{k^2},{\text{ }}0 =  - 2kx - {k^2}$

correct working     A1

eg$\,\,\,\,\,$$2kx =  - {k^2}$

$x =  - \frac{k}{2}$     AG     N0

METHOD 2

valid approach     (M1)

eg$\,\,\,\,\,$${\text{gradient}} = \frac{{{y_2} - {y_1}}}{{{x_2} - {x_1}}},{\text{ }} - 2k = \frac{{{\text{rise}}}}{{{\text{run}}}}$

recognizing $y = 0$ at B     (A1)

attempt to substitute coordinates of A and B into slope formula     (M1)

eg$\,\,\,\,\,$$\frac{{{k^2} - 0}}{{ - k - x}},{\text{ }}\frac{{ - {k^2}}}{{x + k}}$

correct equation     A1

eg$\,\,\,\,\,$$\frac{{{k^2} - 0}}{{ - k - x}} =  - 2k,{\text{ }}\frac{{ - {k^2}}}{{x + k}} =  - 2k,{\text{ }} - {k^2} =  - 2k(x + k)$

correct working     A1

eg$\,\,\,\,\,$$2kx =  - {k^2}$

$x =  - \frac{k}{2}$     AG     N0

[5 marks]

valid approach to find area of triangle     (M1)

eg$\,\,\,\,\,$$\frac{1}{2}({k^2})\left( {\frac{k}{2}} \right)$

area of ${\text{ABC}} = \frac{{{k^3}}}{4}$     A1     N2

[2 marks]

METHOD 1 ($\int {f - {\text{triangle}}}$)

valid approach to find area from $- k$ to 0     (M1)

eg$\,\,\,\,\,$$\int_{ - k}^0 {{x^2}{\text{d}}x,{\text{ }}\int_0^{ - k} f }$

correct integration (seen anywhere, even if M0 awarded)     A1

eg$\,\,\,\,\,$$\frac{{{x^3}}}{3},{\text{ }}\left[ {\frac{1}{3}{x^3}} \right]_{ - k}^0$

substituting their limits into their integrated function and subtracting     (M1)

eg$\,\,\,\,\,$$0 - \frac{{{{( - k)}^3}}}{3}$, area from $- k$ to 0 is $\frac{{{k^3}}}{3}$

Note:     Award M0 for substituting into original or differentiated function.

attempt to find area of $R$     (M1)

eg$\,\,\,\,\,$$\int_{ - k}^0 {f(x){\text{d}}x - {\text{ triangle}}}$

correct working for $R$     (A1)

eg$\,\,\,\,\,$$\frac{{{k^3}}}{3} - \frac{{{k^3}}}{4},{\text{ }}R = \frac{{{k^3}}}{{12}}$

correct substitution into ${\text{triangle}} = pR$     (A1)

eg$\,\,\,\,\,$$\frac{{{k^3}}}{4} = p\left( {\frac{{{k^3}}}{3} - \frac{{{k^3}}}{4}} \right),{\text{ }}\frac{{{k^3}}}{4} = p\left( {\frac{{{k^3}}}{{12}}} \right)$

$p = 3$     A1     N2

METHOD 2 ($\int {(f - L)}$)

valid approach to find area of $R$     (M1)

eg$\,\,\,\,\,$$\int_{ - k}^{ - \frac{k}{2}} {{x^2} - ( - 2kx - {k^2}){\text{d}}x + \int_{ - \frac{k}{2}}^0 {{x^2}{\text{d}}x,{\text{ }}\int_{ - k}^{ - \frac{k}{2}} {(f - L) + \int_{ - \frac{k}{2}}^0 f } } }$

correct integration (seen anywhere, even if M0 awarded)     A2

eg$\,\,\,\,\,$$\frac{{{x^3}}}{3} + k{x^2} + {k^2}x,{\text{ }}\left[ {\frac{{{x^3}}}{3} + k{x^2} + {k^2}x} \right]_{ - k}^{ - \frac{k}{2}} + \left[ {\frac{{{x^3}}}{3}} \right]_{ - \frac{k}{2}}^0$

substituting their limits into their integrated function and subtracting     (M1)

eg$\,\,\,\,\,$$\left( {\frac{{{{\left( { - \frac{k}{2}} \right)}^3}}}{3} + k{{\left( { - \frac{k}{2}} \right)}^2} + {k^2}\left( { - \frac{k}{2}} \right)} \right) - \left( {\frac{{{{( - k)}^3}}}{3} + k{{( - k)}^2} + {k^2}( - k)} \right) + (0) - \left( {\frac{{{{\left( { - \frac{k}{2}} \right)}^3}}}{3}} \right)$

Note:     Award M0 for substituting into original or differentiated function.

correct working for $R$     (A1)

eg$\,\,\,\,\,$$\frac{{{k^3}}}{{24}} + \frac{{{k^3}}}{{24}},{\text{ }} - \frac{{{k^3}}}{{24}} + \frac{{{k^3}}}{4} - \frac{{{k^3}}}{2} + \frac{{{k^3}}}{3} - {k^3} + {k^3} + \frac{{{k^3}}}{{24}},{\text{ }}R = \frac{{{k^3}}}{{12}}$

correct substitution into ${\text{triangle}} = pR$     (A1)

eg$\,\,\,\,\,$$\frac{{{k^3}}}{4} = p\left( {\frac{{{k^3}}}{{24}} + \frac{{{k^3}}}{{24}}} \right),{\text{ }}\frac{{{k^3}}}{4} = p\left( {\frac{{{k^3}}}{{12}}} \right)$

$p = 3$     A1     N2

[7 marks]