| Date | November 2016 | Marks available | 2 | Reference code | 16N.2.sl.TZ0.9 |
| Level | SL only | Paper | 2 | Time zone | TZ0 |
| Command term | Find | Question number | 9 | Adapted from | N/A |
Question
A particle P starts from a point A and moves along a horizontal straight line. Its velocity v cms−1 after t seconds is given by
v(t)={−2t+2,for 0⩽
The following diagram shows the graph of v.
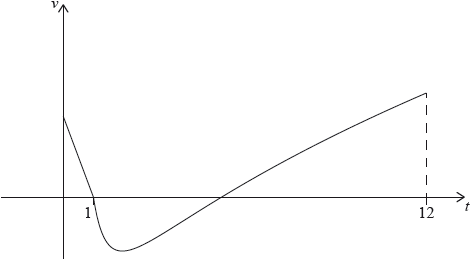
P is at rest when t = 1 and t = p.
When t = q, the acceleration of P is zero.
Find the initial velocity of P.
Find the value of p.
(i) Find the value of q.
(ii) Hence, find the speed of P when t = q.
(i) Find the total distance travelled by P between t = 1 and t = p.
(ii) Hence or otherwise, find the displacement of P from A when t = p.
Markscheme
valid attempt to substitute t = 0 into the correct function (M1)
eg\,\,\,\,\, - 2(0) + 2
2 A1 N2
[2 marks]
recognizing v = 0 when P is at rest (M1)
5.21834
p = 5.22{\text{ }}({\text{seconds}}) A1 N2
[2 marks]
(i) recognizing that a = v' (M1)
eg\,\,\,\,\,v' = 0, minimum on graph
1.95343
q = 1.95 A1 N2
(ii) valid approach to find their minimum (M1)
eg\,\,\,\,\,v(q),{\text{ }} - 1.75879, reference to min on graph
1.75879
speed = 1.76{\text{ }}(c\,{\text{m}}\,{{\text{s}}^{ - 1}}) A1 N2
[4 marks]
(i) substitution of correct v(t) into distance formula, (A1)
eg\,\,\,\,\,\int_1^p {\left| {3\sqrt t + \frac{4}{{{t^2}}} - 7} \right|{\text{d}}t,{\text{ }}\left| {\int {3\sqrt t + \frac{4}{{{t^2}}} - 7{\text{d}}t} } \right|}
4.45368
distance = 4.45{\text{ }}({\text{cm}}) A1 N2
(ii) displacement from t = 1 to t = p (seen anywhere) (A1)
eg\,\,\,\,\, - 4.45368,{\text{ }}\int_1^p {\left( {3\sqrt t + \frac{4}{{{t^2}}} - 7} \right){\text{d}}t}
displacement from t = 0 to t = 1 (A1)
eg\,\,\,\,\,\int_0^1 {( - 2t + 2){\text{d}}t,{\text{ }}0.5 \times 1 \times 2,{\text{ 1}}}
valid approach to find displacement for 0 \leqslant t \leqslant p M1
eg\,\,\,\,\,\int_0^1 {( - 2t + 2){\text{d}}t + \int_1^p {\left( {3\sqrt t + \frac{4}{{{t^2}}} - 7} \right){\text{d}}t,{\text{ }}\int_0^1 {( - 2t + 2){\text{d}}t - 4.45} } }
- 3.45368
displacement = - 3.45{\text{ }}({\text{cm}}) A1 N2
[6 marks]

