| Date | November 2014 | Marks available | 6 | Reference code | 14N.1.sl.TZ0.6 |
| Level | SL only | Paper | 1 | Time zone | TZ0 |
| Command term | Find | Question number | 6 | Adapted from | N/A |
Question
The following diagram shows the graph of f(x)=xx2+1, for 0≤x≤4, and the line x=4.
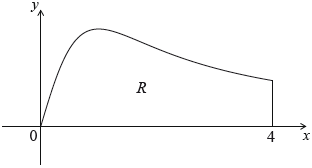
Let R be the region enclosed by the graph of f , the x-axis and the line x=4.
Find the area of R.
Markscheme
substitution of limits or function (A1)
egA=∫40f(x), ∫xx2+1dx
correct integration by substitution/inspection A2
12ln(x2+1)
substituting limits into their integrated function and subtracting (in any order) (M1)
eg12(ln(42+1)−ln(02+1))
correct working A1
eg12(ln(42+1)−ln(02+1)), 12(ln(17)−ln(1)), 12ln17−0
A=12ln(17) A1 N3
Note: Exception to FT rule. Allow full FT on incorrect integration involving a ln function.
[6 marks]
Examiners report
Very few candidates earned full marks in this question. While most candidates knew to integrate, many seemed unfamiliar with integrating using substitution or inspection. This topic is part of the syllabus, but it did not occur to many candidates to use a substitution method. A large number of them tried to integrate the individual terms in the numerator and denominator as though this were a polynomial function. While there were some candidates who knew the integral would involve a natural log function and substituted 4 and 0 into their function, many ended up with undefined values such as or did not know what to do with expressions containing ln1.

