| Date | May 2014 | Marks available | 7 | Reference code | 14M.1.sl.TZ2.10 |
| Level | SL only | Paper | 1 | Time zone | TZ2 |
| Command term | Find | Question number | 10 | Adapted from | N/A |
Question
Let f(x)=2xx2+5.
Use the quotient rule to show that f′(x)=10−2x2(x2+5)2.
Find ∫2xx2+5dx.
The following diagram shows part of the graph of f.
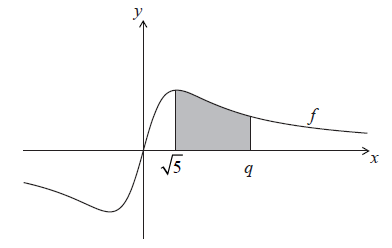
The shaded region is enclosed by the graph of f, the x-axis, and the lines x=√5 and x=q. This region has an area of ln7. Find the value of q.
Markscheme
derivative of 2x is 2 (must be seen in quotient rule) (A1)
derivative of x2+5 is 2x (must be seen in quotient rule) (A1)
correct substitution into quotient rule A1
eg (x2+5)(2)−(2x)(2x)(x2+5)2, 2(x2+5)−4x2(x2+5)2
correct working which clearly leads to given answer A1
eg 2x2+10−4x2(x2+5)2, 2x2+10−4x2x4+10x2+25
f′(x)=10−2x2(x2+5)2 AG N0
[4 marks]
valid approach using substitution or inspection (M1)
eg u=x2+5, du=2xdx, 12ln(x2+5)
∫2xx2+5dx=∫1udu (A1)
∫1udu=lnu+c (A1)
ln(x2+5)+c A1 N4
[4 marks]
correct expression for area (A1)
eg [ln(x2+5)]q√5, q∫√52xx2+5dx
substituting limits into their integrated function and subtracting (in either order) (M1)
eg ln(q2+5)−ln(√52+5)
correct working (A1)
eg ln(q2+5)−ln10, lnq2+510
equating their expression to ln7 (seen anywhere) (M1)
eg ln(q2+5)−ln10=ln7, lnq2+510=ln7, ln(q2+5)=ln7+ln10
correct equation without logs (A1)
eg q2+510=7, q2+5=70
q2=65 (A1)
q=√65 A1 N3
Note: Award A0 for q=±√65.
[7 marks]

