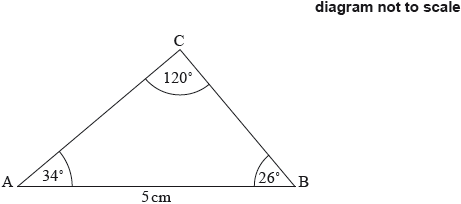
Find the length of BC.

Find the area of the postage stamp.

$\frac{{{\text{BC}}}}{{\sin 34^\circ }} = \frac{5}{{\sin 120^\circ }}$     (M1)(A1)

Note:     Award (M1) for substituted sine rule formula, (A1) for correct substitutions.

${\text{BC}} = 3.23{\text{ (cm) }}\left( {3.22850 \ldots {\text{ (cm)}}} \right)$     (A1)     (C3)

[3 marks]

$\frac{1}{2}(5)(3.22850)\sin 26^\circ$     (M1)(A1)(ft)

Note:     Award (M1) for substituted area of a triangle formula, (A1) for correct substitutions.

$= 3.54{\text{ }}({\text{c}}{{\text{m}}^2}){\text{ }}\left( {3.53820 \ldots {\text{ }}({\text{c}}{{\text{m}}^2})} \right)$     (A1)(ft)     (C3)

Note:     Follow through from part (a).

[3 marks]